Mass center of a system of a segment of a uniform circular rod(arc)
Find location of mass center of a thin uniform rod bent into shape of an arc.Solution in Gujrati
Consider a thin rod of uniform line mass density lambda(mass per unit length) and radius r subtending angle 2theta on its center 0.
The angle bisector OP is the line of symmetry, and mass center lies on it. Therefore if we assume the angle bisector as one of the coordinate axes say x-axis, y-coordinate of mass center becomes zero. Let two very small segments A and B located to the line of symmetry (x-axis). Mass center of these two segments is on P at a distance x=rcos∝ from center O.Total mass of these two elements is dm=2lambdardprop. Now using eq., we havexc=∫xdm−−−→M xc∫00(rcosα)(2λraα)λrθ=rsinθθ
Mass center of a thin uniform are shaped rod of radius r subtending angle 2theta at the center lies on its angle bisector at distance OC from the center. OC=rsinθθ
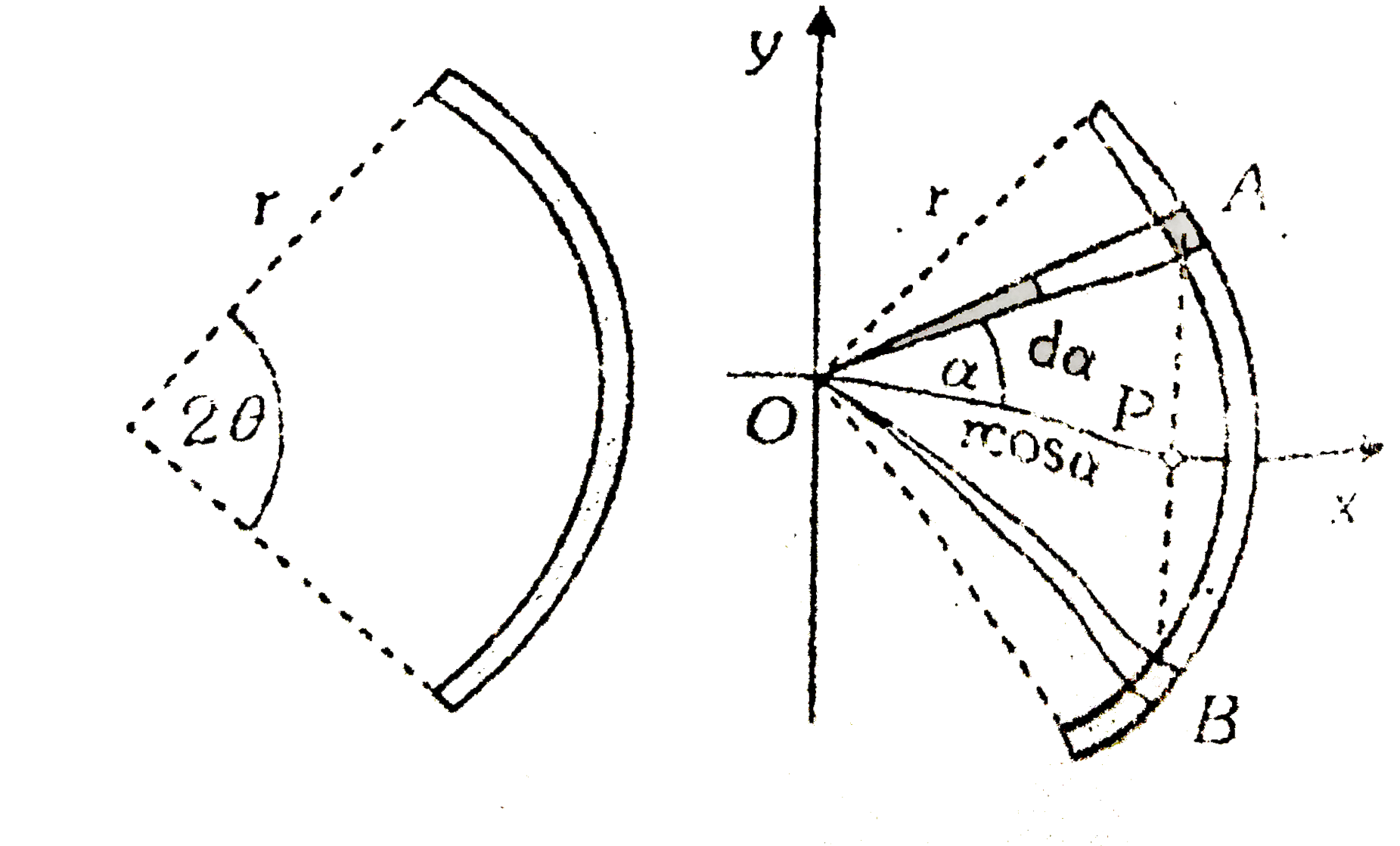
Topper's Solved these Questions
- CENTRE OF MASSBOOK - ALLEN CHAPTER - CENTRE OF MASSEXERCISE - EXERCISE-I
40 Videos
- CENTRE OF MASSBOOK - ALLEN CHAPTER - CENTRE OF MASSEXERCISE - EXERCISE-II
43 Videos
- BASIC MATHEMATICS USED IN PHYSICS &VECTORS BOOK - ALLEN CHAPTER - BASIC MATHEMATICS USED IN PHYSICS &VECTORS EXERCISE - EXERCISE-IV ASSERTION & REASON
11 Videos
- ELASTICITY, SURFACE TENSION AND FLUID MECHANICSBOOK - ALLEN CHAPTER - ELASTICITY, SURFACE TENSION AND FLUID MECHANICSEXERCISE - Exercise 5 B (Integer Type Questions)
3 Videos
Similar Questions
The centre of mass of a non uniform rod of length L whoose mass per unit length varies as p=kx2/L, (where k is a constant and x is the distance measured form one end) is at the following distances from the same end
View SolutionTwo particle A and B start moving due to their mutual interaction only. If at any tiem 't', →aA and →aB are their respective accelerations, →vA and →vB are their respective velocities, and upto that time WA and WB are the work done on A and B respectively by the mutual force, mA and mB are their masses respectively, then which of the following is alwys correct.
View SolutionAssertion: If the initial and final positions coincide, the displacement is a null vector.
Reason: A physical quantity can not be called a vector, if its magnitude is zero.
View SolutionTwo soap bubbles A and B are kept in a closed chamber where the air is maintained at pressure 8N/m2.The radii of bubbles A and B are 2cm and 4cm, respectively. Surface tension of the soap-water used to make bubbles is 0.04N/m. Find tha ratio nB/nA, where nA and nB are the number of moles of air in bubbles A and B, respectively. [Neglect the effect of gravity.]
View SolutionA uniform thin rod is bent in the form of closed loop ABCDEFA as shown in the figure. The y- coordinate of the centre of mass of the system is
View SolutionMass Center of a sector of a uniform circular plate Find location of mass center of a sector of a thin uniform plate.
View SolutionA sector cut from a uniform disk of radius 12cm and a uniform rod of the same mass bent into shape of an arc are arranged facing each other as shown in the figure. If center of mass of the combination is at the origin, what is the raius of the arc?
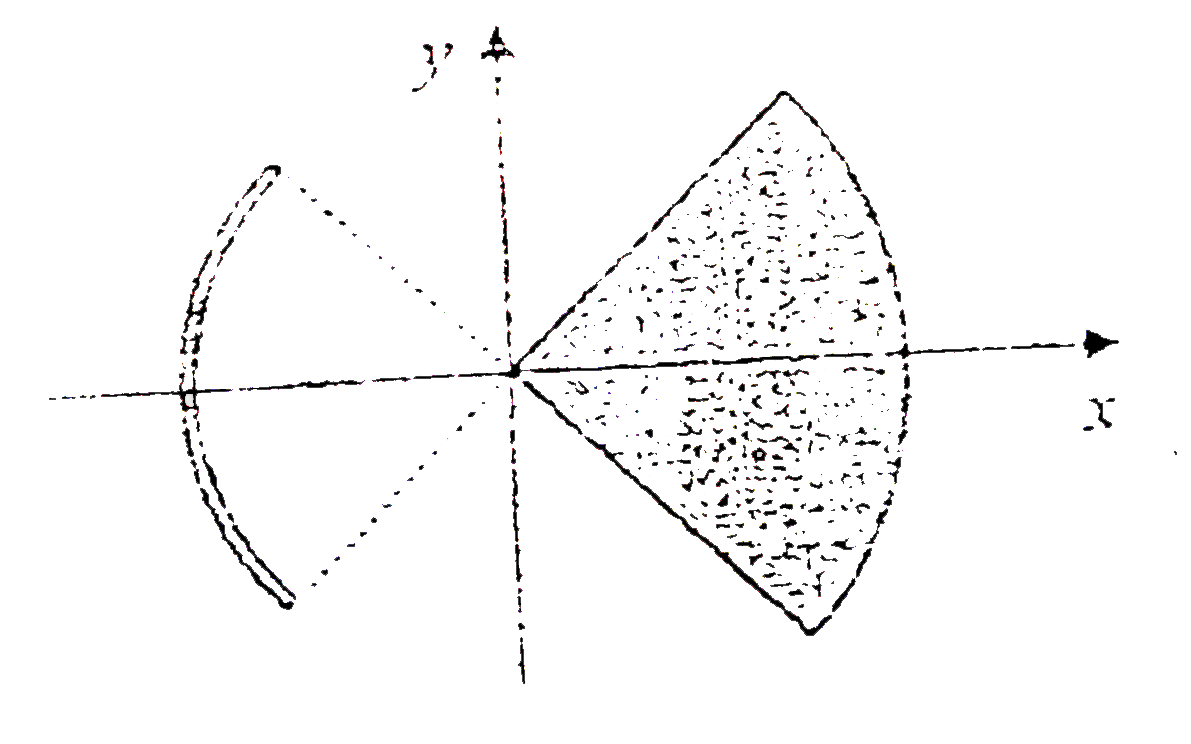
View SolutionFigure shows a composite system of two uniform rods of lengths as indicated. Then the coordinate of the center of mass of the system of rods are
View Solutionद्रव्यमान केन्द्र की परिभाषा दीजिए। L लम्बाई की एकसमान छड़ के द्रव्यमान केन्द्र की स्थिति का निगमन कीजिए ।
View Solutionएकसमान पतली छड़ का द्रव्यमान केन्द्र कहाँ होता है ?
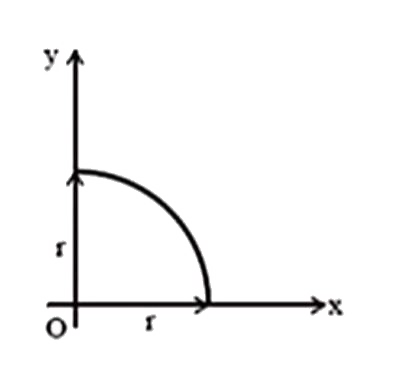
View SolutionThe coordinates of the center of mass of the following quarter circular arc are
View SolutionA thick uniform wire is bent into the shape of the letter “U” as shown. Which point indicates the location of the center of mass of this wire?
View SolutionThe center of mass is located at position i EX.5.4. Locate the center of mass of a uniform rod of mass M and length
View Solutionएक समान पतली छड़ के द्रव्यमान केन्द्र की स्थिति लिखिए।
View SolutionFind out the location of centre of mass of a uniform rod.

View SolutionFigure shows a composite system of two uniform rods of lengths as indicated. Then the coordinate of the center of mass of the system of rods are
View Solution
