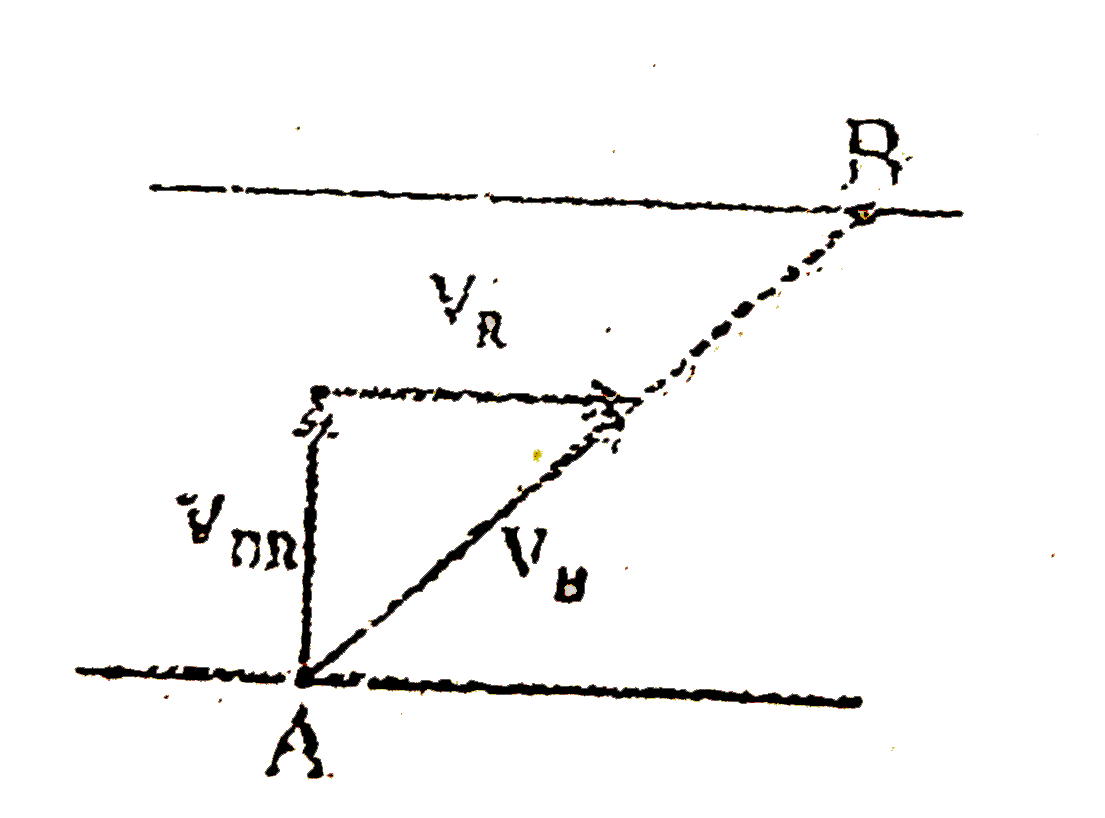
Velocity of a boat relative to river current and river current velocity are equal in magnitude. Starting from point A on the southern bank, the boatman wants to reach a point B on the nothern bank in north−east from A. In which direction he has to steer the boat?
 Solution in Gujrati
Solution in Gujrati
North−west
North
North−west
Not possible
The correct Answer is:B
Topper's Solved these Questions
- TEST PAPERBOOK - ALLEN CHAPTER - TEST PAPEREXERCISE - PHYSICS
4 Videos
- TEST PAPERBOOK - ALLEN CHAPTER - TEST PAPEREXERCISE - PHYSICS
4 Videos
- SIMPLE HARMONIC MOTIONBOOK - ALLEN CHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Example
1 Videos
- TEST PAPER 1BOOK - ALLEN CHAPTER - TEST PAPER 1EXERCISE - PHYSICS
11 Videos
Similar Questions
Choose the correct statement:
View SolutionChoose the correct statement:
View SolutionA rod with rectangular cross section oscillates about a horizontal axis passing through one of its ends and it behaves like a seconds pendulum, its length will be
View SolutionWhy are electric field lines perpendicular at a point on an equipotential surface of a conductor ?
View SolutionThe current velocity of river grows in proportion to the distance from its bank and reaches the maximum value v_0 in the middle. Near the banks the velocity is zero. A boat is moving along the river in such a manner that the boatman rows his boat always perpendicular to the current. The speed of the boat in still water is u. Find the distance through which the boat crossing the river will be carried away by the current, if the width of the river is c. Also determine the trajectory of the boat.
View SolutionA river of width a with straight parallel banks flows due north with speed u. The points O and A are on opposite banks and A is due east of O. Coordinate axes O_x and O_y are taken in the east and north directions respectively. A boat, whose speed is v relative to water, starts from O and crosses the river. If the boat is steered due east and u varies with x as : u = x(a-x) v/a^2. Find (a) equation of trajectory of the boat, (b) time taken to cross the river, (c) absolute velocity of boatman when he reaches the opposite bank, (d) the displacement of boatman when he reaches the opposite bank from the initial position.
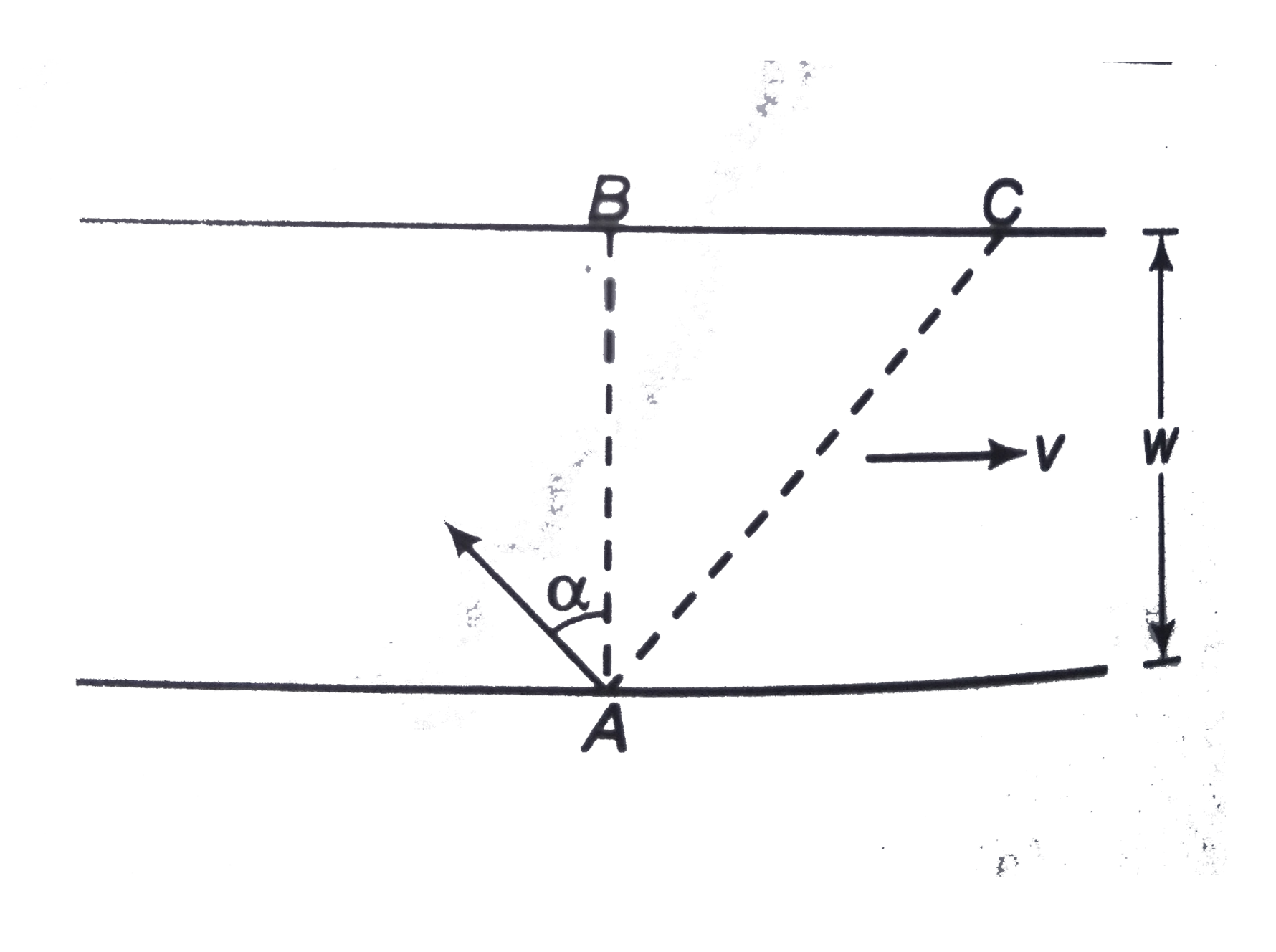
View SolutionA man in a boat crosses a river from point A. If he rows perpendicular to the banks he reaches point C (BC=120m) in 10 min. If the man heads at a certain angle α to the straight line AB (AB is perpendicular to the banks) against the current he reaches point B in 12.5 min. Find the width of the river w, the rowing velocity u, the speed of the river current v and the angle α. Assume the velocity of the boat relative to water to be constant and the same magnitude in both cases.
View SolutionA boat travels from south bank to north bank of a river with a maximum speed of 8km//h . A river current flows from west to east with a speed of 4 km/h. To arrive at a point opposite to the point of start, the boat should start at an angle:
View SolutionThe river 500 m wide is flowing with a current of 4kph. A boat starts from one bank of the river wishes to cross the river at right angle to stream direction. Boatman can row the boat at 8 kph. In which direction he should row the boat. What time he ''ll take to cross the river ?
View SolutionThe width of a rivers is 25m and in it water is flowing with a velocity of 4m/min . A boatman is standing on the bank of the river . He want to sail the boat to a point at the other bank which is directly opposite to him . In what time will he cross the river , if he can sail the boat at 8m/min. relative to the water ?
View SolutionA boat can be rowed at 5 m/s on still water. It is used to cross a 200m wide river from the south bank to the north bank. The river current has uniform velocity of 3 m/s due east.
(a) In which direction must it be steered to cross the river perpendicular to current ?
(b) How long will it take to cross the river in a direction perpendicular to the river flow ?
(c) In which direction must the boat be steered to cross the river in minimum time ? How far will it drift ?
View SolutionVelocity of the boat with respect to river is 10m/s. From point A it is steered in the direction shown. Where will it reach on the opposite bank ?

View SolutionA boat was steered over a flowing river in such a way that it reached the opposite bank following the shortest path. Time required in this case was double the time the boat would have taken to cross the river if there was no river current. If the velocity of the boat was 2 m *s^(-1) , what was the velocity of the current?
View Solutionएक नाव को धारा को प्रतिकूल दिशा में 30∘ (अभिलम्ब से) दूसरी ओर स्थित बिन्दु पर पहुचना है। नदी 5m/s के वेग से बह रही है। नाव का जल के सापेक्ष वेग 5√3m/s है। नाविक को किसी कोण पर नाव चलानी होगा।
View SolutionA river is 20m wide. River speed is 3m/s. A boat starts with velocity 2√2m/s at angle 45∘ from the river current (relative to river)
(a) Find the time taken by the boat to reach the opposite bank.
(b) How far from the point directly opposite to the starting point does the boat reach the opposite bank?
View SolutionThe current velocity of river grows in proportion to the distance from its bank and reaches the maximum value v_0 in the middle. Near the banks the velocity is zero. A boat is moving along the river in such a manner that the boatman rows his boat always perpendicular to the current. The speed of the boat in still water is u. Find the distance through which the boat crossing the river will be carried away by the current, if the width of the river is c. Also determine the trajectory of the boat.
View Solution