Question

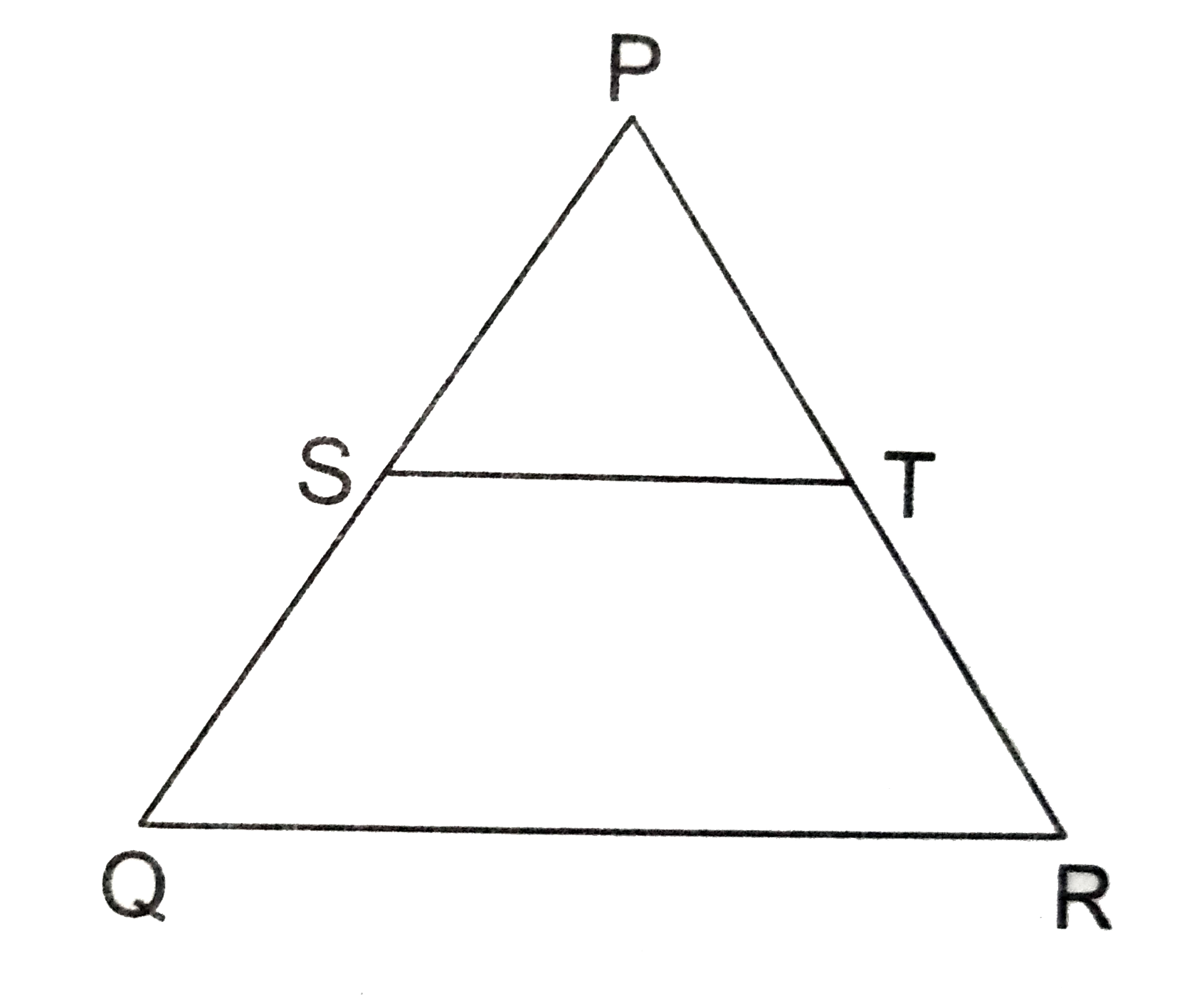
PQR is a triangle. S and T are the midpoints of the sides PQ and PR respectively. Which of the following is TRUE?
I. Triangle PST is similar to triangle PQR.
II. ST = 1/2(QR)
III. ST is parallel to QR. / ST, QR

Options

A. Only I and II
B. Only II and III
C. Only I and III
D. All I, II and III

Explanation

To solve the problem, we will analyze the triangle PQR and the midpoints S and T of sides PQ and PR respectively. We will verify each of the statements provided.

1. Identify the Triangle and Midpoints:
- Let triangle PQR be defined with points P, Q, and R.
- Let S be the midpoint of side PQ.
- Let T be the midpoint of side PR.

2. Apply the Midpoint Theorem:
- According to the Midpoint Theorem, if S and T are midpoints of sides PQ and PR respectively, then the line segment ST is parallel to the third side QR and ST is half the length of QR.
- Therefore, we can conclude:
- Statement III: ST is parallel to QR (True).
- Statement II: ST = 1/2(QR) (True).

3. Check for Similarity of Triangles:
- Since ST is parallel to QR, triangle PST is similar to triangle PQR by the Basic Proportionality Theorem (also known as Thales' theorem).
- This means that the angles of triangle PST correspond to the angles of triangle PQR.
- Therefore, we can conclude:
- Statement I: Triangle PST is similar to triangle PQR (True).

4. Conclusion:
- All three statements are true:
- I. Triangle PST is similar to triangle PQR.
- II. ST = 1/2(QR).
- III. ST is parallel to QR.

Final Answer:
All statements (I, II, and III) are true.

---