In a parallelogram ABCD, a side AB is extended to E such that BE = AB. If DE cut the line BC at Q then point Q divide BC in what ratio?
To solve the problem, we will follow these steps:
Step 1: Understand the Geometry of the Parallelogram
We have a parallelogram ABCD where AB is extended to point E such that BE = AB. This means that point E is located such that the length of BE is equal to the length of AB.
Hint: Visualize the parallelogram and the extension of side AB to point E.
Step 2: Identify Key Points and Angles
In parallelogram ABCD:
- AB is parallel to CD.
- AD is parallel to BC.
- Since BE = AB, point B is the midpoint of AE.
Hint: Remember that in a parallelogram, opposite sides are equal and parallel.
Step 3: Establish Similar Triangles
Since DE cuts BC at point Q, we can analyze triangles formed:
- Triangle EQB and triangle EDA are formed.
- Since B is the midpoint of AE, we can conclude that triangles EQB and EDA are similar by the AA (Angle-Angle) criterion (corresponding angles are equal).
Hint: Look for properties of similar triangles to establish ratios.
Step 4: Set Up Ratios from Similar Triangles
From the similarity of triangles EQB and EDA, we can set up the following ratios:
- EBEA=EQED=QBAD
Since BE = AB, we have:
- EB=AB
- EA=AB+BE=2AB
Thus, we can express the ratios:
- EBEA=AB2AB=12
This implies:
- EQED=12
- QBAD=12
Hint: Use the property of similar triangles to derive the ratios.
Step 5: Solve for the Ratio of QB to QC
From the ratio QBAD=12, we can express QB in terms of AD:
- Let QB=x and AD=2x.
Since BC = AD (opposite sides of the parallelogram are equal), we have:
- BC=2x
Now, since Q divides BC into segments QB and QC, we can express QC as:
- QC=BC−QB=2x−x=x
Thus, the ratio of QB to QC is:
- QBQC=xx=1
Conclusion
Therefore, point Q divides line segment BC in the ratio of 1:1.
Final Answer: Q divides BC in the ratio 1:1.
---
We have a parallelogram ABCD where AB is extended to point E such that BE = AB. This means that point E is located such that the length of BE is equal to the length of AB.
In parallelogram ABCD:
- AB is parallel to CD.
- AD is parallel to BC.
- Since BE = AB, point B is the midpoint of AE.
Since DE cuts BC at point Q, we can analyze triangles formed:
- Triangle EQB and triangle EDA are formed.
- Since B is the midpoint of AE, we can conclude that triangles EQB and EDA are similar by the AA (Angle-Angle) criterion (corresponding angles are equal).
From the similarity of triangles EQB and EDA, we can set up the following ratios:
- EBEA=EQED=QBAD
- EB=AB
- EA=AB+BE=2AB
- EBEA=AB2AB=12
- EQED=12
- QBAD=12
From the ratio QBAD=12, we can express QB in terms of AD:
- Let QB=x and AD=2x.
- BC=2x
- QC=BC−QB=2x−x=x
- QBQC=xx=1
Therefore, point Q divides line segment BC in the ratio of 1:1.
Topper's Solved these Questions
Similar Questions
Knowledge Check
In a parallelogram ABCD, a side AB is extended to E such that BE = AB. If DE cut the line BC at Q then point Q divide BC in what ratio?
A1:2B1:1C2:3D2:1ABCD is a parallelogram. BC is produced to Q such that BC = CQ. Then
A(a) area (ΔBCP) = area (ΔDPQ)B(b) area (ΔBCP)> area (ΔDPQ)C(c) area (ΔBCP)< area (ΔDPQ)D(d) area (ΔBCP) + area (ΔDPQ) = area (ΔBCD)In parallelogram ABCD, if P is the mid-point of side AB, then
ADP bisects diagonal ACBDP bisects diagonal BDCDP trisects diagonal BDDDP trisects diagonal AC and trisected by AC
ABCD is a parallelogram. E is a point on extended side AB such that AB = BE. By joining D to E it intersects side BC at P. Find length of PC if BC = 15 cm.
View SolutionIn the given figure, Here, ABCD is a parallellogram in which DC is extended to F such that AF intersects BC at E.
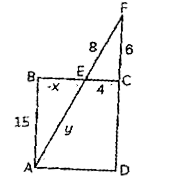
Perimeter of ΔABE=
View SolutionABCD is a cyclic quadrilateral. The side AB is extended to E in such a way that BE =BC. If ∠ADC=70∘,∠BAD=95∘, then ∠DCE is equal to
View SolutionIn triangle ABC , sides AB and AC are extended to D and E, respectively, such that AB=BD and AC =CE,F∈dDE,ifBC=6 cm.
View Solutionin ΔABC, a line parallel to side BC cuts the side AB and AC at points D and E respectively and also point D divide AB in the ratio of 1:4. If area of ΔABC is 200 cm2, then what is the area (in cm2) of quadrilateral DECB?
View Solution
