A circular planar wire loop is dipped in a soap solution and after taking it out, held with its plane vertical in air assuming thickness of film at the very small as sunlight falls on te soap film & observer receives reflected light.
Th top portion appears dark while the first colour to be observed as one moves down is red.
The top portion appears violet while the first colour to be observed as one moves down in indigo
The top portion appears dark while the first colour to be observed as one move down in violet.
the top portion appears dark while the first to be observed as one move down depends on the refractive index of the soap solution.
The correct Answer is:C
To solve the problem regarding the circular planar wire loop dipped in a soap solution, we need to analyze the behavior of light as it interacts with the soap film. Here’s a step-by-step solution:
Step 1: Understand the Setup
The circular wire loop is dipped in a soap solution and then held vertically in air. The soap film formed on the loop has varying thickness, being thicker at the top and thinner at the bottom.
Hint: Remember that the thickness of the soap film affects how light is reflected and refracted.
Step 2: Light Interaction with the Soap Film
When sunlight falls on the soap film, it reflects and refracts. The colors we see depend on the thickness of the film and the wavelength of light. Thicker films tend to reflect different colors compared to thinner films.
Hint: Consider how different wavelengths of light (colors) interact with varying thicknesses of the film.
Step 3: Color Absorption and Reflection
As the thickness of the soap film decreases from top to bottom, the colors of light will be absorbed in a specific order. The colors of visible light can be arranged from violet (shortest wavelength) to red (longest wavelength).
Hint: Recall the order of colors in the visible spectrum: Violet, Indigo, Blue, Green, Yellow, Orange, Red.
Step 4: Determine the Appearance of the Top Portion
At the top of the loop, where the film is thick, the light will reflect in such a way that it appears dark because the thicker film absorbs certain wavelengths more effectively.
Hint: Think about how thicker films can lead to destructive interference for certain wavelengths.
Step 5: Identify the First Color to be Absorbed
As you move down the loop, the first color that will be absorbed is red because it has the longest wavelength. As you continue moving down, the colors will change, and the first color that can be seen will be violet at the very bottom.
Hint: Remember that the order of absorption is influenced by the thickness of the film.
Step 6: Conclusion
Based on the analysis, the top portion of the soap film appears dark, and the first color to be absorbed as one moves down is red. Therefore, the correct option is:
Answer: The top portion appears dark while the first color to be absorbed as one moves down is red.
Final Answer
Option A: The top portion appears dark while the first color to be absorbed as one moves down is red.
---
The circular wire loop is dipped in a soap solution and then held vertically in air. The soap film formed on the loop has varying thickness, being thicker at the top and thinner at the bottom.
When sunlight falls on the soap film, it reflects and refracts. The colors we see depend on the thickness of the film and the wavelength of light. Thicker films tend to reflect different colors compared to thinner films.
As the thickness of the soap film decreases from top to bottom, the colors of light will be absorbed in a specific order. The colors of visible light can be arranged from violet (shortest wavelength) to red (longest wavelength).
At the top of the loop, where the film is thick, the light will reflect in such a way that it appears dark because the thicker film absorbs certain wavelengths more effectively.
As you move down the loop, the first color that will be absorbed is red because it has the longest wavelength. As you continue moving down, the colors will change, and the first color that can be seen will be violet at the very bottom.
Based on the analysis, the top portion of the soap film appears dark, and the first color to be absorbed as one moves down is red. Therefore, the correct option is:
Option A: The top portion appears dark while the first color to be absorbed as one moves down is red.
Topper's Solved these Questions
- WAVE OPTICSBOOK - ALLENCHAPTER - WAVE OPTICSEXERCISE - Exercise 3 (Miscellaneous type Questions)
27 Videos
- WAVE OPTICSBOOK - ALLENCHAPTER - WAVE OPTICSEXERCISE - Exercise 4 A (Conceptual subjective Exercise)
9 Videos
- WAVE OPTICSBOOK - ALLENCHAPTER - WAVE OPTICSEXERCISE - Exercise 1 (Check your Grasp)
75 Videos
- UNIT & DIMENSIONS, BASIC MATHS AND VECTOR BOOK - ALLENCHAPTER - UNIT & DIMENSIONS, BASIC MATHS AND VECTOR EXERCISE - Exercise (J-A)
7 Videos
Similar Questions
A wire forming a loop is dipped into soap solution and taken out so that a film of soap solution is formed. A loop of 6.28 cm long thread is gently put on the film and the film is pricked with a needle inside the loop. The thread loop takes the shape of a circle. Find the tension in the thread. Surface tension of soap solution =0.030Nm−1.
View SolutionA wire forming a loop is dipped into soap solution and taken out so that a film of soap solution is formed. A loop of 6.28 cm long thread is gently put on the film and the film is pricked with a needle inside the loop. The thread loop takes the shape of a circle. Find the tension in the thread. Surface tension of soap solution =0.030Nm−1.
View SolutionConsider a U-shaped frame with a sliding wire of length l and mass 'm' on its arm. It is dipped in a soap solution, taken out and placed in vertical position as shown in figure. Choose minimum value of m so that does not descent (Surface tension of soap solution is S)

View SolutionA U-shaped wire is dipped in a soap solution, and removed. A thin soap film formed between the wire and a light slider supports a weight of 1.5×10−2N (which includes the small weigh of the slider). The length of the slider is 30cm. What is the surface tension of the film?
View SolutionA thin and light thread of sufficient length L is attached to a wire frame. The frame (along with the thread) is dipped into soap solution. When the frame is taken out of the solution, the thread takes the form of a semicircle with a soap film extending between the frame and the thread as shown in the figure (a). Now the thread is deformed into two semicircles by applying a force of F at the middle of the thread, as a result the film gets strectched as shown in the figure. (b). Calculate the surface tension of the soap solution.
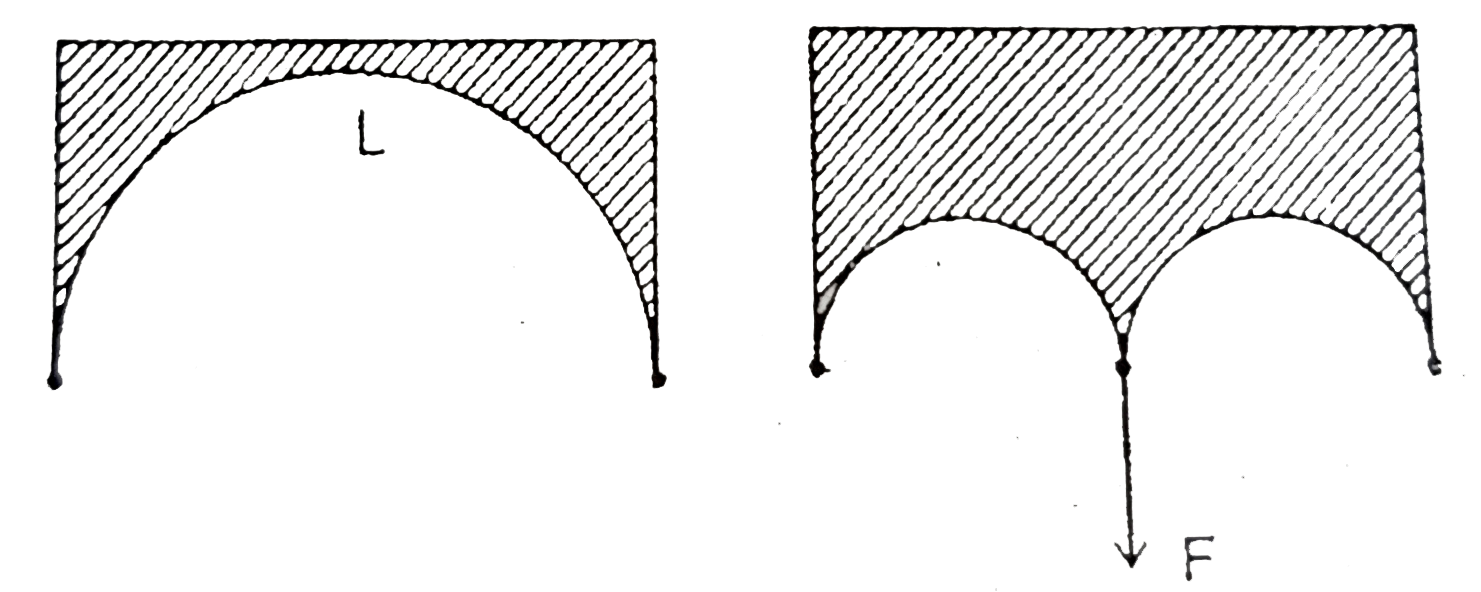
View Solution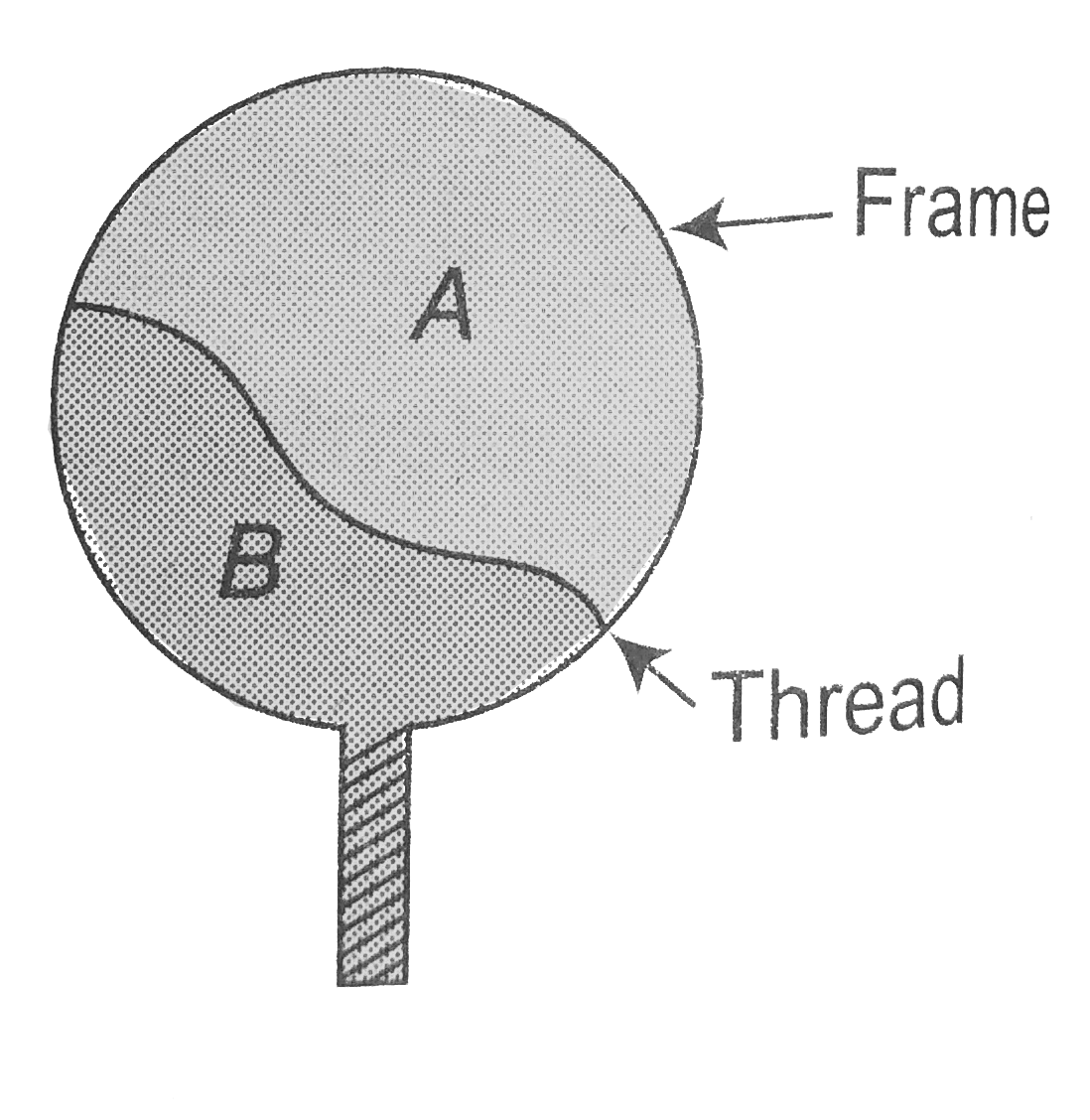
A thread is tied slightly loose to a wire frame as in figure and the frame is dipped into a soap solution and taken out . The frame is comletely covered with the film. When the portion A puntured with a pin The thread.
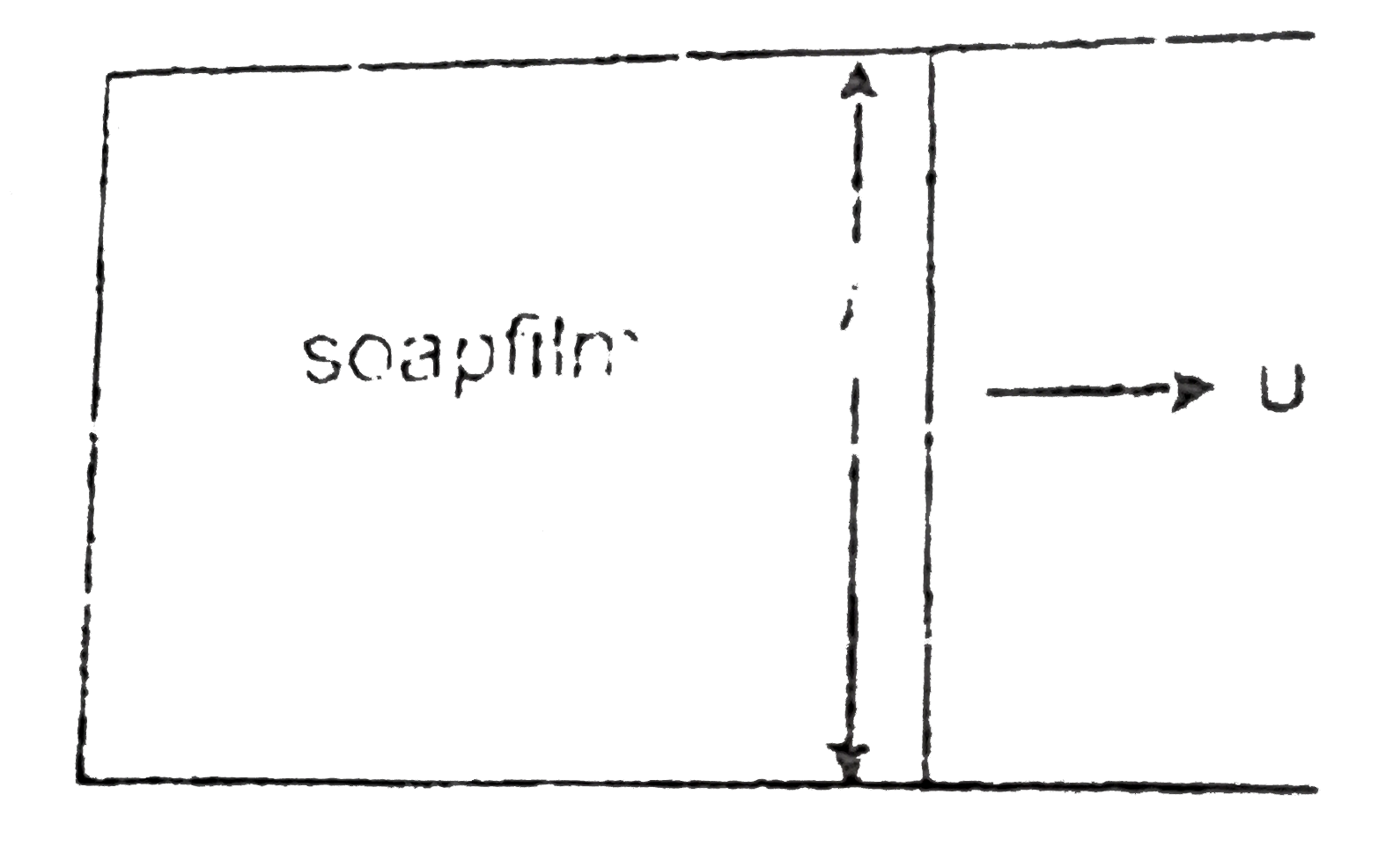
View SolutionA soap film is created in a small wire frame as shown in the figure. The sliding wire of mass m is given a velocity u to the right and assume that u is small enough so that film does not break. Plane of the film is horizontal and surface tension is T, then time to region the original position of wire is equal to :
View SolutionA soap film is formed on a wire ring held vertically and allowed to drain. A diffuse source of while light is observed by reflection in the soap film . In this connection indicate the wrong statement :
View SolutionA certain region of a soap bubble reflects red light of vacuum wavelength λ=650nm. What is the minimum thickness that this region of the soap bubble could be have? Take the index of reflection of the soap film to be 1.41.
View SolutionRefractive index of a thin soap film of a uniform thickness is 1.34. Find the smallest thickness of the film that gives in interference maximum in the reflected light when light of wavelength 5360Å fall at normal incidence.
View Solution
