A,B,C and D are the points in a vertical line such that AB=BC=CD .If a body falls from rest at A, then the times of descent through AB,BC and CD are in the ratio
1:√2:√3
√2:√3:1
√3:1:√2
1:(√2−1):(√3−√2)
The correct Answer is:D
t1=√2hg
t2=√2×2hg
t3=√2×3hg
Required ratio t1:(t2−t1):(t3−t2)
=1:(√2−1):(√3−√2)
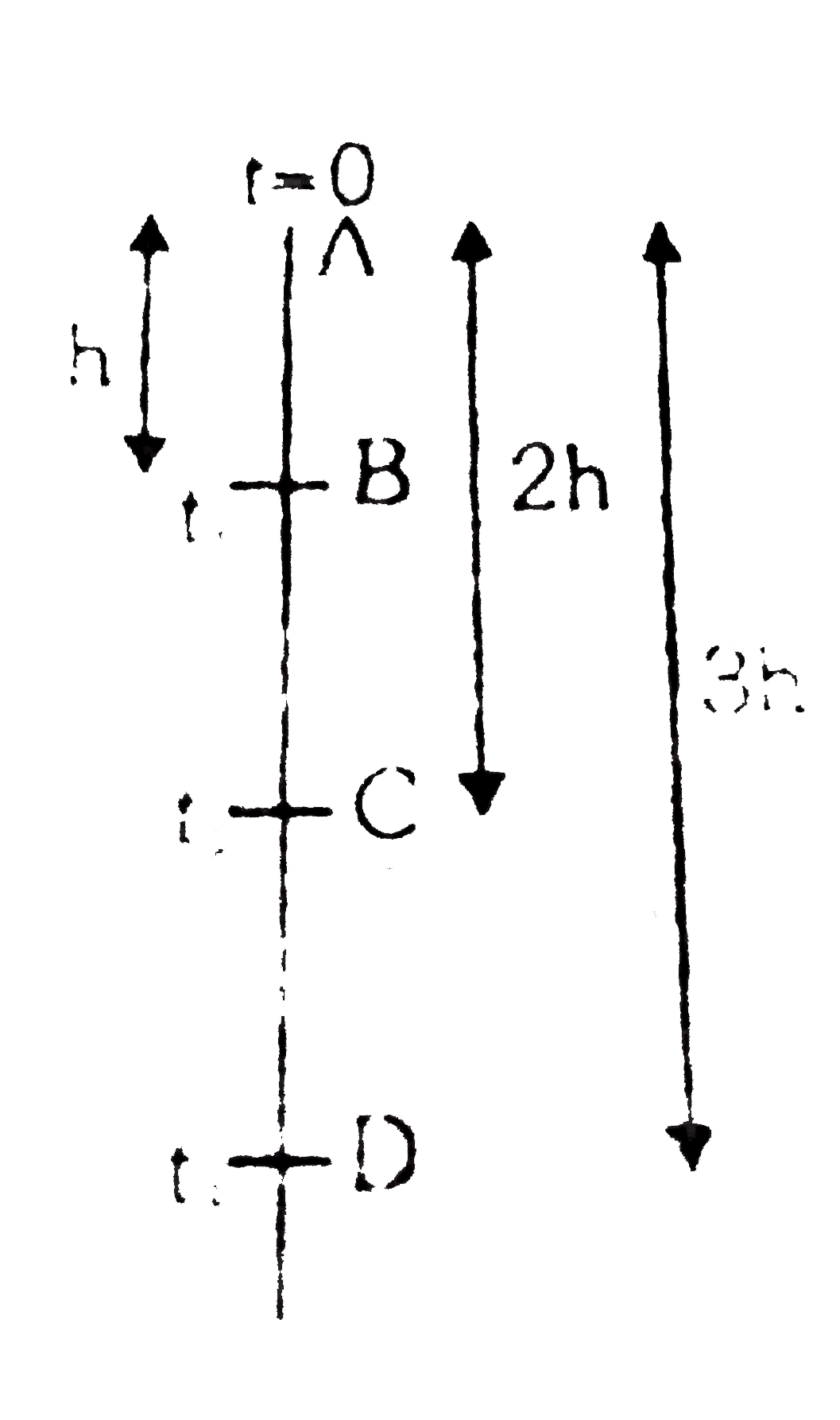
Topper's Solved these Questions
- KINEMATICSBOOK - ALLENCHAPTER - KINEMATICSEXERCISE - EXERCISE-02
57 Videos
- KINEMATICSBOOK - ALLENCHAPTER - KINEMATICSEXERCISE - EXERCISE-03
6 Videos
- KINEMATICSBOOK - ALLENCHAPTER - KINEMATICSEXERCISE - EXERCISE-2
89 Videos
- ERROR AND MEASUREMENTBOOK - ALLENCHAPTER - ERROR AND MEASUREMENTEXERCISE - Part-2(Exercise-2)(B)
22 Videos
- KINEMATICS (MOTION ALONG A STRAIGHT LINE AND MOTION IN A PLANE)BOOK - ALLENCHAPTER - KINEMATICS (MOTION ALONG A STRAIGHT LINE AND MOTION IN A PLANE)EXERCISE - BEGINNER S BOX-7
8 Videos
Similar Questions
Let A, B, C, D be points on a vertical line such that AB = BC = CD. If a body is released from position A, the times of descent through AB, BC and CD are in the ratio.
View SolutionA, B, C are points in a vertical line such that AB = BC. If a body falls freely from rest at A and t1 and t2 are times taken to travel distances AB and BC, then ratio (t2/t1) is
View Solution
Knowledge Check
Points P,Q and R are in vertical line such that PQ=QR. A ball at (P) is allowed to fall freely. What is the ratio of the times of descent through PQ and QR ?
A0.042361111111111B1 : √2C1:(√2 - 1)D1 : (√2 + 1)A, B,C and D are points on a line such that point B biscets line AC and point A bisects line CD. What is the ratio of AB to CD ?
A1/4B1/3C1/2D2/3
In the following graph, the slope of curve AB, BC and CD are
View SolutionLet A, B and C be points in a vertical line at height h, 4h5andh5 and from the ground. A particle released from rest from the point A reaches the ground in time T. The time taken by the particle to go from B to C is TBC . Then T(TBC)2=
View SolutionA body is falling freely from a point A at certain height from the ground and passes through point B, C and D (vertically as shown) so that BC=CD. The time taken by the particle to move from B to C is 2 seconds and from C to D 1 second. Time taken to move from A to B in second is
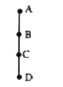
View SolutionABCD is a square. P, Q and Rare the points on AB, BC and CD respectively, such that AP = BQ = CR. Prove that:
PQ = QR
View SolutionABCD is a square. P, Q and Rare the points on AB, BC and CD respectively, such that AP = BQ = CR. Prove that:
PB = QC
View SolutionA particle is dropped from point A at a certain height from ground. It falls freely and passes through thre points B,C and D with BC=CD. The time taken by the particle to move from B to CD is 2s and from C to D is 1s. The time taken to move from A to B is s
View Solution
