Question

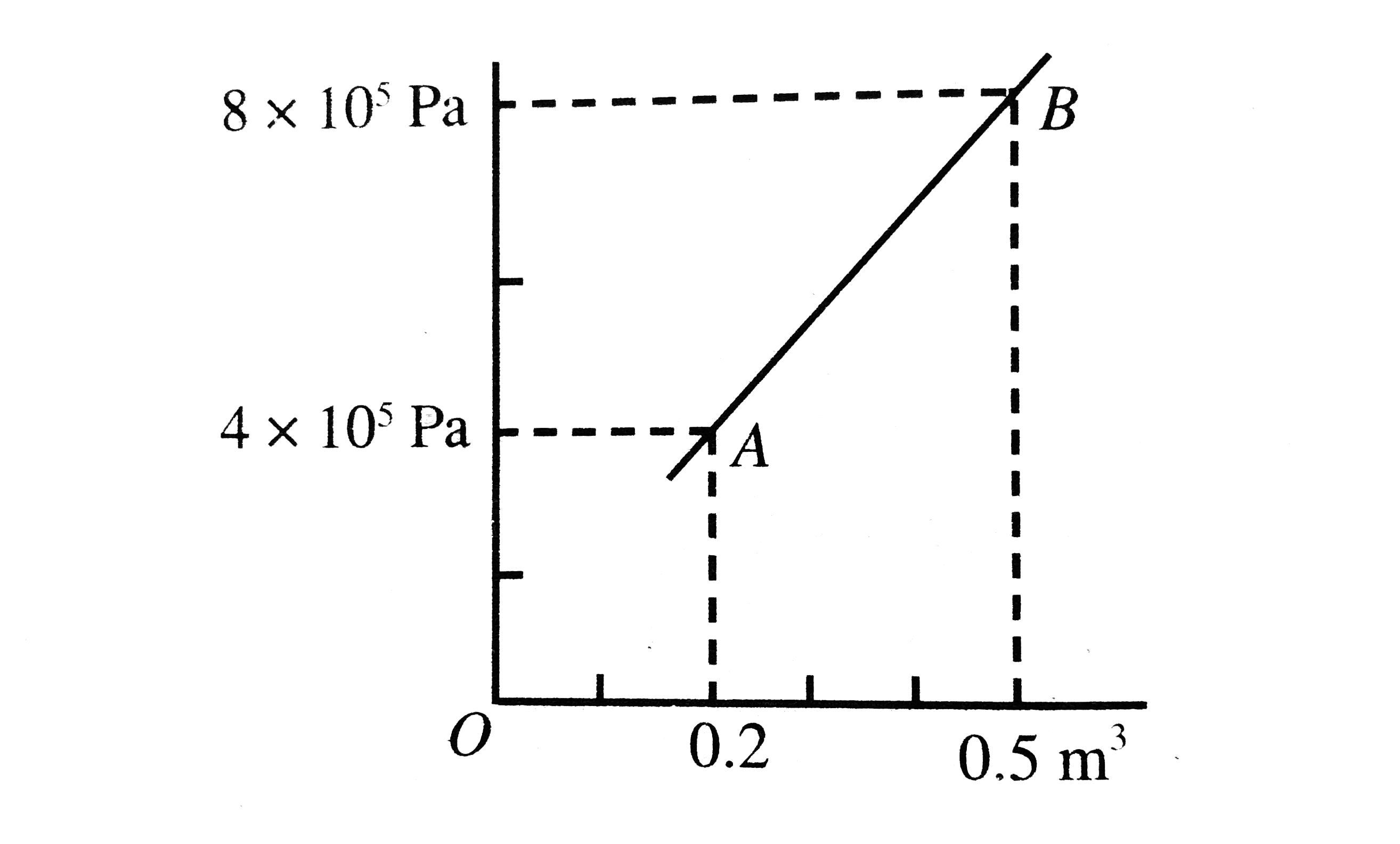
The pressure in monoatomic gas increases linearly from $=4\times {10}^{5}N{m}^{-2}$ to $8\times {10}^{+5}N{m}^{-2}$ when its volume increases from $0.2m^3$ to $0.5m^3$. Calculate .
(i) Work done by the gas ,
(ii) Increase in the internal energy,
(iii) Amount of heat supplied,
(iv) Molar heat capacity of the gas $R=8.31Jmo{l}^{-1}{K}^{-1}$

Explanation

To solve the problem step by step, let's break it down into the required parts:

Given Data:
- Initial pressure, $$P_1=4\times {10}^5{\text{N/m}}^2$$
- Final pressure, $$P_2=8\times {10}^5{\text{N/m}}^2$$
- Initial volume, $$V_1=0.2{\text{m}}^3$$
- Final volume, $$V_2=0.5{\text{m}}^3$$
- The gas is monoatomic.

(i) Work Done by the Gas

The work done by the gas during expansion can be calculated using the area under the pressure-volume graph. Since the pressure increases linearly, the shape formed is a trapezium.

1. Calculate the average pressure:
\( P_{\text{avg}} = \frac{P_1 + P_2}{2} = \frac{4 \times 10^5 + 8 \times 10^5}{2} = \frac{12 \times 10^5}{2} = 6 \times 10^5 \, \text{N/m}^2 \)

2. Calculate the work done (W):
\( W = P_{\text{avg}} \times (V_2 - V_1) = 6 \times 10^5 \times (0.5 - 0.2) = 6 \times 10^5 \times 0.3 = 1.8 \times 10^5 \, \text{J} \)

(ii) Increase in Internal Energy

The change in internal energy (\( \Delta U \)) for a monoatomic ideal gas can be calculated using the formula:
\( \Delta U = \frac{3}{2} n R \Delta T \)
However, we can also use the relationship:
\( \Delta U = P_{\text{final}} V_{\text{final}} - P_{\text{initial}} V_{\text{initial}} \)

1. Calculate \( P_{\text{final}} V_{\text{final}} \) and \( P_{\text{initial}} V_{\text{initial}} \):
\( P_{\text{final}} V_{\text{final}} = 8 \times 10^5 \times 0.5 = 4.0 \times 10^5 \, \text{J} \)
\( P_{\text{initial}} V_{\text{initial}} = 4 \times 10^5 \times 0.2 = 0.8 \times 10^5 \, \text{J} \)

2. Calculate \( \Delta U \):
\( \Delta U = 4.0 \times 10^5 - 0.8 \times 10^5 = 3.2 \times 10^5 \, \text{J} \)

(iii) Amount of Heat Supplied

The heat supplied (\( Q \)) can be calculated using the first law of thermodynamics:
\( Q = W + \Delta U \)

1. Substituting the values:
\( Q = 1.8 \times 10^5 + 3.2 \times 10^5 = 5.0 \times 10^5 \, \text{J} \)

(iv) Molar Heat Capacity

The molar heat capacity (\( C \)) can be calculated using the formula:
\( C = \frac{Q}{n \Delta T} \)
Where \( n \) is the number of moles and \( \Delta T \) is the change in temperature.

1. Calculate the number of moles (\( n \)):
Using the ideal gas law, \( PV = nRT \):
\( n = \frac{P_1 V_1}{RT} \quad \text{(we need to find \( T \) first)}
\)

2. Find \( T \) using \( P_1 \) and \( V_1 \):
Rearranging gives:
\( T = \frac{P_1 V_1}{nR} \)

3. Calculate \( C \):
Since we already have \( Q \) and \( \Delta U \), we can use the values to find \( C \):
\( C = \frac{Q}{n \Delta T} \)
We can substitute the values we have to find \( C \).

Final Answers:
- (i) Work done by the gas: \( 1.8 \times 10^5 \, \text{J} \)
- (ii) Increase in internal energy: \( 3.2 \times 10^5 \, \text{J} \)
- (iii) Amount of heat supplied: \( 5.0 \times 10^5 \, \text{J} \)
- (iv) Molar heat capacity: \( 17.14 \, \text{J/mol K} \)