Question

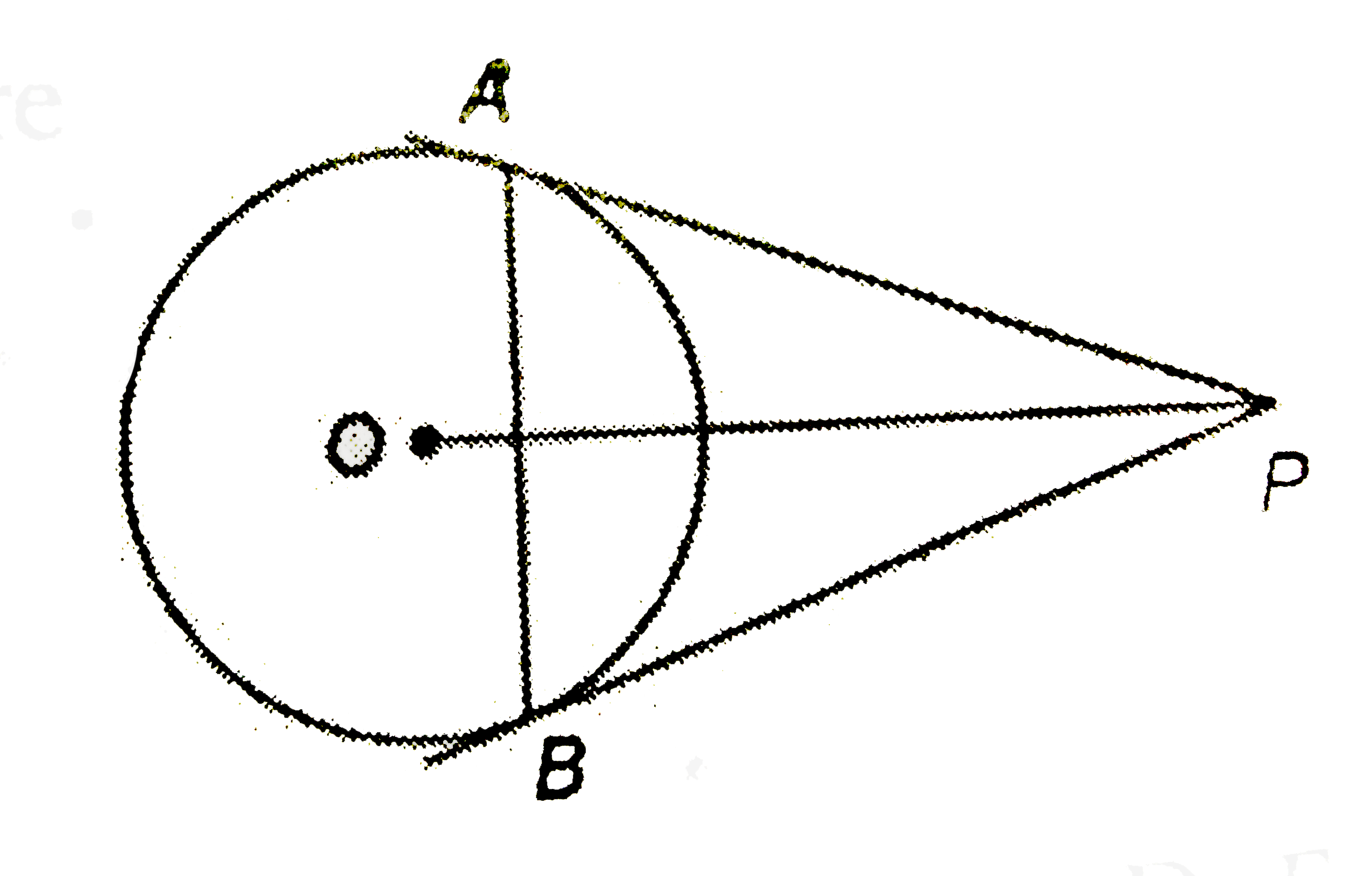
From a point P, two tangents PA and PB are drawn to a circle with centre O. If OP is equal to diameter of the circle, then $\angle APB$is

Options

A. `45^@`
B. `90^@`
C. `30^@`
D. `60^@`

Answer

D

Explanation

To solve the problem, we need to find the angle ∠APB formed by two tangents PA and PB drawn from point P to a circle with center O, given that OP is equal to the diameter of the circle.

1. Understand the Problem: We have a circle with center O and two tangents PA and PB drawn from an external point P. We know that OP (the distance from the center O to point P) is equal to the diameter of the circle.

2. Define Variables: Let the radius of the circle be R. Therefore, the diameter of the circle is 2R. Since OP = 2R, we can denote OP as the length from O to P.

3. Identify Right Angles: The radius at the point of tangency is perpendicular to the tangent line. Thus, OA (radius to point A) is perpendicular to PA, and OB (radius to point B) is perpendicular to PB. This gives us two right angles: ∠OAP = 90° and ∠OBP = 90°.

4. Use Triangle Properties: In triangle OAP, we can apply the sine function. We know:
- OA = R (radius)
- OP = 2R (diameter)

Using the sine definition:
\( \sin(\theta) = \frac{OA}{OP} = \frac{R}{2R} = \frac{1}{2} \)

5. Calculate Angle θ: The angle whose sine is \(\frac{1}{2}\) is 30°. Therefore, we have:
\( \theta = 30° \)

6. Determine Angle APB: The line OP bisects the angle ∠APB. Therefore, we can express ∠APB as:
\( \angle APB = 2 \times \theta = 2 \times 30° = 60° \)

7. Final Answer: Thus, the angle ∠APB is 60°.

Summary:
The angle ∠APB formed by the two tangents PA and PB from point P to the circle is 60°.

---