An object is placed beyond the centre of curvature C of the given concave mirror . If the distance of the object is d1 from C and the distance of the image formed is d2 from C , the radius of curvature of this mirror is
d1d2d1+d2
d1d2d1−d2
2d1d2d1−d2
2d1d2d1+d2
To solve the problem, we will use the mirror formula and the relationship between the radius of curvature and the focal length of a concave mirror.
1. Identify the Variables:
- Let the distance of the object from the center of curvature C be d1.
- Let the distance of the image from the center of curvature C be d2.
- The radius of curvature R is related to the focal length F by the equation R=2F.
2. Assign Sign Conventions:
- For a concave mirror, the object distance U is taken as negative. Therefore, U=−d1.
- The image distance V is also negative when the image is formed on the same side as the object. Thus, V=−d2.
3. Use the Mirror Formula:
- The mirror formula is given by:
1V+1U=1F
- Substituting the values of U and V:
1−d2+1−d1=1F
- This simplifies to:
−(1d2+1d1)=1F
- Therefore:
1F=−(1d2+1d1)
4. Find the Focal Length:
- Rearranging gives:
F=d1d2d1+d2
5. Calculate the Radius of Curvature:
- Using the relationship R=2F:
R=2(d1d2d1+d2)
- Thus, the radius of curvature is:
R=2d1d2d1+d2
Final Answer:
The radius of curvature R of the concave mirror is given by:
R=2d1d2d1+d2
- Let the distance of the object from the center of curvature C be d1.
- Let the distance of the image from the center of curvature C be d2.
- The radius of curvature R is related to the focal length F by the equation R=2F.
- For a concave mirror, the object distance U is taken as negative. Therefore, U=−d1.
- The image distance V is also negative when the image is formed on the same side as the object. Thus, V=−d2.
- The mirror formula is given by:
1V+1U=1F
- Substituting the values of U and V:
1−d2+1−d1=1F
- This simplifies to:
−(1d2+1d1)=1F
- Therefore:
1F=−(1d2+1d1)
- Rearranging gives:
F=d1d2d1+d2
- Using the relationship R=2F:
R=2(d1d2d1+d2)
- Thus, the radius of curvature is:
R=2d1d2d1+d2
The radius of curvature R of the concave mirror is given by:
R=2d1d2d1+d2
Topper's Solved these Questions
- JEE MAINS 2021BOOK - JEE MAINS PREVIOUS YEARCHAPTER - JEE MAINS 2021EXERCISE - Physics (Section B)
40 Videos
- JEE MAINS 2021BOOK - JEE MAINS PREVIOUS YEARCHAPTER - JEE MAINS 2021EXERCISE - Physics (Section A )
20 Videos
- JEE MAINS 2021BOOK - JEE MAINS PREVIOUS YEARCHAPTER - JEE MAINS 2021EXERCISE - Physics Section B
20 Videos
- JEE MAINS 2020BOOK - JEE MAINS PREVIOUS YEARCHAPTER - JEE MAINS 2020EXERCISE - PHYSICS
246 Videos
- JEE MAINS 2022BOOK - JEE MAINS PREVIOUS YEARCHAPTER - JEE MAINS 2022EXERCISE - PHYSICS (SECTION -B)
10 Videos
Similar Questions
A concave mirror forms a real image four times as tall as the object placed 10 cm infront of mirror. Find the position of the image and the radius of curvature of the mirror.
View SolutionA point object is placed at a distance 20 cm from the focus of a concave mirror of radius of curvature 20cm. Find the distance (incm) of the image from the focus.
View Solution
Knowledge Check
An object is placed at the centre of curvature of a concave mirror . The distance between its image and the pole is
Aequal to fBbetween f and 2fCequal to 2fDgreater than 2fWhen an object is placed beyond the centre of curvature © of a concave mirror, the image will be formed :
ABeyond CBBetween F and CCAt CDAt focusAn object is placed at the center of curvature of a concave mirror. The distance between image and pole is:
AEqual to fBEqual to 2fCgreater than f but less than 2fDgreater than 2f
An object is placed at the focus of a concave lens. Where will be image ?
View SolutionFollowing figure illustrates the ray diagram for the formation of image by a concave mirror. The position of the object is beyond the centre of curvature of the concave mirror. On the basis of given diagram .
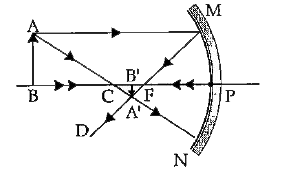
In case of concave mirror, the image distance is ____ when image is formed in front of the mirror and ____when the image is formed behind the mirror.
View SolutionAn object is at a distance of 10 cm from a concave mirror and the image of the object is at a distance of 30 cm from the mirror on the same side as that of the object. The radius of curvature of the concave mirror is
View SolutionThe radius of curvature of a convex mirror is 'x'. The distance of an object from focus of this mirror is 'y'. Then what is the distance of image from the focus ?
View SolutionAn object is placed at a distance of 20 cm from the pole of a concave mirror of focal length 10 cm. The distance of the image formed is
View Solution
