As shown in the diagram, a ray of light incident on the surface of a parallel glass slab of thickness t and refractive index 54. Ray emerges from the opposite face parallel toits initial direction but displaced sideways. Considering the incident angle θ to be very small, the displacement is given by x=tθn. Then the value of n is

The correct Answer is:05.00
sinQsinr=54
θr=54
r=4Q5
In ΔABC
sin(Q−r)≅BCAB
∴sinθ5=BCAB
BC=x=ABsinθ5
x≈tsinθ5≈tQ5
n=5

Topper's Solved these Questions
Similar Questions
A ray of light is incident on a parallel slab of thickness t and refractive index n. If the angle of incidence θ is small, than the lateral displacement in the incident and emergent ray will be
View SolutionA ray of light is incident on one face of a transparent glass slab of thickness 9cm at an angle of incidence 60∘. Lateral displacement of the ray on emerging from the opposite parallel face is 3√3cm. Refractive index of the material of slab is:
View Solution
Knowledge Check
A ray of light passes throught a plane glass slab of thickness t and refractive index μ=1.5. The angle between incident ray and emergent ray will be
A0∘B30∘C45∘D60∘A ray of light is incident on a parallel slab of thickness t and refractive index n. If the angle of incidence θ is small, than the lateral displacement in the incident and emergent ray will be
Atθ(n−1)nBtθnCtθnn−1Dtθ(n+1)nA rays of light is incident on a thick slab of glass of thickness t as shown in figure. The emergent ray is parallel to the incident ray but displaced sideways by a distance d. If the angles are small then d is
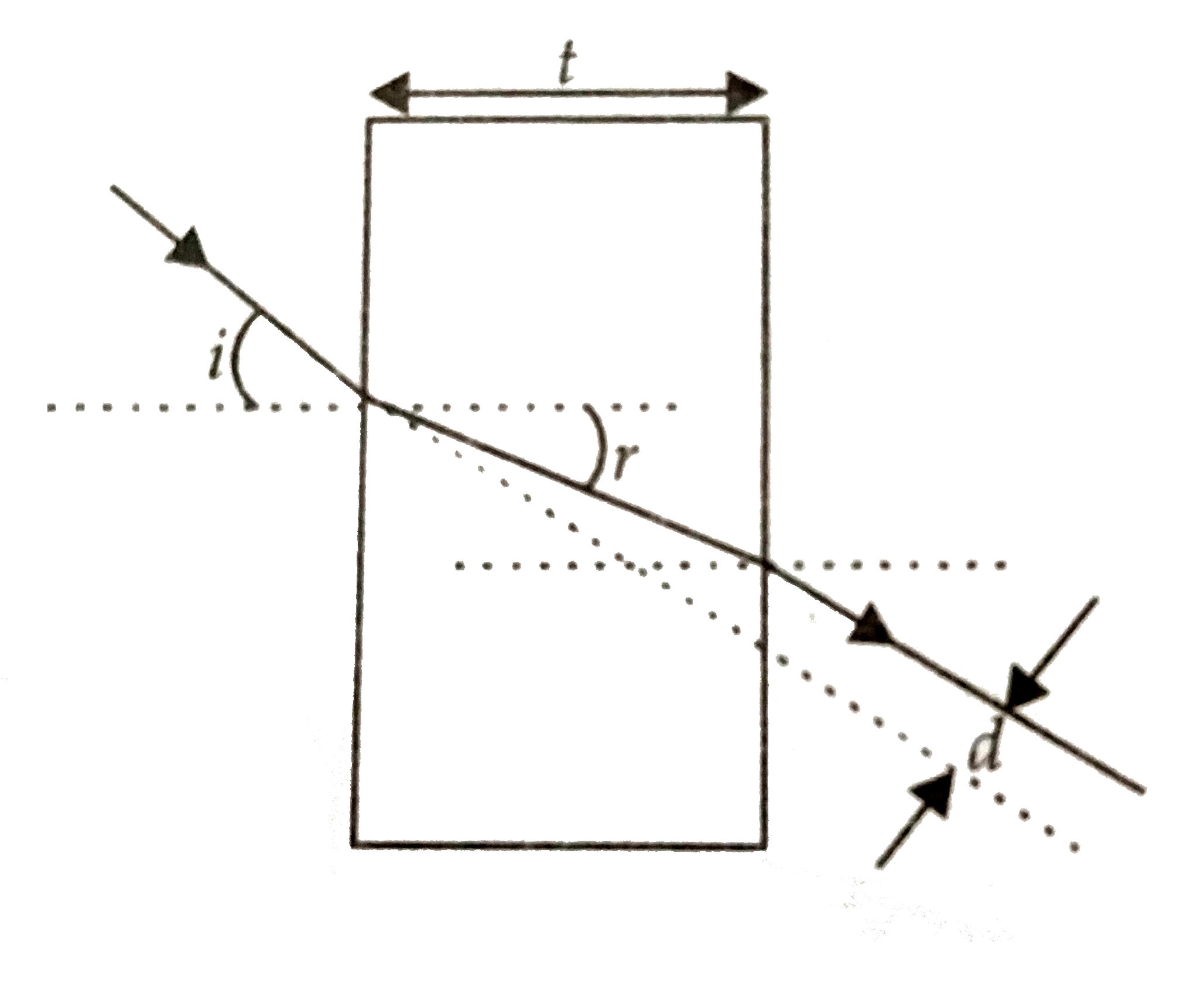 At(1+ir)Brt(1−ir)Cit(1−ri)Dt(1+ri)
At(1+ir)Brt(1−ir)Cit(1−ri)Dt(1+ri)
A rays of light is incident on a thick slab of glass of thickness t as shown in figure. The emergent ray is parallel to the incident ray but displaced sideways by a distance d. If the angles are small then d is

View SolutionA ray of light strikes a glass slab of thickness t. It emergens on the opposite face, parallel to the incident ray but laterally displaced. The lateral displacement, Δx is
View SolutionA ray of light is incident on one face of a transparent glass slab of thickness 9cm at an angle of incidence 60∘. Lateral displacement of the ray on emerging from the opposite parallel face is 3√3cm. Refractive index of the material of slab is:
View SolutionA ray of light is incident on the surface of a glass plate of thickness t. I fthe angle of incidence θ is small, the emerging ray would be displaced side ways by an amount
[Taken = refractive index of glass)
View SolutionA ray of light is incident on a glass slab of thickness t, at an angle i,r is the angle of refraction in the glass slab. Distance travelled in the glass slab is
View Solution
