A light rope fixed t one end of a wooden clamp on the ground passes over a tree branch and hangs on the other side. It mamkes an angle of 300 wilth the ground. A man weighing (60 kg) wants to climb up the rope.The wooden clamp can come out of the ground if an upward force greater than 360 N is applied it. Find the maximu acceleration in the upward direction with which the man can clim,b safely. Neglect friction at the tree branch. Take g=10ms2
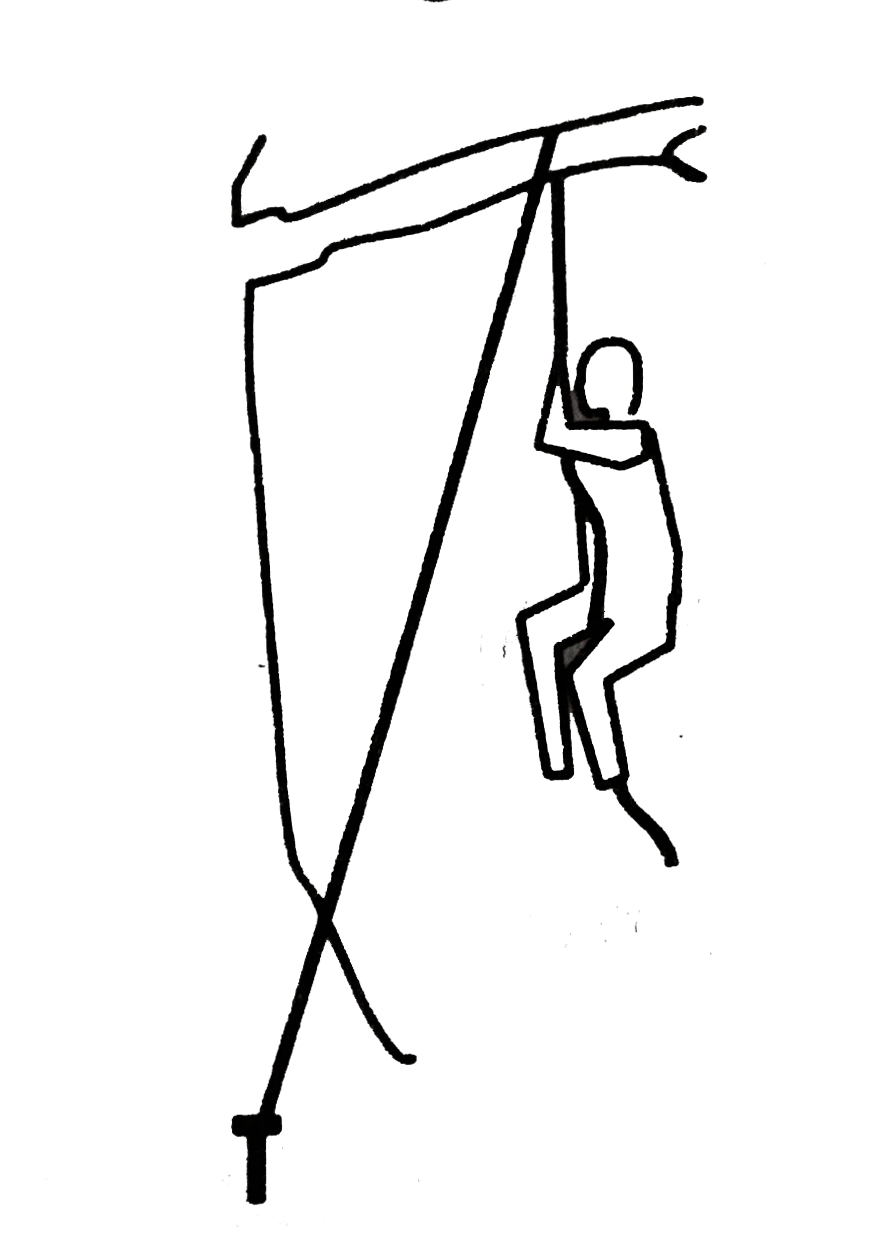
Let T be the tension in the rope. The upward force on the clamp is T sin30^0=T/2.Themaxiμmtensionthtwill¬detachtheclampomthegroundis,∴,givenby<br>T/2=360 N<br>or,T=720 N.<br>Iftheae≤rationoftheman∈theupwarddirectionisatheequationofmotionofthemanis<br>T-600 N=(60 kg)a<br>Themaxiμmae≤rationofthemanforsafeclimb∈gis∴,<br>a=(720N-600N)/(60 Kg) =2m/s^2
Topper's Solved these Questions
- NEWTON'S LAWS OF MOTIONBOOK - HC VERMACHAPTER - NEWTON'S LAWS OF MOTIONEXERCISE - Short Answer
17 Videos
- NEWTON'S LAWS OF MOTIONBOOK - HC VERMACHAPTER - NEWTON'S LAWS OF MOTIONEXERCISE - Objective 1
14 Videos
- NEWTON'S LAWS OF MOTIONBOOK - HC VERMACHAPTER - NEWTON'S LAWS OF MOTIONEXERCISE - Exercises
42 Videos
- LAWS OF THERMODYNAMICSBOOK - HC VERMACHAPTER - LAWS OF THERMODYNAMICSEXERCISE - Short Answer
15 Videos
- PHYSICS AND MATHEMATICSBOOK - HC VERMACHAPTER - PHYSICS AND MATHEMATICSEXERCISE - Exercises
34 Videos
Similar Questions
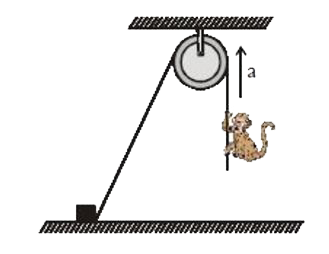
A light string fixed at one end to a clamp on ground passes over a fixed pulley and hangs at the other side. It makes an angle of 30∘ with the ground. A monkey of mass 5 kg climbs up the rope. The clamp can tolerate a vertical force of 40 N only. The maximum acceleration in upward direction with which the monkey can climb safely is:
(neglect friction and take g=10m/s2)
View SolutionA light rope fixed to a peg on the ground passes over a tree branch and hangs on the other side A man weighing 60 kg wants to climb up the rope. If the peg comes out of the ground by a vertical force greater than (360√3) N, with what maximum acceleration can the man climb up safely ? (g=10m/s2)
View Solution
Knowledge Check
A light string fixed at one end to a clamp on ground passes over a fixed pulley and hangs at the other side. It makes anangle of 30∘ with the ground. A monkey if makes an angle of 30∘ with the ground. A monkey of mass 5kg climbs up the rope. The clamp can tolerate is vertical force of 40N only. the maximum accelration in upward direction with which the monkey can climb safety is (Neglect friciton and take g=10m/s2)
 A2m/s2B4m/s2C6m/s2D8m/s2
A2m/s2B4m/s2C6m/s2D8m/s2A monkey of 25 kg is holding a vertical rope. The rope does not break if a body of mass 30 kg is suspended with the rope exceeds 30 kg. What will be the maximum acceleration with which the monkey can climb up along the rope ? ( Take ,=10ms−2)
A2ms−2B2.5ms−2C3ms−2D4ms−2A body of mass 40 kg wants to climb up a rope hanging vertically. The rope can withstands a maximum tension of 500 N. What is the maximum acceleration with which the boy can climb rope? Tak e g=10m/s2
A1.5m/s2B2.0m/s2C2.5m/s2D3.0m/s2
The upper part of a tree broken over by the wind makes an angle of 30∘ with the ground and the distance from the root to the point where the top of the tree touches the ground is 15 m. Using sine rule, find the height of the tree.
View SolutionA block weighing 4N is supported by two ropes Once rope is horizontal and the other makes an angle of 30∘ with the ceilling The tension (in newton) in the rope attached to the ceilling is .
View SolutionThe upper part of a tree broken over by the wind makes an angle of 60∘ with the ground and the horizontal distance from the foot of the tree to the point where the top of the tree meets the ground is 10 metres. Find the height of the tree before broken.
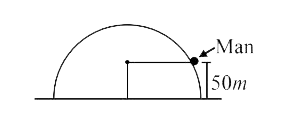
View SolutionA large hemi sphere of radius 100m is fixed on the ground. A man weighing 700 N stands a at height of 50m above the ground. N and f represent the normal and frictional force acting on him. Then :
View SolutionThe maximum tension a rope can withstand is 60 kg - wt. The ratio of maximum acceleration with which two boys of masses 20kg and 30kg can climb up the rope at the same time is
View Solution
