A paticle of mass 50 g moves on a straight line. The vatiation of speed with time is shown in figure. Find the force acting on the particle at t=2,4 and 6 secons.
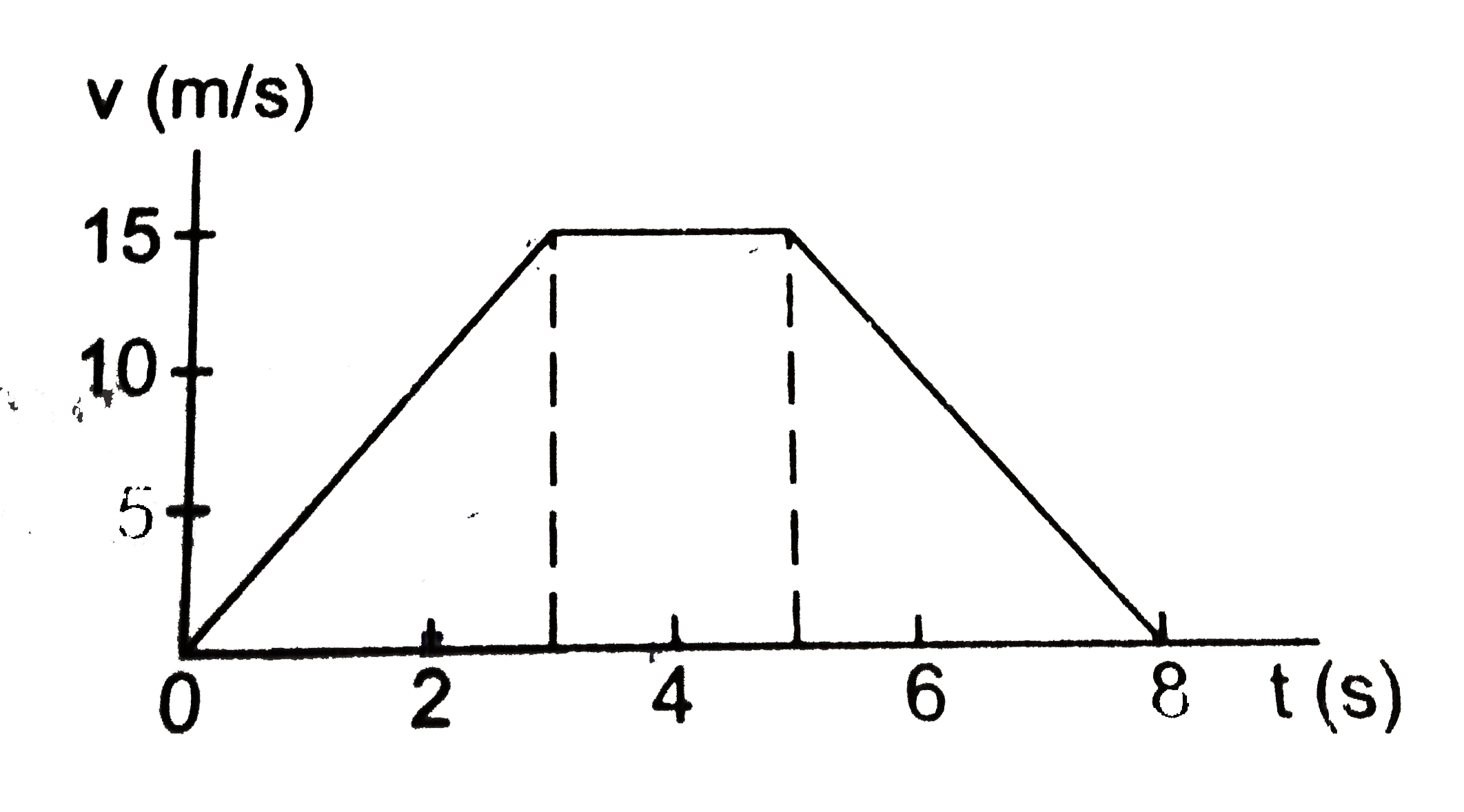
Given m=50g=5×10−2kg
As shown in the ure
Slopd of OA=tanθ
=ADOD=153=ms2
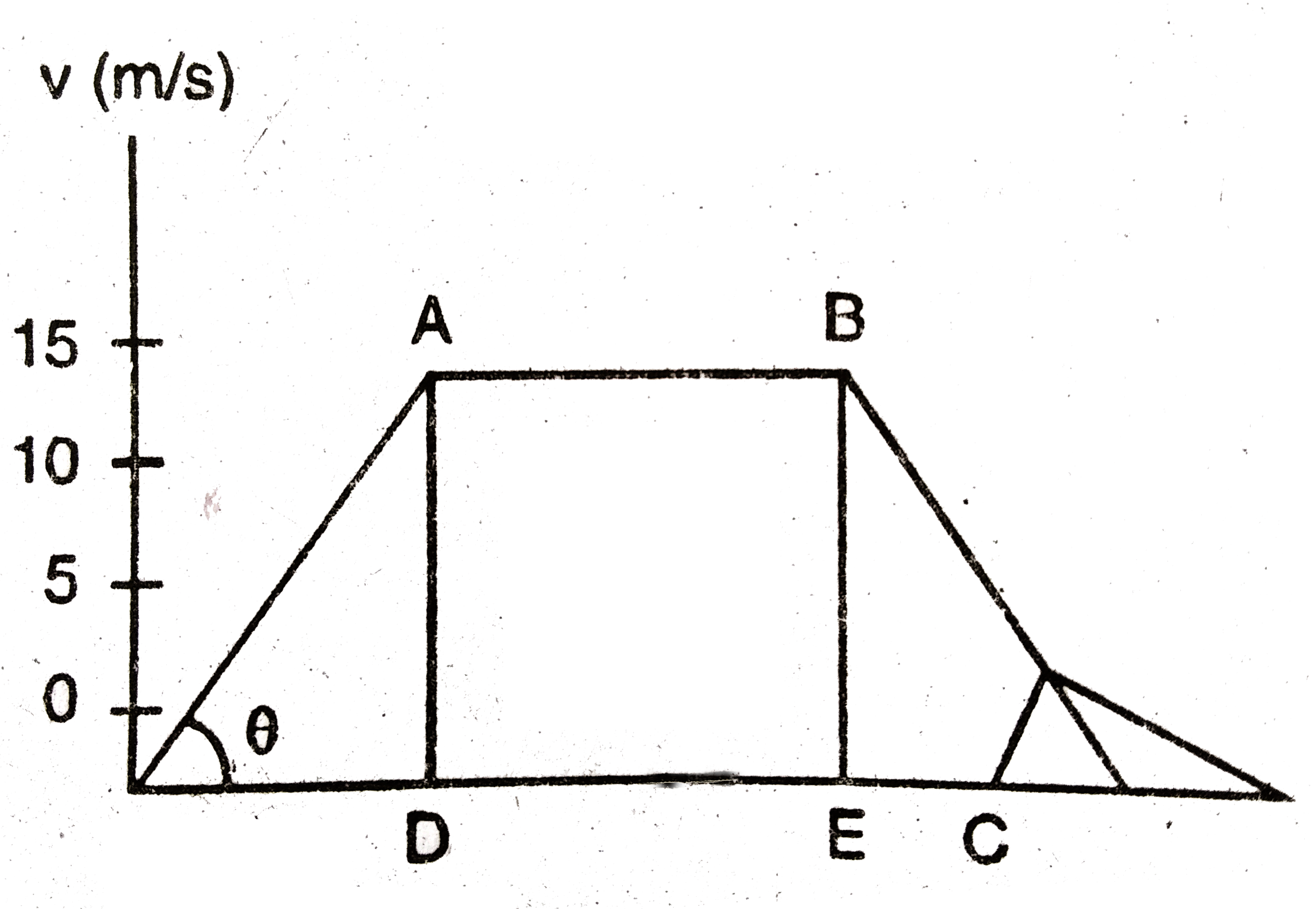
So, at t=2 sec, acceleration is 5 m/s^2
Force =ma=5×10−2×5
=0.25N along the motion ltBrgt At t=4sec,
Slope of ab=0
and acceleration =0 [Since tanθ=00]
tt=6sec,
acceleration = slope of BC
In△BEC,tanθ=5
SlopeofBC=tan(1080−θ)−tanθ
=5ms2(dece≤ration)<br>:. Force = ma= 5xx 10^-2 xx5<Br>=0.25 N
Opposite to the motion.
Topper's Solved these Questions
- NEWTON'S LAWS OF MOTIONBOOK - HC VERMACHAPTER - NEWTON'S LAWS OF MOTIONEXERCISE - Objective 2
9 Videos
- LAWS OF THERMODYNAMICSBOOK - HC VERMACHAPTER - LAWS OF THERMODYNAMICSEXERCISE - Short Answer
15 Videos
- PHYSICS AND MATHEMATICSBOOK - HC VERMACHAPTER - PHYSICS AND MATHEMATICSEXERCISE - Exercises
34 Videos
Similar Questions
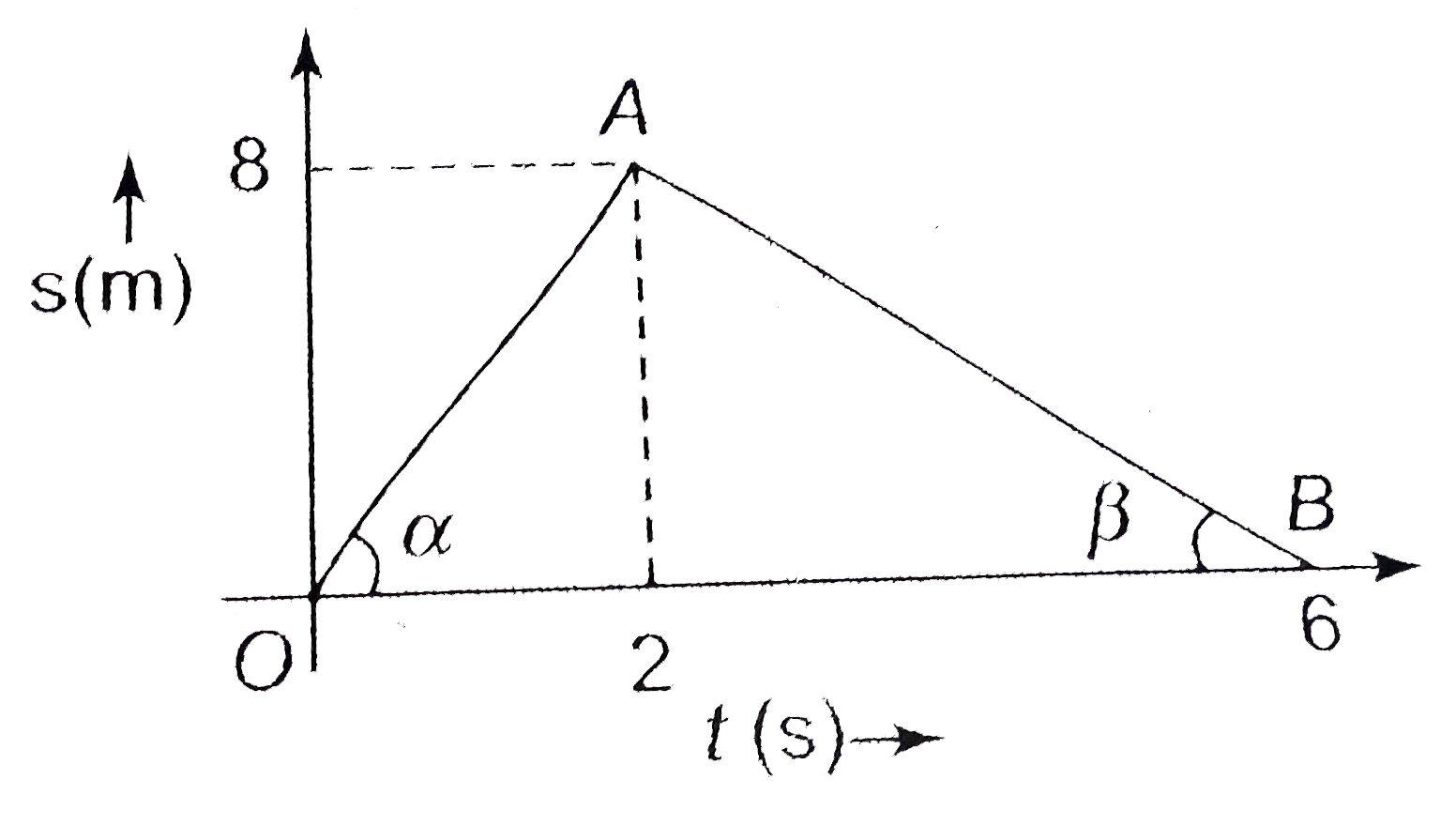
For a particle moving in a straight line, the position-time graph is as shown in the figure. Find the velocity of the particle at time t=1s and t=4s. Also sketch the v-t graph.
View SolutionA particle starts moving from rest on a straight line. Its acceleration a verses time t is shown in the figure. The speed of the particle is maximum at the instant
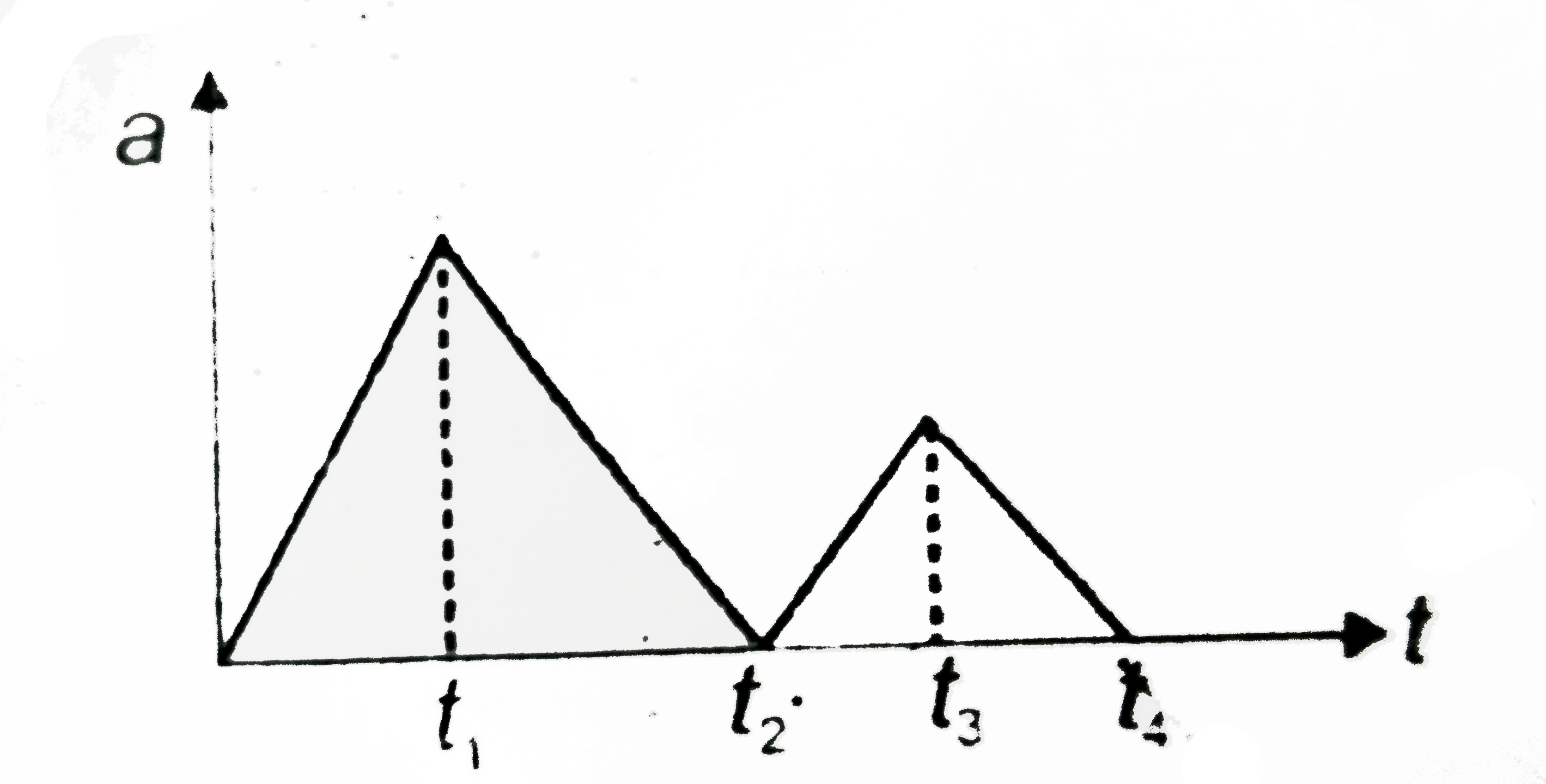
View Solution
Knowledge Check
The kinetic energy of a body moving along a straight line varies with time as shown in figure. The force acting on the body:
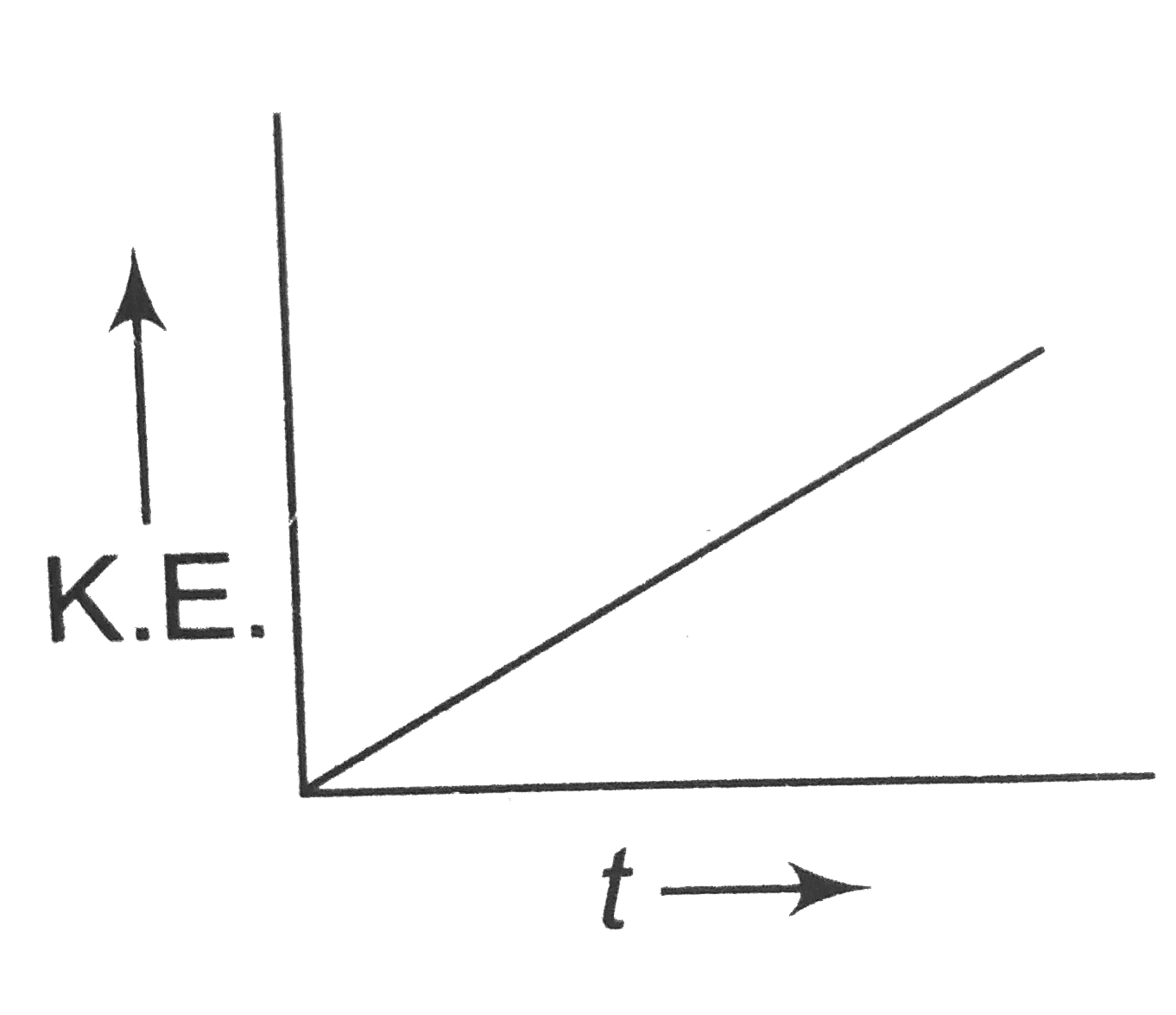 AzeroBconstantCdirectly proportional to velocityDinversely proportional to velocity
AzeroBconstantCdirectly proportional to velocityDinversely proportional to velocityKinetic energy of a particle moving in a straight line is proportional to the time t. The magnitude of the force acting on the particle is :
ADirectly proportional to the speed of the particleBInversely proportional to √tCInversely proportional to the speed of the particleDDirectly proportional to √tA particle of mass 10 kg is moving in a straight line. If its displacement, x with time tis given by x=(t3−2t−10)m then the force acting on it at the end of 4 seconds is
A24NB240NC300ND1200N
A particle starts motion from rest and moves along a straight line. Its acceleration-time graph is shown. Find out speed of particle at t=2s and at t=3s.

View SolutionA particle starts motion from rest and moves along a straight line. Its acceleration-time graph is shown. Find out speed of particle at t=2s and at t=3s.

View SolutionThe displacement of a particle of mass 2kg moving in a straight line varies with times as x=(2t3+2)m. Impulse of the force acting on the particle over a time interval between t = 0 and t = 1 s is
View SolutionThe displacement time curve of a particle is shown in the figure. The external force acting on the particle is :
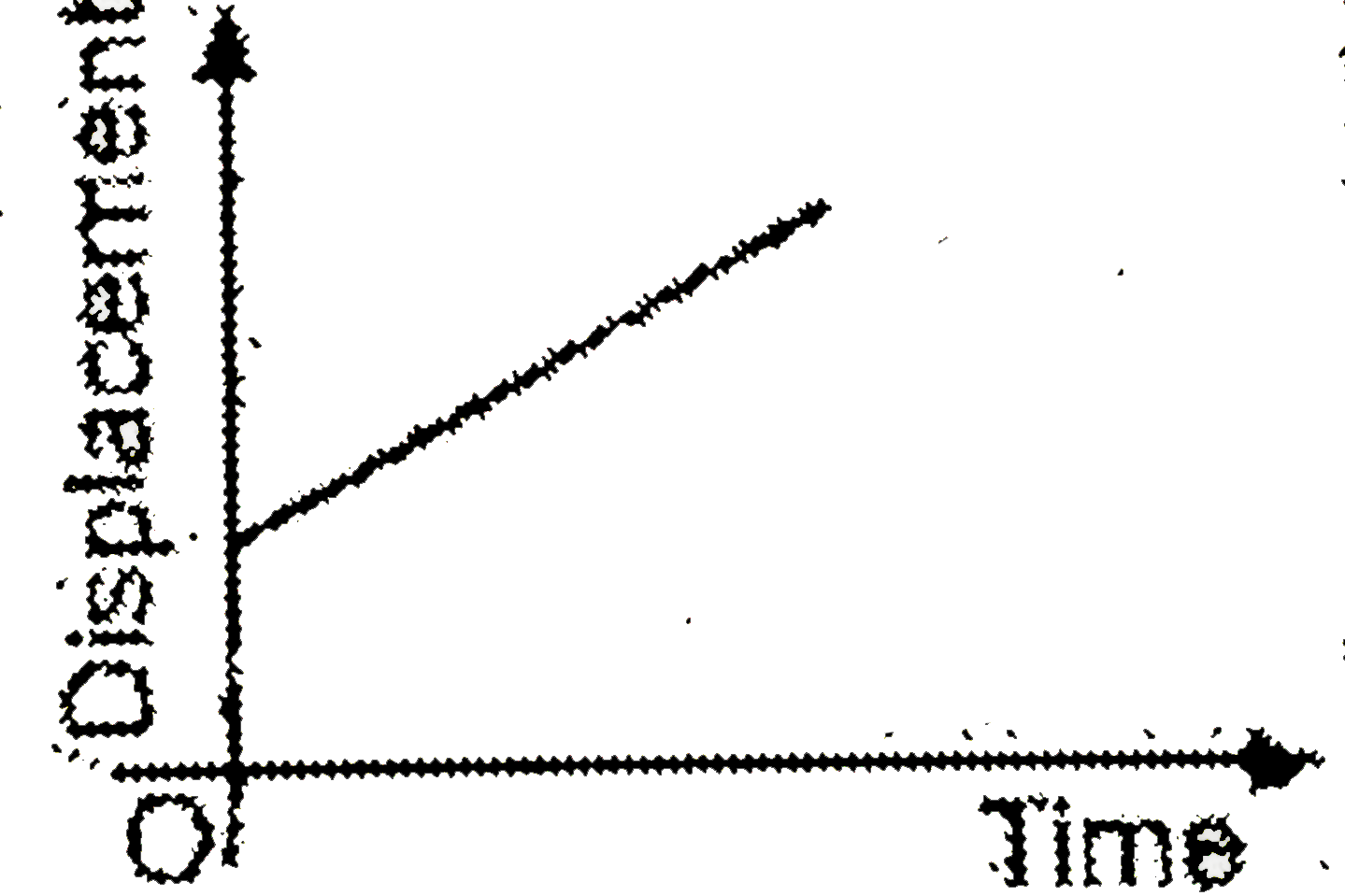
View SolutionKinetic energy of a particle moving in a straight line varies with time t as K=4t2. The force acting on the particle
View Solution
