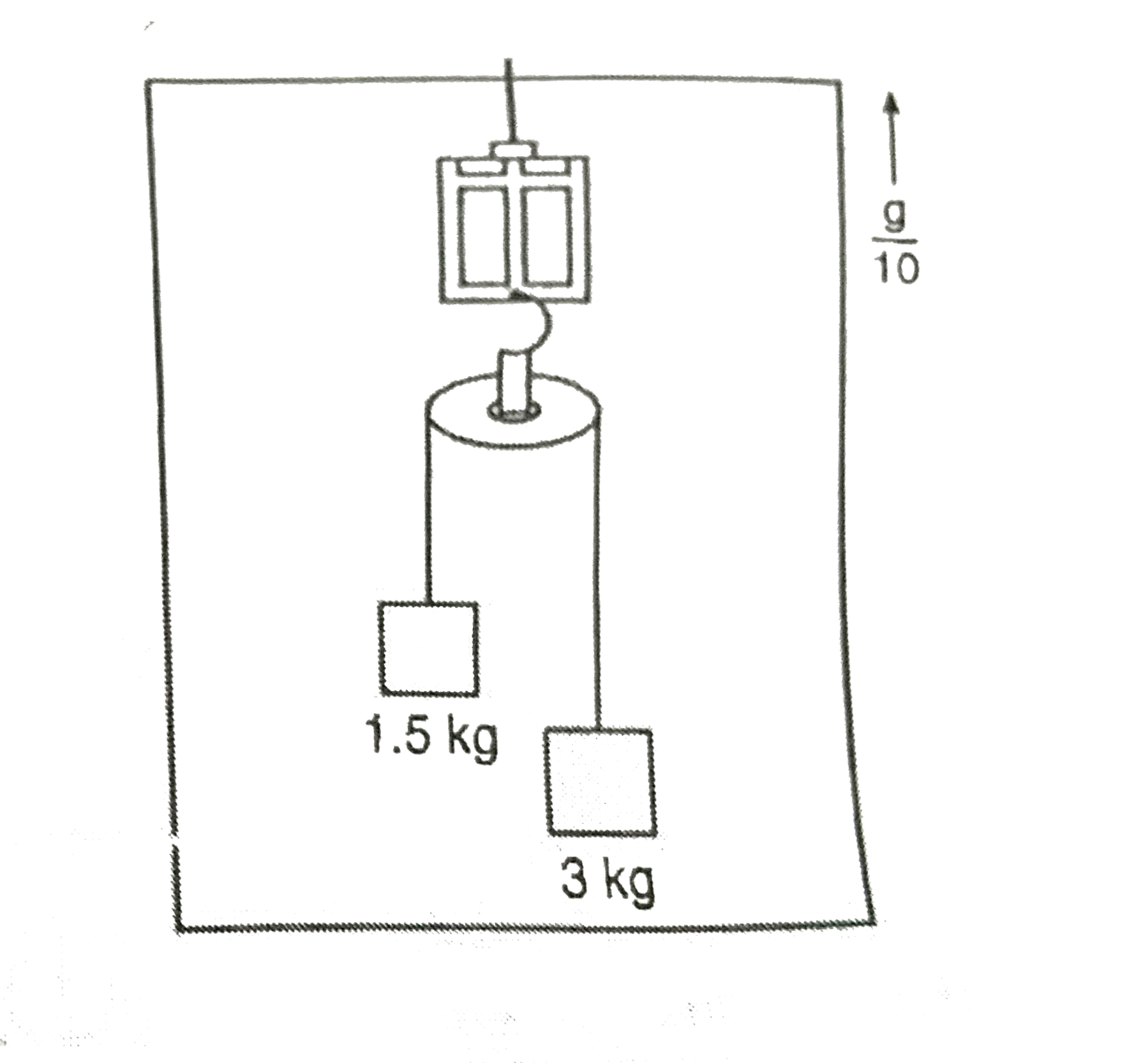
Find the reading of th spring balance shown in figure. The elevator is going up with an accelertion of g/10, the pulley and the strng are light and the pulley is smooth.
To solve the problem of finding the reading of the spring balance when the elevator is accelerating upwards with an acceleration of g/10, we can follow these steps:
Step 1: Understand the Effective Gravity
When the elevator is accelerating upwards, the effective acceleration due to gravity increases. The effective gravitational force can be calculated as:
geffective=g+a
where a is the acceleration of the elevator. In this case, a=g10, so:
geffective=g+g10=10g10+g10=11g10
Step 2: Set Up the Free Body Diagram
Consider two masses, M1 (the heavier mass) and M2 (the lighter mass). The tension in the string (T) acts upwards on both masses. For M1, the forces acting on it are:
- Weight downwards: M1geffective
- Tension upwards: T
For M2, the forces acting on it are:
- Tension upwards: T
- Weight downwards: M2geffective
Step 3: Write the Equations of Motion
Using Newton's second law, we can write the equations for both masses.
For M1:
M1geffective−T=M1a
For M2:
T−M2geffective=M2a
Step 4: Substitute geffective and Solve
Substituting geffective=11g10 into the equations gives:
1. M111g10−T=M1a
2. T−M211g10=M2a
Step 5: Add the Two Equations
Adding the two equations to eliminate T:
M111g10−M211g10=M1a+M2a
This simplifies to:
(M1−M2)11g10=(M1+M2)a
Step 6: Solve for Tension T
From the first equation, we can express T:
T=M111g10−M1a
Substituting a=(M1−M2)11g10(M1+M2) into the equation for T will yield the tension.
Step 7: Find the Spring Balance Reading
The reading of the spring balance is equal to 2T (since there are two segments of the string pulling on the spring):
Reading=2T
Final Calculation
After substituting and simplifying, we find:
T=4.4g10
Thus, the reading of the spring balance will be:
Reading=2T=4.4 kg
Conclusion
The reading of the spring balance is 4.4 kg.
---
When the elevator is accelerating upwards, the effective acceleration due to gravity increases. The effective gravitational force can be calculated as:
geffective=g+a
where a is the acceleration of the elevator. In this case, a=g10, so:
geffective=g+g10=10g10+g10=11g10
Consider two masses, M1 (the heavier mass) and M2 (the lighter mass). The tension in the string (T) acts upwards on both masses. For M1, the forces acting on it are:
- Weight downwards: M1geffective
- Tension upwards: T
- Tension upwards: T
- Weight downwards: M2geffective
Using Newton's second law, we can write the equations for both masses.
M1geffective−T=M1a
For M2:
T−M2geffective=M2a
Substituting geffective=11g10 into the equations gives:
1. M111g10−T=M1a
2. T−M211g10=M2a
Adding the two equations to eliminate T:
M111g10−M211g10=M1a+M2a
This simplifies to:
(M1−M2)11g10=(M1+M2)a
From the first equation, we can express T:
T=M111g10−M1a
Substituting a=(M1−M2)11g10(M1+M2) into the equation for T will yield the tension.
The reading of the spring balance is equal to 2T (since there are two segments of the string pulling on the spring):
Reading=2T
After substituting and simplifying, we find:
T=4.4g10
Thus, the reading of the spring balance will be:
Reading=2T=4.4 kg
The reading of the spring balance is 4.4 kg.
Topper's Solved these Questions
- NEWTON'S LAWS OF MOTIONBOOK - HC VERMACHAPTER - NEWTON'S LAWS OF MOTIONEXERCISE - Objective 2
9 Videos
- LAWS OF THERMODYNAMICSBOOK - HC VERMACHAPTER - LAWS OF THERMODYNAMICSEXERCISE - Short Answer
15 Videos
- PHYSICS AND MATHEMATICSBOOK - HC VERMACHAPTER - PHYSICS AND MATHEMATICSEXERCISE - Exercises
34 Videos
Similar Questions
The elevator is going up with an acceleration of g/10 the pulley and the string are light and the pulley is smooth. If reading of spring balance shown is 0.8x Calculate x. (Take g=10m/s2)
View SolutionFind the reading of spring balance in the adjoining figure, pulley and strings are ideal.
View Solution
Knowledge Check
In the arrangement shown in the figure the elevator is going up with an acceleration of g/10. If the pulley and the string are light and the pulley is smooth, the tension in the string AB is
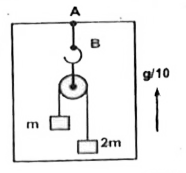 A4415mgB8815mgC2215mgD44150mg
A4415mgB8815mgC2215mgD44150mgReading of spring balance S1 and S2 (Pulley are ideal)
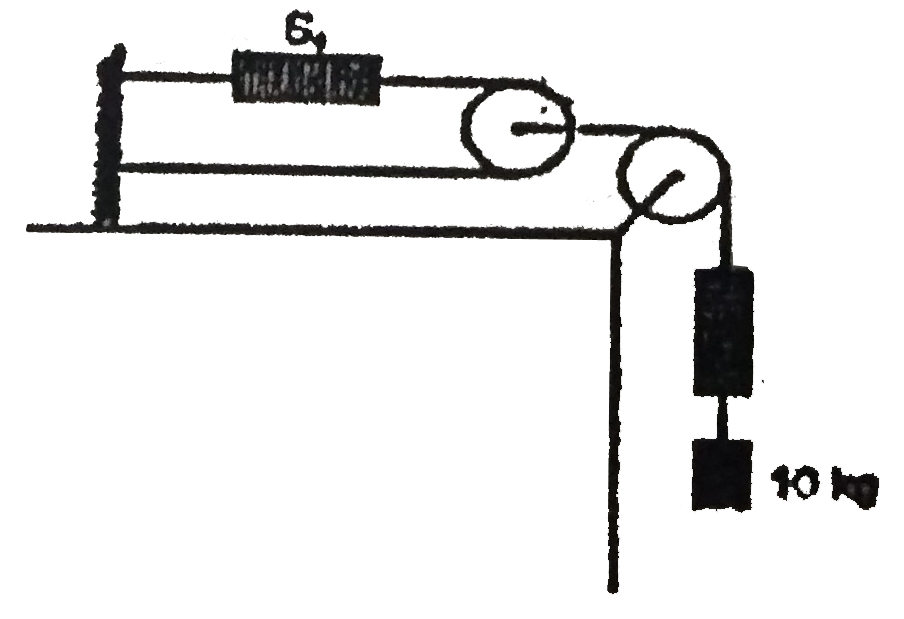 A10 kg, 10 kgB5 kg,5 kgC5 kg, 10 kgD10 kg,5 kg
A10 kg, 10 kgB5 kg,5 kgC5 kg, 10 kgD10 kg,5 kg
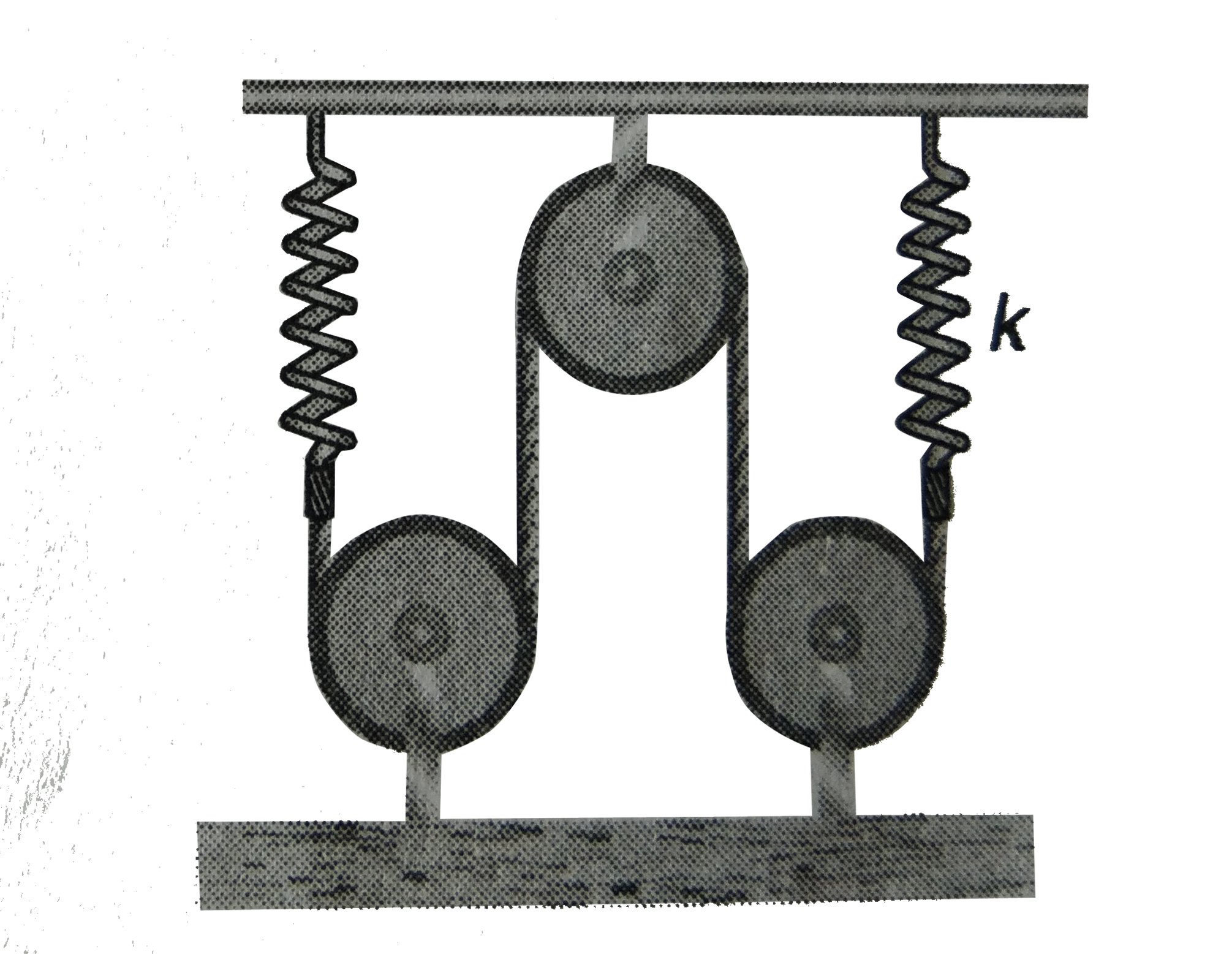
Find the natural frequency of the system shown in figure. The pulleys are smooth and massless.
View SolutionConsider the stituation shown in figure. The elevator is going up with an acceleration of 2.00 ms^-2 and the focal length of the mirror is 12.0 cm. All the surfaces are smooth and the pulley is light. The mass-pulley system is released from rest (with respect to the elevator) at t=0 when the distance of B from the mirror is 42.0 cm. Find the distance between the image of the block B and the mirror at t=0.200 s. Take g=10ms−2

View SolutionFind the reading of spring balance as shown in fig. Assume that mass M in equilibrium. (All surfaces are smooth).

View Solutionfind the acceleration of the block of mass M in the situation shown in figure. All the surfaces are frictionless and the pulleys and the string are light.

View SolutionFind the acceleration of each block in the situation shown in the figure. All the surface are smooth and the pulleys and sttings are light.
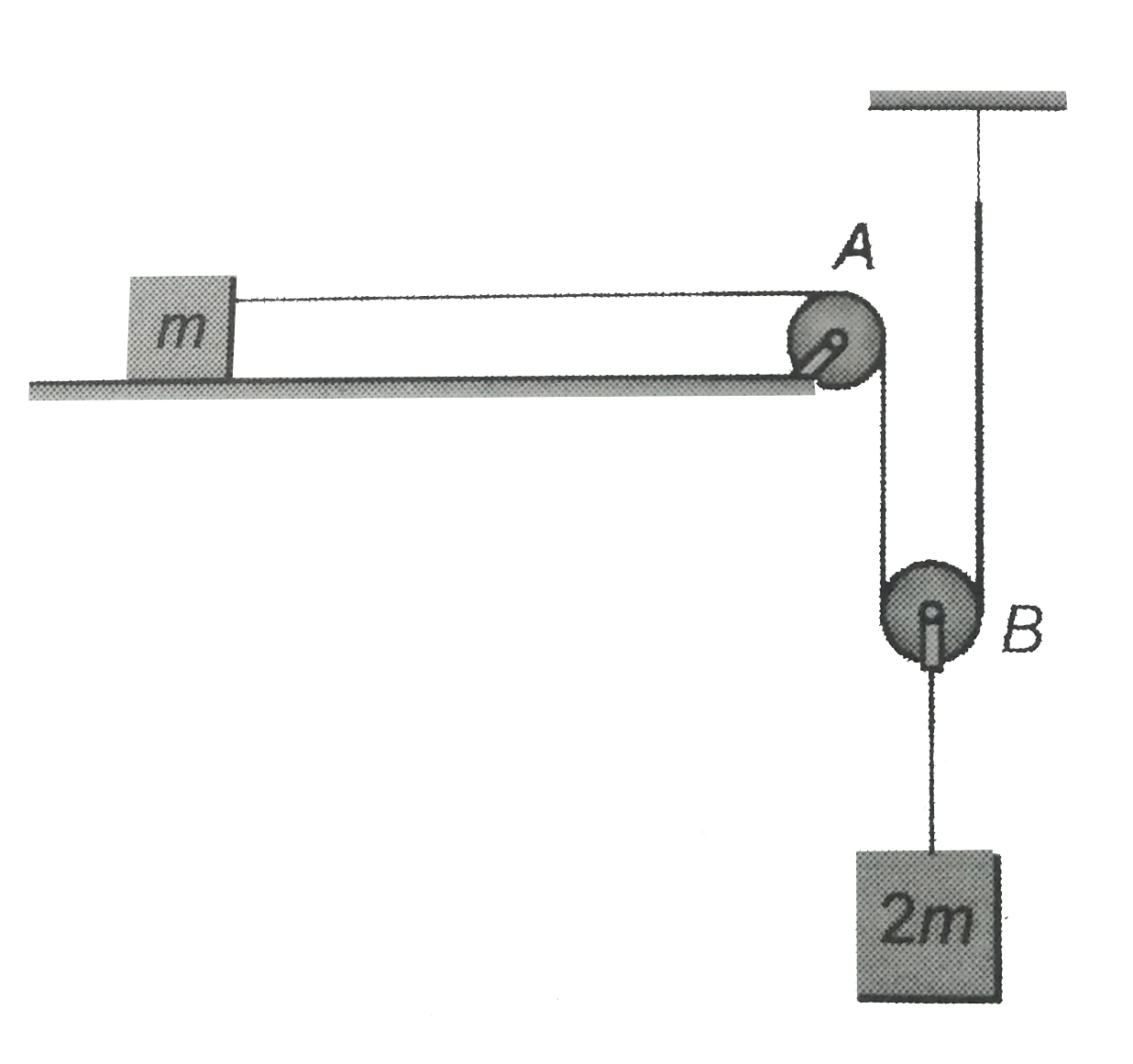
View SolutionFind the acceleration of two masses as shown in figure . The pulley are light and frictionless and string are light and frictionless and string are light and inextensible.
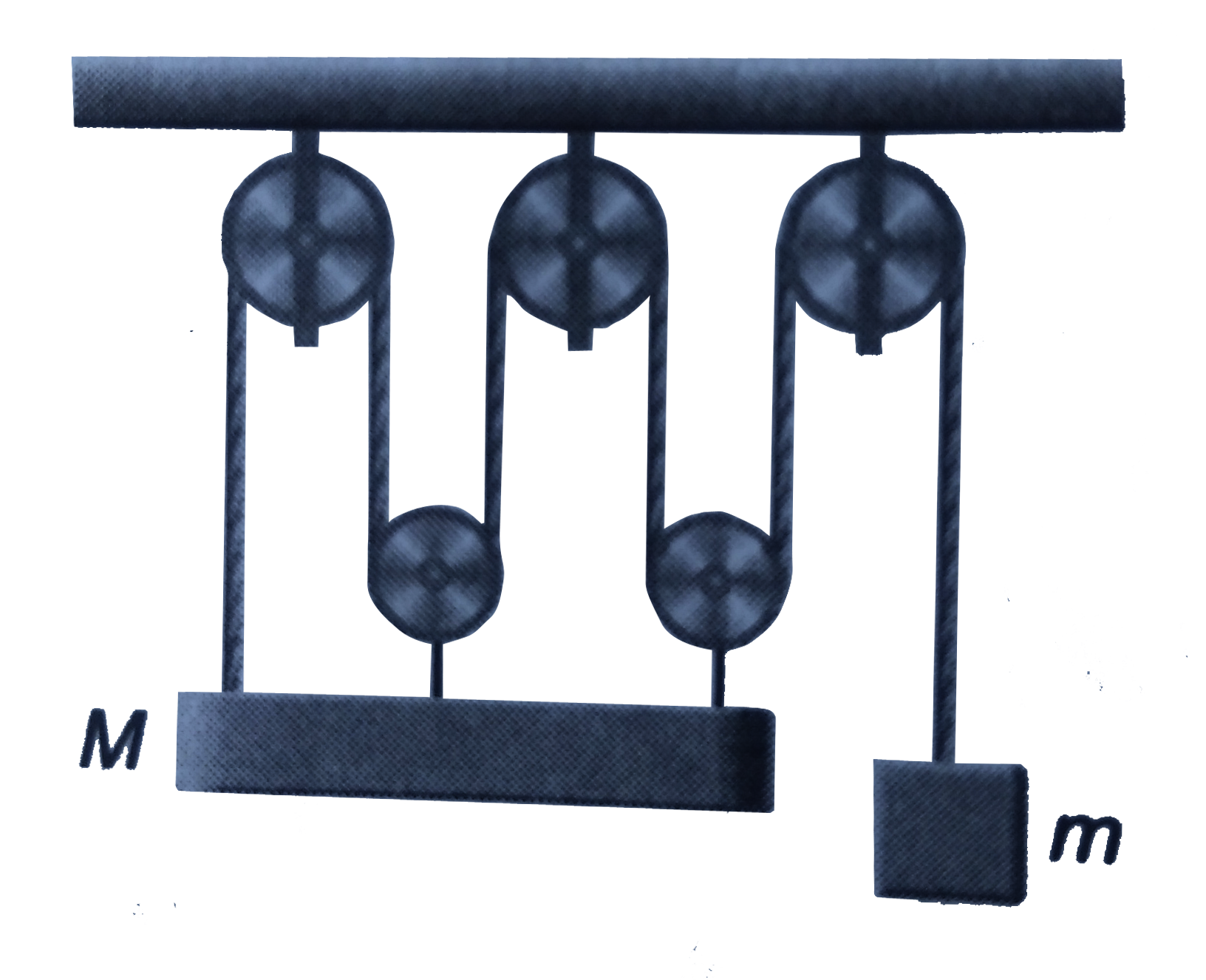
View Solution