A long narrow horizontal slit is placed 1 mm above a horizontal plane mirror. The interference between the light coming directly from the slit and that after reflection is seen on a screen 1.0 in away from the slit, Find the fringe-width if the light used has a wavelength of 700 nm.
The correct Answer is:C
Givent that D=1m
λ=700mm=700×10−3m<br>Sincea=1mm<br>d=2a=2mm<br>=2xx10^-3m<br>[Lloyd'smirrorexperiment]
Fringe width =(lamdaD)/d<br>=(700xx10^9xx1m)/(2xx0^-3m)<br>0.35mm
Topper's Solved these Questions
Similar Questions
A long narrow horizontal slit lies 1mmabove a plane mirror.The interference pattern produced by the slit and its image is viewed on a screen distant 1mfrom the slit .The wavelength of light is600nm.Find the distance of first maximum above the mirror.
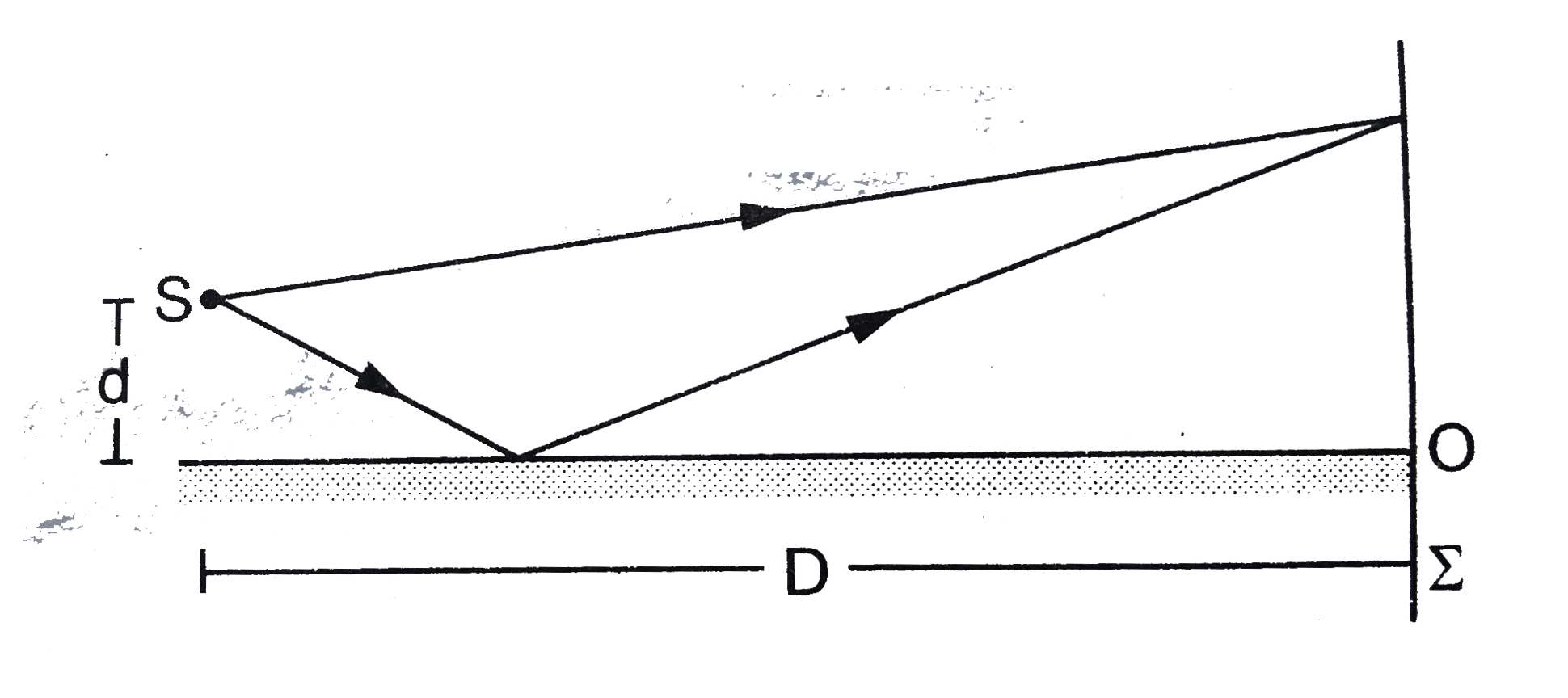
View SolutionA narrow slit S transmitting light of wavelength λ is placed a distance d above a large plane mirror as shown in figure. The light coming directly from the slit and that coming after the reflection interference at a screen ∑ placed at a distance LD from the slit. a. What will be the intensity at a point just above the mirror. i.e., just above O? b. At what distance from O does the first maximum occur?
?
View Solution
Knowledge Check
A long horizontal slit is placed 1 mm above a horizontal plane mirror. The interference between the light coming directly from the slit and the after reflection is seen on a screen 1 m away from the slit. If the mirror reflects only 64% of the light falling on it, the ratio of the maximum to the minimum intensity in the interference pattern observed on the screen is
A8:1B3:1C81:1D9:1In a Lloyds's mirror experiment as narraow slit S iransmitting a light of wavelength λ is placed 3 mm above a small plane mirror ( as shown). The light coming directly from the the slit and that coming after the reflection interfere on a screen placed at a distance of 90 cm from the slit. Length of mirror is 2 mm and the middle point of mirror is 2 mm from point P.

If fringe width is 0.1 mm then what is the wavelength of light used ?A3.33×10−7mB6.67×10−7mC1.0×10−7mD4×10sA long narrow horizontal slit lies 1mm above a plane mirror. The interference pattern produced by the slit and its and its image is viewed on a screen ∑ distance 1m from the slit. The wavelength of light is 600nm. Then the distance of the first maxima above the mirror is equal to (d<<D) :
 A0.30mmB0.15mmC60mmD7.5mm
A0.30mmB0.15mmC60mmD7.5mm
Two slits in YDSE are placed 2 millimeter from each other. Interference pattern is observed on a screen placed 2m from the plane of slits. What is the fringe width for a light of wavelength 400 nm ?
View SolutionTwo slits in YDSE are placed 1 mm from each other. Interference pattern is observed on a screen placed 1m from the plane of slits. What is the angular fringe width for a light of wavelength 400 nm
View SolutionTwo slits in YDSE are placed 2 millimetre from each other. Interference pattern is observedon a screen placed 2 m from the plane of slits. What is the fringe width for a light of wavelength 400 nm?
View SolutionIn a Lloyds's mirror experiment as narraow slit S iransmitting a light of wavelength λ is placed 3 mm above a small plane mirror ( as shown). The light coming directly from the the slit and that coming after the reflection interfere on a screen placed at a distance of 90 cm from the slit. Length of mirror is 2 mm and the middle point of mirror is 2 mm from point P.
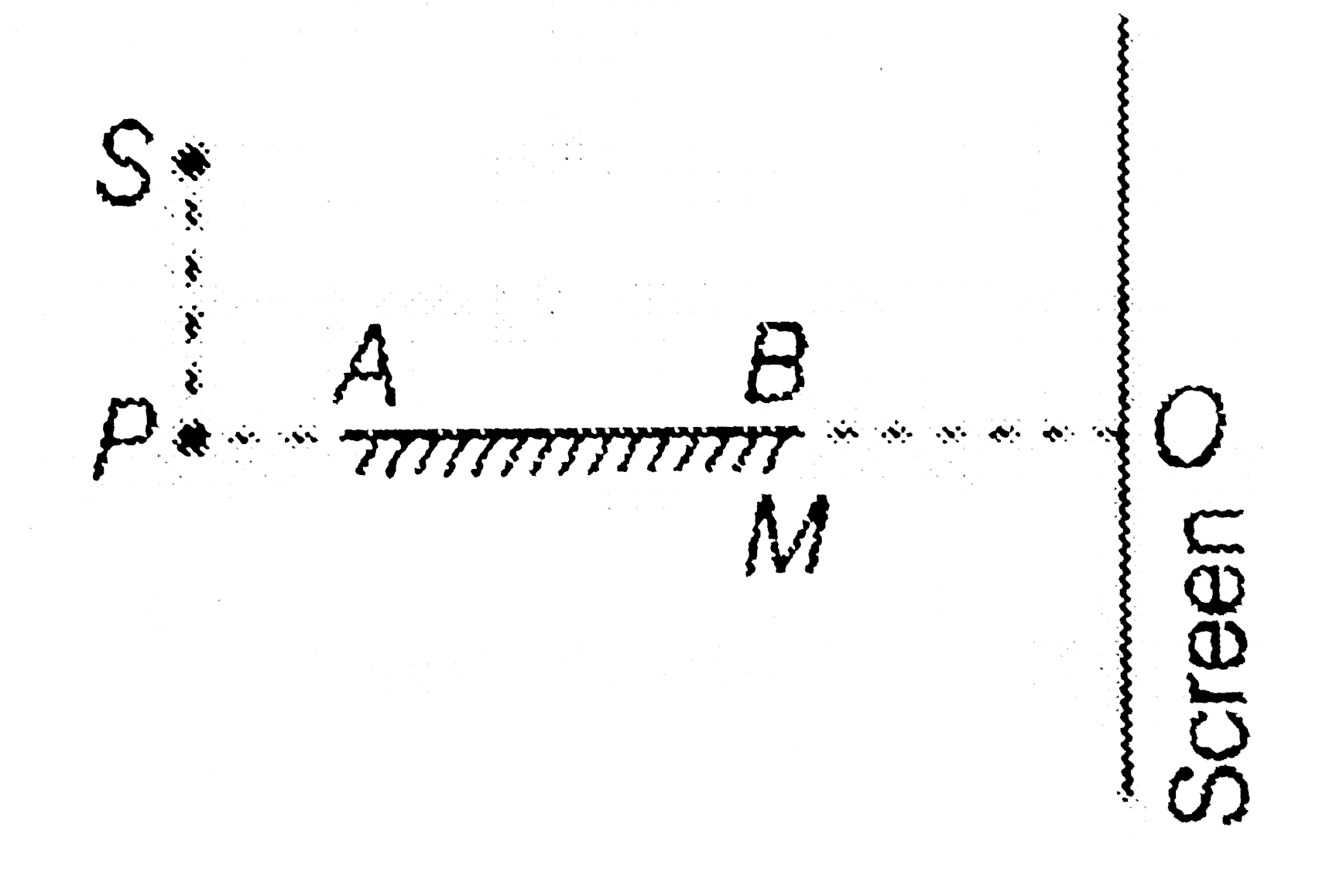
If the mirror is shifted towards left then how does the fringe pattern on screen changes ?
View SolutionThe distance between the first and the sixth minima in the diffraction pattern of a single slit is 0.5 mm. The screen is 0.5 m away from the slit. If the wavelength of light used is 5000 Å. Then the slit width will be
View Solution
