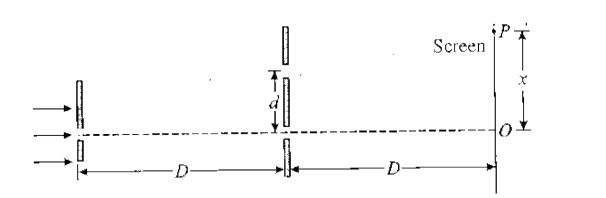
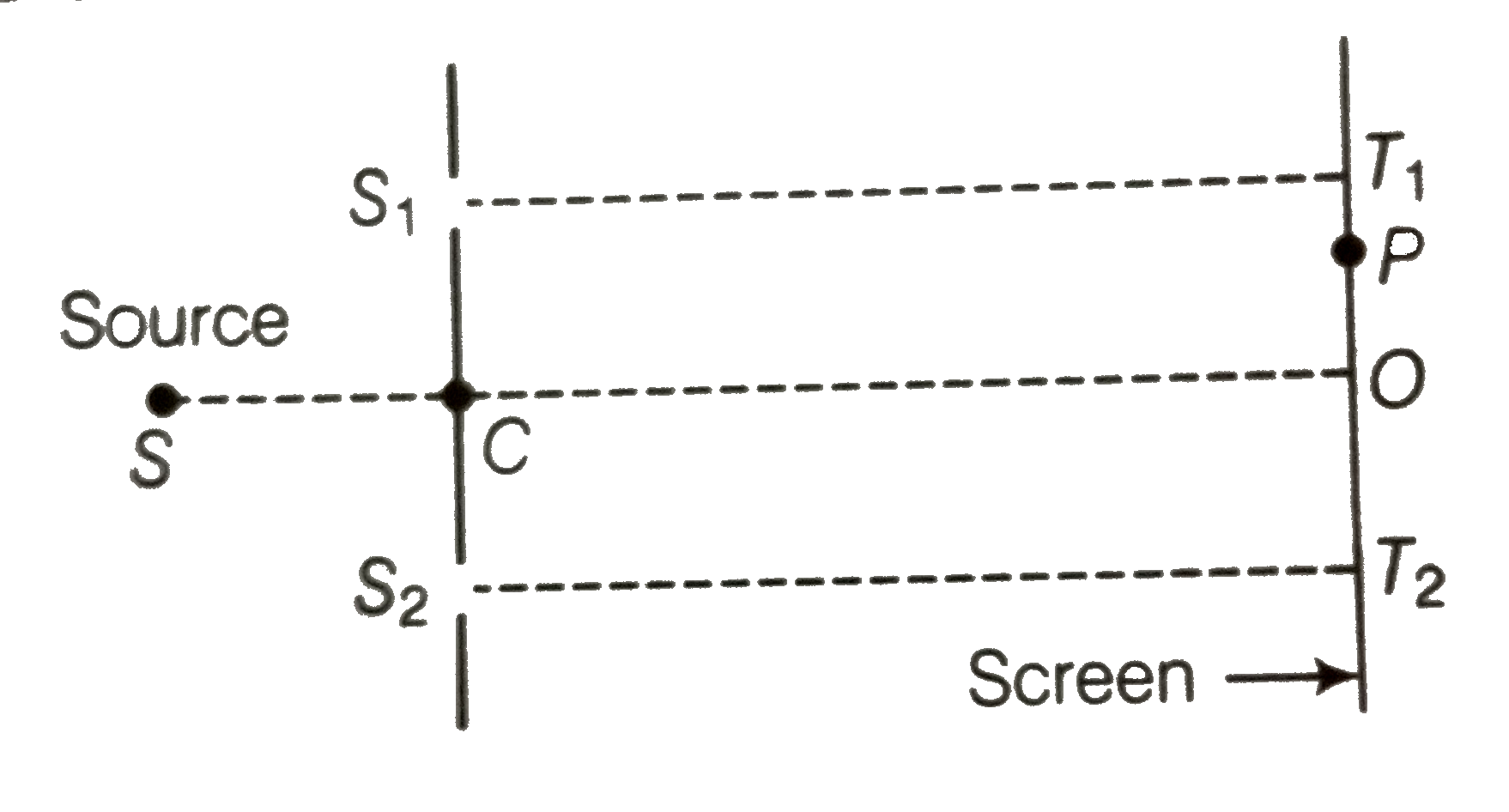
Consider the arrangement shown in figure. The distance D is large compared to the searated d between the slits. a. Find the minimum value of d so that there is a dark fringe at O. b. Suppose d has this value. Find the distance x at which the next bright firnnge is formed. c.Find teh fringe width.

The correct Answer is:A, B, C, D
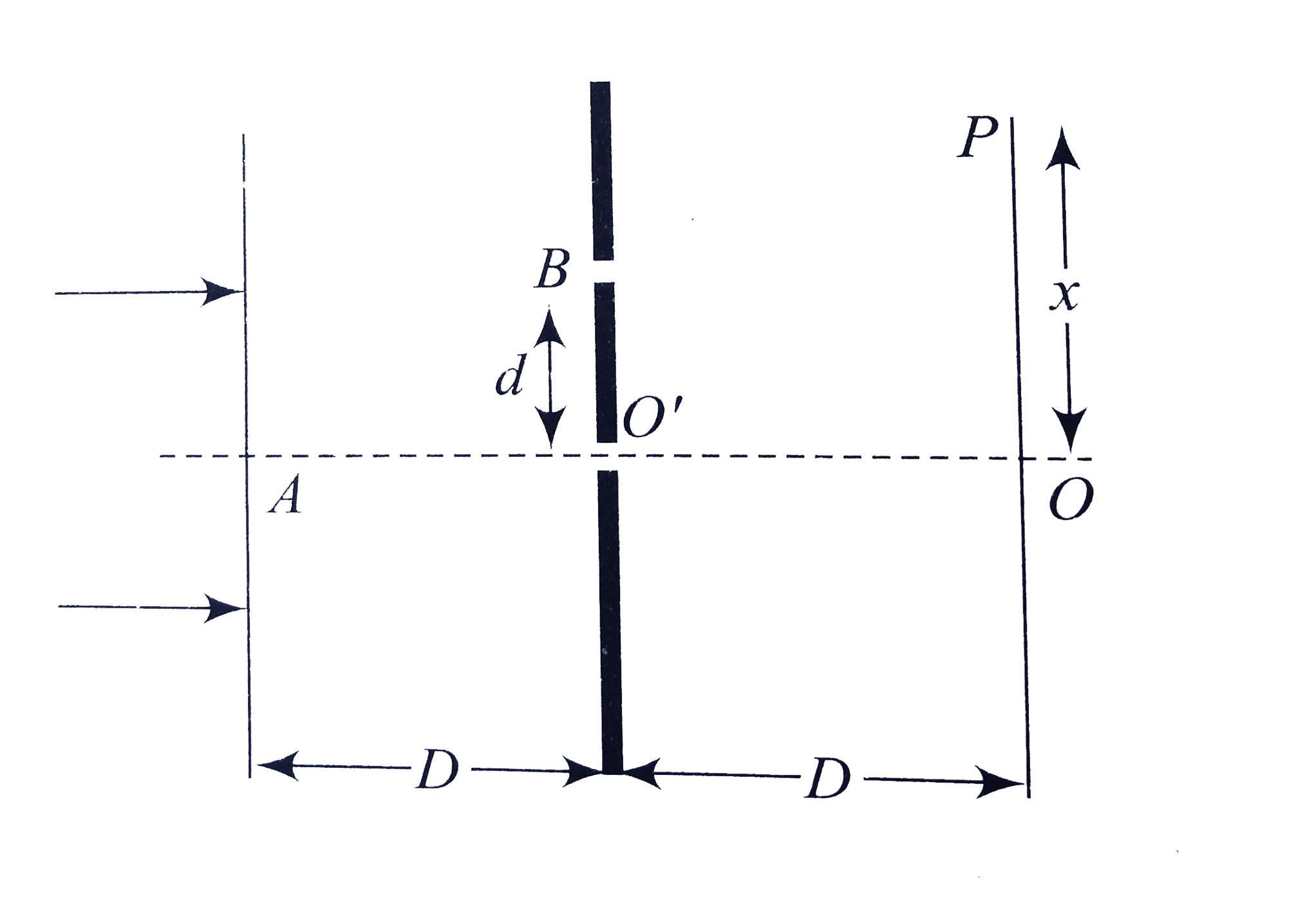
From the diagram it can be seen that at point O,
Path differene =(AB+BO)−(AC+CO)
=2(AB−AC)
[Since AB=BO and AC=CO]<br>=2(sqrt(D^2+d^2D))<br>Fordark∈≥pathd⇔erenceshod––beoddμ<ip≤oflamda/2<br>So, 2(sqrt(D^2+d^2-D))=(2n+1)lamda/2<br>rarrsqrtD^2+d^2=D+(2n+1)lamda/4<br>rarr D^2+d^2=D^2+(2n+1)^2lamda^2/16+(2n+1)(lamdaD)/2<br>Neg≤ct∈g(2n+1)^2lamda^2/16asitisverysmallwe≥td=(sqrt2n+1)(lamdaD)/2<br>forminiμmdpu∈gn=0<br>rarr d_(min)=sqrt(((lamdaD)/2))
Topper's Solved these Questions
Similar Questions
Consider the arrangement shown in figure-6.38. The distance D is large compared to the separation d between the slits.
(a) Find the minimum value of d so that there is a dark fringe at O.
(b) Suppose d has this value. Find the distance x at which the next bright fringe is formed.
(c ) Find the fringe width.
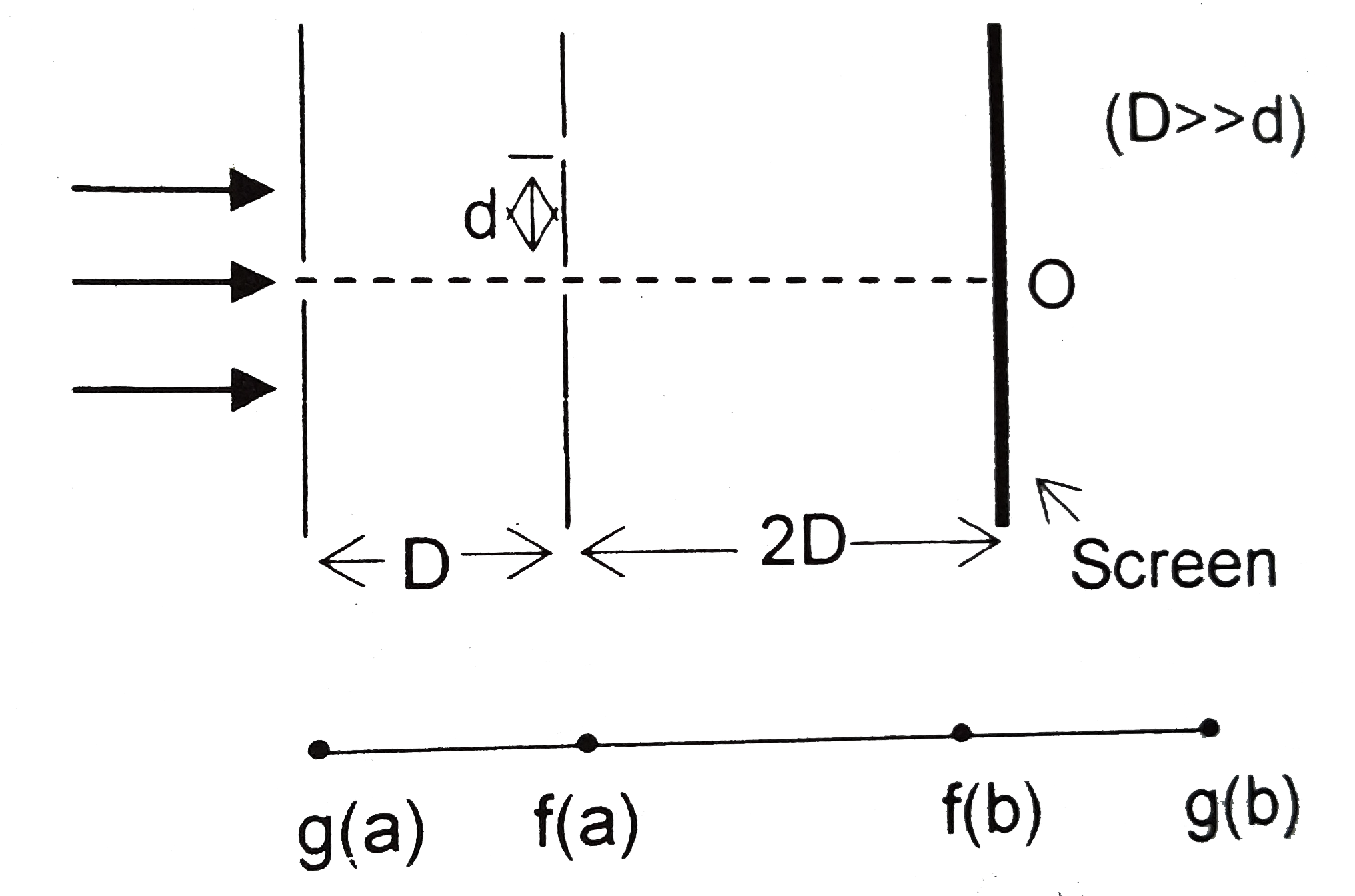
View SolutionThe minimum value of d os that there is a dark fringe at O is dmin. For the value of dmin, the distance at which the next bright fringe is formed is x. Then
View Solution
Knowledge Check
In a arrangement shown in the fig.the distance D is latge compared to the separation d between this slits ,find:
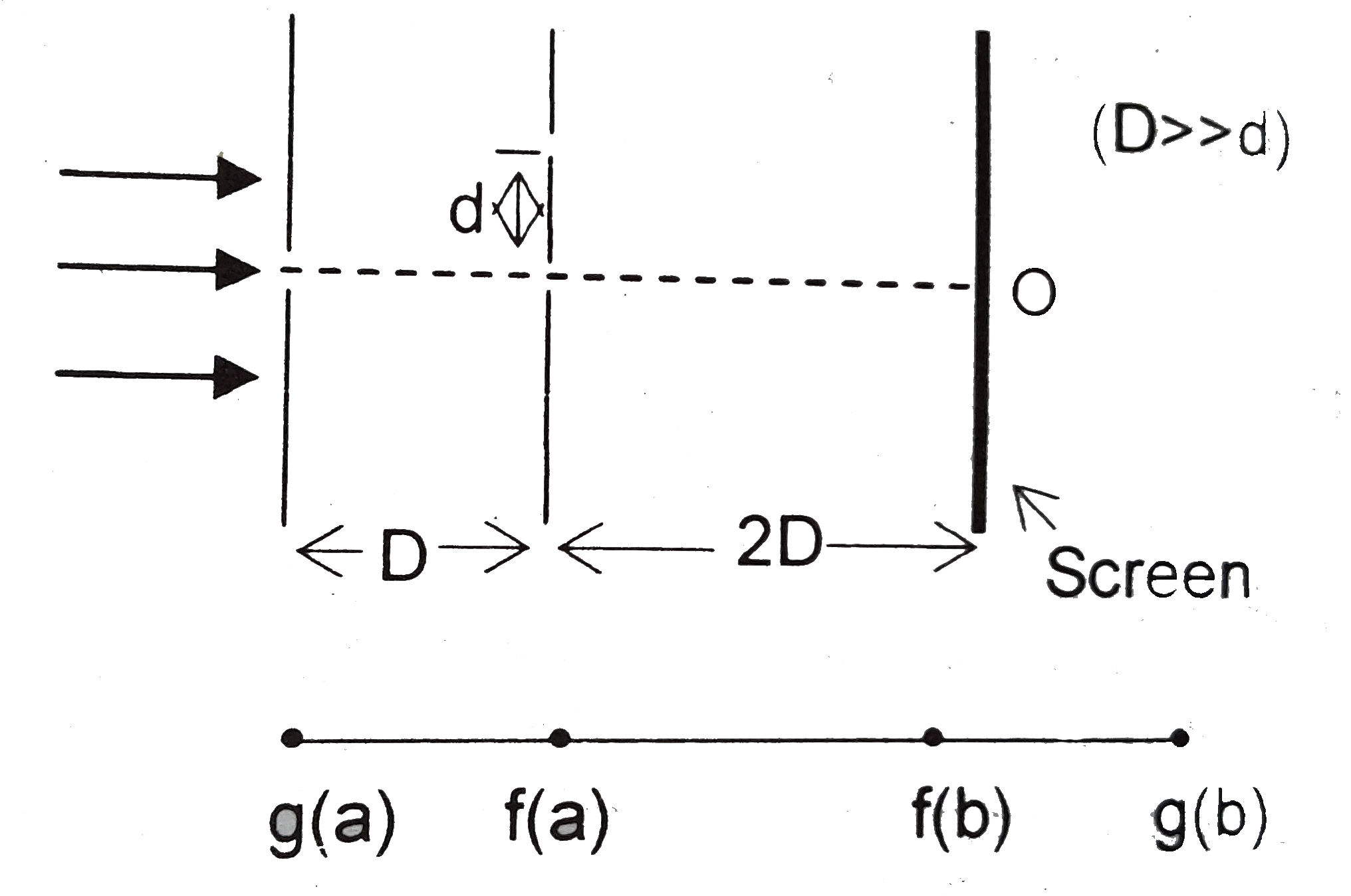
The minimum value of d for which there is a dark fringe at the point O.A√λD/2B√Dλ/3C√λDDcannot be calcualtedThe minimum value of d os that there is a dark fringe at O is dmin. For the value of dmin, the distance at which the next bright fringe is formed is x. Then
 Admin=√λDBdmin=√λD2Cx=dmin2Dx=dmin
Admin=√λDBdmin=√λD2Cx=dmin2Dx=dminIn a arrangement shown in the fig.the distance D is latge compared to the separation d between this slits ,find:
The fringe width isA3Dλ4dB3Dλ2dCDλdD2Dλd
In the arrangment shown in fin.

Find the distance x at which the next fringe is formed.
View SolutionConsider a two slit interference arrangements such that the distance of the screen from the slits is half the distance between the slits. Obtain the value of D in terms of λ such that the first minima on the screen falls at a distance D from the centre O.

View SolutionConsider a two slit interference arrangements (figure) such that the distance of the screen from the slits is half the distance between the slits. Obtain the value of D in terms of λ such that the first minima on the screen falls at a distance D from the centre O.
View SolutionIn the arrangment shown in fin.

Find the distance x at which the next fringe is formed.
View SolutionIn a arrangement shown in the fig.the distance D is latge compared to the separation d between this slits ,find:
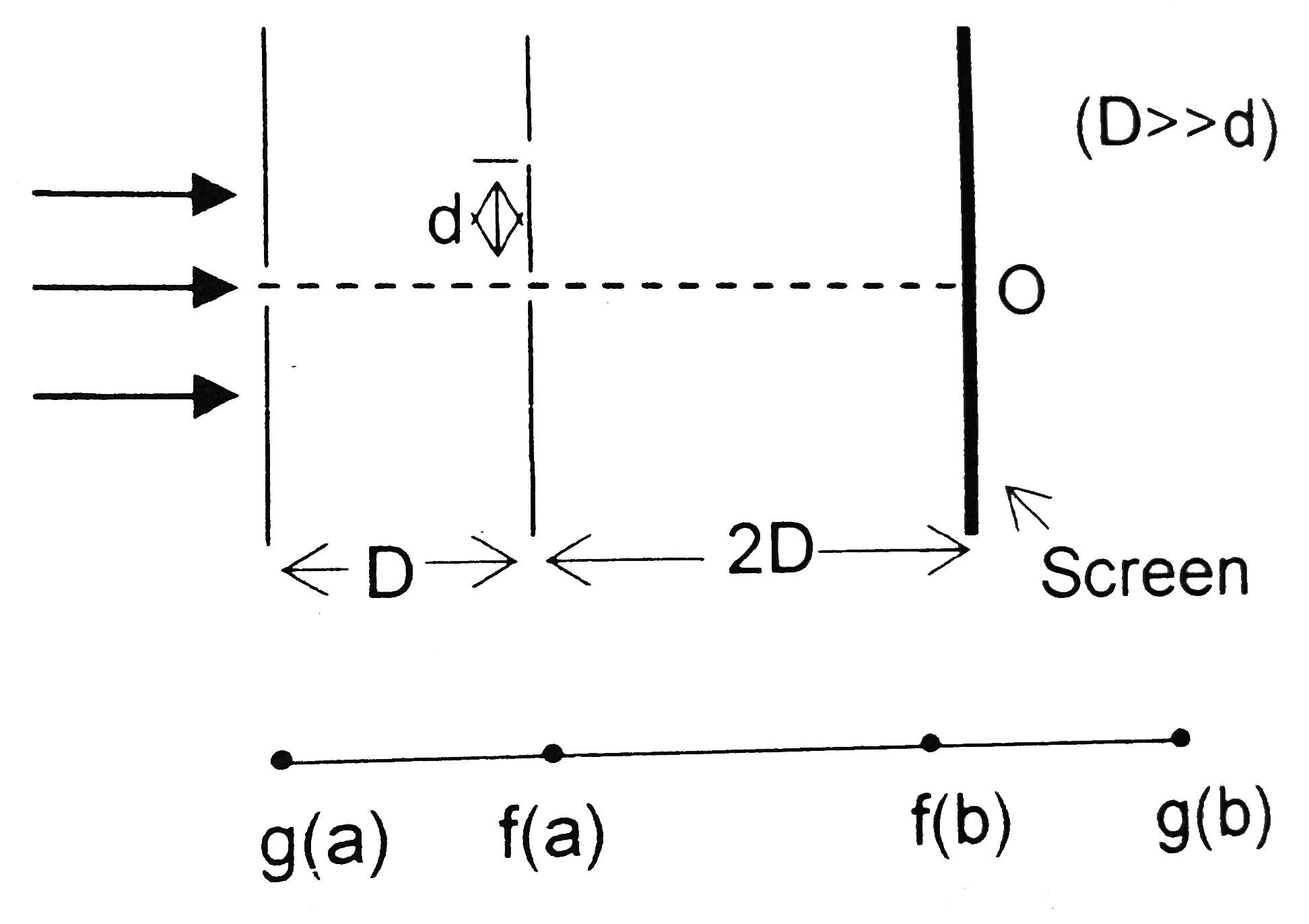
The position of first bright fringe for the minimum value of dis
View Solution
