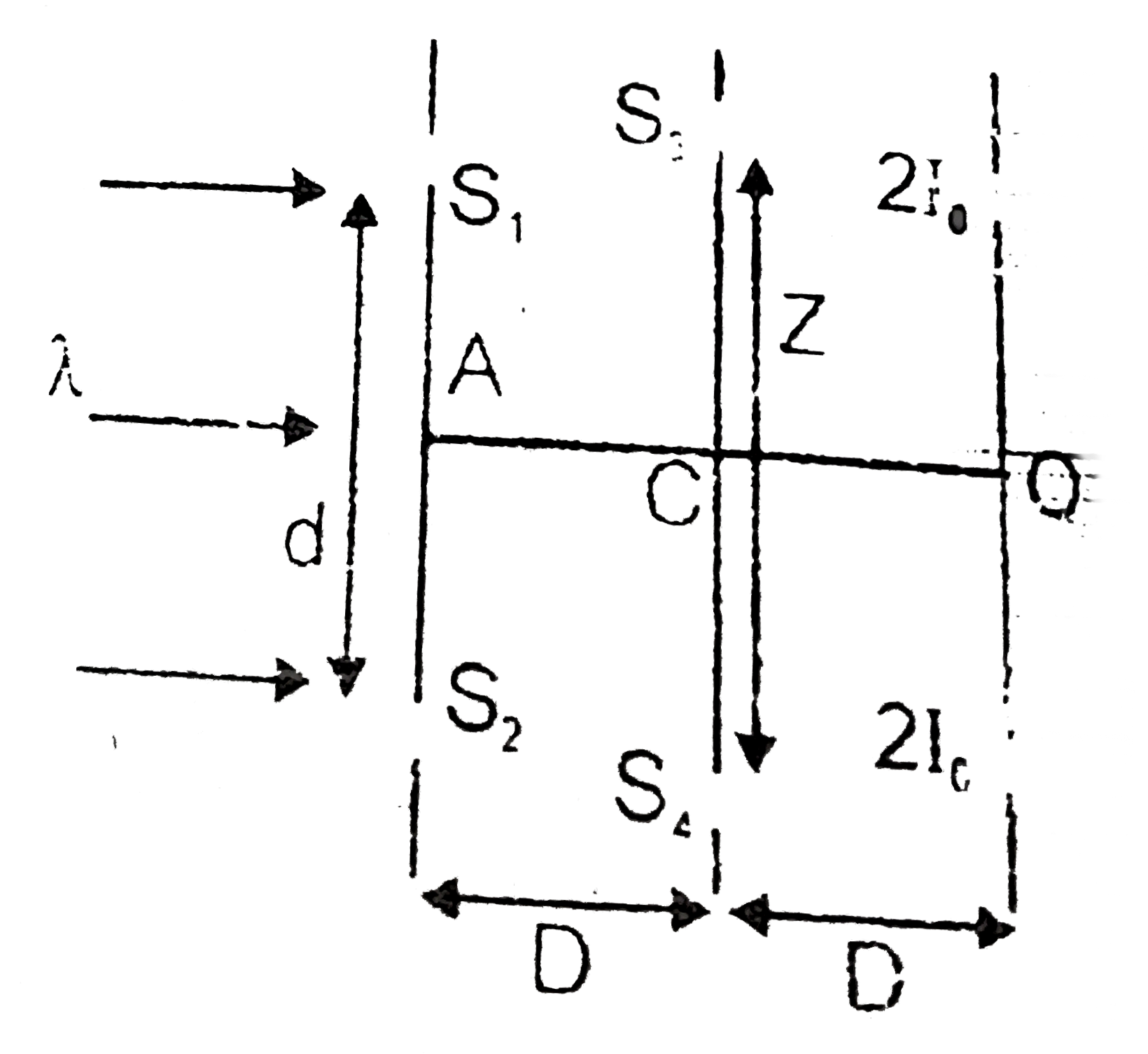
Consider the arrangement shownin figure. By some mechanism,the separation between the slits S3andS4 can be changed. The intensity in measured at the point P which is at the common perpendicular bisector of S1S2andS3S4.Whenz=(Dlamda)/(2d)the∫ensitymeasuredatPisI.F∈dthis∫ensitywhenzisequal→<br>a.Dλd,b.3Dλ2d,c.2Dλd

The correct Answer is:A, B, C
a. When z=λDd
So, OS3=OS4=λDd
→Dark∈≥atS_3 and S_4<br>rarr At S_3∫ensityatS_3=0<br>rarrl_1=0<br>AtS_4 Intensity at S_4=0<br>rarr l2=0
→AtPpathd⇔erence=0<br>rarr Phase difference =0<br>rarr l=l_1+l_2+2sqrt(l_1l_2)costheta^@=0+0+0=0<br>rarr intensilty at P=0
Topper's Solved these Questions
Similar Questions
Consider the arrangement shown in figure. By some mechanism,
the separation between the slits S3andS4 can be changed. The intensity is
measured at the point P which is at the common perpendicular bisector of S1S2
and S3S4. When z=Dλ2d, the intensity measured at P is I. Find the intensity when
z is equal to
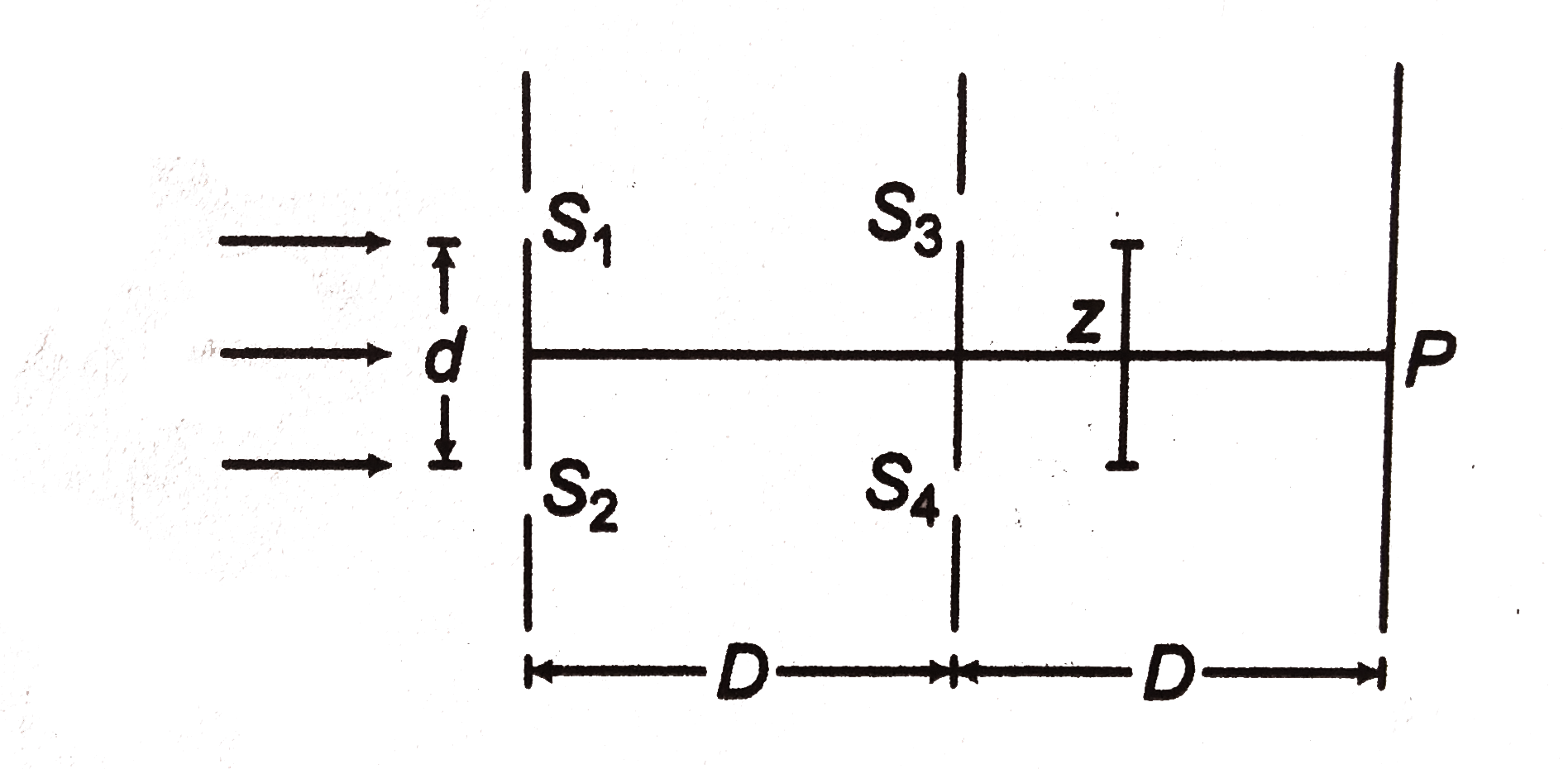
(a)Dλd(b)3Dλ2d(c)2Dλd .
View SolutionConsider the arrangement shown in figure. By some mechanism,
the separation between the slits S3andS4 can be changed. The intensity is
measured at the point P which is at the common perpendicular bisector of S1S2
and S3S4. When z=Dλ2d, the intensity measured at P is I. Find the intensity when
z is equal to

(a)Dλd(b)3Dλ2d(c)2Dλd .
View Solution
Knowledge Check
In the arrangement shown in Fig., slits S1 and S4are having a variable separation Z. Point O on the screen is at the common perpendicular bisector of S1S2 and S3S4.
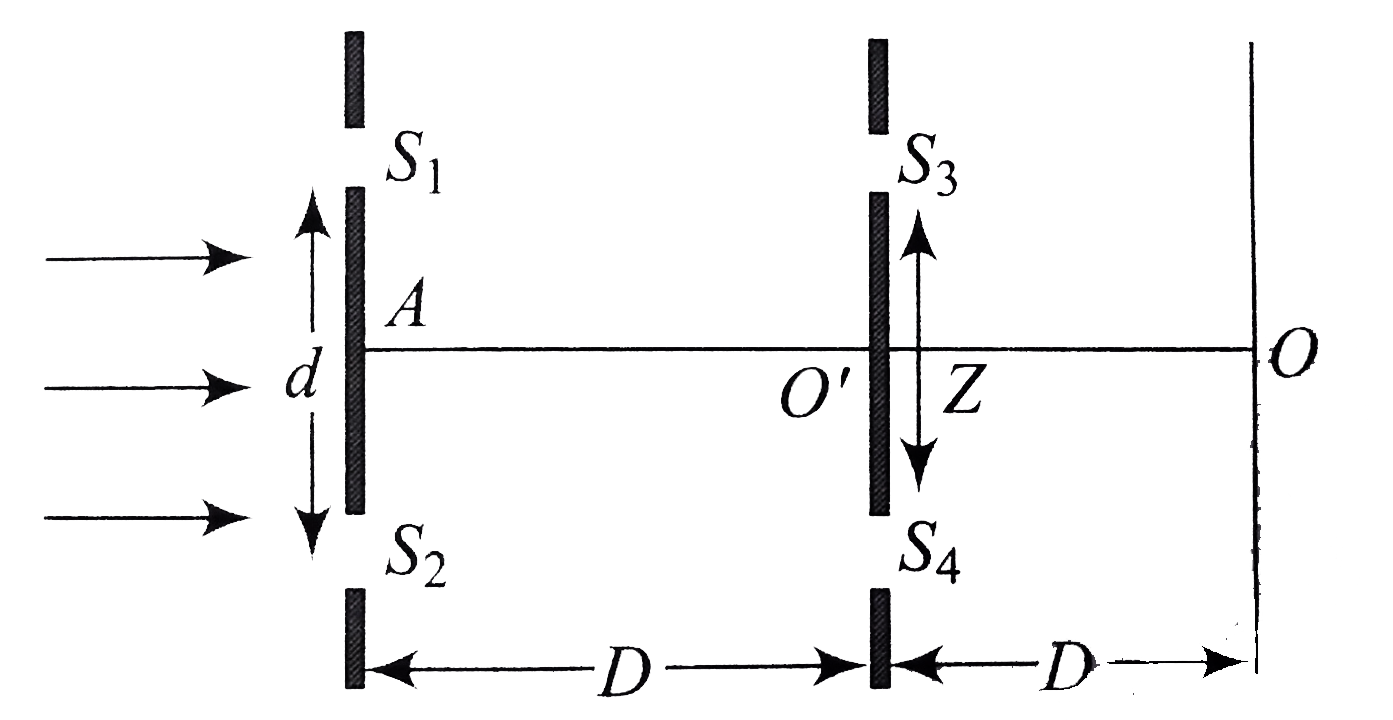
When Z=λD2d, the intensity measured at O is I0. The intensity at O When Z=2λDd isAI0B2I0C3I0D4I0In the arrangement shown in Fig., slits S1 and S4are having a variable separation Z. Point O on the screen is at the common perpendicular bisector of S1S2 and S3S4.
The minimum value of Z for which the intensity at O is zero isA2λDdBλD2dCλD3dDλDdIn the arrangement shown in Fig., slits S1 and S4are having a variable separation Z. Point O on the screen is at the common perpendicular bisector of S1S2 and S3S4.
If a hole is made at O' on AO' O and the slit S4 is closed, then the ratio of the maximum to minimum observed on screen at O , if O'S3 is equal to λD4d, isA1BinfinityC34D4
Parallel monochromatic beam is falling normally on two slits S1 and S2 separated by d as shown in figure. By some mechanism, the separation between the slits S3 and S4 can be changed. The intensity is measured at the point P which is at the common perpendicular bisector of S1S2 and S3S4. When z=Dλ2d, the intensity measured at P is I. and when z is equal to 4Dλd, Intensity is xl. Find x.

View SolutionIn the arrangement shown in Fig., slits S1 and S4are having a variable separation Z. Point O on the screen is at the common perpendicular bisector of S1S2 and S3S4.

When Z=λD2d, the intensity measured at O is I0. The intensity at O When Z=2λDd is
View SolutionIn the arrangement shown in Fig., slits S1 and S4are having a variable separation Z. Point O on the screen is at the common perpendicular bisector of S1S2 and S3S4.

The minimum value of Z for which the intensity at O is zero is
View SolutionIn the arrangement shown in Fig., slits S1 and S4are having a variable separation Z. Point O on the screen is at the common perpendicular bisector of S1S2 and S3S4.

If a hole is made at O' on AO' O and the slit S4 is closed, then the ratio of the maximum to minimum observed on screen at O , if O'S3 is equal to λD4d, is
View SolutionIn arrangement shown in figure, plane wavefront of monochromatic light of wavelength λ is incident on identical slits S1 and S2. There is another pair of indentical slits S3 and S4 which are having separation Z=λD2d Point O is on the screen at the common perpendicular bisector of S1S2 and S3S4.I1 is the intensity at point O Now the board having slits S3S4 is moved upward parallel to itself and perpendicular to line AO till slit S4 is on line AO and it is observed that now intensity at point O is I2 then I2I1 is :
View Solution
