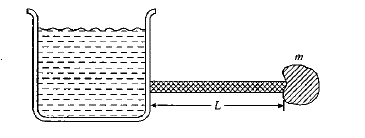
Figure shows a large tank of water at a constant temperature $θ_{0}$ . and a small vessel containinng a mass m of water at an initial temperature $θ (< θ_{0} . A m η l r o d o f \leq n > h L, a r e a o f c r o s s sec t i o n A and t h e r m a l c o n d u c t i v i t y K c o \cap e c t t h e t w o v e s s e l s . F \in d t h e t i m e t a k e n f or t h e t e m p e r a t u r e o f t h e w a t e r \in t h e s m a l \leq r v e s s e l \to b e c o m e$ theta_(2)(theta_(1)lttheta_(2)lttheta_(0)) . Specific heat capacity of water is s and all other heat capacities are negligible.

Video Solution

Text Solution

Verified by Experts

Suppose, the temperature of the water in the smaller vessel is theta at time t. In the next time interval dt, a heat (DeltaQ) is transferred to it where
$Δ Q = \frac{K A}{L} (θ_{0} - θ) d t$ . This heat increases the temperature of the water of mass m to $θ + d θ w h e r e$ DeltaQ=msd theta $. F r o m (i) and (i i), < b r > \frac{K A}{L} (θ_{0} - θ) d t = m s d θ$ . or, $d t = \frac{L m s}{K A} \frac{d θ}{θ_{0} - θ}$ . or, $\int_{0}^{T} d t = \frac{L m s}{K A} \int_{θ_{1}}^{θ^{2}} \frac{d θ}{θ_{0} - θ}$ . where T is the time requierd for the temperature of the water to become $θ_{2}$ . Thus, $T = \frac{L m s}{K A} ln$ (theta_(0)-theta_(1))/(theta_(0)-theta_(2)) .

Updated on:21/07/2023

Class 11 PHYSICS THERMAL EXPANSION AND CALORIMETRY

Topper's Solved these Questions

Knowledge Check

Two liquids of specific heat ratio 1:2 are at temperature $2 θ and θ$
Aif equal amounts of them are mixed, then temperature of mixture is $1.5 θ$
Bif equal amounts of them are mixed, then temperature of mixture is $\frac{4}{3} θ$
Cfor their equal amounts, the ratio of heat capacities is 1:1
Dfor their equal amounts, the ratio of their heat capacities is 1:2.
Two adiabatic vessels, each containing the same mass m of water but at different temperatures, are connected by a rod of length L, cross-section A, and thermal conductivity K. the ends of the rod are inserted into the vessels, while the rest of the rod is insulated so that .there is negligible loss of heat into the atmosphere. The specific heat capacity of water is s, while that of the rod is negligible. The temperature difference between the two vessels reduces to $l / e$ of its original value after a time, $δ t$ . The thermal conductivity (K) of the rod may be expressed by:
A $\frac{m s L}{A δ t}$
B $\frac{e m s L}{A δ t}$
C $\frac{m s L}{2 e A δ t}$
D $\frac{m s L}{2 A δ t}$
Two rods of same length and cross section are joined along the length. Thermal conductivities of first and second rod are $K_{1}$ and $K_{2}$ . The temperature of the free ends of the first and seconds rods are maintained at $θ_{1}$ and $θ_{2}$ respectively. The temperature of the common junction is
A $\frac{θ_{1} + θ_{2}}{2}$
B $\frac{K_{2} K_{2}}{K_{1} + K_{2}} (θ_{1} + θ_{2})$
C $\frac{K_{1} θ_{1} + K_{2} θ_{2}}{K_{1} + K_{2}}$
D $\frac{K_{2} θ_{1} + K_{1} θ_{2}}{K_{1} + K_{2}}$

HC VERMA-HEAT TRANSFER-Worked Out Examples

Home
Ask Doubt
Profile

Topper's Solved these Questions

Similar Questions

Knowledge Check

Two liquids of specific heat ratio 1:2 are at temperature 2θandθ

Two rods of same length and cross section are joined along the length. Thermal conductivities of first and second rod are K1 and K2. The temperature of the free ends of the first and seconds rods are maintained at θ1 and θ2 respectively. The temperature of the common junction is

HC VERMA-HEAT TRANSFER-Worked Out Examples

Two liquids of specific heat ratio 1:2 are at temperature $2 θ and θ$