A and B are two thin concentric hollow conductors having radii a and 2a and charge 2Q and Q, respectively. If potential of outer sphere is 5V, then potential of inner sphere is
20 V
10 V
253V
503V
The correct Answer is:C
To find the potential of the inner sphere (A) given the potential of the outer sphere (B) and their respective charges, we can follow these steps:
1. Identify the Charges and Radii:
- Charge on inner sphere (A): 2Q
- Charge on outer sphere (B): Q
- Radius of inner sphere (A): a
- Radius of outer sphere (B): 2a
2. Formula for Electric Potential:
The electric potential V at a distance r from a point charge Q is given by:
V=kQr
where k is Coulomb's constant.
3. Calculate the Potential of the Outer Sphere:
The potential VB at the surface of the outer sphere (radius 2a) due to both the inner and outer charges is:
VB=k(2Q)2a+k(Q)2a
Simplifying this:
VB=k(2Q)2a+k(Q)2a=2kQ2a+kQ2a=3kQ2a
We know from the problem statement that VB=5V:
3kQ2a=5
4. Solve for kQ/a:
Rearranging the equation gives:
kQ=10a3
5. Calculate the Potential of the Inner Sphere:
The potential VA at the surface of the inner sphere (radius a) is given by:
VA=k(2Q)a+k(Q)2a
Substituting kQ from the previous step:
VA=2kQa+kQ2a
This simplifies to:
VA=2⋅kQa+12⋅kQa=2kQ/a+kQ2a
Converting 2kQ/a into a common denominator:
VA=4kQ2a+kQ2a=5kQ2a
6. Substituting kQ/a into VA:
Now, substituting kQ/a=103:
VA=52⋅103=506=253V
Final Answer:
The potential of the inner sphere (A) is:
VA=253V
- Charge on inner sphere (A): 2Q
- Charge on outer sphere (B): Q
- Radius of inner sphere (A): a
- Radius of outer sphere (B): 2a
The electric potential V at a distance r from a point charge Q is given by:
V=kQr
where k is Coulomb's constant.
The potential VB at the surface of the outer sphere (radius 2a) due to both the inner and outer charges is:
VB=k(2Q)2a+k(Q)2a
Simplifying this:
VB=k(2Q)2a+k(Q)2a=2kQ2a+kQ2a=3kQ2a
We know from the problem statement that VB=5V:
3kQ2a=5
Rearranging the equation gives:
kQ=10a3
The potential VA at the surface of the inner sphere (radius a) is given by:
VA=k(2Q)a+k(Q)2a
Substituting kQ from the previous step:
VA=2kQa+kQ2a
This simplifies to:
VA=2⋅kQa+12⋅kQa=2kQ/a+kQ2a
Converting 2kQ/a into a common denominator:
VA=4kQ2a+kQ2a=5kQ2a
Now, substituting kQ/a=103:
VA=52⋅103=506=253V
The potential of the inner sphere (A) is:
VA=253V
Topper's Solved these Questions
- ELECTROSTATIC POTENTIAL AND CAPACITORSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITORSEXERCISE - (B) Chapter exercises
17 Videos
- ELECTROSTATIC POTENTIAL AND CAPACITORSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITORSEXERCISE - (C) Chapter exercises
50 Videos
- ELECTROSTATIC POTENTIAL AND CAPACITORSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITORSEXERCISE - Check point 2.5
20 Videos
- ELECTROMAGNETIC WAVESBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - Sec C
22 Videos
- ELECTROSTATICSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - Medical entrances gallery
37 Videos
Similar Questions
Two concentric conducting shells of radii R and 2R are having charges Q and -2Q respectively.In a region r
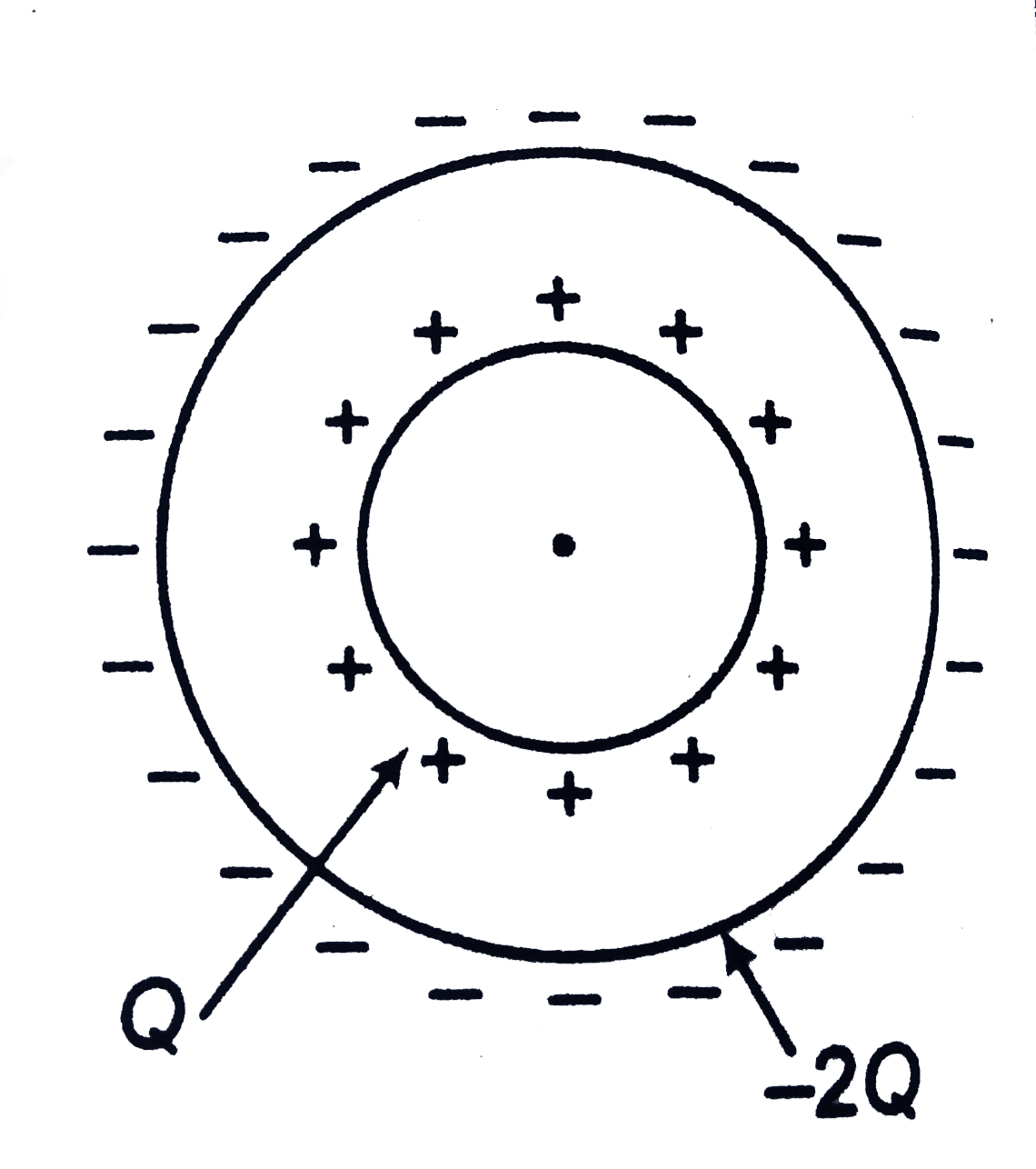
View SolutionTwo concentric conducting spheres of radii R and 2R are crrying charges Q and −2Q, respectively. If the charge on inner sphere is doubled, the potetial differece between the two spheres will
View SolutionTwo hollow concentric non-conducting spheres of radius the a and b(a>b) contain charges Qa and Qb respectively. Prove that potential difference between the two spheres is independent of charge on outer sphere. If outer sphere is given an extra charge, is there any change in potential difference ?
View SolutionConcentric metallic hollow spheres of radii R and 4R hold charges Q1andQ2 respectively. Given that surface charge densities of the concentric spheres are equal, the potential difference V(R)−V(4R) is :
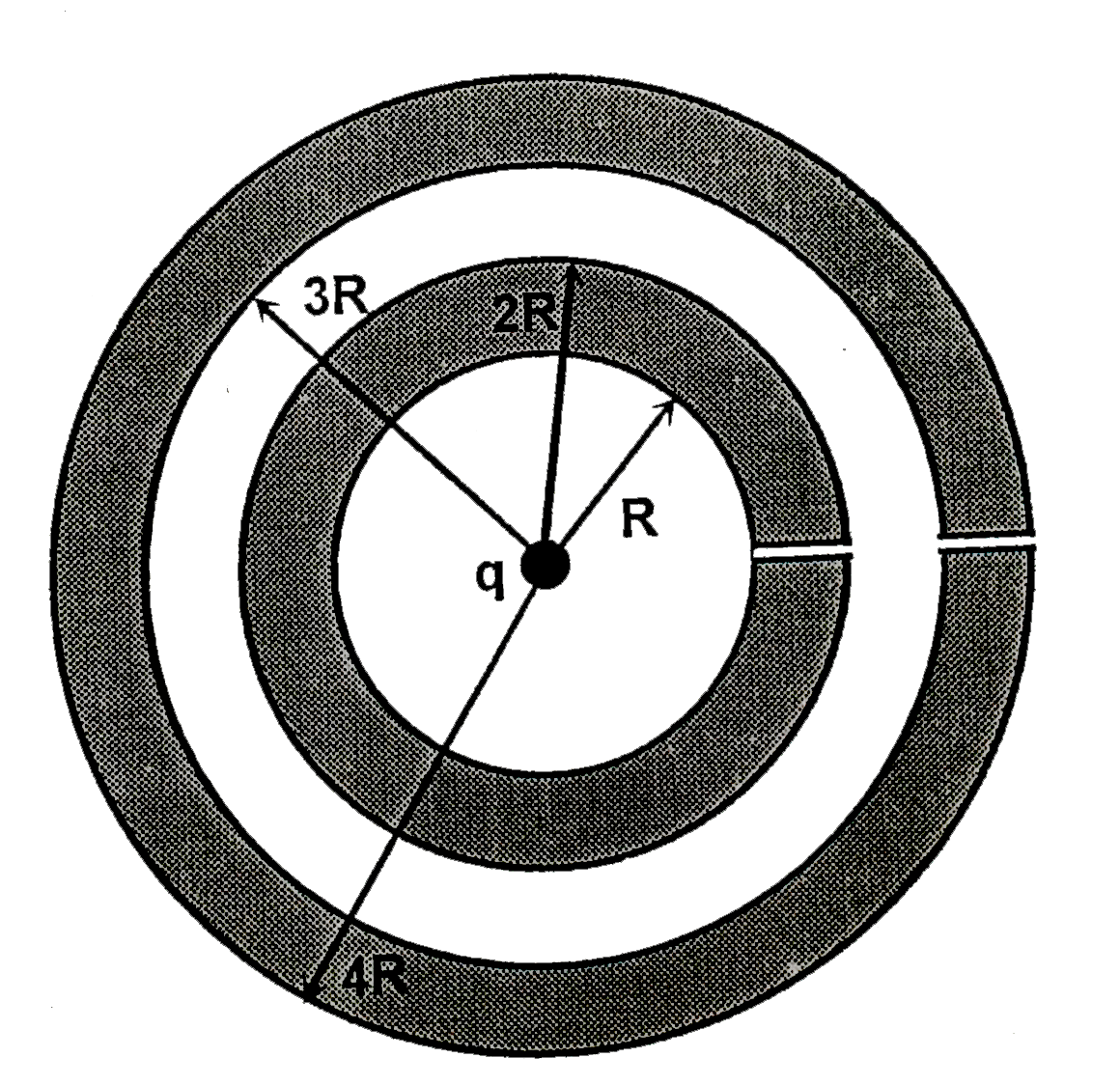
View SolutionA charge particle 'q' lies at the centre of two concentric hollow sphere of inner radii R and 3R and outer radii 2R and 4R respectively. What amount of work has to be performed to slowly transfer the charge 'q' from center rough the orifice to infinity.
View SolutionTwo concentric hollow conducting spheres of radius a and b (b>a) contains charges Qa and Qb respectively. If they are connected by a conducting wire then find out following
(i) Final charges on inner and outer spheres.
(ii) Heat produced during the process.
View SolutionThere are two thin concentric conducting shells of radii a and b(a<b) . Equal charges Q given to each shell. Find the charge distribution on the inner and outer shell.
View SolutionThe insulated spheres of radii R1 and R2 having charges Q1 and Q2 respectively are connected to each other. There is
View SolutionThe insulated spheres of radii R1 and R2 having charges Q1 and Q2 respectively are connected to each other. There is
View SolutionStatement-1 : If two concentric conducing sphere which are connected by a conducting wire. No charge can exist on inner sphere.
Statement-2: When charge on outer sphere will exist then potential of inner shell and outer shell will be same.
View Solution
