A pendulum bob of mass m charge q is at rest with its string making an angle θ with the vertical in a uniform horizontal electric field E. The tension in the string in
mgsinθ
mg
qEsinθ
qEcosθ
The correct Answer is:C
To find the tension in the string of a pendulum bob of mass m and charge q that is at rest while making an angle θ with the vertical in a uniform horizontal electric field E, we can follow these steps:
Step 1: Understand the Forces Acting on the Pendulum Bob
The forces acting on the pendulum bob are:
1. The weight of the bob, mg, acting vertically downward.
2. The tension in the string, T, acting along the string.
3. The electrostatic force due to the electric field, FE=qE, acting horizontally in the direction of the electric field.
Step 2: Resolve the Tension into Components
The tension T can be resolved into two components:
- Vertical component: Tcosθ
- Horizontal component: Tsinθ
Step 3: Apply the Conditions for Equilibrium
Since the pendulum bob is at rest, it is in equilibrium. Therefore, we can set up the following equations based on the equilibrium conditions:
Vertical Equilibrium
In the vertical direction, the upward force (tension's vertical component) must balance the downward force (weight of the bob):
Tcosθ=mg(1)
Horizontal Equilibrium
In the horizontal direction, the horizontal component of tension must balance the electrostatic force:
Tsinθ=qE(2)
Step 4: Solve for Tension T
From equation (1):
T=mgcosθ(3)
From equation (2):
T=qEsinθ(4)
Step 5: Equate the Two Expressions for Tension
Since both equations (3) and (4) represent the tension T, we can set them equal to each other:
mgcosθ=qEsinθ
Step 6: Rearranging to Find Tension
From this equation, we can express T in terms of either mass, charge, electric field, and angle:
T=qEsinθ(from equation (4))
Final Expression for Tension
Thus, the tension in the string is given by:
T=qEsinθ
Conclusion
The correct expression for the tension in the string of the pendulum bob is:
T=qEsinθ
The forces acting on the pendulum bob are:
1. The weight of the bob, mg, acting vertically downward.
2. The tension in the string, T, acting along the string.
3. The electrostatic force due to the electric field, FE=qE, acting horizontally in the direction of the electric field.
The tension T can be resolved into two components:
- Vertical component: Tcosθ
- Horizontal component: Tsinθ
Since the pendulum bob is at rest, it is in equilibrium. Therefore, we can set up the following equations based on the equilibrium conditions:
In the vertical direction, the upward force (tension's vertical component) must balance the downward force (weight of the bob):
Tcosθ=mg(1)
In the horizontal direction, the horizontal component of tension must balance the electrostatic force:
Tsinθ=qE(2)
From equation (1):
T=mgcosθ(3)
T=qEsinθ(4)
Since both equations (3) and (4) represent the tension T, we can set them equal to each other:
mgcosθ=qEsinθ
From this equation, we can express T in terms of either mass, charge, electric field, and angle:
T=qEsinθ(from equation (4))
Thus, the tension in the string is given by:
T=qEsinθ
The correct expression for the tension in the string of the pendulum bob is:
T=qEsinθ
Topper's Solved these Questions
- ELECTROSTATICSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - Level 1 Subjective
15 Videos
- ELECTROSTATICSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - SUBJECTIVE_TYPE
6 Videos
- ELECTROSTATICSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - Level 1 Assertion And Reason
19 Videos
- ELECTROSTATIC POTENTIAL AND CAPACITORSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITORSEXERCISE - (C) Chapter exercises
50 Videos
- GRAVITATIONBOOK - DC PANDEY ENGLISHCHAPTER - GRAVITATIONEXERCISE - All Questions
135 Videos
Similar Questions
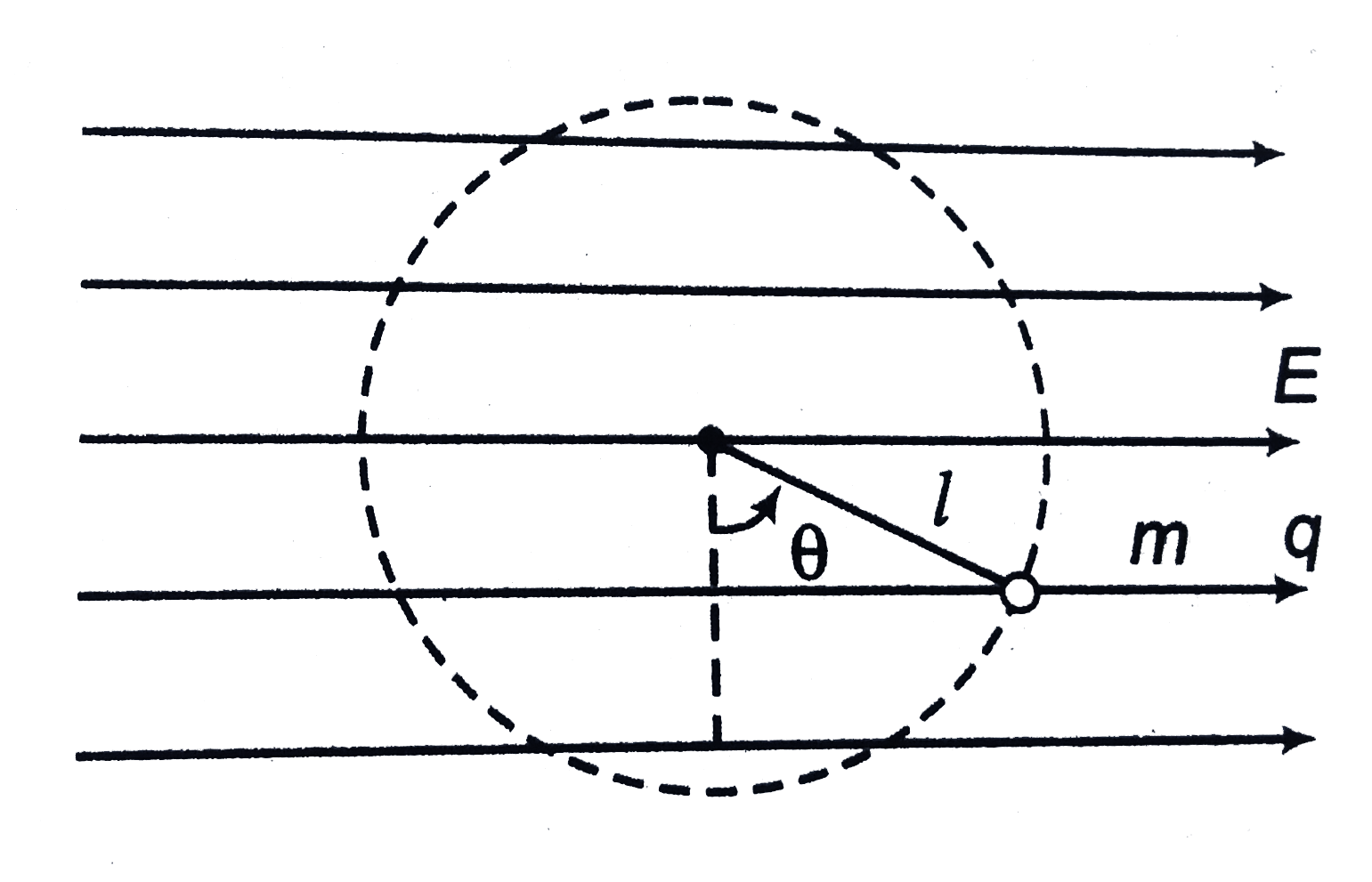
A small ball of masss m and charge +q tied with a string of length l, rotating in a veticle circle under gravity and a uniform horizontal electric field E as shown. The tension in the string will be minimum for
View SolutionA pendulum bob of mass 80mg and carrying a charge of 2×10−8C is at rest in a uniform, horizontal electric field of 20k Vm−1. Find the tension in the thread.
View SolutionA small ball is suspended by a string from the ceiling of a car. As the car accelerates at a rate ‘a’ the string makes an angle '/theta' with the vertical in equilibrium. Then the tension in the string is :
View SolutionThe block of mass m is at rest. Find the tension in the string A .

View SolutionA pendulum bob of mass m = 80 mg, carrying a charge of q=2×10−8C, is at rest in a horizontal, uniform electric field of E = 20,000 V/m. The tension T in the thread of the pendulum and the angle α it makes with vertical, is (take g=9.8m/s2)
View SolutionA simple pendulum is constructed by attaching a bob of mass m to a string of length L fixed at its upper end. The bob oscillates in a vertical circle. It is found that the speed of the bob is v when the string makes an angle θ with the vertical. Find the tension in the string at this instant.
View SolutionA simple pendulum is constructed by attaching a bob of mas m to a string of length L fixed at its upper end. The bob oscillates in a vertical circle. It is found that the speed of the bob is v when the string makes an angle θ with the vertical. Find the tension in the string at this instant.
View SolutionA body of mass m is suspended by two strings making angles αandβ with the horizontal. Find the tensions in the strings.
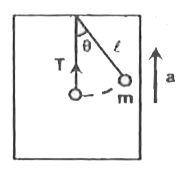
View SolutionA particle of mass m is attached to the celling of a cabin with an inextensible light string of length l. The cabin is moving upward with an acceleration a . The particle is taken to a position such that string makes an angle θ with vertical. When string becomes vertical, find the tension in the string.
View SolutionA simple pendulum consists of a 50 cm long string connected to a 100 g ball. The ball is pulled aside so that the string makes an angel of 370 with the vertical and is then released. Find the tension in the string when the bob is at its lowest position.

View Solution
