A pendulum of mass m hangs from a support fixed to a trolley. The direction of the string when the trolley rolls up a plane of inclination $α$ with acceleration $a_{0}$ is

A

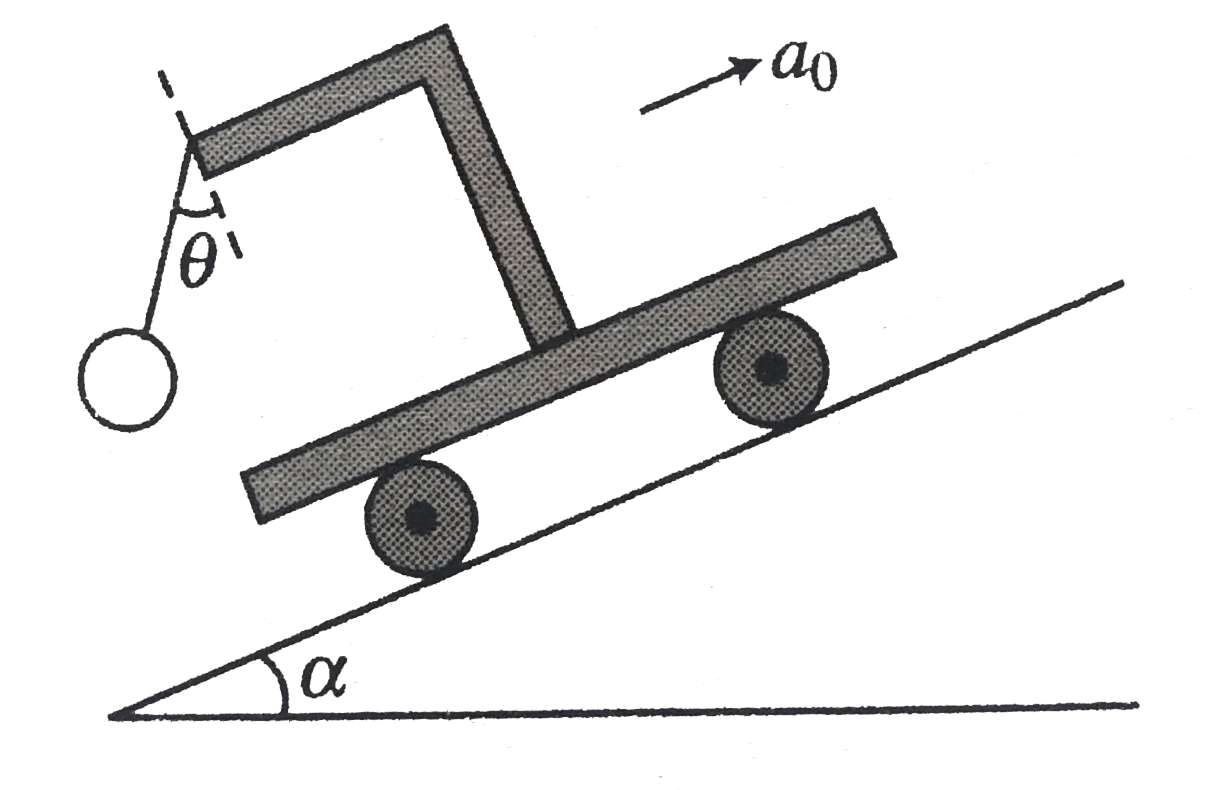
$θ = {tan}^{- 1} α$

B

$θ = {tan}^{1} (\frac{a_{0}}{g})$

C

$θ = {tan}^{1} (\frac{g}{a_{0}})$

D

$θ = {tan}^{1} (\frac{a_{0} + g sin α}{g cos α})$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:D

As in fig.

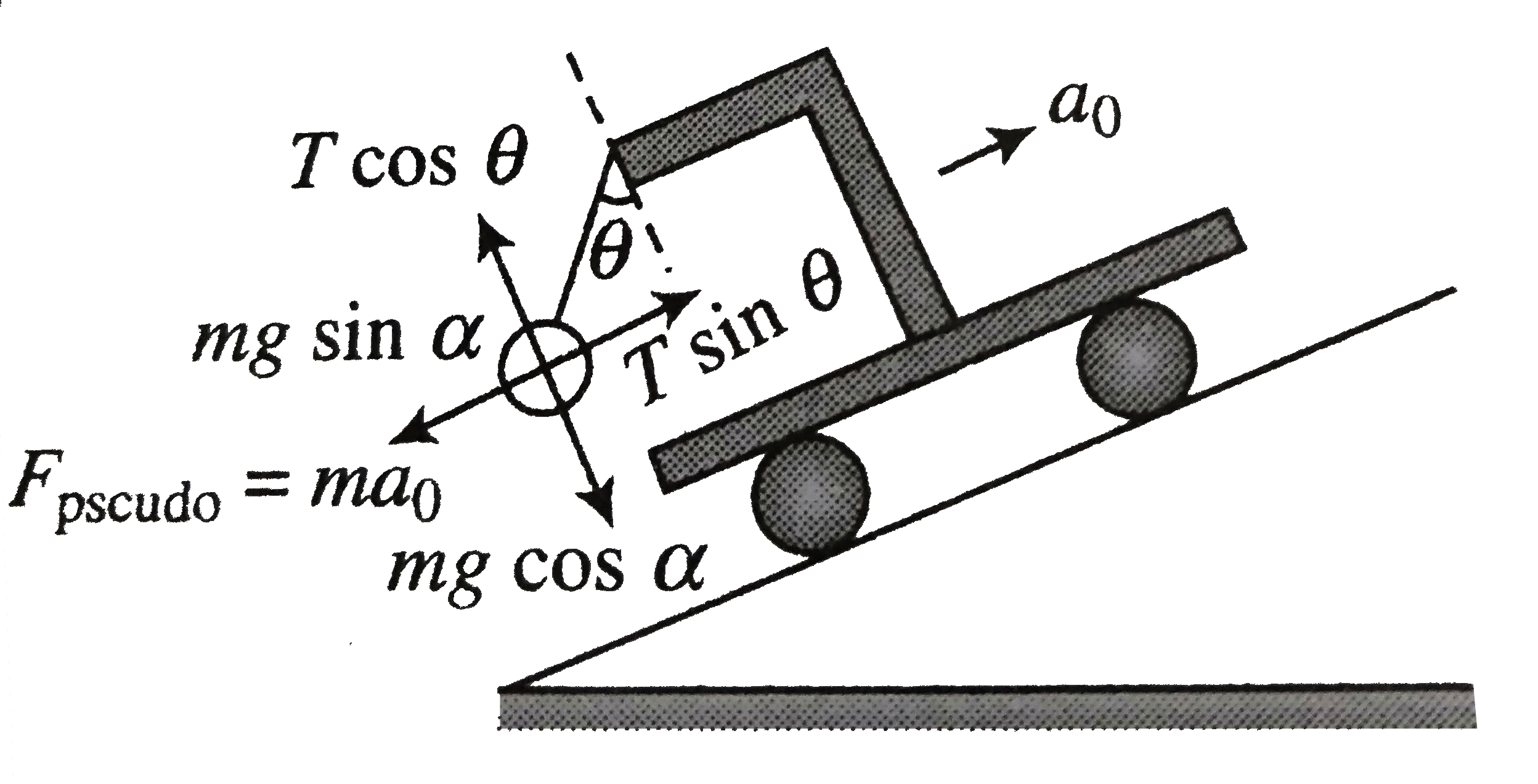
$T sin θ = m a_{0} + m g sin α$
$T cos θ = m g cos α$
$tan θ = \frac{a_{0} + g sin α}{g cos θ}$ .

|

Updated on:21/07/2023

Class 11 PHYSICS NEWTONS LAW OF MOTION

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
A pendulum of mass m hangs from a support fixed to a trolley which is moving up to the inclined plane with uniform acceleration 'a'. The angle which the string makes with vertical in equilibrium is

A0
B ${tan}^{- 1} α$
C ${tan}^{- 1} (\frac{a + g sin α}{g cos α})$
D ${tan}^{- 1} (\frac{a cos α}{g + a sin α})$
Question 2 - Select One
A pendulum has a ball of mass m attached to the stringa and is suspended from the roof of a trolley. If the trolley rolls upwards with acceleration a, then what is the angle made by the string with the inclined plane? (Given that, $a = 5 m / s^{2}, g = 10 m / s^{2}$ and the angle of inclination of the plane is $30^{\circ}$ )
A ${cos}^{- 1} (\frac{2}{\sqrt{3}})$
B ${cot}^{- 1} (\frac{2}{\sqrt{3}})$
C ${sin}^{- 1} (\frac{2}{\sqrt{3}})$
D ${tan}^{- 1} (\frac{2}{\sqrt{3}})$
Question 3 - Select One
A pendulum bob is hanging from the roof of an elevator with the help of a light string. When the elevator moves up with uniform acceleration 'a' the tension in the string is $T_{1}$ .When the elevator moves down with the same acceleration, the tension in the string is $T_{1}$ .If the elevator were stationary, the tension in the string would be
A $\frac{T_{1} + T_{2}}{2}$
B $\sqrt{T_{1} + T_{2}}$
C $\frac{T_{1} T_{2}}{T_{1} + T_{2}}$
D $\frac{2 T_{1} T_{3}}{T_{1} + T_{2}}$

CENGAGE PHYSICS-NEWTON'S LAWS OF MOTION 1-Single Correct

Home
Ask Doubt
Profile