A rectangular, a square , a circular and an elliptical loop, all in the (x−y) plane, are moving out of a uniform magnetic field with a constant velocity →v=vˆi . The magnetic field is directed along the negative z-axis direction. The induced emf, during the passage of these loops , out of the field region, will not remain constant for :
the rectangular, circular and elliptical loops
the circular and the elliptical loops
only the elliptical loop
any of the four loops
The correct Answer is:B
To solve the problem, we need to analyze the induced electromotive force (emf) in the different shapes of loops as they move out of a uniform magnetic field. The key point here is to understand how the area of the loops that is within the magnetic field changes over time.
1. Understand the Setup:
- We have four types of loops: rectangular, square, circular, and elliptical.
- All loops are in the (x-y) plane and moving out of a uniform magnetic field directed along the negative z-axis with a constant velocity →v=v^i.
2. Induced EMF Formula:
- The induced emf (E) in a loop moving out of a magnetic field can be calculated using Faraday's law of electromagnetic induction:
E=−dΦBdt
- Where ΦB is the magnetic flux through the loop, given by:
ΦB=B⋅A
- Here, B is the magnetic field strength and A is the area of the loop within the magnetic field.
3. Analyze Each Loop:
- Rectangular Loop:
- As it moves out of the magnetic field, the area A that is within the field decreases uniformly. Therefore, dAdt is constant, leading to a constant induced emf.
- Square Loop:
- Similar to the rectangular loop, the area decreases uniformly as it exits the magnetic field. Thus, E remains constant.
- Circular Loop:
- As the circular loop exits the magnetic field, the area that is within the field changes in a non-linear manner. The rate of change of area dAdt is not constant throughout the motion, leading to a variable induced emf.
- Elliptical Loop:
- The situation is similar to the circular loop. The area changes non-linearly as it exits the magnetic field, resulting in a variable induced emf.
4. Conclusion:
- The induced emf will not remain constant for the circular and elliptical loops due to the non-linear change in area as they exit the magnetic field. In contrast, the rectangular and square loops have a constant rate of change of area and thus a constant induced emf.
Final Answer:
The induced emf will not remain constant for the circular and elliptical loops.
---
- We have four types of loops: rectangular, square, circular, and elliptical.
- All loops are in the (x-y) plane and moving out of a uniform magnetic field directed along the negative z-axis with a constant velocity →v=v^i.
- The induced emf (E) in a loop moving out of a magnetic field can be calculated using Faraday's law of electromagnetic induction:
E=−dΦBdt
- Where ΦB is the magnetic flux through the loop, given by:
ΦB=B⋅A
- Here, B is the magnetic field strength and A is the area of the loop within the magnetic field.
- Rectangular Loop:
- As it moves out of the magnetic field, the area A that is within the field decreases uniformly. Therefore, dAdt is constant, leading to a constant induced emf.
- Square Loop:
- Similar to the rectangular loop, the area decreases uniformly as it exits the magnetic field. Thus, E remains constant.
- As the circular loop exits the magnetic field, the area that is within the field changes in a non-linear manner. The rate of change of area dAdt is not constant throughout the motion, leading to a variable induced emf.
- The situation is similar to the circular loop. The area changes non-linearly as it exits the magnetic field, resulting in a variable induced emf.
- The induced emf will not remain constant for the circular and elliptical loops due to the non-linear change in area as they exit the magnetic field. In contrast, the rectangular and square loops have a constant rate of change of area and thus a constant induced emf.
The induced emf will not remain constant for the circular and elliptical loops.
Topper's Solved these Questions
- BOOK - DC PANDEY ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Medical entrance special format questions17videos
ELECTROMAGNETIC INDUCTION
- BOOK - DC PANDEY ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Match the columns5videos
ELECTROMAGNETIC INDUCTION
- BOOK - DC PANDEY ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Check point60videos
ELECTROMAGNETIC INDUCTION
- BOOK - DC PANDEY ENGLISHCHAPTER - CURRENT ELECTRICITYEXERCISE - Medical entrances gallery97videos
CURRENT ELECTRICITY
- BOOK - DC PANDEY ENGLISHCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - Sec C22videos
ELECTROMAGNETIC WAVES
Similar Questions
A rectangular loop and a circular loop are moving out of a uniform magnetic field to a field-free region with a constant velocity 'v' as shown in the figure . Explain the which loop do you expect the induced emf to the constant during the passage out of the field region . The magnetic field is normal to the loops .
View SolutionA semi-circular loop of radius R is placed in a uniform magnetic field as shown. It is pulled with a constant velocity. The induced emf in the loop is
View SolutionA closed loop with the geometry shown in Fig. 3.9 is placed in a uniform magnetic field direction into the plane of the paper. If the magnetic field decreases with time, determine the direction of the induced emf in this loop.
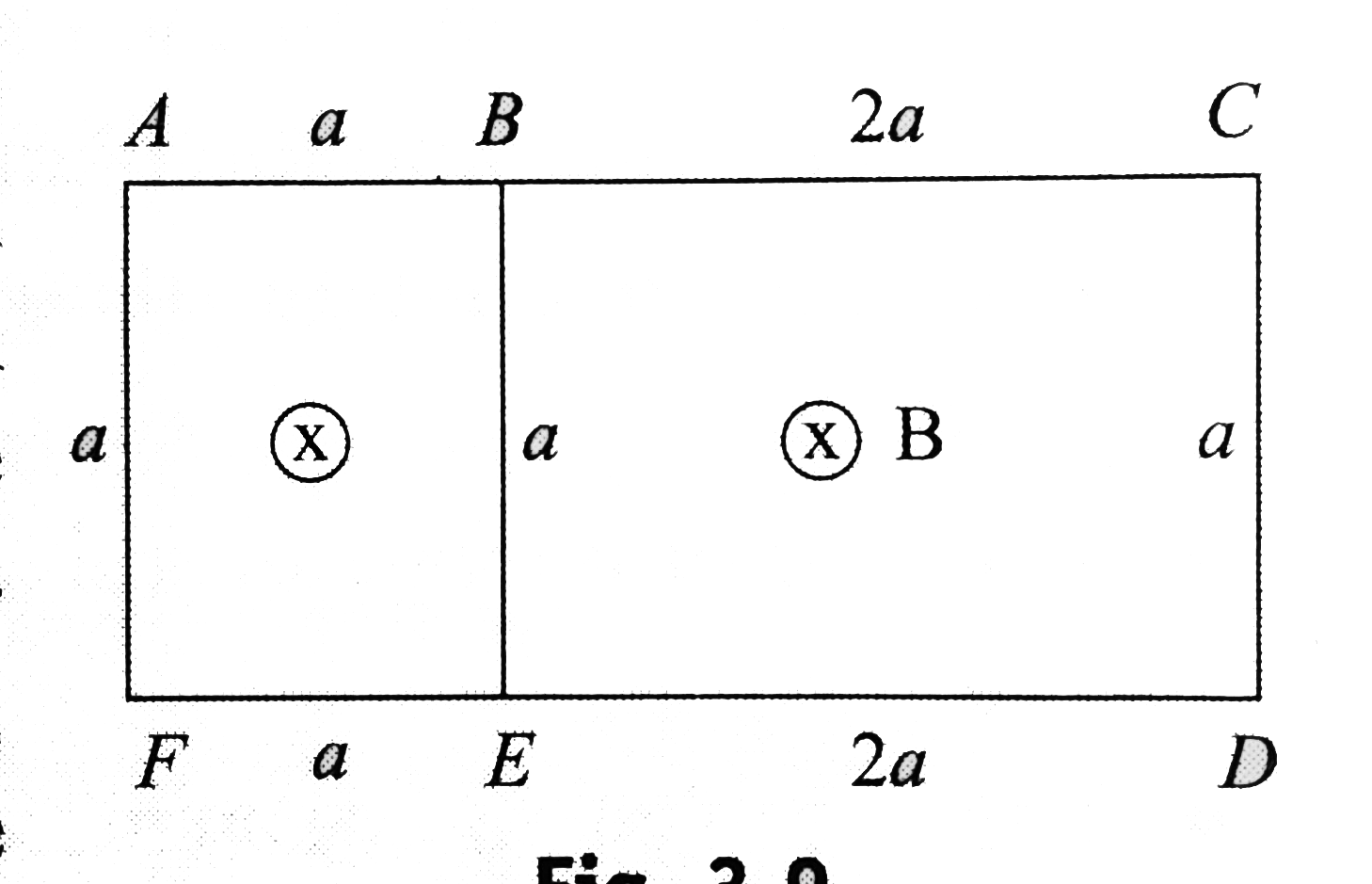 View Solution
View Solution(a) A closed loop is held stationary in the magnetic field between the north and south poles of two permanent magnets held fixed. Can we hope to generate current in the loop by using very strong magnets?
(b) A closed loop moves normal to the constant electric field between the plates of a large capacitor. Is a current induced in the loop (i) when it is wholly inside the region between the capacitor plates (ii) when it is partially outside the plates of the capacitor? The electric field is normal to the plane of the loop.
(c) A rectangular loop and a circular loop are moving out of a uniform magnetic field region (Fig. 6.8) to a field-free region with a constant velocity v. In which loop do you expect the induced emf to be constant during the passage out of the field region? The field is normal to the loops.

(d) Predict the polarity of the capacitor in the situation described by Fig. 6.9.
 View Solution
View SolutionA proton is moving along the negative direction of X-axis in a magnetic field directed along the positive direction of Y-axis. The proton will be deflected along the negative direction of
View SolutionA triangular loop as shown in the figure is started to being pulled out at t=0 form a uniform magnetic field with a constant velocity v. Total resistancee of the loop is constant and equal to R. Then the variation of power produced in the loop with time will be :
 View Solution
View SolutionA neutral metal bare moves at a constant velocity v to the right through a region of uniform magnetic field directed out the page, as shown. Therefore,
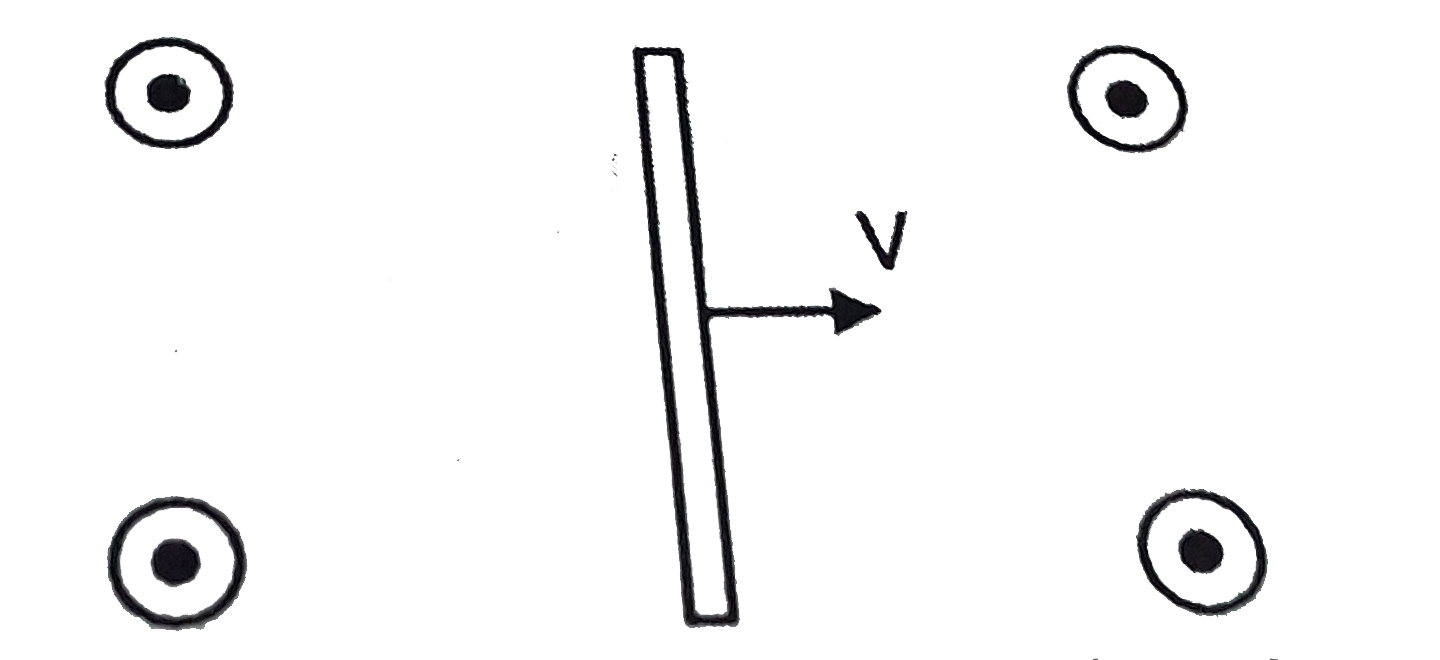 View Solution
View SolutionA uniform electric field and a uniform magnetic field are acting along the same direction in a certain region. If an electron is projected along the direction of the fields with a certain velocity then
View SolutionA uniform electric field and a uniform magnetic field are acting along the same direction in a certain region. If an electron is projected along the direction of the fields with a certain velocity then
View SolutionA uniform electric field and a uniform magnetic field are acting along the same direction in a certain region. If an electron is projected along the direction of the fields with a certain velocity then
View Solution

