A short-circuited coil is placed in a time-varying magnetic field. Electrical power is dissipated due to the current induced in the coil. If the number of turns were to be quadrupled and the wire radius halved, the electrical power dissipated would be
halved
the same
doubled
quadrupled
The correct Answer is:B
To solve the problem, we need to analyze how the electrical power dissipated in a short-circuited coil changes when the number of turns is quadrupled and the wire radius is halved.
1. Understanding Power Dissipation:
The electrical power P dissipated in a coil due to the induced current can be expressed as:
P=E2R
where E is the induced electromotive force (emf) and R is the resistance of the coil.
2. Finding the Induced EMF:
According to Faraday's law of electromagnetic induction, the induced emf E in the coil is given by:
E=−dΦdt
where Φ is the magnetic flux. For a coil with n turns and area A, the induced emf can be expressed as:
E∝n⋅A⋅dBdt
3. Calculating the Area:
The area A of the coil is given by:
A=πr2
where r is the radius of the coil.
4. New Configuration:
- The number of turns is quadrupled: n′=4n
- The radius is halved: r′=r2
5. Calculating New Area:
The new area A′ becomes:
A′=π(r′)2=π(r2)2=πr24=A4
6. Finding New Induced EMF:
The new induced emf E′ can be expressed as:
E′∝n′⋅A′⋅dBdt=(4n)⋅(A4)⋅dBdt=n⋅A⋅dBdt=E
Thus, E′=E.
7. Calculating Resistance:
The resistance R of the coil is given by:
R=ρLAw
where L is the length of the wire and Aw is the cross-sectional area of the wire. The cross-sectional area of the wire when the radius is halved is:
A′w=π(rw2)2=Aw4
The length of the wire increases with the number of turns, so:
L′=4L
Therefore, the new resistance becomes:
R′=ρ4LAw4=16⋅ρLAw=16R
8. Calculating New Power:
The new power P′ is given by:
P′=E′2R′=E216R
9. Finding the Ratio of Powers:
The ratio of the new power to the original power is:
P′P=E216RE2R=116
Conclusion:
The electrical power dissipated in the coil when the number of turns is quadrupled and the wire radius is halved will be 116 of the original power.
The electrical power P dissipated in a coil due to the induced current can be expressed as:
P=E2R
where E is the induced electromotive force (emf) and R is the resistance of the coil.
According to Faraday's law of electromagnetic induction, the induced emf E in the coil is given by:
E=−dΦdt
where Φ is the magnetic flux. For a coil with n turns and area A, the induced emf can be expressed as:
E∝n⋅A⋅dBdt
The area A of the coil is given by:
A=πr2
where r is the radius of the coil.
- The number of turns is quadrupled: n′=4n
- The radius is halved: r′=r2
The new area A′ becomes:
A′=π(r′)2=π(r2)2=πr24=A4
The new induced emf E′ can be expressed as:
E′∝n′⋅A′⋅dBdt=(4n)⋅(A4)⋅dBdt=n⋅A⋅dBdt=E
Thus, E′=E.
The resistance R of the coil is given by:
R=ρLAw
where L is the length of the wire and Aw is the cross-sectional area of the wire. The cross-sectional area of the wire when the radius is halved is:
A′w=π(rw2)2=Aw4
The length of the wire increases with the number of turns, so:
L′=4L
Therefore, the new resistance becomes:
R′=ρ4LAw4=16⋅ρLAw=16R
The new power P′ is given by:
P′=E′2R′=E216R
The ratio of the new power to the original power is:
P′P=E216RE2R=116
The electrical power dissipated in the coil when the number of turns is quadrupled and the wire radius is halved will be 116 of the original power.
Topper's Solved these Questions
- BOOK - DC PANDEY ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Medical entrance special format questions17videos
ELECTROMAGNETIC INDUCTION
- BOOK - DC PANDEY ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Match the columns5videos
ELECTROMAGNETIC INDUCTION
- BOOK - DC PANDEY ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Check point60videos
ELECTROMAGNETIC INDUCTION
- BOOK - DC PANDEY ENGLISHCHAPTER - CURRENT ELECTRICITYEXERCISE - Medical entrances gallery97videos
CURRENT ELECTRICITY
- BOOK - DC PANDEY ENGLISHCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - Sec C22videos
ELECTROMAGNETIC WAVES
Similar Questions
A close loop is placed in a time-varying magnetic field. Electrical power is dissipated due to the current induced in the coil. If the number of turns were to be quadrupled and the wire radius halved keeping the radius of the loop unchanged, the electrical power dissipated would be :
View SolutionA coil carrying electric current is placed in uniform magnetic field
View Solution
Knowledge Check
- Question 1 - Select One
A circular coil of 25 turns and radius 12 cm is placed in a uniform magnetic field of 0.5 T normal to the plane of the coil. If the current in the coil is 6 A then total torque acting on the coil is
AzeroB3.4 NmC3.8 NmD4.4 Nm - Question 2 - Select One
A circular coil of 25 turns and radius of 12 cm is placed in a uniform magnetic field of 0.5 T normal to the plane of coil. If the current in the coil is 5 A, then total torque experienced by the coil is
A1.5NmB2.5NmC3.5NmDzero - Question 3 - Select One
A ciruclar coil of 20 turns and radius 10cm is placed in a uniform magnetic field of 0.1T normal to the plane of the coil . If the current in the coil is 5.0A what is the average force on each electron in the coil due to the magnetic field ( The coil is made of copper wire of cross− sectional area 10−5m2 and the free electron density in copper is given to be about 1029m−3).
A2.5×10−25NB5×10−25NC4×10−25ND3×10−25N
A circular loop of radius 1m is placed in a varying magnetic field given as B=6t Tesla, where t is time in sec.
(a)Find the emf induced in the coil if the plane of the coil is perpendicular to the magnetic field.
(b) Find the electric field in the tangential directin, induced due to the changing magnetic field.
(c)Find the current in the loop if its resistance is 1Ω/m.View SolutionFigure shows a wire frame PQSTXYZ placed in a time varying magnetic field given as B=βt, where β is a positive constant.Resistance per unit length of the wire is λ.Find the current induced in the wire and draw its electrical equivalent diagram.
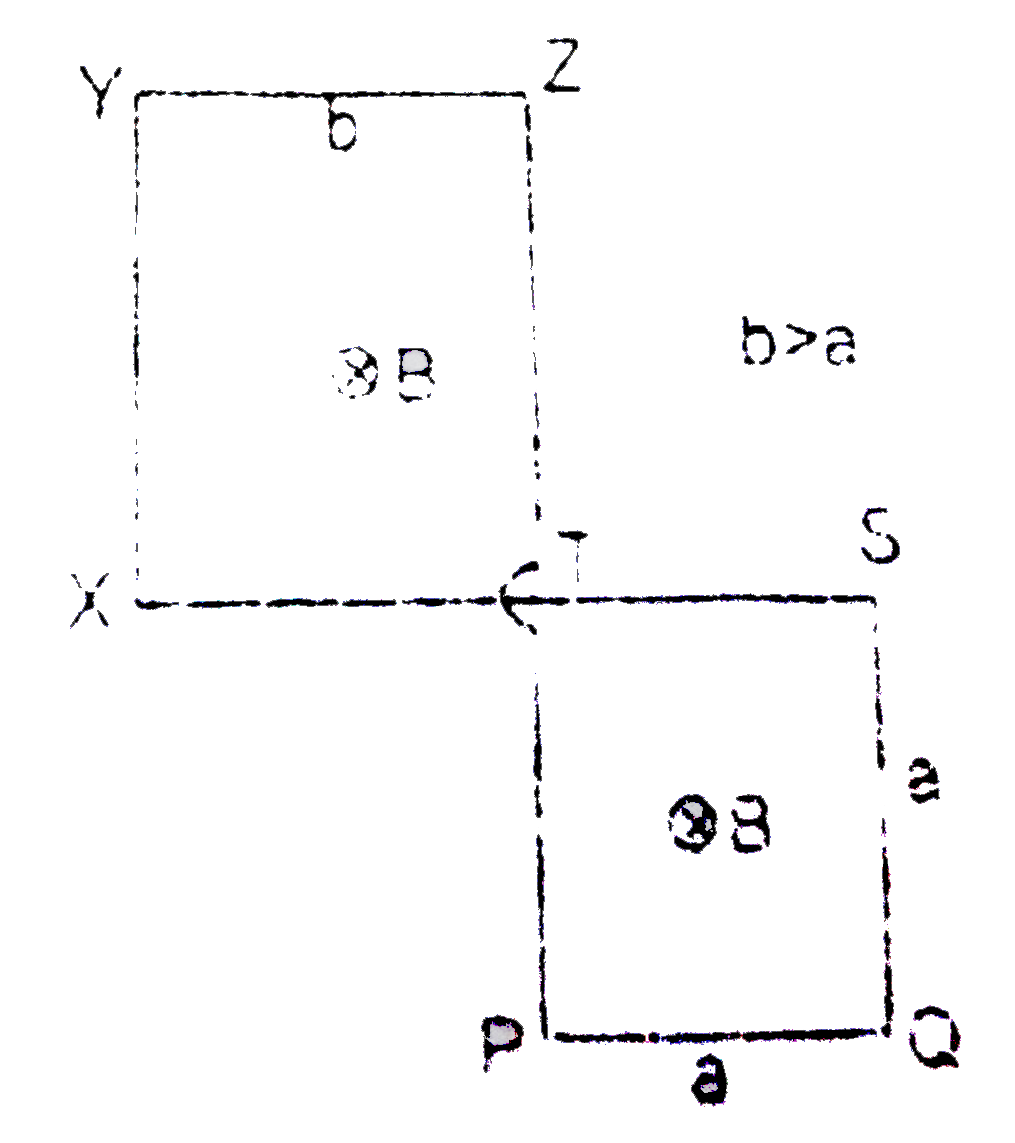 View Solution
View SolutionHow will the magnetic field induction at the centre of a circular coil carrying current change, if the current through the coil is doubled and the radius of the coil is halved?
View SolutionA circular coil of n turns and radius r carries a current I. The magnetic field at the centre is
View SolutionWhen equal current is passed through two coils equal magnetic field is produced at their centers. If the ratio of number of turns in the coils is 8:15 then the ratio of the their radii will be
View Solution

