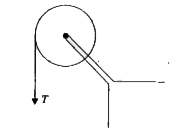
A uniform disc of mass M and radius R is mounted on an axle supported in frictionless bearings. A light cord is wrapped around the rim of the disc and a steady downward pull T is exerted on the cord. The angular acceleration of the disc is
TMR
MRT
2TMR
MR2T
The correct Answer is:C
To find the angular acceleration of the uniform disc when a steady downward pull T is exerted on a cord wrapped around its rim, we can follow these steps:
1. Identify the Forces Acting on the Disc:
- The weight of the disc, Mg, acts downwards at the center of the disc.
- The tension T in the cord acts tangentially at the rim of the disc.
2. Calculate the Torque:
- The torque τ caused by the tension T about the axis of the disc is given by:
τ=T⋅R
where R is the radius of the disc.
3. Moment of Inertia of the Disc:
- The moment of inertia I of a uniform disc about its central axis is:
I=12MR2
4. Apply the Relation Between Torque and Angular Acceleration:
- According to Newton's second law for rotation, the torque is also related to the moment of inertia and angular acceleration α:
τ=I⋅α
- Substituting the expressions for torque and moment of inertia, we have:
T⋅R=(12MR2)⋅α
5. Solve for Angular Acceleration α:
- Rearranging the equation gives:
α=T⋅R12MR2
- Simplifying this expression:
α=2TMR
Final Answer:
The angular acceleration α of the disc is:
α=2TMR
- The weight of the disc, Mg, acts downwards at the center of the disc.
- The tension T in the cord acts tangentially at the rim of the disc.
- The torque τ caused by the tension T about the axis of the disc is given by:
τ=T⋅R
where R is the radius of the disc.
- The moment of inertia I of a uniform disc about its central axis is:
I=12MR2
- According to Newton's second law for rotation, the torque is also related to the moment of inertia and angular acceleration α:
τ=I⋅α
- Substituting the expressions for torque and moment of inertia, we have:
T⋅R=(12MR2)⋅α
- Rearranging the equation gives:
α=T⋅R12MR2
- Simplifying this expression:
α=2TMR
The angular acceleration α of the disc is:
α=2TMR
Topper's Solved these Questions
- RIGID BODY DYNAMICS 1BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - RIGID BODY DYNAMICS 1EXERCISE - Multiple Correct
9 Videos
- RIGID BODY DYNAMICS 1BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - RIGID BODY DYNAMICS 1EXERCISE - Linked Comprehension
28 Videos
- RIGID BODY DYNAMICS 1BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - RIGID BODY DYNAMICS 1EXERCISE - Subjective
13 Videos
- PROPERTIES OF SOLIDS AND FLUIDSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - PROPERTIES OF SOLIDS AND FLUIDSEXERCISE - INTEGER_TYPE
2 Videos
- RIGID BODY DYNAMICS 2BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - RIGID BODY DYNAMICS 2EXERCISE - Interger
2 Videos
Similar Questions
Fig. shows a uniform disc of mass 1 kg and radius 0.2 m mounted on an axle supported in fixed frictionless bearings. A light string is wrapped around the rim of the wheel. A constant downwards pull of 2 kg wt. is exerted on the string. What is the angular acceleration of the wheel and the tangential acceleration of a point on the rim ?
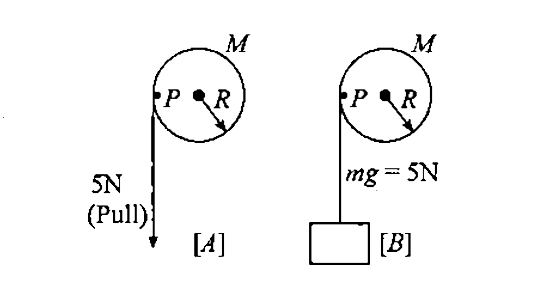
View SolutionA uniform disc of mass M =2.50 kg and radius R=0.20 m is mounted on an axle supported on fixed frictionless bearings. A light cord wrapped around the rim is pulled with a force 5 N. On the same system of pulley and string, instead of pulling it down, a body of weight 5 N is suspended. If the first processis termed A and the second B, the tangential acceleration of point P will be:
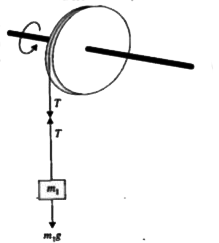
View SolutionA uniform disc of mass M and radius R is mounted on a fixed horizontal axis. A block of mass m hangs from a massless string that is wrapped around the rim of the disc. The magnitude of the acceleration of the falling block (m) is
View SolutionThe uniform speed of a body is the same as seen from any point in the body. A light cord is wrapped around the rim of the disc and mass of 1kg is tied to the free end. If it is released from rest,
View SolutionA wheel of mass 1.4 kg and radius 0.4 m is mounted on a frictionless, horizontal axle as shown in Fig. 7.2.50. Alight string wrapped around the rim supports a mass of 2 kg. What is the angular acceleration of the wheel and the tangential acceleration of a point on the rim ? Also find the tension in the string.
View SolutionA uniform disc of mass M and radius R is hinged at its centre C . A force F is applied on the disc as shown . At this instant , angular acceleration of the disc is

View Solution
A uniform disc of mass M and radius R is pivoted about the horizontal axis through its centre C A point mass m is glued to the disc at its rim, as shown in figure. If the system is released from rest, find the angular velocity of the disc when m reaches the bottom point B.
View SolutionA solid cylinder of mass M and of radius R is fixed on a frictionless axle over a well. A rope negligible mass is wrapped around the cylinder. A bucket of uniform mass m is suspended from it. The linear acceleration bucket will:
View SolutionA disc of a mass M and radius R can rotate freely in vertical plane about a horizontal axis at O. Distant r from the center of disc as shown in the figure. The disc is released from rest in the shown position.

The angular acceleration of disc when OC rotates by an angles of 37∘ is
View SolutionA uniform disc of mass M, radius R is attached to a frictionless horizontal axis. Its rim is wound with a light string and a tension T is applied to it, the angular acceleration will be?
View Solution
