A uniform rod is kept vertically on a horizontally smooth surface at a point O. IF it is rotated slightly and released, it falls down on the horizontal surface. The lower end will remain
at O
at a distance less than l2 from O
at a distance l2 from O
at a distance larger than l2 from O
The correct Answer is:C
To solve the problem, we need to analyze the motion of the uniform rod as it falls after being slightly rotated from the vertical position.
1. Understanding the Setup:
- A uniform rod of length L is placed vertically on a smooth horizontal surface at point O. The center of mass of the rod is located at a distance L2 from point O.
2. Initial Position of the Center of Mass:
- When the rod is vertical, the center of mass is at L2 above point O.
3. Rotation and Release:
- When the rod is slightly rotated and released, it will begin to fall due to gravity. The lower end of the rod (point O) will remain in contact with the ground.
4. Analyzing the Motion:
- As the rod falls, the center of mass will follow a path that is influenced by the pivot point at O. Since there are no horizontal forces acting on the rod (the surface is smooth), the horizontal position of the center of mass will not change.
5. Final Position of the Center of Mass:
- After the rod has fallen and comes to rest on the horizontal surface, the center of mass will still be located at a distance L2 from point O because the horizontal position of the center of mass does not change during the fall.
6. Conclusion:
- Therefore, the lower end of the rod will remain at point O, and the center of mass will be at a distance L2 from point O when the rod is lying flat on the surface.
Final Answer:
The lower end of the rod will remain at a distance L2 from point O.
---
- A uniform rod of length L is placed vertically on a smooth horizontal surface at point O. The center of mass of the rod is located at a distance L2 from point O.
- When the rod is vertical, the center of mass is at L2 above point O.
- When the rod is slightly rotated and released, it will begin to fall due to gravity. The lower end of the rod (point O) will remain in contact with the ground.
- As the rod falls, the center of mass will follow a path that is influenced by the pivot point at O. Since there are no horizontal forces acting on the rod (the surface is smooth), the horizontal position of the center of mass will not change.
- After the rod has fallen and comes to rest on the horizontal surface, the center of mass will still be located at a distance L2 from point O because the horizontal position of the center of mass does not change during the fall.
- Therefore, the lower end of the rod will remain at point O, and the center of mass will be at a distance L2 from point O when the rod is lying flat on the surface.
The lower end of the rod will remain at a distance L2 from point O.
Topper's Solved these Questions
- BOOK - HC VERMA ENGLISHCHAPTER - ROTATIONAL MECHANICSEXERCISE - Objective -214videos
ROTATIONAL MECHANICS
- BOOK - HC VERMA ENGLISHCHAPTER - ROTATIONAL MECHANICSEXERCISE - Exercises86videos
ROTATIONAL MECHANICS
- BOOK - HC VERMA ENGLISHCHAPTER - ROTATIONAL MECHANICSEXERCISE - worked out Examples29videos
ROTATIONAL MECHANICS
- BOOK - HC VERMA ENGLISHCHAPTER - REST AND MOTION : KINEMATICSEXERCISE - Question for short Answer13videos
REST AND MOTION : KINEMATICS
- BOOK - HC VERMA ENGLISHCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Question for short Answer15videos
SIMPLE HARMONIC MOTION
Similar Questions
A uniform rod of length l is kept vertically on a rough horizontal surface at x = 0 . It is rotated slightly and released . When the rod finally falls on the horizontal surface , the lower end will remain at
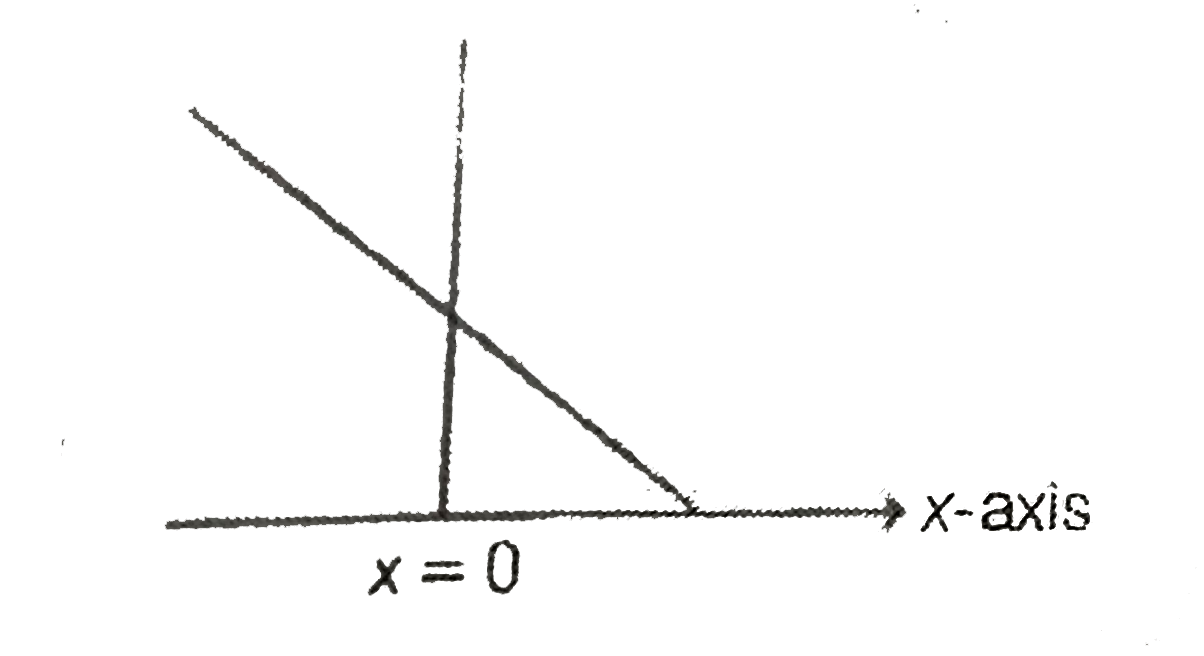 View Solution
View SolutionA particle, moving horizontally, collides perpendicularly at one end of a rod having equal mass and placed on a smooth horizontal surface.
 View Solution
View SolutionA solid sphere rolling on a rough horizontal surface. Acceleration of contact point is zero.
A solid sphere can roll on the smooth surface.View SolutionA rod of mass m and length L is kept on a horizontal smooth surface. The rod is hinged at its one end A. There are two springs, perpendicular to the rod, kept in the same horizontal plane. The spring of spring constant k is rigidly attached to the lower end of rod and spring of spring constant 12k just touches the rod at its midpoint C, as shown in the figure. If the rod is slightly displaced leftward and released then, the time period for small osciallation of the rod will be
 View Solution
View SolutionA uniform sphere is place on a smooth horizontal surface and as horizontal force F is appied on it at a distance h above the surface. The acceleratioin of the centre
View SolutionA uniform rod of length L and mass M is pulled horizontally on a smooth surface with a force F. Determine the elongation of rod if Young's modulus of the material is Y.
View SolutionA ball of mass moving with constant velocity u collides with a smooth horizontal surface at O as shown in Fig. Neglect gravity and friction. The y-axis is drawn normal to the horizontal surface at the point of impact O and x-axis is horizontal as shown. About which point will the angular momentum of ball be conserved?
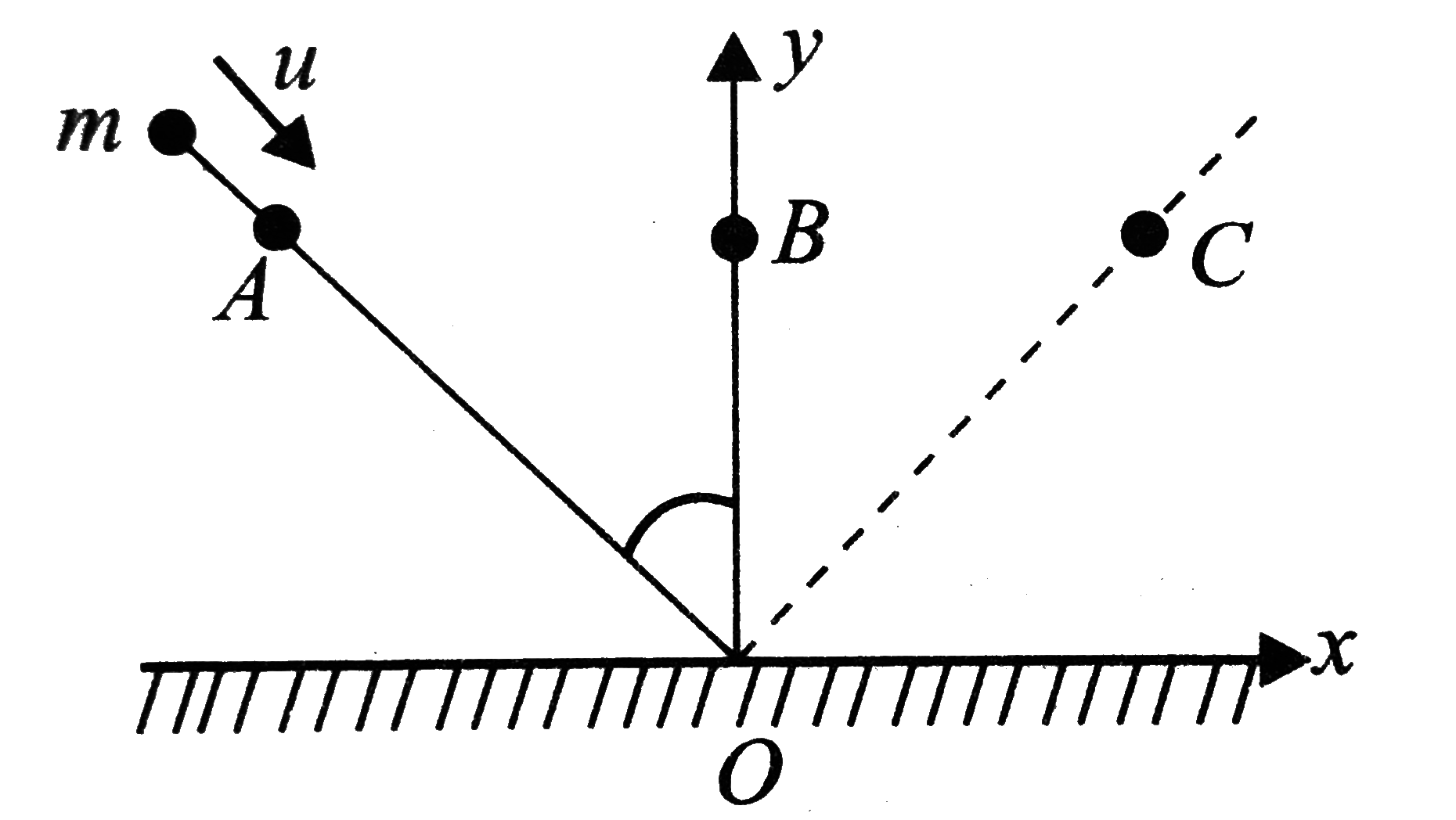 View Solution
View SolutionA uniform rod of mass M and length L is standing vertically along the y-axis on a smooth horizontal surface as shown. A small disturbance causes the lower end to slip on the smooth horizontal surface and rod starts falling. The path followed by centre of mass of the rod during the fall is
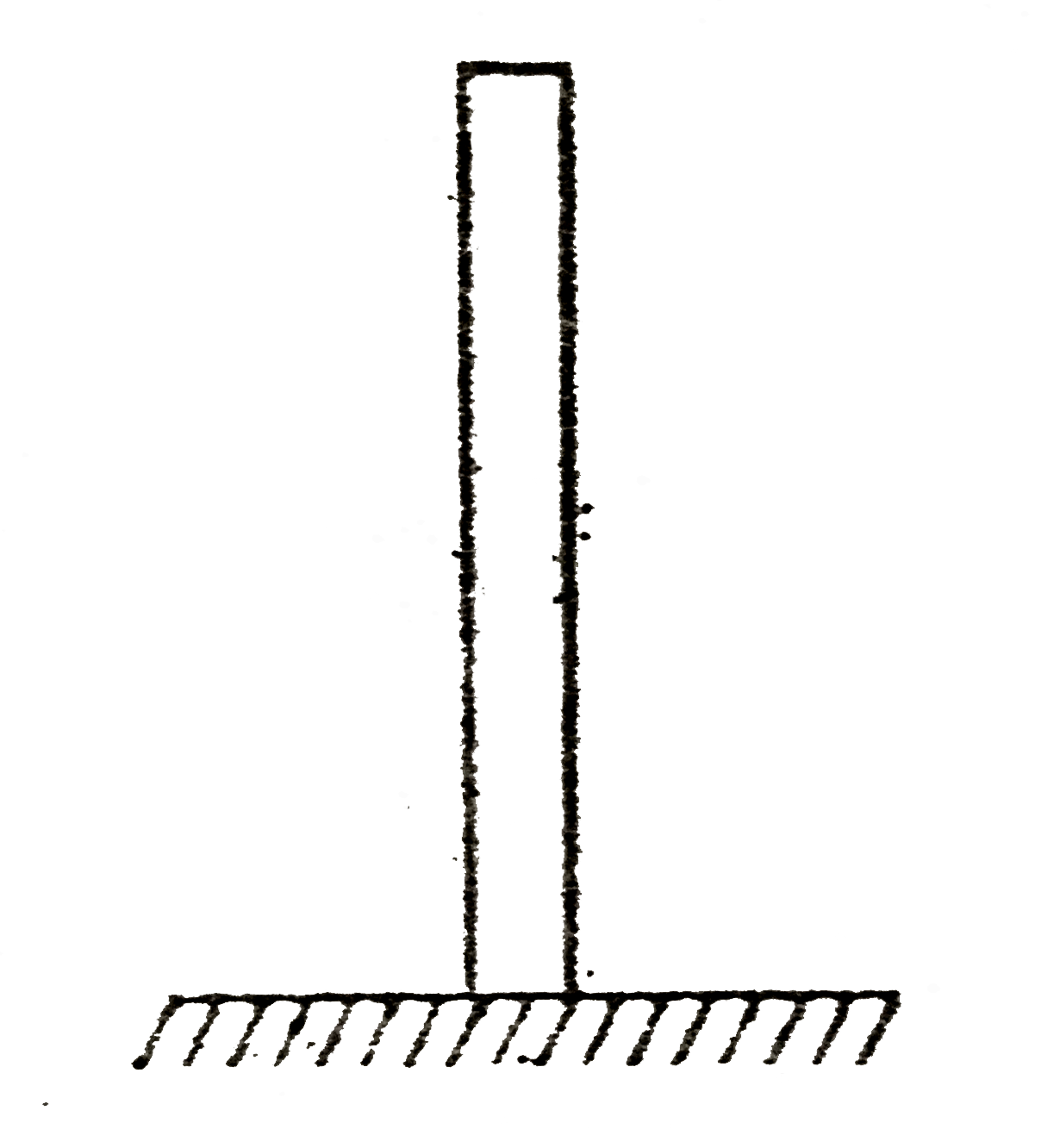 View Solution
View SolutionA uniform straight rod is placed in vertical position on a smooth horizontal surface and released. As the rod is in motion, the centre of mass moves
View SolutionA uniform rod of mas m and length l is kept vertical with the lower end clamed. It is slightly pushed to let it fall down under gravity. Find its angular speed when the rod is passing through its lowest positon. Neglect any friction at the clamp. What will be the linear speed of the free end at this instant?
View Solution

