Question

A uniform solid cylinder of density $0.8g/c{m}^3$ floats in equilibrium in a combination of two non-mixing liquids A and B with its axis vertical.
The densities of the liquids A and B are $0.7g/c{m}^3$ and $1.2g/c{m}^3$, respectively. The height of liquid A is $h_A=1.2cm.$ The length of the part of the cylinder immersed in liquid B is $h_B=0.8cm$.

(a) Find the total force exerted by liquid A on the cylinder.
(b) Find h, the length of the part of the cylinder in air.
(c) The cylinder is depressed in such a way that its top surface is just below the upper surface of liquid A and is then released. Find the acceleration of the cylinder immediately after it is released.

Explanation

(a) Liquid A is applying the hydrostatic force on cylinder uniformly from all the sides. So, net force is zero.

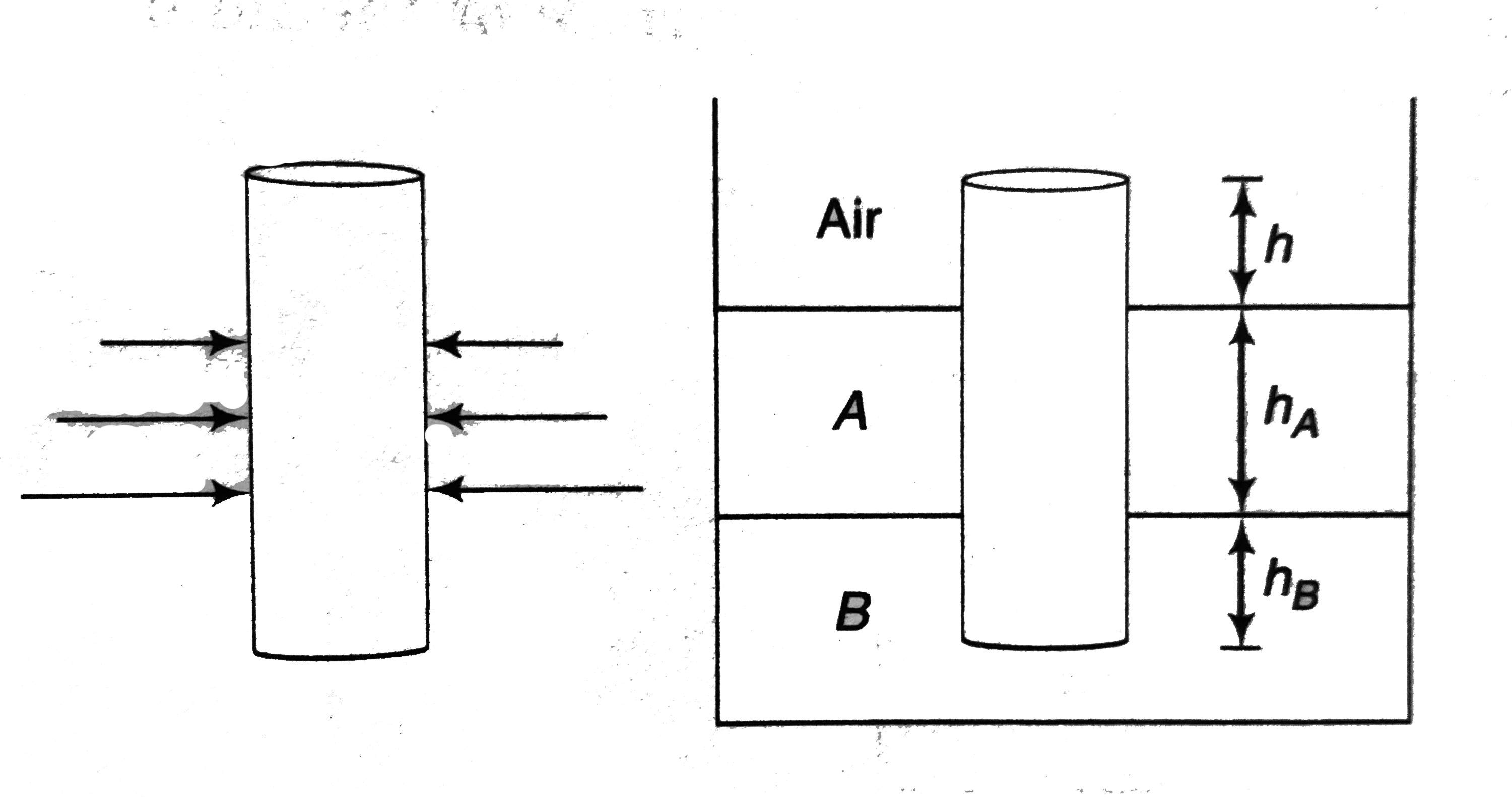
(b) In equilibrium
Weight of cylinder =Net upthrust on the cylinder
Weight `=(s)(h+ h_(A)+ h_(B)) rho_("cylender")g`
and upthrust on the cylinder
=upthurst due to liquid `A +` upthurst due to liquid `B`
`=s(h_(A) rho_(A)g+sh_(B) rho_(B)g`
Equating these two,
`s(h+h_(A)+ h_(B)) rho_("cylender")g=sg (h_(A) rho_(A)+h_(B) rho_(B))`
or `(h+h_(A)+h_(B)) rho_("cylender")g=h_(A) rho_(A)+ h_(B) rho_(B)`
Substituting, `h_(A)=1.2 cm ,h_(B)=0.8 cm` and `rho_(A)=0.7g//cm^(3)`
`rho_(B)=1.2 g//cm^(3)` and `rho_("cylinder")=0.8g//cm^(3)`
In the above equation, we get `h=0.25 cm`.
(c ) Net upward force=extra upthrust `=shrho_(B)g`
`:.` Net acceleration `a=(Force)/(mass of cylinder)`
or,` a=(shrho_(B)g)/(s(h+h_(A)+h_(B))rho_("cylender"))`
Substituting the value of` h,h_(A),h_(B),rho_(B)`and `rho_("cylinder")`.
we get `a=(g)/(6)`.