At which distance along the centre axis of a uniformaly charged plastic disc of radius R is the magnitude of the electric field equal to one-half the magnitude of the field at the centre of the surface of the disc ?
R√2
R√3
√2R
√3R
The correct Answer is:B
To solve the problem, we need to find the distance x along the center axis of a uniformly charged plastic disc of radius R where the electric field is equal to one-half the magnitude of the electric field at the center of the disc.
1. Understanding the Electric Field at the Center of the Disc:
The electric field EC at the center of a uniformly charged disc is given by the formula:
EC=2kπσ
where k is Coulomb's constant and σ is the surface charge density.
2. Electric Field at a Distance x Along the Axis:
The electric field Ex at a distance x along the axis of the disc is given by:
Ex=2kπσ(1−x√x2+R2)
3. Setting Up the Equation:
According to the problem, we need to find the distance x such that:
Ex=12EC
Substituting the expression for EC:
Ex=12(2kπσ)=kπσ
4. Equating the Two Expressions:
Now we set Ex equal to kπσ:
2kπσ(1−x√x2+R2)=kπσ
5. Simplifying the Equation:
Dividing both sides by kπσ (assuming σ≠0):
2(1−x√x2+R2)=1
Simplifying further:
2−2x√x2+R2=1
2x√x2+R2=1
x√x2+R2=12
6. Squaring Both Sides:
Squaring both sides to eliminate the square root:
x2=14(x2+R2)
7. Rearranging the Equation:
Rearranging gives:
4x2=x2+R2
3x2=R2
8. Solving for x:
Finally, we solve for x:
x2=R23
x=R√3
Final Answer:
The distance x along the center axis of the uniformly charged plastic disc where the electric field is equal to one-half the magnitude of the field at the center of the disc is:
x=R√3
The electric field EC at the center of a uniformly charged disc is given by the formula:
EC=2kπσ
where k is Coulomb's constant and σ is the surface charge density.
The electric field Ex at a distance x along the axis of the disc is given by:
Ex=2kπσ(1−x√x2+R2)
According to the problem, we need to find the distance x such that:
Ex=12EC
Substituting the expression for EC:
Ex=12(2kπσ)=kπσ
Now we set Ex equal to kπσ:
2kπσ(1−x√x2+R2)=kπσ
Dividing both sides by kπσ (assuming σ≠0):
2(1−x√x2+R2)=1
Simplifying further:
2−2x√x2+R2=1
2x√x2+R2=1
x√x2+R2=12
Squaring both sides to eliminate the square root:
x2=14(x2+R2)
Rearranging gives:
4x2=x2+R2
3x2=R2
Finally, we solve for x:
x2=R23
x=R√3
The distance x along the center axis of the uniformly charged plastic disc where the electric field is equal to one-half the magnitude of the field at the center of the disc is:
x=R√3
Topper's Solved these Questions
- ELECTROSTATICSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - Assertion and Reason
15 Videos
- ELECTROSTATICSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - Match the columns
5 Videos
- ELECTROSTATICSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - Check point 1.5
20 Videos
- ELECTROSTATIC POTENTIAL AND CAPACITORSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITORSEXERCISE - (C) Chapter exercises
50 Videos
- GRAVITATIONBOOK - DC PANDEY ENGLISHCHAPTER - GRAVITATIONEXERCISE - All Questions
135 Videos
Similar Questions
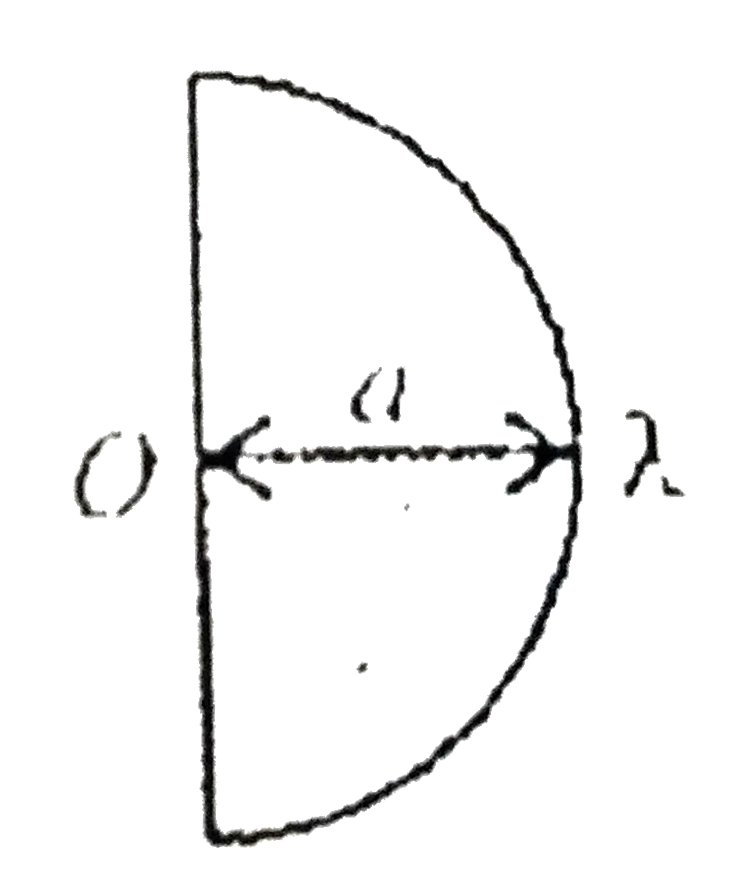
Electric field at centre of a uniforly charged semicirlce of radius a is
View SolutionIf the potential at the centre of a uniformly charged hollow sphere of radius R is V, then electric field at a distance r from the centre of sphere will be (r>R).

View SolutionThe surface charge density of a thin charged disc of radius R is σ. The value of the electric field at the centre of the disc σ2∈0. With respect to the field at the centre, the electric field along the axis at a distance R from the centre of the disc is
View SolutionA charge q is uniformly distributed over a quarter circular ring of radius r. The magnitude of electric field strength at the centre of the ring will be
View SolutionFor a uniformly charged ring of radius R, the electric field on its exis has the largest magnitude at a distance h from its centre. Then value of h is :
View SolutionA sphere of radius R carries charge such that its volume charge density is proportional to the square of the distance from the centre. What is the ratio of the magnitude of the electric field at a distance 2 R from the centre to the magnitude of the electric field at a distance of R/2 from the centre?
View SolutionThe magnitude of the electric field on the surface of a sphere of radius r having a uniform surface charge density σ is
View Solution3 charges are placed in a circle of radius d as shown in figure. Find the electric field along x-axis at centre of circle.
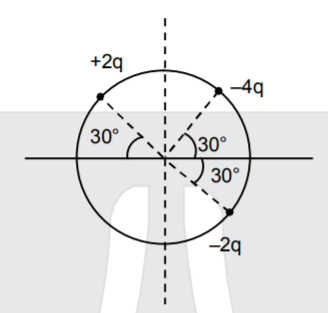
View Solution3 charges are placed in a circle of radius d as shown in figure. Find the electric field along x-axis at centre of circle.

View SolutionThe electric field intensity at a distance 20cm from the centre of a uniformly charged non conducting solid sphere of radius 10cm is E.Then what is the electric field intensity at a distance 5cm from the centre it will be.....
View Solution
