Bob of the pendulum is at its bottom most point when it is given horizontal velocity √57 m/s . Length of the string is 1.5 m .
(i) Find maximum height attained by the bob above its lowest point .
(b) What will be the answer to part (a) if intial velocity given s √95 m/s ?
To solve the problem step by step, we will analyze the motion of the pendulum bob when given a horizontal velocity and find the maximum height it can reach.
Given Data:
- Length of the pendulum string, L=1.5m
- Initial horizontal velocity, u=√57m/s for part (a)
- Gravitational acceleration, g=10m/s2
Part (a): Finding Maximum Height with u=√57
1. Determine the velocity required for a full circular motion:
The velocity required to complete a full circular motion is given by:
vrequired=√gL=√10×1.5=√15≈3.87m/s
Since √57 is greater than √15, the bob cannot complete a full revolution.
2. Calculate the angle at which the string becomes slack:
The condition for the string to become slack is when the centripetal force is equal to the gravitational component acting along the string:
u2L=gcosθ
Substituting the values:
571.5=10cosθ⟹38=10cosθ⟹cosθ=3810=3.8
Since cosθ cannot exceed 1, we need to find the maximum height using energy conservation.
3. Using conservation of mechanical energy:
The total mechanical energy at the lowest point is equal to the total mechanical energy at the maximum height:
12mu2=mgh+12mv2
Here, h is the height above the lowest point when the string becomes slack, and v is the velocity at that point.
4. Finding the height:
The height can be found using the vertical component of the motion:
h=L(1−cosθ)
We can calculate h using the energy conservation approach:
h=u22g
Substituting u=√57:
h=572×10=5720=2.85m
5. Total height above the lowest point:
The total height attained by the bob is:
H=L+h=1.5+2.85=4.35m
Part (b): Finding Maximum Height with u=√95
1. Repeat the process for u=√95:
- Calculate the velocity required for a full circular motion:
vrequired=√gL=√10×1.5=√15≈3.87m/s
Since √95 is greater than √15, the bob cannot complete a full revolution.
2. Calculate the angle at which the string becomes slack:
Using the same centripetal force condition:
u2L=gcosθ⟹951.5=10cosθ⟹63.33=10cosθ⟹cosθ=6.33
Again, since cosθ cannot exceed 1, we will use energy conservation.
3. Using conservation of mechanical energy:
12mu2=mgh+12mv2
Finding the height:
h=u22g
Substituting u=√95:
h=952×10=9520=4.75m
4. Total height above the lowest point:
The total height attained by the bob is:
H=L+h=1.5+4.75=6.25m
Final Answers:
- (a) Maximum height with u=√57: 4.35m
- (b) Maximum height with u=√95: 6.25m
- Length of the pendulum string, L=1.5m
- Initial horizontal velocity, u=√57m/s for part (a)
- Gravitational acceleration, g=10m/s2
The velocity required to complete a full circular motion is given by:
vrequired=√gL=√10×1.5=√15≈3.87m/s
Since √57 is greater than √15, the bob cannot complete a full revolution.
The condition for the string to become slack is when the centripetal force is equal to the gravitational component acting along the string:
u2L=gcosθ
Substituting the values:
571.5=10cosθ⟹38=10cosθ⟹cosθ=3810=3.8
Since cosθ cannot exceed 1, we need to find the maximum height using energy conservation.
The total mechanical energy at the lowest point is equal to the total mechanical energy at the maximum height:
12mu2=mgh+12mv2
Here, h is the height above the lowest point when the string becomes slack, and v is the velocity at that point.
The height can be found using the vertical component of the motion:
h=L(1−cosθ)
We can calculate h using the energy conservation approach:
h=u22g
Substituting u=√57:
h=572×10=5720=2.85m
The total height attained by the bob is:
H=L+h=1.5+2.85=4.35m
- Calculate the velocity required for a full circular motion:
vrequired=√gL=√10×1.5=√15≈3.87m/s
Since √95 is greater than √15, the bob cannot complete a full revolution.
Using the same centripetal force condition:
u2L=gcosθ⟹951.5=10cosθ⟹63.33=10cosθ⟹cosθ=6.33
Again, since cosθ cannot exceed 1, we will use energy conservation.
12mu2=mgh+12mv2
Finding the height:
h=u22g
Substituting u=√95:
h=952×10=9520=4.75m
The total height attained by the bob is:
H=L+h=1.5+4.75=6.25m
- (a) Maximum height with u=√57: 4.35m
- (b) Maximum height with u=√95: 6.25m
Topper's Solved these Questions
- BOOK - MODERN PUBLICATIONCHAPTER - WORK, ENERGY AND POWER EXERCISE - NCERT FILE (NCERT Textbook Exercise)23videos
WORK, ENERGY AND POWER
- BOOK - MODERN PUBLICATIONCHAPTER - WORK, ENERGY AND POWER EXERCISE - NCERT FILE (NCERT Additional Exercise)7videos
WORK, ENERGY AND POWER
- BOOK - MODERN PUBLICATIONCHAPTER - WORK, ENERGY AND POWER EXERCISE - Conceptual Questions20videos
WORK, ENERGY AND POWER
- BOOK - MODERN PUBLICATIONCHAPTER - WAVESEXERCISE - CHAPTER PRACTICE TEST14videos
WAVES
Similar Questions
The bob of pendulum is project with horizontal velocity of √3gf I is the length of string. Find the angular displacement of string before it becomes slack.
 View Solution
View SolutionThe bob of a pendulum at rest is given a sharp hit to impat a horizontal velocity √10gl where l is the length of the pendulum. Find the tension in the string when a. the string is horizontal. B. The bob is at its highest point and c. the string makes an angle of 600 with teh upward vertical.
View Solution
Knowledge Check
- Question 1 - Select One
A particle is thrown vertically upward. Its velocity at half of the height is 10 m/s. Then the maximum height attained by it : -
(g=10m/s2)A8 mB20 mC10 mD16 m - Question 2 - Select One
If a body is thrown up with the velocity of 15 m/s then maximum height attained by the body is (g=10m/s)
A11.25mB16.2mC24.5mD7.62m - Question 3 - Select One
When a particle is thrown vertically upwards, its velocity at one third of its maximum height is 10√2m/s. The maximum height attained by it is
A20√2mB30mC15 mD12.8 m
The bob of a simple pendulum is given a sharp hit impart it a horizontal speed of √3gL. Find an angle made by the string with the upper verticle before it becomes slack. Also, calculate the maximum height attained by the bob above the point of suspension. L is length of the string.
View SolutionBob B of the pendulum AB is given an initial velocity √3Lg in horizontal direction. Final the maximum height of the bob from the starting point,
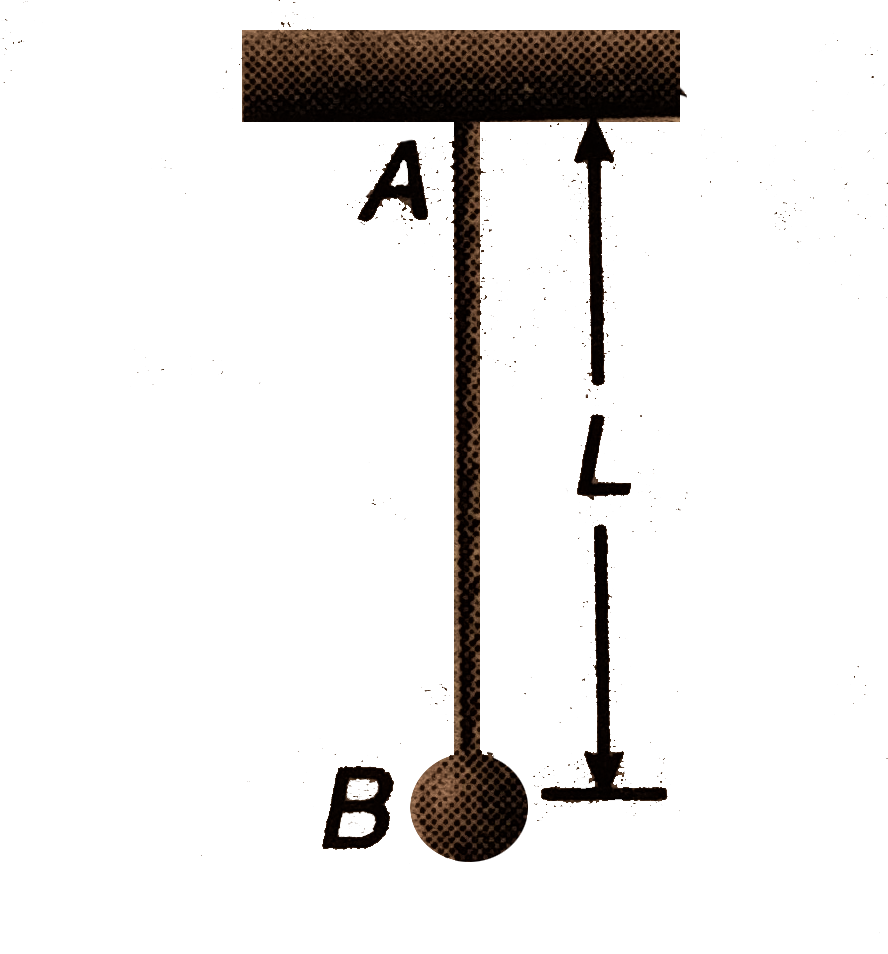
(a) if AB is a massless rod,
(b) if AB is a massless staring.View SolutionA bob is attached to a string of length l whose other end is fixed and is given horizontal velocity at the lowest point of the circle so that the bob moves in a vertical plane. Match the velocity given at the lowest point of circle in column I with tension and velocity at the highest point of the circle corresponding to velocity of column I of column II
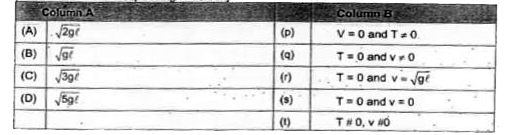 View Solution
View SolutionA stone is projected vertically upwards.When it reaches half the maximum height,its velocity is 20m/s.What is the maximum height attained by the stone? (g=10m/s^(2)
View SolutionA particle is thrown vertically upwards. If its velocity is half of the maximum height is 20m/s, then maximum height attained by it is
View Solution

