Constant temperature of ends are T1 and T2 (where T1>T2) for a conducting rod of 'L' length and cross-sectional area A. What would be the rate of heat transfer in thermal steady state ? Solution in Gujrati
dQdt=KA(T1−T2)L
dQdt=KAL(T1−T2)
dQdt=KA(T1−T2)2
dQdt=KL(T1−T2)A
The correct Answer is:C
Knowledge based question
Topper's Solved these Questions
- BOOK - KUMAR PRAKASHANCHAPTER - THERMAL PROPERTIES OF MATTEREXERCISE - Section - F Question from Module (Sample questions for preparation of competitive exams)18videos
THERMAL PROPERTIES OF MATTER
- BOOK - KUMAR PRAKASHANCHAPTER - THERMAL PROPERTIES OF MATTEREXERCISE - Question Paper (Section - A) Answer following in one sentence : (Word limit of answer is 10) (Each question carries 1 mark)6videos
THERMAL PROPERTIES OF MATTER
- BOOK - KUMAR PRAKASHANCHAPTER - THERMAL PROPERTIES OF MATTEREXERCISE - Section - D NCERT Exemplar Solution (Long Answer Type Questions )8videos
THERMAL PROPERTIES OF MATTER
- BOOK - KUMAR PRAKASHANCHAPTER - SYSTEMS OF PARTICLES AND ROTATIONAL MOTIONEXERCISE - SECTION-F (SECTION-D) QUESTIONS PAPER1videos
SYSTEMS OF PARTICLES AND ROTATIONAL MOTION
- BOOK - KUMAR PRAKASHANCHAPTER - THERMODYANMICS EXERCISE - Question Paper11videos
THERMODYANMICS
Similar Questions
In . . . .. process, gravitation is necessary for transfer of heat energy.
01:10View SolutionGive SI unit of heat current.
03:02View SolutionWe would like to prepare a scale whose length does not change with temperature. It is proposed to prepare a unit scale of this type whose length remains, say 10 cm. We can use a bimetallic strip made of brass and iron each of different length whose length (both components) would change in such a way that difference between their lengths remain constant. If αiron=1.2×10−5/K and αbrass=1.8×10−5/K what should we take as length of each strip ?
08:55View SolutionA rope of negligible mass is wound round a hollow cylinder of mass 3 kg and radius 40 cm. What is the angular acceleration of the cylinder if the rope is pulled with a force of 30 N? What is the linear acceleration of the rope? Assume that there is no slipping.
05:24View SolutionWhy is a steam burn more damaging than a burn with boiling water of the same ?
02:13View SolutionThe two ends of a rod of length L and a uniform cross-sectional area A are kept at two temperature T1 and T2 (T1>T2). The rate of heat transfer. dQdt, through the rod in a steady state is given by
01:38View SolutionA rod of length L with sides fully insulated is made of a material whose thermal conductivity K varies with temperature as K=αT where α is constant. The ends of rod are at temperature T1 and T2(T2>T1) Find the rate of heat flow per unit area of rod .
03:27View SolutionOne end of rod of length L and cross-sectional area A is kept in a furance of temperature T_(1) . The other end of the rod is kept at at temperature T_(2) . The thermal conductivity of the material of the rod is K and emissivity of the rod is e . It is given that T_(2)=T_(S)+DeltaT where DeltaT lt lt T_(S) , T_(S) being the temperature of the surroundings. If DeltaT prop (T_(1)-T_(S)) , find the proportionality constant. Consider that heat is lost only by radiation at the end where the temperature of the rod is T_(2) .
04:22View SolutionThree rods of same cross-section but different length and conductivity are joined in series . If the temperature of the two extreme ends are T1andT2(T1>T2) find the rate of heat transfer H.
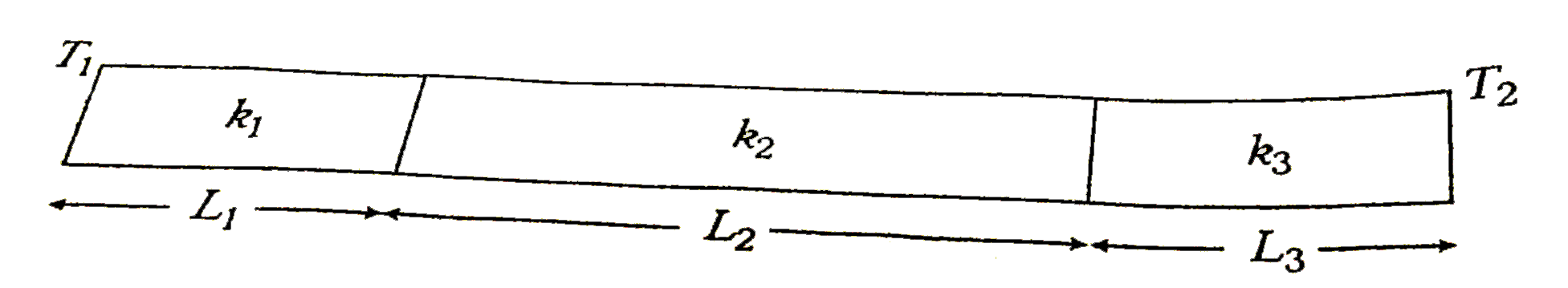 03:12View Solution
03:12View SolutionA rod of length l and cross-section area A has a variable thermal conductivity given by K = alpha T, where alpha is a positive constant and T is temperature in kelvin. Two ends of the rod are maintained at temperature T_(1) and T_(2) (T_(1)gtT_(2)) . Heat current flowing through the rod will be
View SolutionThe two ends of a rod of length L and a uniform cross-sectional area A are kept at two temperatures f_(1) and T_(2) rod in a steady state is given by :
04:03View SolutionLet the length L and the two ends of a rod of constant intersection area A be two temperatures. T_(1) And T_(2) (whereas T_(1) gt T_(2) Is) being kept constant at. The rate of heat transfer from the rods to the stationary state, (dQ)/(dt) Will be
04:09View SolutionAn amount of heat passing through a metallic rod in time t is given by Q=(KA(T_(1)-T_(2))t)/(I) where k= thermal conductivity A= Cross sectional area, T_(1) and T_(2) are temeprature of hot and cold ends respectively and L= length. So the dimesional formula for k=.....
01:55View SolutionA rod of length l and of a uniform cross-sectional area A is made of a material of non-uniform thermal conductivity. Its thermal conductivity is temperature depenndent and varies as K=(B)/(T) , where B is a constant. If ends of the rod are maintained at constant temperatures T_(1) and T_(2) with T_(1)gt T_(2) , rate of flow of heat in steady state will be :
06:39View SolutionEleven rods each of length L , cross section A and coeffeicient of thermal conductivity K are arranged as shown . Find the rate of heat transfere from P to Q(T1>T2) .
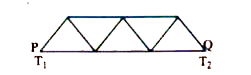 02:29View Solution
02:29View Solution

