Find how much mass m will rise if 4m falls away. Blocks are at rest and in equilibrium.
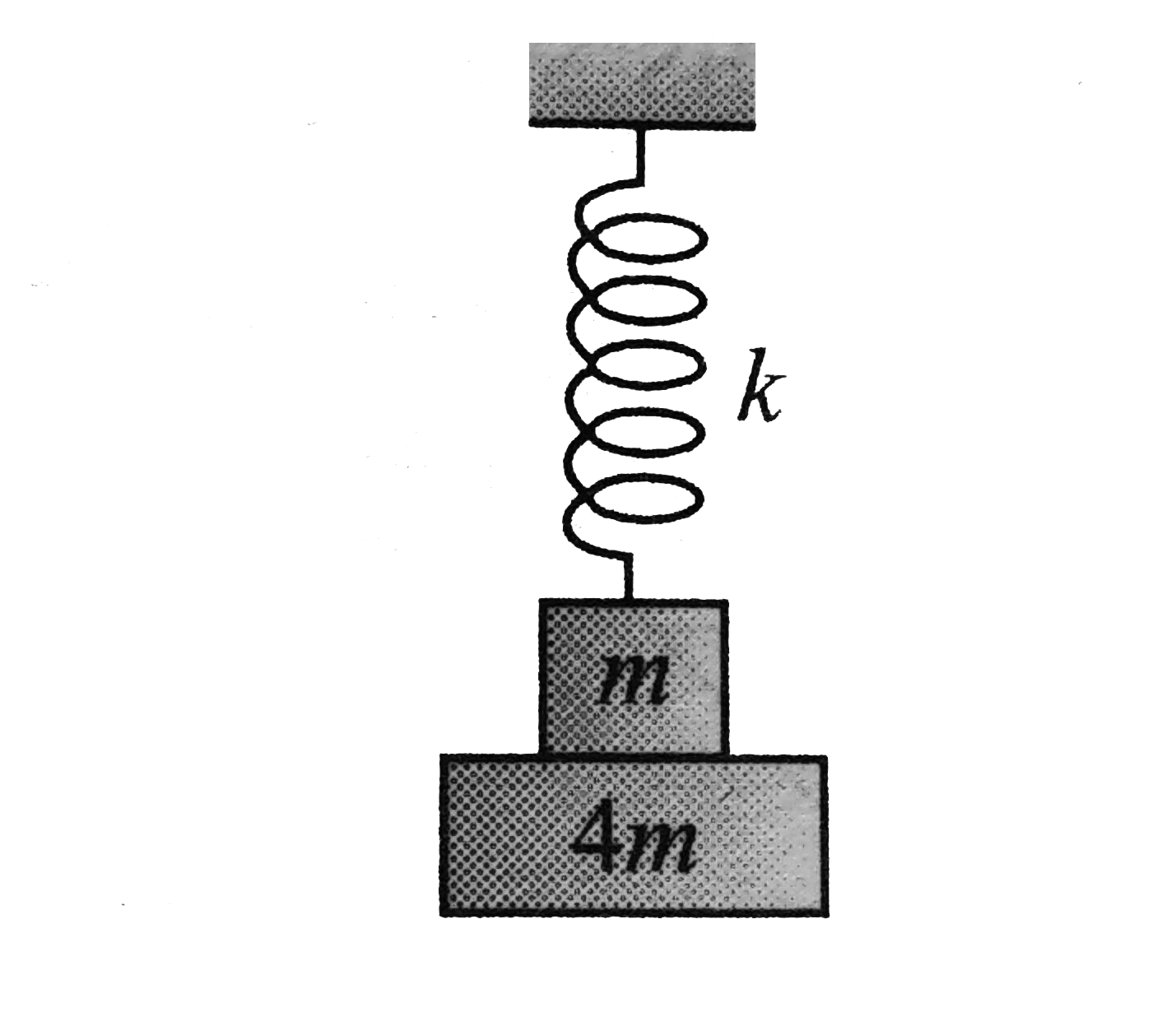
The correct Answer is:8mgk
Applying WET on block of mass m
Wg+Wsp=Kf−Ki
Let finally displacement of block from equilibrium is x.
−mg(5mgk+x)+12k(25m2g2k2)−12kx2=0
12kx2+mgx−15m2g22k=0
x=3mgk
Displacement from initial is 5mgk+3mgk=8mgk
Topper's Solved these Questions
- WORK, POWER & ENERGYBook:CENGAGE PHYSICSChapter:WORK, POWER & ENERGYExercise:Single Correct
Explore 100 Videos
- WORK, POWER & ENERGYBook:CENGAGE PHYSICSChapter:WORK, POWER & ENERGYExercise:Multiple Correct
Explore 25 Videos
- WORK, POWER & ENERGYBook:CENGAGE PHYSICSChapter:WORK, POWER & ENERGYExercise:Exercise 8.5
Explore 15 Videos
- VECTORSBook:CENGAGE PHYSICSChapter:VECTORSExercise:Exercise Multiple Correct
Explore 5 Videos
Similar Questions
Find how much m will rise if 4m falls away. Block are at rest and in equilibrium.
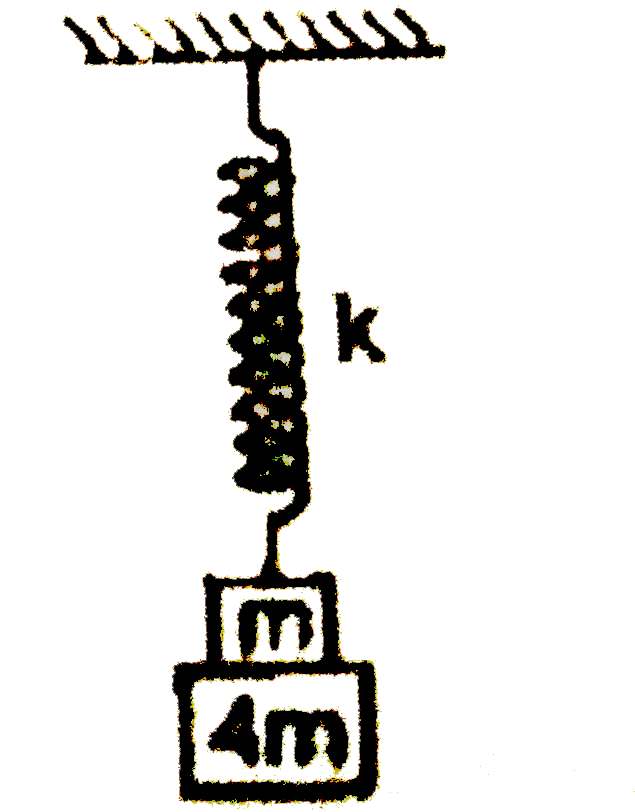
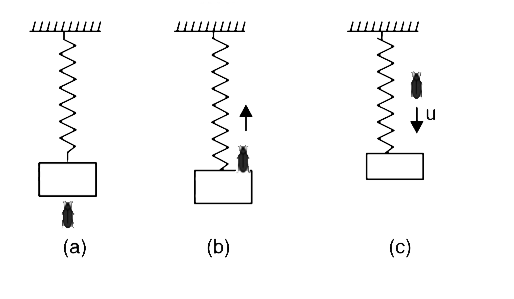
View SolutionA block of mass M is tied to a spring of force constant K and the system is suspended vertically. Consider three situations shown in fig. (a), (b) and (c).
(a) In fig. (a), an insect of mass M is clinging to the block and the system is in equilibrium. The insect leaves the block and falls. Find the amplitude of resulting oscillations.
(b) In fig. (b), an insect of mass M is resting on the top of the block and the system is in equilibrium. The insect suddenly jumps up with a speed u=g√MK and the block starts oscillating. Find amplitude of oscillation assuming that the insect never falls back on the block
(c) In fig. (c), an insect of mass M falls on the block this in equilibrium. The insect hits the block with velocity u=g√MK while moving downwards sticks to the block. Find the amplitude of oscillation.
View Solution
Knowledge Check
Two blocks A and B are connected by as string as shown in figure. Friction coefficient of the incline plane and block is 0.5. The mass of the block A is 5kg. If minimum & maximum values of masses of the block B for which the block remains in equilibrium are m1 & m2 then find value of (m2−m1).
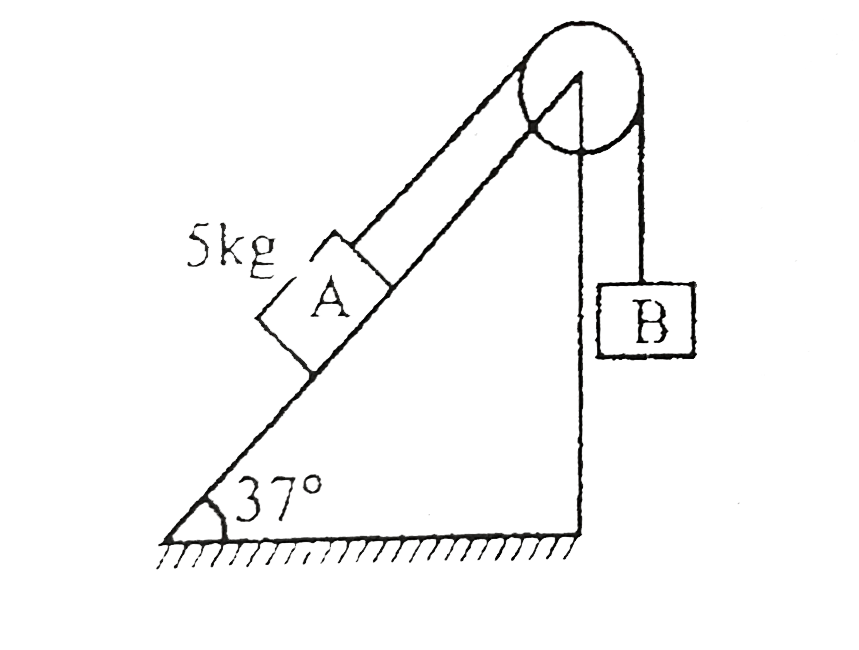 A4B6C3D2
A4B6C3D2Three blocks A,B, and C are suspended as shown in fig. Mass of each of blocks A and B is m. If the system is in equilibrium, and mass of C is M then
 AM>2mBM=2mCM<2mDNone of these
AM>2mBM=2mCM<2mDNone of theseA bob of mass 2m hanges by a string attached to the block of mass m of a spring blocks syetem. The whole arrangement is in a state of equilibrium. The bob of mass 2m is pulled down slowely by a distance x0 and released.
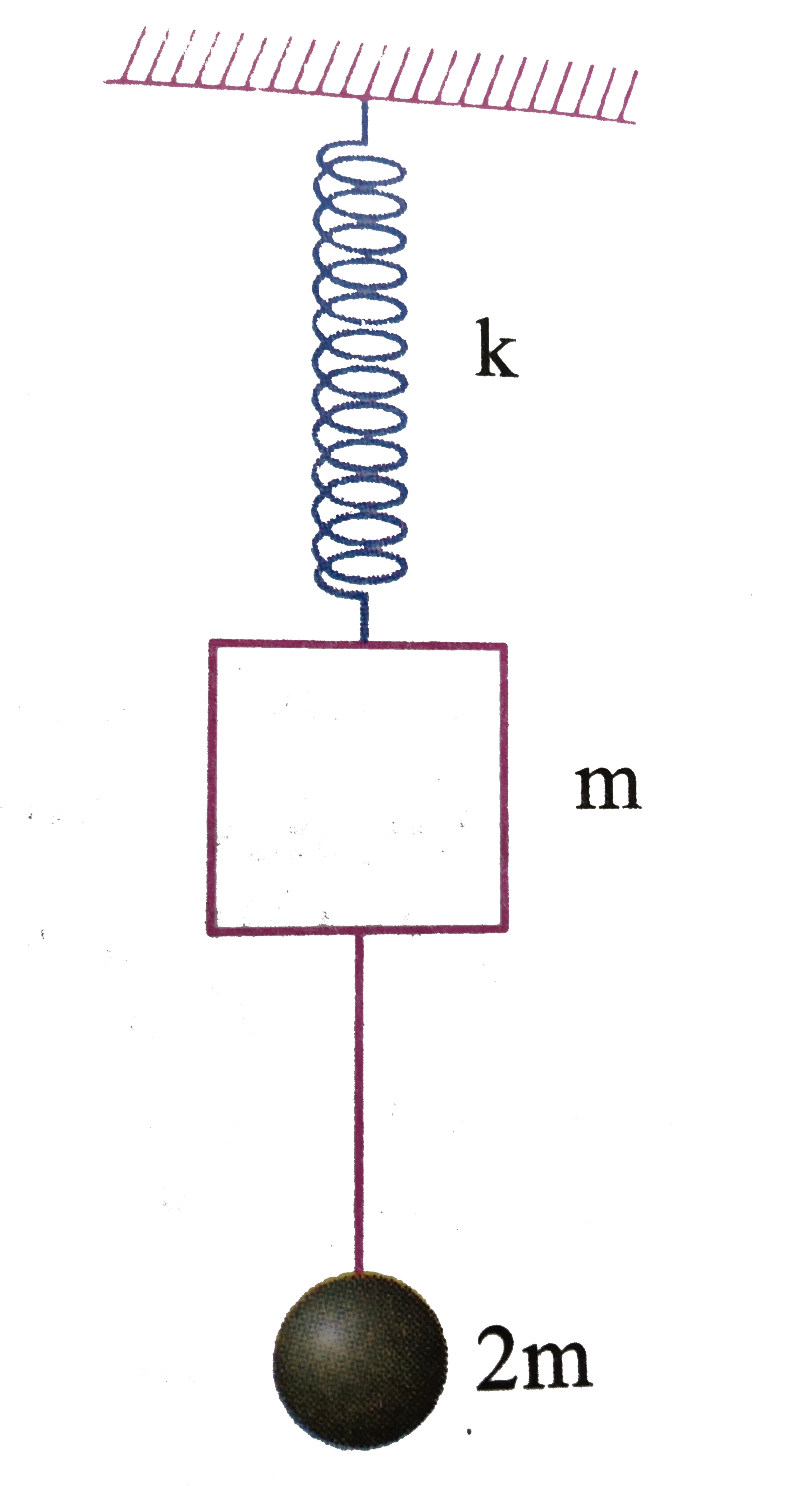 AFor x0=3mgk, maximum tension in string is 4mgBFor x0>3mgk, minimum tension in string is mgCFrequency of oscillation of system is 12π√k3m, for all non-zero values of x0DThe motion will remain simple harmonic for x0≥3mgk
AFor x0=3mgk, maximum tension in string is 4mgBFor x0>3mgk, minimum tension in string is mgCFrequency of oscillation of system is 12π√k3m, for all non-zero values of x0DThe motion will remain simple harmonic for x0≥3mgk
A block of mass 4m is attached to a vertical spring of spring constant k. The block is made by gluing two blocks of mass 3m and m, respectively. Initially, the block is in equilibrium and at rest (Fig. 15.13a). At t=0, the part of the block having ass 3m falls down. Considering hanging point on ceiling as y=0 and downward direction as positive y, find y-coordinate of mass m as a function of time.
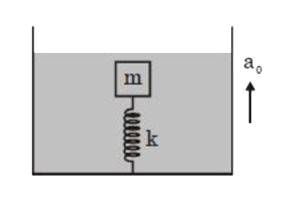
View SolutionA block of mass m and relative density y(<1) si attached to an ideal spring of constant K. The system is initially at rest and at equilibrium. If the container acceleration upwards with a0, find the increase in the elongation of the spring in equilibrium.
View SolutionA block of mass m is suspened through a spring of spring constant k and is in equlibrium. A sharp blow gives the block an initial downward velocity v. How far below the equilibrium psitin, the block comes to an instantaneous rest?
View SolutionA block of mass 'm' is connected to another block of mass 'M' by a spring (massless) of spring constant 'k' The blocks are kept on a smooth horizontal plane. Initially the blocks are at rest and the spring is unstretched Then a constant force 'F' starts acting on the block of mass 'M' to pull it. Find the force on the block of mass 'm' :
View SolutionA block of mass m is connected to another block of mass M by a spring (massless) of spring constant k. The block are kept on a smooth horizontal plane. Initially the blocks are at rest and the spring is unstretched. Then a constant force F starts acting on the block of mass M to pull it. Find the force of the block of mass M.
View Solution