Given in fig are examples of some potential energy functions in one dimension. The total enrgy of the particle is indicated by a cross on the ordinate axis. In each case, specify the regions, if any, in which the particle cannot be found for the given energy. Also, indicate the minimum total energy the particle must have in each case. Think of simple physical contexts for which these potential energy shapes are relevant.

Text Solution

Verified by Experts

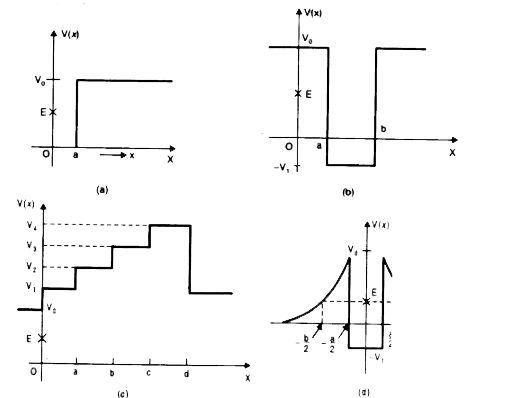
We know, that total energy $E = K . E + P . E$ or $K . E = E - P . E$ and kinetic energy can never be negative. The object can not exists in the region, where its K.E. Would become negative. A) IN the region between x=0 and x=a, potential energy is zero. So, kinetic energy will be negative in this region. Thus, the particle cannot be present in the region xgta. The minimum total energy that the particle can have in this case is zero.

Updated on:21/07/2023

The kinetic energy of the particles is maximum in the case of ____.
Aliquids
Bgases
Csolids
Dplasma
A particle with total energy $E$ moves in one dimemsional region where the potential energy is $U (x)$ The speed of the particle is zero where
A $U (x) = 0$
B $U (x) = E$
C $\frac{d U (x)}{d x} = 0$
D $\frac{d^{2} U (x)}{{d x}^{2}} = 0$
The total energy of a particle having a displacement x, executing simple harmonic motion is
A $\propto x$
B $\propto x^{2}$
Cindependent of x
D $\propto x^{1 / 2}$