Refer to figure Find a the magnitude, b x and y components and c. the angle with the X-axis of the resultant of −−→OA,−−→BCand−−→DE.
 .
.
The correct Answer is:A, B, C, D
x component of −−→OA=2cos30∘=√3
x component of −−→BC=1.5cos120∘ =- 1.5/2=-0.75)
x compon ent of −−→DE=1cos270∘= 1×0=0,
Topper's Solved these Questions
- BOOK - HC VERMA ENGLISHCHAPTER - PHYSICS AND MATHEMATICSEXERCISE - Question for short Answer14videos
PHYSICS AND MATHEMATICS
- BOOK - HC VERMA ENGLISHCHAPTER - PHYSICS AND MATHEMATICSEXERCISE - Objective 25videos
PHYSICS AND MATHEMATICS
- BOOK - HC VERMA ENGLISHCHAPTER - NEWTON'S LAWS OF MOTIONEXERCISE - Questions for short Answer17videos
NEWTON'S LAWS OF MOTION
- BOOK - HC VERMA ENGLISHCHAPTER - REST AND MOTION : KINEMATICSEXERCISE - Question for short Answer13videos
REST AND MOTION : KINEMATICS
Similar Questions
The magnitude of x and y components of →A are 7 and 6 respectively . Also , the magnitudes of x and y components of →A+→B are 11 and 9 respectively . Calculate the magnitude of vector →B
View SolutionThe magnitude of vectors −−→OA,−−→OBand−−→OC in figure are equal. Find the direction of −−→OA+−−→OB−−−→OC.
 .View Solution
.View Solution
Knowledge Check
- Question 1 - Select One
Two vectors →Aand→B inclined at an angle θ have a resultant →R which makes an angle α with →A. If the directions of →Aand→B are interchanged, the resultant will have the same
AdirectionBmagnitudeCdirection as well as magnitudeDnone of these - Question 2 - Select One
The magnitude of the x-component of vector →A is 3 and the magnitude of vector →A is 5. What is the magnitude of the y-component of vector →A ?
A3B4C5D8
Find the magnitude of the sum of →Aand→B and its direction with respect to the X - axis .
View SolutionTwo vectors →Aand→B inclined at an angle θ have a resultant →R which makes an angle α with →A. If the directions of →Aand→B are interchanged, the resultant will have the same
View SolutionThree vector as shown in (figure) have magnitudes |→a|=3,∣∣→b∣∣=4,and |→c|=10
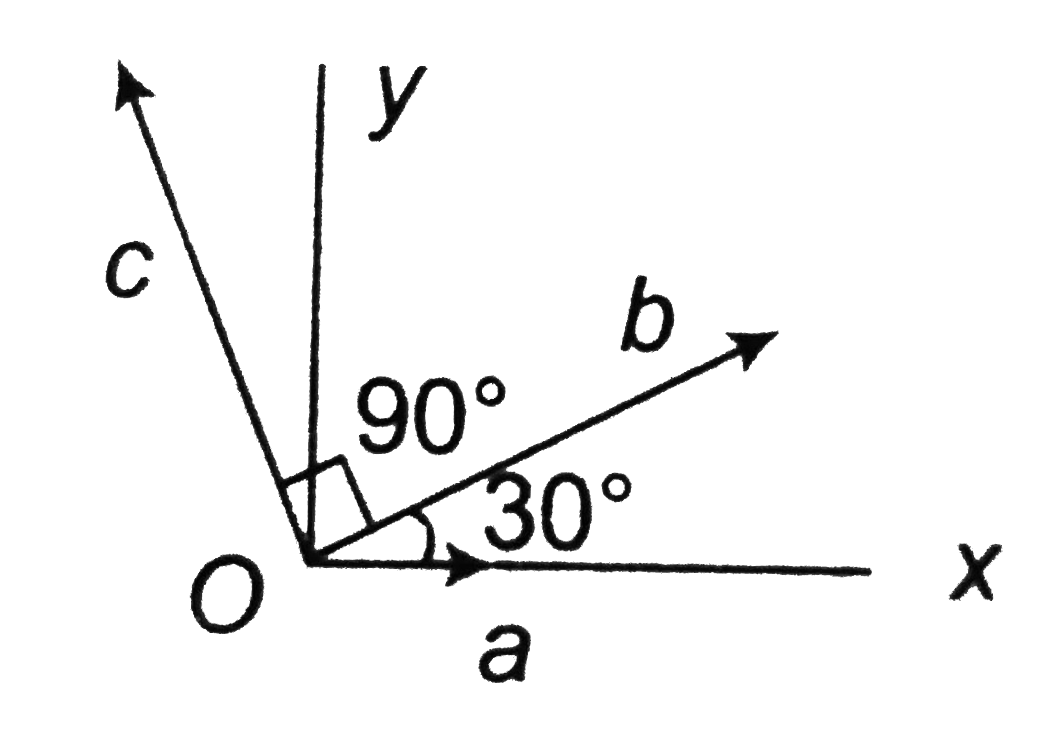
a.Find the x and y components of these vectors.
b.Find the numbers p and q such that →c=p→a+q→b.View SolutionTwo vectors →A and →B have equal magnitudes . If magnitude of →A+→B is equal to n× the magnitude of →A−→B , then the angle between →A and →B is
View SolutionThe resultant of two vectors →A and →B is perpendicular to the vector →A and its magnitudes is equal to half of the magnitudes of vector →B (figure). The angle between →A and →B is
 View Solution
View SolutionA vector →B which has a magnitude 8.0 is added to a vector →A which lies along the x-axis. The sum of these two vector is a third vector which lies along the y-axis and has a magnitude that is twice the magnitude of →A. Find the magnitude of →A
View Solution
