Show that the adiabatic curve is steeper than the isothermal curve.
To show that the adiabatic curve is steeper than the isothermal curve, we will analyze the equations governing both processes and derive their slopes.
1. Understanding the Processes:
- An adiabatic process is one in which no heat is exchanged with the surroundings. For an ideal gas, the relationship between pressure (P), volume (V), and temperature (T) can be described by the equation:
PVγ=constant
where γ (gamma) is the heat capacity ratio Cp/Cv.
2. Differentiating the Adiabatic Equation:
- To find the slope of the adiabatic curve, we differentiate the equation PVγ=C:
ddV(PVγ)=0
- Applying the product rule:
VγdPdV+P⋅γVγ−1dVdV=0
- Simplifying gives:
VγdPdV+γPVγ−1=0
- Rearranging leads to:
dPdV=−γPV
- This indicates that the slope of the adiabatic curve is:
madiabatic=−γPV
3. Understanding the Isothermal Process:
- An isothermal process occurs at constant temperature. The relationship for an ideal gas is given by:
PV=constant
4. Differentiating the Isothermal Equation:
- We differentiate the equation PV=C:
ddV(PV)=0
- Using the product rule:
VdPdV+P⋅dVdV=0
- This simplifies to:
VdPdV+P=0
- Rearranging gives:
dPdV=−PV
- Thus, the slope of the isothermal curve is:
misothermal=−PV
5. Comparing the Slopes:
- Now we compare the slopes of the adiabatic and isothermal curves:
madiabaticmisothermal=−γPV−PV=γ
- Since γ (the heat capacity ratio) is always greater than 1 for any ideal gas, we conclude that:
madiabatic>misothermal
- Therefore, the adiabatic curve is steeper than the isothermal curve.
Conclusion:
The adiabatic curve is indeed steeper than the isothermal curve due to the factor of γ, which is greater than 1.
- An adiabatic process is one in which no heat is exchanged with the surroundings. For an ideal gas, the relationship between pressure (P), volume (V), and temperature (T) can be described by the equation:
PVγ=constant
where γ (gamma) is the heat capacity ratio Cp/Cv.
- To find the slope of the adiabatic curve, we differentiate the equation PVγ=C:
ddV(PVγ)=0
- Applying the product rule:
VγdPdV+P⋅γVγ−1dVdV=0
- Simplifying gives:
VγdPdV+γPVγ−1=0
- Rearranging leads to:
dPdV=−γPV
- This indicates that the slope of the adiabatic curve is:
madiabatic=−γPV
- An isothermal process occurs at constant temperature. The relationship for an ideal gas is given by:
PV=constant
- We differentiate the equation PV=C:
ddV(PV)=0
- Using the product rule:
VdPdV+P⋅dVdV=0
- This simplifies to:
VdPdV+P=0
- Rearranging gives:
dPdV=−PV
- Thus, the slope of the isothermal curve is:
misothermal=−PV
- Now we compare the slopes of the adiabatic and isothermal curves:
madiabaticmisothermal=−γPV−PV=γ
- Since γ (the heat capacity ratio) is always greater than 1 for any ideal gas, we conclude that:
madiabatic>misothermal
- Therefore, the adiabatic curve is steeper than the isothermal curve.
The adiabatic curve is indeed steeper than the isothermal curve due to the factor of γ, which is greater than 1.
Topper's Solved these Questions
- INTERNAL ENERGY BOOK - ICSECHAPTER - INTERNAL ENERGY EXERCISE - VERY SHORT ANSWER QUESTIONS
17 Videos
- INTERNAL ENERGY BOOK - ICSECHAPTER - INTERNAL ENERGY EXERCISE - SELECTED PROBLEMS (FROM FIRST LAW OF THERMODYNAMICS )
6 Videos
- INTERNAL ENERGY BOOK - ICSECHAPTER - INTERNAL ENERGY EXERCISE - LONG ANSWER QUESTIONS
8 Videos
- GRAVITATIONBOOK - ICSECHAPTER - GRAVITATIONEXERCISE - FROM THE HUBBLE TELESCOP
2 Videos
- MOTION IN FLUIDS BOOK - ICSECHAPTER - MOTION IN FLUIDS EXERCISE - SELECTED PROBLEMS (FROM POISEUILLE.S FORMULA)
19 Videos
Similar Questions
An ideal gas (1 mol ,monatomic) is in the initial state P (see diagram) on an isothermal curve A at a temperature T0 . It is brought under a constant volume (2V0) process to Q which lies on an adiabatic curve B intersecting the isothermal curve A at (P0,V0,T0) . The change in the internal energy of the gas (in terms of T0 ) during the process is (22/3=1.587)
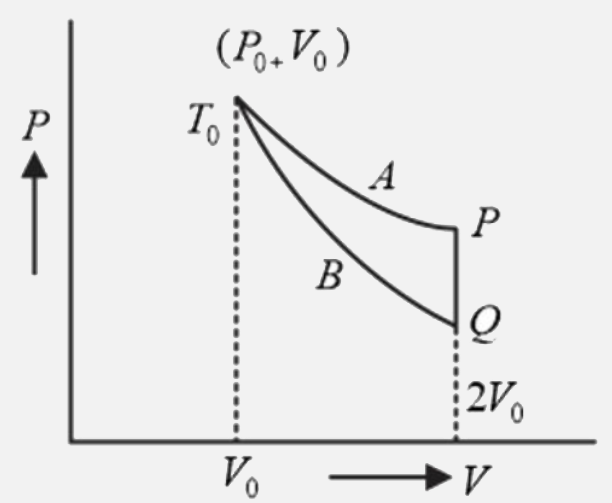
View SolutionStatement-1: Temperature of a system can be increased without supplying heat.
Statement-2: If the initial volume of system is equal to final volume in a process then work done is necessarily zero.
Statement-3: If an adiabatic curve for a gas intersect with an isothermal curve for the same gas then at the point of intersection, the ratio of slope of the adiabatic and the isothermal curve is γ.
View SolutionShow that the slope of adiabatic curve at any point is Yama times the slope of an isothermal curve at the corresponding point.
View SolutionState true or false. Work done by an ideal gas in adiabatic expansion is less than that in isothermal process (for same temperature range)
View SolutionShow that the slope of p−V diagram is greater for an adiabatic process as compared to an isothermal process.
View SolutionA sphere is inscribed in a cylinder such that spere touches the cylinder . Show that the curved surface of spere is equal to the curved surface of cylinder.
View SolutionThe curves A and B in the figure shown P-V graphs for an isothermal and an adiabatic process for an idea gas. The isothermal process is represented by the curve A.
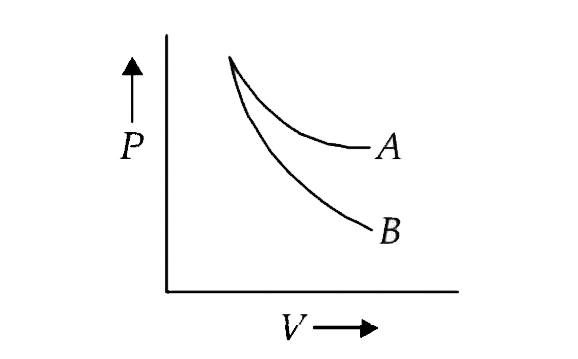
View SolutionThe curves A and B in the figure shown P-V graphs for an isothermal and an adiabatic process for an idea gas. The isothermal process is represented by the curve A.

View SolutionFor a p-V plot, the slope of an adiabatic curve = x × slope of isothermal at the same point. Here x is :
View SolutionShow that there is no cubic curve for which the tangent lines at two distinct points coincide.
View Solution