Three particles of msses 1.0 kg 2.0 kg and 3.0 kg are placed at the corners A,B and C respectively of an equilateral triangle ABC of edge 1m. Locate the centre ofmass of the system.
To locate the center of mass of the system of three particles placed at the corners of an equilateral triangle, we can follow these steps:
Step 1: Define the Coordinates of the Particles
We have three particles with the following masses and positions:
- Particle A (mass = 1.0 kg) at coordinates (0.5, √32)
- Particle B (mass = 2.0 kg) at coordinates (0, 0)
- Particle C (mass = 3.0 kg) at coordinates (1, 0)
Step 2: Calculate the Total Mass
The total mass M of the system is the sum of the individual masses:
M=mA+mB+mC=1.0kg+2.0kg+3.0kg=6.0kg
Step 3: Calculate the x-coordinate of the Center of Mass
The x-coordinate of the center of mass xcm can be calculated using the formula:
xcm=mAxA+mBxB+mCxCM
Substituting the values:
xcm=(1.0kg⋅0.5)+(2.0kg⋅0)+(3.0kg⋅1)6.0kg=0.5+0+3.06.0=3.56.0≈0.5833
Step 4: Calculate the y-coordinate of the Center of Mass
The y-coordinate of the center of mass ycm can be calculated using the formula:
ycm=mAyA+mByB+mCyCM
Substituting the values:
ycm=(1.0kg⋅√32)+(2.0kg⋅0)+(3.0kg⋅0)6.0kg=√326.0=√312≈0.1443
Step 5: Conclusion
The coordinates of the center of mass of the system are approximately:
(xcm,ycm)≈(0.5833,0.1443)
We have three particles with the following masses and positions:
- Particle A (mass = 1.0 kg) at coordinates (0.5, √32)
- Particle B (mass = 2.0 kg) at coordinates (0, 0)
- Particle C (mass = 3.0 kg) at coordinates (1, 0)
The total mass M of the system is the sum of the individual masses:
M=mA+mB+mC=1.0kg+2.0kg+3.0kg=6.0kg
The x-coordinate of the center of mass xcm can be calculated using the formula:
xcm=mAxA+mBxB+mCxCM
Substituting the values:
xcm=(1.0kg⋅0.5)+(2.0kg⋅0)+(3.0kg⋅1)6.0kg=0.5+0+3.06.0=3.56.0≈0.5833
The y-coordinate of the center of mass ycm can be calculated using the formula:
ycm=mAyA+mByB+mCyCM
Substituting the values:
ycm=(1.0kg⋅√32)+(2.0kg⋅0)+(3.0kg⋅0)6.0kg=√326.0=√312≈0.1443
The coordinates of the center of mass of the system are approximately:
(xcm,ycm)≈(0.5833,0.1443)
Topper's Solved these Questions
- CENTRE OF MASS, LINEAR MOMENTUM, COLLISIONBOOK - HC VERMACHAPTER - CENTRE OF MASS, LINEAR MOMENTUM, COLLISIONEXERCISE - Objective 2
11 Videos
- CALORIMETRYBOOK - HC VERMACHAPTER - CALORIMETRYEXERCISE - Exercises
18 Videos
- CIRCULAR MOTIONBOOK - HC VERMACHAPTER - CIRCULAR MOTIONEXERCISE - Exercises
30 Videos
Similar Questions
Three particles of masses 1kg, 2kg and 3kg are placed at the corners A, B and C respectively of an equilateral triangle ABC of edge 1m. Find the distance of their centre of mass from A.
View SolutionThree masses 3,4 and 5 kg are located at the corners of an equilateral triangle of side 1m. Locate to centre of mass of the system.
View Solution
Knowledge Check
Three particles of masses 1kg,32kg,and2kg are located at the vertices of an equilateral triangle of side a. The x,y coordinates of the centre of mass are.
A(5a9,2a3√3)B(2a33,5a9)C(5a9,2a√3)D(2a√3,5a9)
Three masses 3,4 and 5 kg are located at the corners of an equilateral triangle of side 1m. Locate to centre of mass of the system.
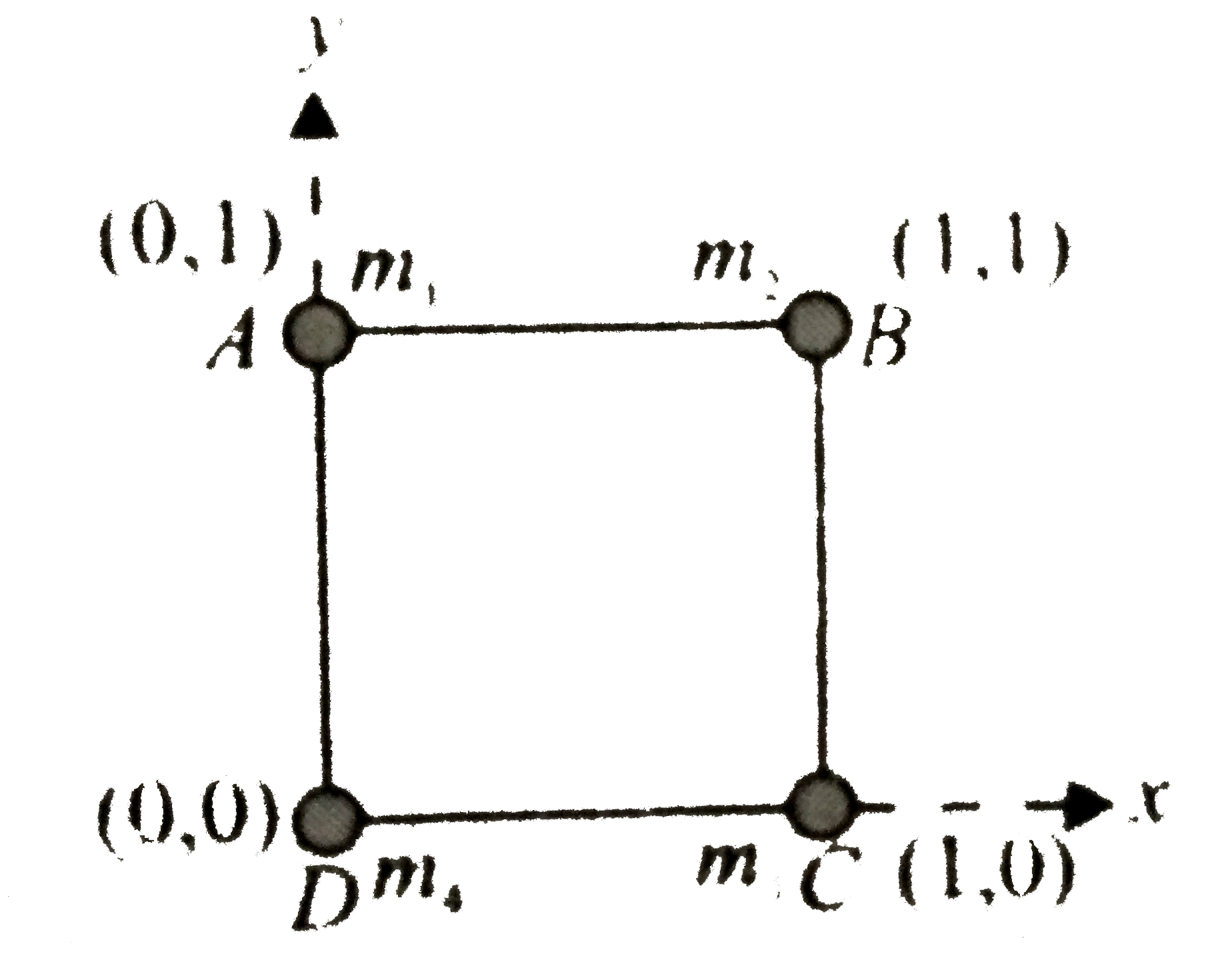
View SolutionFour particles of masses 1kg,2kg,3kg and 4kg are placed the four vertices A,B,C and D, respectively, of a square of side 1m. Find the position of centre of mass of the particles.
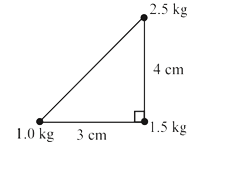
View SolutionThree point particles of masses 1.0 kg, 1.5 kg and 2.5 kg are placed at three corners of a right angle triangle as shown in figure. The centre of mass of the system is at a point :
View SolutionThree point masses m1, m2, m3 are placed at the corners of an equilateral triangle of side 2 m. Locate the centre of mass of the system.
m1=2kg,m2=3kg,m3=4kg
View SolutionThree balls of masses 1kg,2kgand3kg are arranged at the corners of an equilateral triangle of side 1m. What will be the moment of inertia of the system and perpendicular to the plane of the triangle.
View SolutionThree particles of msses 1.0 kg , , 2.0 kg and 3.0 kg are placed at the corners A,B and C respectively of an equilateral triangle ABC of edge 1m. Locate the centre of mass of the system.
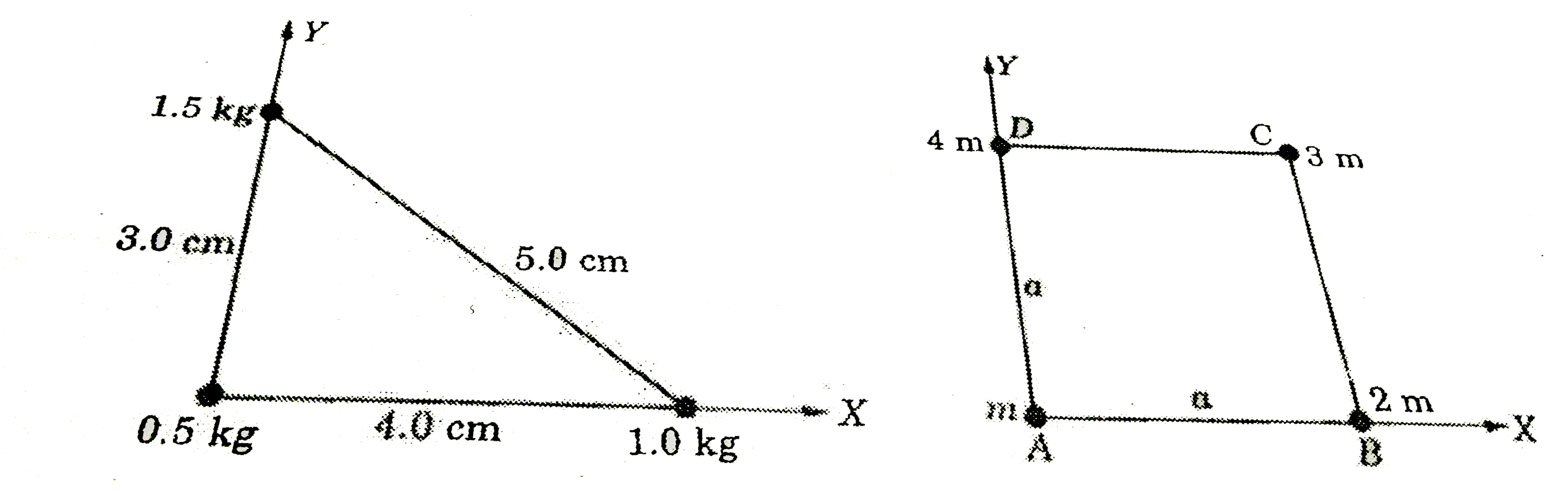
View SolutionThree particles of masses 0.50 kg, 1.0 kg and are placed at the corners of a right angle triangle, as shown in fig. Locate the centre of mass of the system.
View Solution
