Two blocks of masses 6kg and 4kg are attached to the two ends of a massless string passing over a smooth fixed pulley. if the system is released, the acceleration of the centre of mass of the system will be
g, vertically downwards
g5, vertically downwards
g25, vertically downwards
zero
The correct Answer is:C
To find the acceleration of the center of mass of the system consisting of two blocks of masses 6 kg and 4 kg, we can follow these steps:
Step 1: Identify the masses and the gravitational acceleration
Let:
- m1=6kg (mass of the first block)
- m2=4kg (mass of the second block)
- g=9.8m/s2 (acceleration due to gravity)
Step 2: Determine the net force acting on the system
When the system is released, the heavier mass (6 kg) will accelerate downwards while the lighter mass (4 kg) will accelerate upwards. The net force F acting on the system can be calculated as:
F=m1g−m2g=(m1−m2)g
Substituting the values:
F=(6kg−4kg)⋅g=2g
Step 3: Calculate the total mass of the system
The total mass M of the system is:
M=m1+m2=6kg+4kg=10kg
Step 4: Find the acceleration of the system
Using Newton's second law, the acceleration a of the system can be calculated as:
a=FM=2g10kg=g5
Step 5: Determine the acceleration of the center of mass
The acceleration of the center of mass acm of the system is the same as the acceleration of the system since the two blocks are connected by a string. Thus:
acm=g5
Step 6: Direction of acceleration
Since the 6 kg mass is moving downwards, the acceleration of the center of mass will also be directed downwards.
Final Answer
The acceleration of the center of mass of the system is:
g5 downwards
---
Let:
- m1=6kg (mass of the first block)
- m2=4kg (mass of the second block)
- g=9.8m/s2 (acceleration due to gravity)
When the system is released, the heavier mass (6 kg) will accelerate downwards while the lighter mass (4 kg) will accelerate upwards. The net force F acting on the system can be calculated as:
F=m1g−m2g=(m1−m2)g
Substituting the values:
F=(6kg−4kg)⋅g=2g
The total mass M of the system is:
M=m1+m2=6kg+4kg=10kg
Using Newton's second law, the acceleration a of the system can be calculated as:
a=FM=2g10kg=g5
The acceleration of the center of mass acm of the system is the same as the acceleration of the system since the two blocks are connected by a string. Thus:
acm=g5
Since the 6 kg mass is moving downwards, the acceleration of the center of mass will also be directed downwards.
The acceleration of the center of mass of the system is:
g5 downwards
Topper's Solved these Questions
- CENTRE OF MASSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CENTRE OF MASSEXERCISE - Multiple Correct
25 Videos
- CENTRE OF MASSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CENTRE OF MASSEXERCISE - Assertion - Reasoning
2 Videos
- CENTRE OF MASSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CENTRE OF MASSEXERCISE - Subjective
23 Videos
- CALORIMETRYBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CALORIMETRYEXERCISE - Solved Example
13 Videos
- DIMENSIONS & MEASUREMENTBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - DIMENSIONS & MEASUREMENTEXERCISE - Integer
2 Videos
Similar Questions
Two masses 2 kg and 4 kg are connected at the two ends of light inextensible string passing over a frictionless pulley. If the masses are released, then find the acceleration of the masses and the tension in the string.
View SolutionTwo particles having mass ratio n:1 are interconnected by a light inextensible string that passes over a smooth pulley . If the system is released , then the acceleration of the centre of mass of the system is
View Solution
Knowledge Check
Two blocks of masses of 40 kg and 30 kg are connected by a weightless string passing over a frictionless pulley as shown in the figure.
 A0.7ms−2B0.8ms−2C0.6ms−2D0.5ms−2
A0.7ms−2B0.8ms−2C0.6ms−2D0.5ms−2
Two bodies of masses 4 kg and 6 kg are tied to the ends of a massless string. The string passes over a frictionless pulley. The acceleration of the system is :
View SolutionTwo bodies of masses 1 kg and 2 kg are connected by a very light string passed over a clamped light smooth pulley. If the system is released from rest, find the acceleration of the two masses and the tension in the string
View SolutionTwo unequal masses of 1 kg and 2 kg are attached at the two ends of a light inextensible string passing over a smooth pulley as shown . If the system is released from rest, find the work done by string on both the blocks in 1 s.
(Take g=10m/s2).
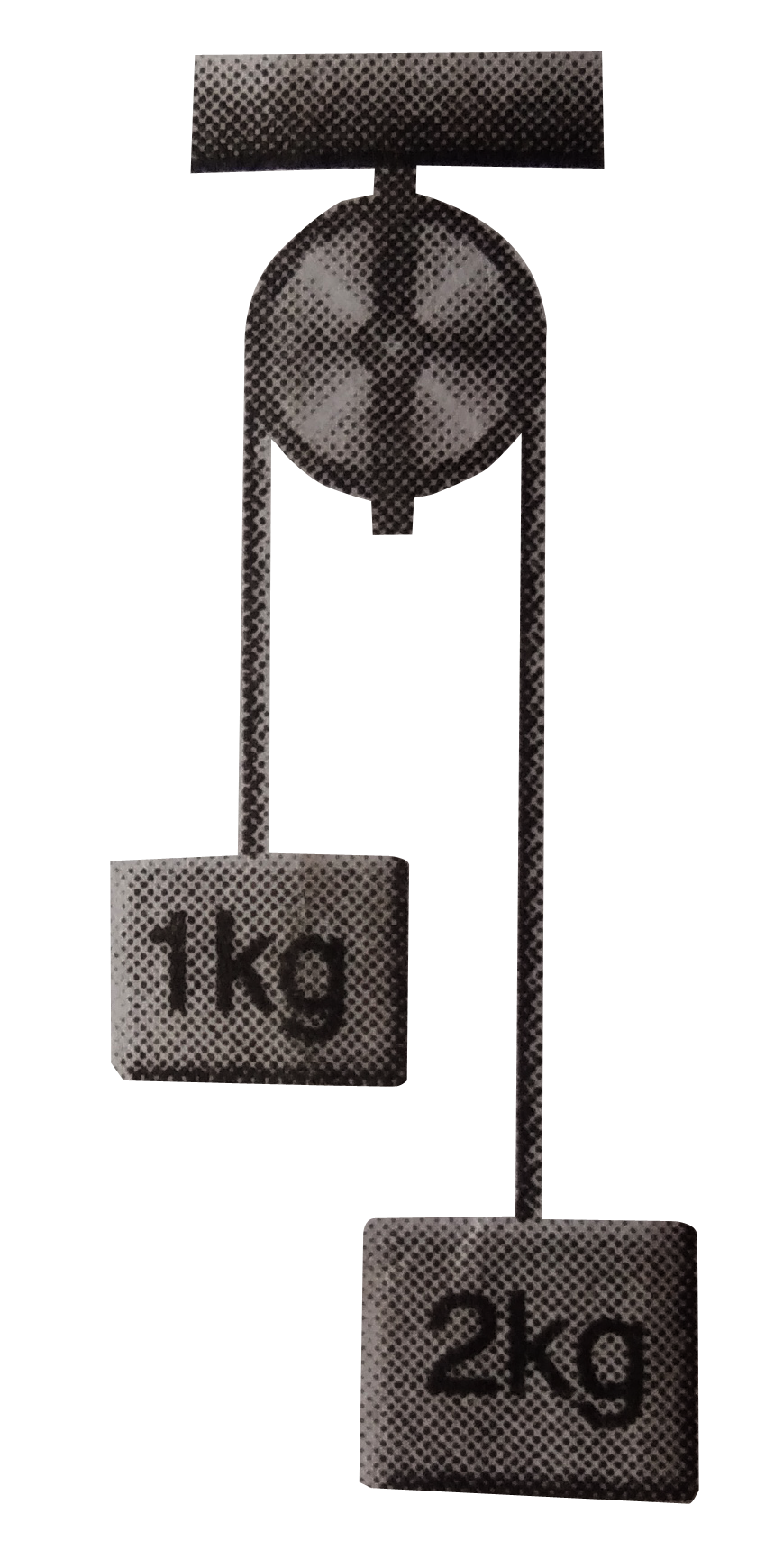
View SolutionTwo masses 2 kg and 4 kg are connected at two ends of light inextensible string passing over a frictionless pulley . If the masses are released , then find the accelaration of the masses and the tension in the string .
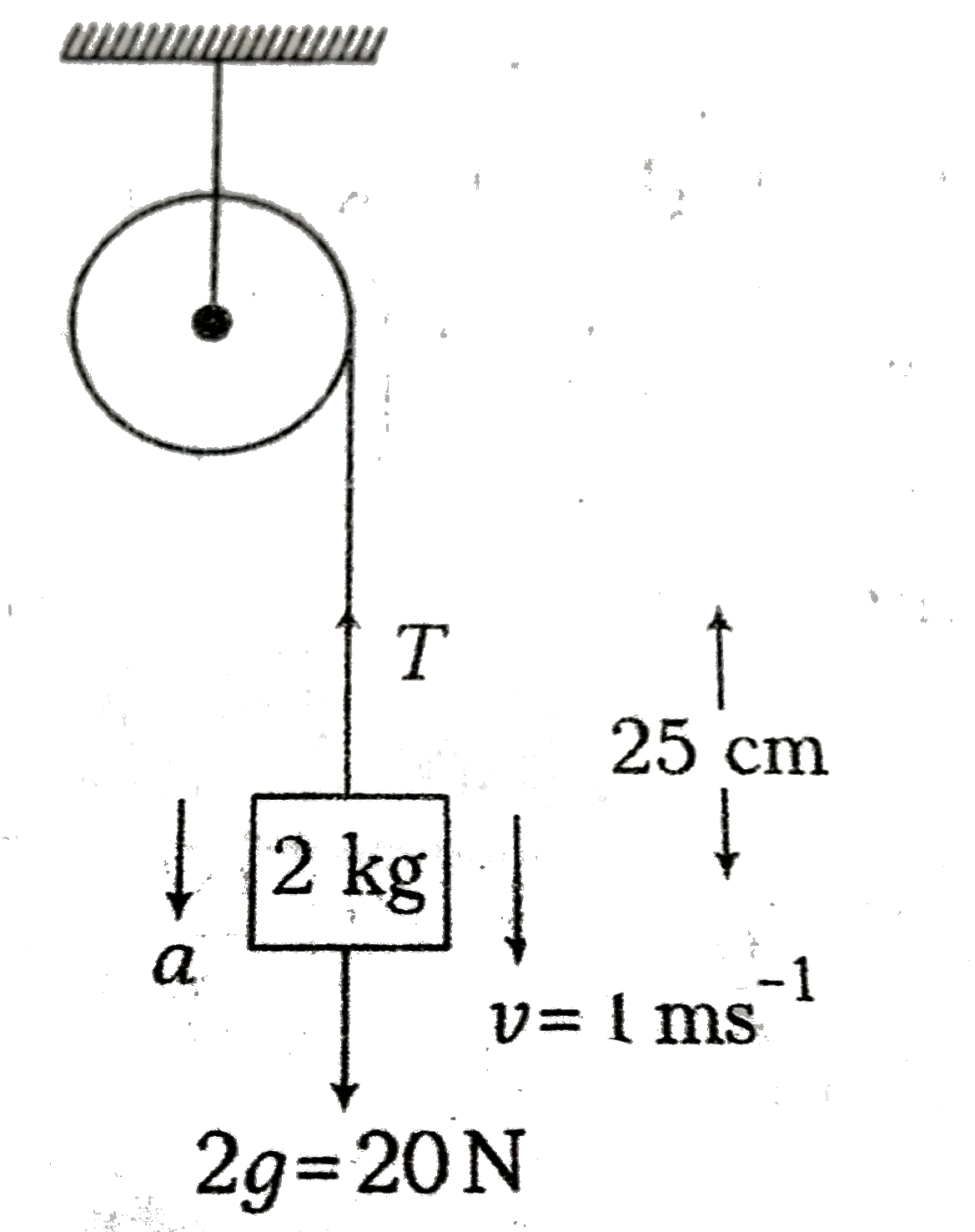
View SolutionTwo unequal masses of 1 kg and 2 kg are attached at the two ends of a light inextensible string passing over a smooth pulley as shown in figure. If the system is released from rest, find the work done by string on both the blocks in 1 s. (Take g = 10 ms−2)
View SolutionTwo blocks of mass 5 kg and 3 kg are attached to the ends of a string passing over a smooth pulley fixed to the ceiling of an elevator. A man inside the elevator accelerated upwards, finds the acceleration of the blocks to be 932g. The acceleration of the elevator is
View SolutionTwo blocks of masses 2 kg and 4 kg are hanging with the help of massless string passing over an ideal pulley inside an elevator. The elevator is moving upward with an acceleration g2. The tension in the string connected between the blocks will be (Take g=10m/s2).
View Solution
