Two identical smooth disc of radius R have been placed on a frictionless table touching each other. Another circular plate is placed between them as shown in figure. The mass per unit area of each object is σ, and the line joining the centers of the plate and the disc is θ
(a) Find the minimum horizontal force F0 that must be applied to the two discs to keep them together.
(b) Angle θ can be changed by changing the size of the circular plate. Find F0 when θ→0.
[use cosθ=1−θ22 and sinθ=θfor smallθ]
(c) Find F0 when θ→π2 . Explain the result .
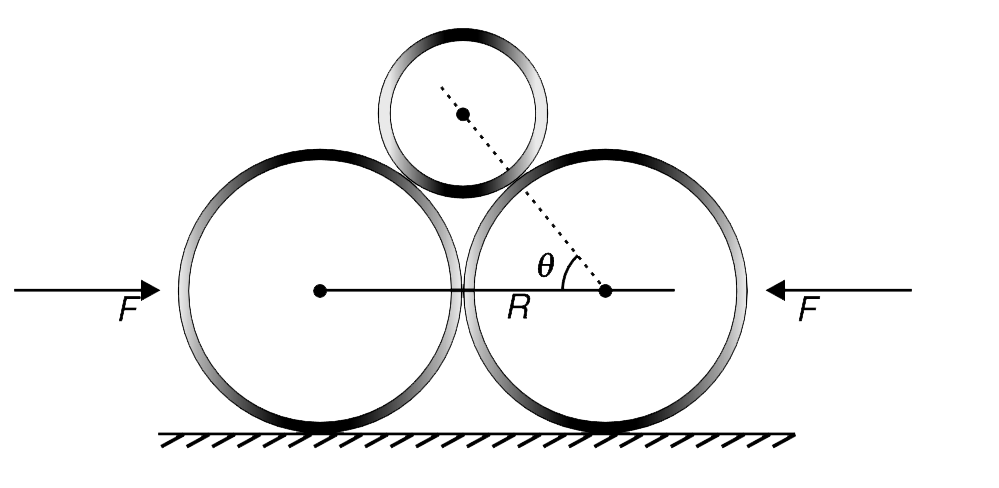
The correct Answer is:(a) F0=σπR2g(1−cosθ)22sinθ.cosθ
(b) F0=0
(c) ∞
Step by step video, text & image solution for Two identical smooth disc of radius R have been placed on a frictionless table touching each other. Another circular plate is placed between them as shown in figure. The mass per unit area of each object is sigma, and the line joining the centers of the plate and the disc is theta (a) Find the minimum horizontal force F_(0) that must be applied to the two discs to keep them together. (b) Angle theta can be changed by changing the size of the circular plate. Find F_(0) when theta rarr 0. [use "cos"theta = 1 - (theta^(2))/(2) and "sin"theta = theta "for small" theta] (c) Find F_(0) when theta rarr (pi)/(2) . Explain the result . by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Topper's Solved these Questions
Similar Questions
A disc of radius R and mass M is under pure rolling under the action of a force F applied at the topmost point as shown in figure. There is sufficient friciton between the disc and the horizontal surface. The acceleration is given as
 View Solution
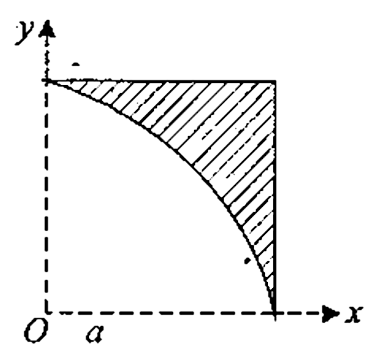
View SolutionFron a sqaure plate of side a,a quarter circular disc of radius a is removed as shown in figure. Find the coordinates of centre of mass of the remaining part.
 View Solution
View Solution
Knowledge Check
- Question 1 - Select One
Three identical spheres each of radius R are placed touching each other on a horizontal table as shown in figure. The co-ordinates of centre of mass are :
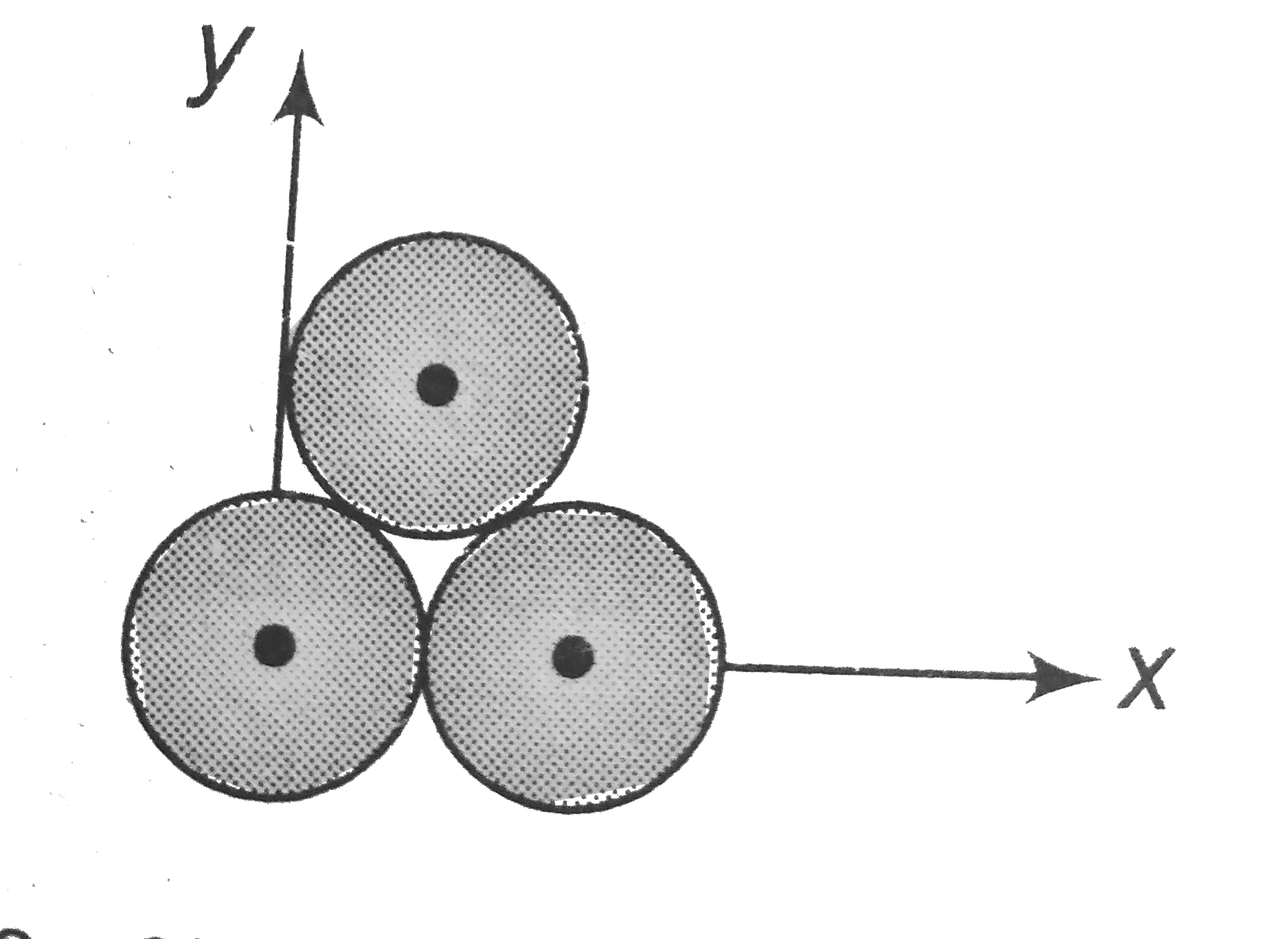 .A(R,R)B(0,0)C(R2,R2)D(R,R√3)
.A(R,R)B(0,0)C(R2,R2)D(R,R√3) - Question 2 - Select One
Three identical metal balls each of radius r are placed touching each other on a horizontal surface such that an equilateral triangle is formed, when the center of three balls are joined. The center of mass of system is located at the
Ahorizontal surfaceBcenter one of the ballsCline joining centers of any two ballsDpoint of intersection of medians
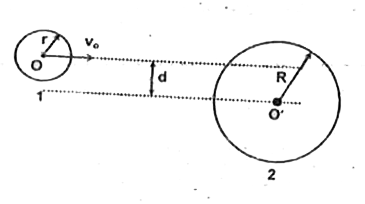
A disc of mass m is moving with constant speed v0 on a smooth horizontal table. Another disc of mass M is placed on the table at rest as shown in the figure. If the collision is elastic, find the velocity of the disc after collision. Both disc lie on the same horizontal plane of the table.
 View Solution
View SolutionA square plate of edge d and a circular disc of diameter d are placed toucing ech other at the midpoint of san edge of th plate as shown in figure. Locate the centre of mass of the combination assuming same mass per unit area for the two plates.
View SolutionThree discs each of mass M and radius R are placed in contact with each other as shown in figure here. Then the MI of the system about an axis XX' is
 View Solution
View Solution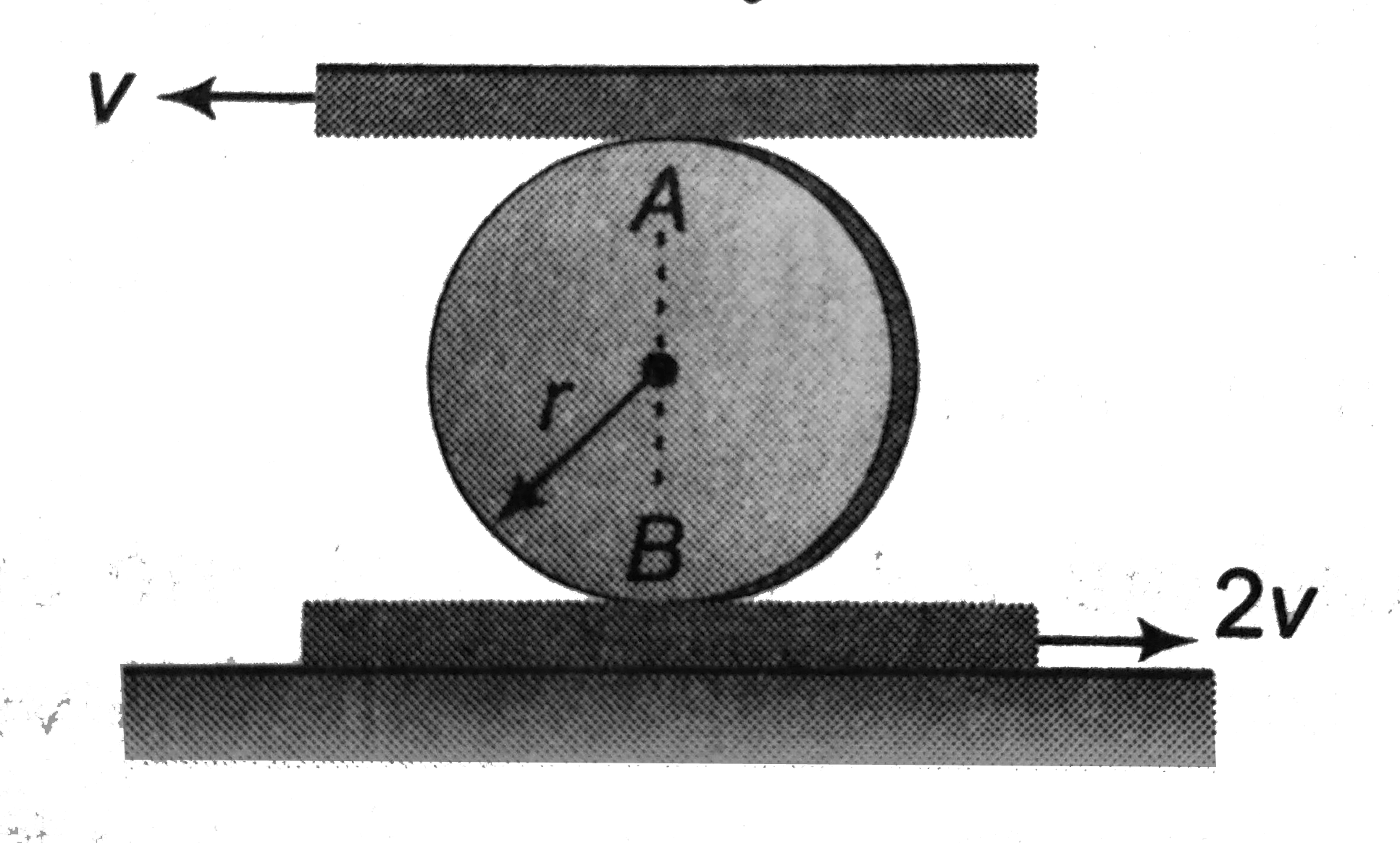
the disc of the radius r is confined to roll without slipping at A and B if the plates have the velocities shown, determine the angular velocity of the disc.View SolutionA disc of radius R is placed on a square plate of edge 4R made up of the same sheet with their planes parallel such that any two adjacent sides ofsquare touch the disc. Find the distance of the centre of mass of the system from the centre of square plate?
View SolutionA circular disc of radius b has a hole of radius a at its centre (see figure). If the mass per unit area of the disc varies as (σ0r). then the radius of gyration of the disc about its axis passing through the centre is:
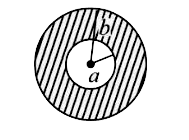 View Solution
View Solution

