Calculate the mean deviation about the median of the following frequency distribution: 5 7 9 11 13 15 17 Having Frequency 2 4 6 8 10 12 8
To calculate the mean deviation about the median for the given frequency distribution, we will follow these steps:
Step 1: List the Data
We have the following observations (Xi) and their corresponding frequencies (fi):
| Xi | Frequency (fi) |
|----|----------------|
| 5 | 2 |
| 7 | 4 |
| 9 | 6 |
| 11 | 8 |
| 13 | 10 |
| 15 | 12 |
| 17 | 8 |
Step 2: Calculate Cumulative Frequency (cf)
We calculate the cumulative frequency (cf) by adding the frequencies cumulatively:
- cf for 5 = 2
- cf for 7 = 2 + 4 = 6
- cf for 9 = 6 + 6 = 12
- cf for 11 = 12 + 8 = 20
- cf for 13 = 20 + 10 = 30
- cf for 15 = 30 + 12 = 42
- cf for 17 = 42 + 8 = 50
So, the cumulative frequency table looks like this:
| Xi | fi | cf |
|----|----|----|
| 5 | 2 | 2 |
| 7 | 4 | 6 |
| 9 | 6 | 12 |
| 11 | 8 | 20 |
| 13 | 10 | 30 |
| 15 | 12 | 42 |
| 17 | 8 | 50 |
Step 3: Find the Median
To find the median, we need to calculate n/2:
- Total frequency n=50
- n/2=50/2=25
Now, we look for the cumulative frequency just greater than 25, which is 30 (corresponding to Xi = 13). Therefore, the median (M) is:
Median (M) = 13
Step 4: Calculate Mean Deviation
The formula for mean deviation about the median is given by:
MD=1n∑(fi|Xi−M|)
Where |Xi−M| is the absolute deviation from the median.
Step 5: Calculate Absolute Deviations
Now we calculate |Xi−M| for each observation:
| Xi | fi | |Xi - M| | fi * |Xi - M| |
|----|----|---------|-----------------|
| 5 | 2 | 8 | 16 |
| 7 | 4 | 6 | 24 |
| 9 | 6 | 4 | 24 |
| 11 | 8 | 2 | 16 |
| 13 | 10 | 0 | 0 |
| 15 | 12 | 2 | 24 |
| 17 | 8 | 4 | 32 |
Step 6: Sum of fi|Xi−M|
Now we sum the last column:
∑(fi|Xi−M|)=16+24+24+16+0+24+32=136
Step 7: Calculate Mean Deviation
Now we can substitute into the mean deviation formula:
MD=1n∑(fi|Xi−M|)=13650=2.72
Final Result
The mean deviation about the median is:
Mean Deviation (MD) = 2.72
We have the following observations (Xi) and their corresponding frequencies (fi):
|----|----------------|
| 5 | 2 |
| 7 | 4 |
| 9 | 6 |
| 11 | 8 |
| 13 | 10 |
| 15 | 12 |
| 17 | 8 |
We calculate the cumulative frequency (cf) by adding the frequencies cumulatively:
- cf for 7 = 2 + 4 = 6
- cf for 9 = 6 + 6 = 12
- cf for 11 = 12 + 8 = 20
- cf for 13 = 20 + 10 = 30
- cf for 15 = 30 + 12 = 42
- cf for 17 = 42 + 8 = 50
|----|----|----|
| 5 | 2 | 2 |
| 7 | 4 | 6 |
| 9 | 6 | 12 |
| 11 | 8 | 20 |
| 13 | 10 | 30 |
| 15 | 12 | 42 |
| 17 | 8 | 50 |
To find the median, we need to calculate n/2:
- n/2=50/2=25
The formula for mean deviation about the median is given by:
Now we calculate |Xi−M| for each observation:
|----|----|---------|-----------------|
| 5 | 2 | 8 | 16 |
| 7 | 4 | 6 | 24 |
| 9 | 6 | 4 | 24 |
| 11 | 8 | 2 | 16 |
| 13 | 10 | 0 | 0 |
| 15 | 12 | 2 | 24 |
| 17 | 8 | 4 | 32 |
Now we sum the last column:
Now we can substitute into the mean deviation formula:
The mean deviation about the median is:
Topper's Solved these Questions
Similar Questions
Find the mean deviation about the median for the following frequency distribution.
 View Solution
View SolutionCalculate the mean deviation from the mean for the following frequency distributions.
 View Solution
View SolutionCalculate the mean deviation from the mean for the following frequency distributions.
 View Solution
View SolutionCalculate the mean deviation from the mean for the following frequency distributions.
 View Solution
View SolutionCalculate the mean deviation from the mean for the following frequency distributions.
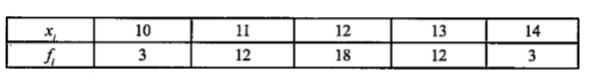 View Solution
View SolutionFind the mean deviation from the median for following frequency distribution :
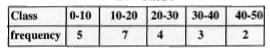 View Solution
View SolutionFind the mean deviation about median for the following continuous frequency distribution data
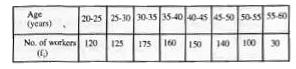 View Solution
View SolutionCalculate the mean deviation from the median of the following frequency distribution:5 7 9 11 13 15 17 2 4 6 8 10 12 8
View SolutionFind the mean deviation about the median for the following data:4,6,9,3,10,13,2
View Solutionmean deviation about median for the following data 2,5,7,2,4,6,10,12,5
View Solution

