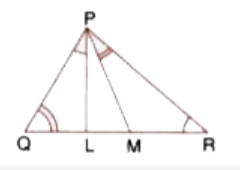
In a triangle PQR, L and M are two points on the base QR, such that ∠LPQ=∠QRP and ∠RPM=∠RQP. Prove that:
PQ2=QR×QL
To prove that PQ2=QR×QL in triangle PQR with points L and M on base QR, we can follow these steps:
Step 1: Draw Triangle PQR
Draw triangle PQR with points L and M on the base QR.
Step 2: Identify Given Angles
We are given that:
- ∠LPQ=∠QRP
- ∠RPM=∠RQP
Step 3: Establish Similarity of Triangles
From the given angles, we can see that:
- ∠LPQ=∠QRP (given)
- ∠Q is common to both triangles PQL and RQP.
Thus, by the Angle-Angle (AA) similarity criterion, we can conclude that:
△PQL∼△RQP
Step 4: Set Up Proportions
Since the triangles are similar, their corresponding sides are in proportion:
PQRQ=QLQP=PLPR
Step 5: Rearrange Proportions
From the proportion PQRQ=QLQP, we can cross-multiply to get:
PQ⋅QP=QL⋅RQ
Step 6: Substitute for QP
Note that QP is the same as PQ since they represent the same length. Thus, we can rewrite the equation as:
PQ2=QR⋅QL
Conclusion
This completes the proof, showing that:
PQ2=QR⋅QL
Draw triangle PQR with points L and M on the base QR.
We are given that:
- ∠LPQ=∠QRP
- ∠RPM=∠RQP
From the given angles, we can see that:
- ∠LPQ=∠QRP (given)
- ∠Q is common to both triangles PQL and RQP.
△PQL∼△RQP
Since the triangles are similar, their corresponding sides are in proportion:
PQRQ=QLQP=PLPR
From the proportion PQRQ=QLQP, we can cross-multiply to get:
PQ⋅QP=QL⋅RQ
Note that QP is the same as PQ since they represent the same length. Thus, we can rewrite the equation as:
PQ2=QR⋅QL
This completes the proof, showing that:
PQ2=QR⋅QL
Topper's Solved these Questions
- MIXED PRACTICE BOOK - ICSECHAPTER - MIXED PRACTICE EXERCISE - SET B
52 Videos
- MEASURES OF CENTRAL TENDENCY (MEAN, MEDIAN, QUARTILES AND MODE)BOOK - ICSECHAPTER - MEASURES OF CENTRAL TENDENCY (MEAN, MEDIAN, QUARTILES AND MODE)EXERCISE - EXERCISE 24 (E)
23 Videos
- PROBABILITYBOOK - ICSECHAPTER - PROBABILITYEXERCISE - EXERCISE 25(C)
106 Videos
Similar Questions
In a triangle PQR, L and M are two points on the base QR, such that ∠LPQ=∠QRP and ∠RPM=∠RQP. Prove that:
QL×RM=PL×PM
View SolutionIn a triangle PQR, L and M are two points on the base QR, such that ∠LPQ=∠QRP and ∠RPM=∠RQP. Prove that:
ΔPQL~ΔRPM
View SolutionIn a triangle PQR, L and M are two points on the base QR, such that ∠LPQ=∠QRP and ∠RPM=∠RQP. Prove that:
PQ2=QR×QL
View SolutionIn a triangle PQR, L and M are two points on the base QR, such that ∠LPQ=∠QRP and ∠RPM=∠RQP. Prove that:
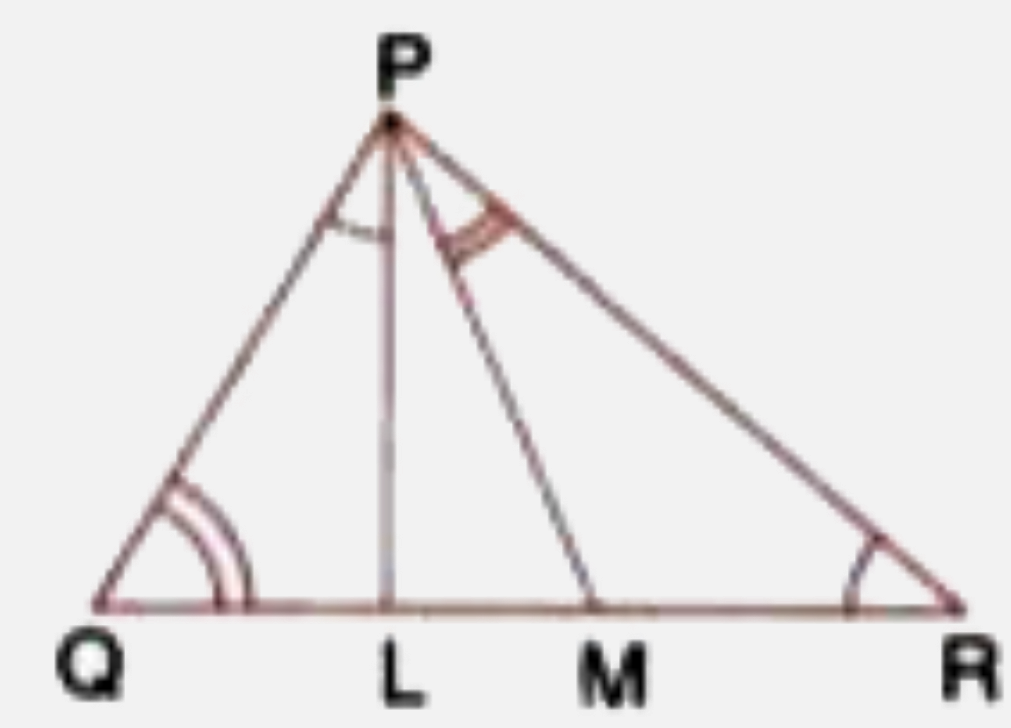
ΔPQL~ΔRPM
View SolutionIn a triangle PQR, L and M are two points on the base QR, such that ∠LPQ=∠QRP and ∠RPM=∠RQP. Prove that:

QL×RM=PL×PM
View SolutionIn the given figure, ∠SPR=∠QRP and ∠RSP=∠PQR. Prove that :
(i) PQ=RS
(ii) PS=QR

View SolutionIn quadrilateral PQRS , PS = QR and ∠SPQ=∠RQP . Prove that :
∠QPR=∠PQS
View SolutionThe sides PQ, PR of triangle PQR are equal, and S, T are points on PR, PQ such that ∠PSQand∠PTR are right angles
If OS and RT intersect at M, prove that the triangles PTM and PSM are congruent.
View SolutionABCD is a square. P, Q and Rare the points on AB, BC and CD respectively, such that AP = BQ = CR. Prove that:
PQ = QR
View SolutionM and N are mid point on sides QR and PQ respectively of △PQR, right-anggled at Q. Prove that :
4PM2=4PQ2+QR2.
View Solution
