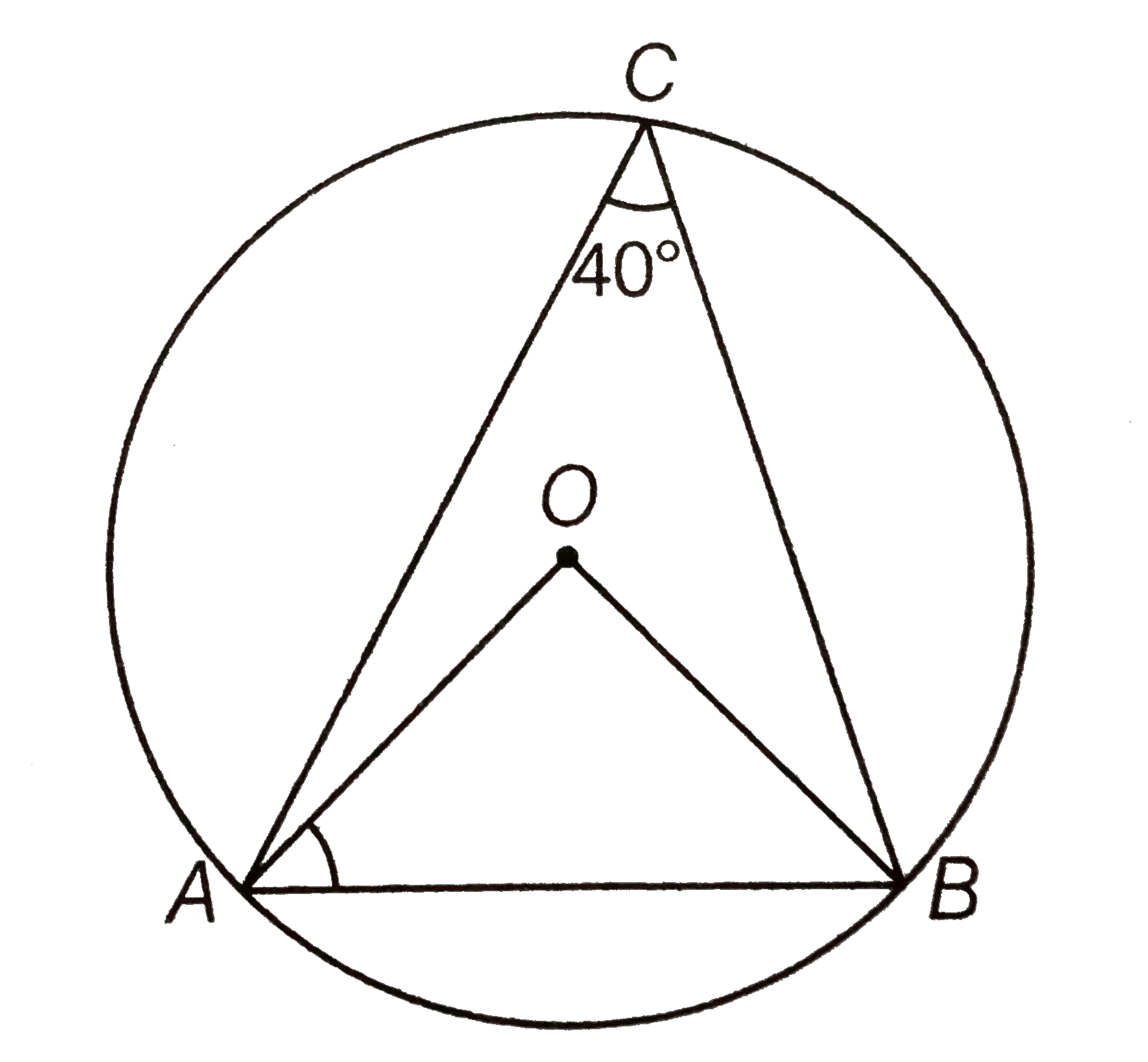
In figure, ∠OAB=30∘and∠OCB=57∘. Find ∠BOCand∠AOC.
Video Solution
Text Solution
Given,∠OAB=30∘and∠OCB=57∘
In ΔAOB,AO=OB [both are the radius of a circle]
⇒∠OBA=∠BAO=30∘
[angles opposite to equal sides are equal]
InΔAOB,
⇒∠AOB+∠OBA+∠BAO=180∘ [by angle sum property of a triangle]
∴∠AOB+30∘+30∘=180∘
∴∠AOB=180∘−2(30∘)
=180∘−60∘=120∘ ...(i)
Now, in ΔOCB,
OC=OB [both are the radius of a circle]
⇒∠OBC=∠OCB=57∘
[angle opposite to equal sides are equal]
In ΔOCB,
∠COB+∠OCB+∠CBO=180∘ [by angle sum property of triangle]
∴∠COB=180∘−(∠OCB+∠OBC)
=180∘−(57∘+57∘)
=180∘−114∘=66∘ ...(ii)
Form Eq. (i), ∠AOB=120∘
⇒∠AOC+∠COB=120∘
⇒∠AOC+66∘=120∘ [from Eq. (ii)]
∴∠AOC=120∘−66∘=54∘
|
Topper's Solved these Questions
- CIRCLESBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - CIRCLESEXERCISE - Exercise 10.4
14 Videos
- CIRCLESBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - CIRCLESEXERCISE - Exercise 10.2
10 Videos
- Areas of Parallelograms and TrianglesBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - Areas of Parallelograms and TrianglesEXERCISE - Exercise-9.4 Long Answer Type Questions
10 Videos
- CONSTRUCTIONSBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - CONSTRUCTIONSEXERCISE - Long Answer Type Questions
5 Videos
Similar Questions
Knowledge Check
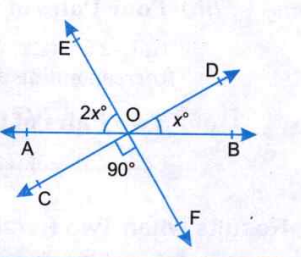
In the given figure, the lines AB, CD and EF intersect at a point O. If ∠BOD=x∘,∠AOE=2x∘and∠COF=90∘, find ∠AOEand∠AOC
 A30∘B40∘C55∘D70∘
A30∘B40∘C55∘D70∘
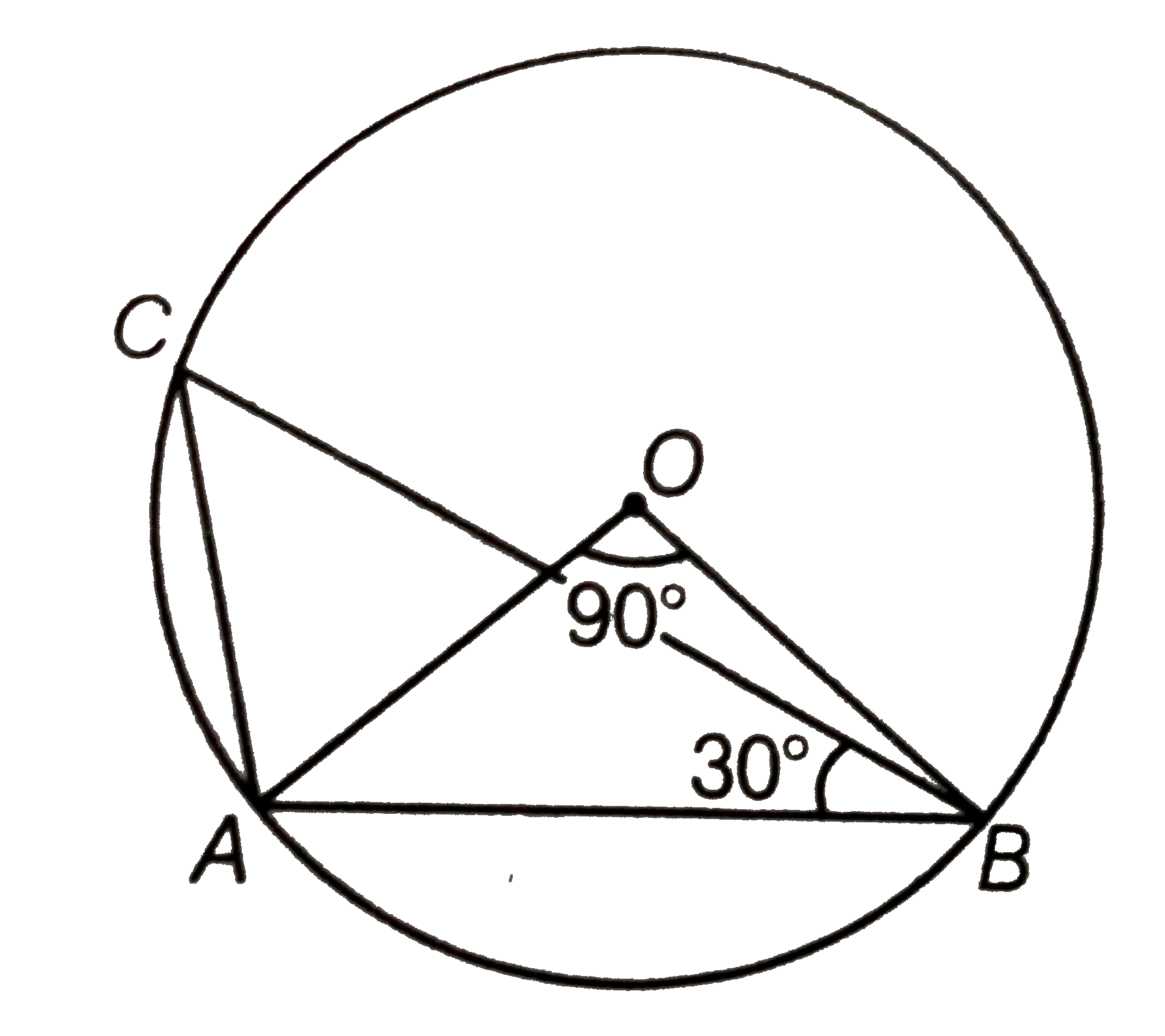
In figure, if ∠AOB=90∘and∠ABC=30∘, then∠CAO is equal to
View SolutionIn figure, if ∠OAB=40∘,then∠ACB is equal to
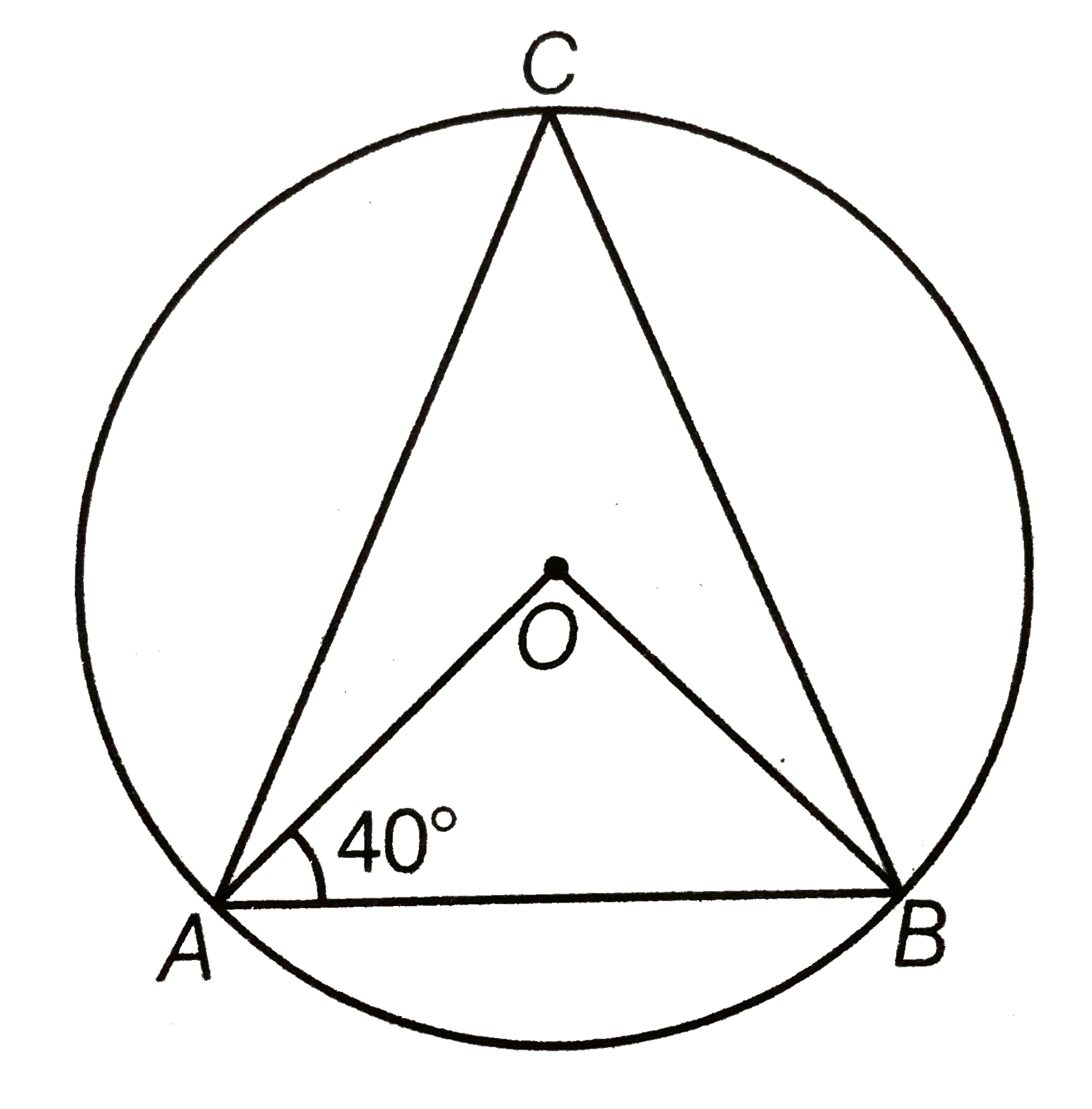
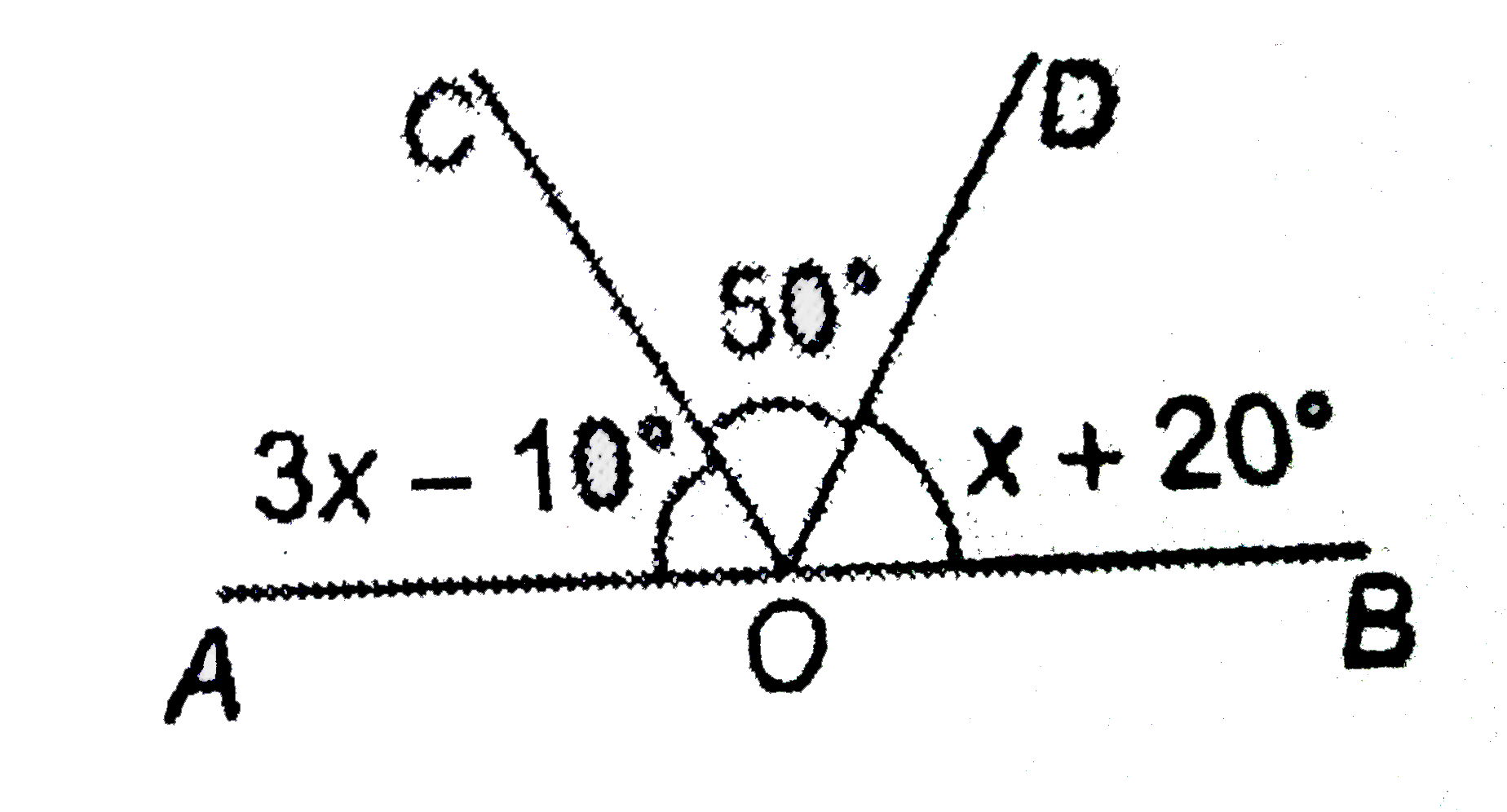
View SolutionIn the given firgue, AOB is a straight line if ∠AOC=(3x−10)∘∠COD=50∘and∠BOd=(x+80)∘ find ∠AOC
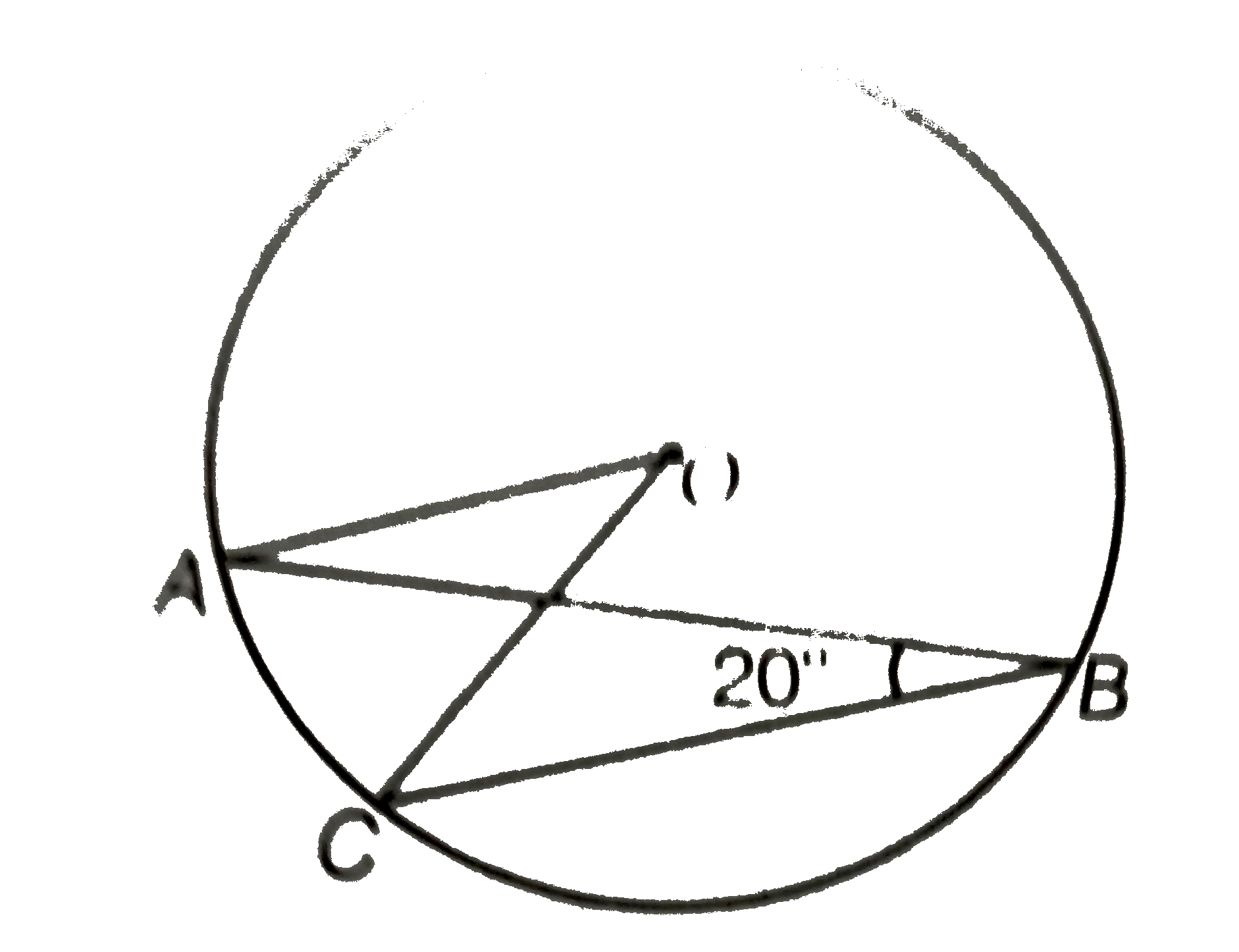
View SolutionIf figure, if∠ABC=20∘,then∠AOC is equal to
View SolutionIn the given figure, AB = BC = DC and ∠AOB=50∘
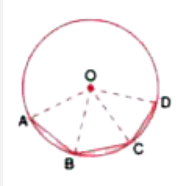
∠AOC =?
View SolutionIn the adjoining figure, AB||CD. If the ∠BAE=25∘and∠CDE=30∘, then find ∠AED
View SolutionIn the adjoining figure, O is the centre of the circle. If ∠AOB=150∘ and ∠BOC=100∘, then find the value of ∠ABC.

View Solution