In Figure, if find
To solve the problem, we need to find the angle ∠XMY given that PQ∥RS, ∠MXQ=135∘, and ∠MYR=40∘.
1. Identify the Given Angles and Parallel Lines:
- We have PQ∥RS.
- ∠MXQ=135∘.
- ∠MYR=40∘.
2. Draw a Parallel Line:
- To facilitate the calculations, we can draw a line TZ parallel to both PQ and RS.
3. Label the Angles:
- Let ∠XMZ=1 and ∠ZMY=2.
4. Use the Transversal:
- Since PQ∥TZ and MX is a transversal, we can use the property of interior angles on the same side of the transversal.
- Thus, ∠QXM+∠1=180∘.
5. Substitute the Known Angle:
- We know ∠QXM=135∘.
- Therefore, we can write:
135∘+∠1=180∘
6. Solve for Angle 1:
- Rearranging gives:
∠1=180∘−135∘=45∘
7. Use the Other Parallel Line:
- Now consider the line RS∥TZ with MY as the transversal.
- Here, ∠2 and ∠MYR are alternate interior angles.
- Therefore, ∠2=∠MYR=40∘.
8. Find Angle XMY:
- Now we can find ∠XMY by adding ∠1 and ∠2:
∠XMY=∠1+∠2=45∘+40∘=85∘
Final Answer:
∠XMY=85∘
- We have PQ∥RS.
- ∠MXQ=135∘.
- ∠MYR=40∘.
- To facilitate the calculations, we can draw a line TZ parallel to both PQ and RS.
- Let ∠XMZ=1 and ∠ZMY=2.
- Since PQ∥TZ and MX is a transversal, we can use the property of interior angles on the same side of the transversal.
- Thus, ∠QXM+∠1=180∘.
- We know ∠QXM=135∘.
- Therefore, we can write:
135∘+∠1=180∘
- Rearranging gives:
∠1=180∘−135∘=45∘
- Now consider the line RS∥TZ with MY as the transversal.
- Here, ∠2 and ∠MYR are alternate interior angles.
- Therefore, ∠2=∠MYR=40∘.
- Now we can find ∠XMY by adding ∠1 and ∠2:
∠XMY=∠1+∠2=45∘+40∘=85∘
∠XMY=85∘
Topper's Solved these Questions
Similar Questions
In Fig. 6.24, if PQ∣∣RS,∠MXQ=135∘ and∠MYR=40∘,find ∠XMY.
View SolutionIn Figure, if QT⊥PR, ∠TQR=40∘ and ∠SPR=30∘, find x and y.
View SolutionIn Fig. 6.31, if PQ∣∣ST,∠PQR=110∘ and∠RST=130∘, find ∠QRS.
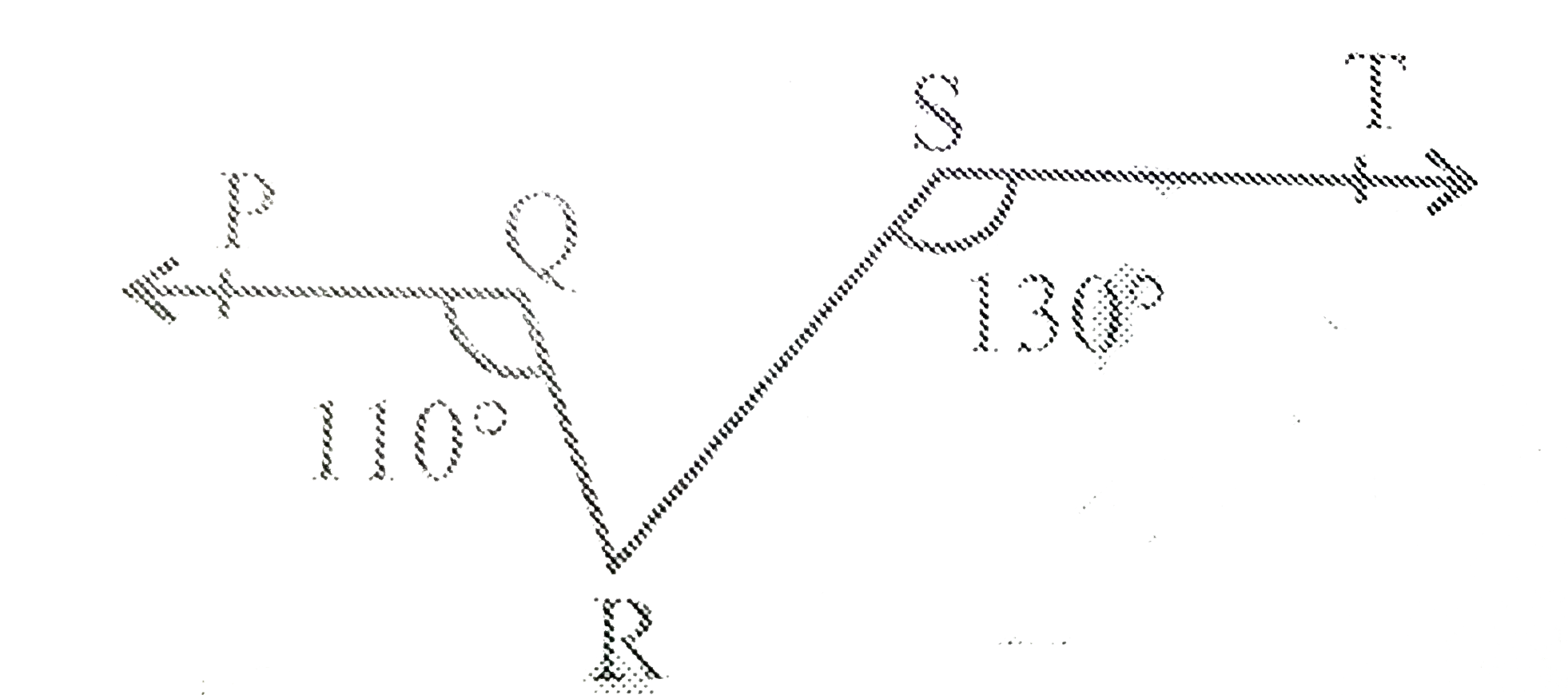 View Solution
View SolutionIn Figure, if AB∣∣CD, ∠APQ=500AND ∠PRD=1270, find x and y
View SolutionIn Figure, if PQ⊥PS, PQSR, ∠SQR=280and ∠QRT=650, then find the values of x and y
View SolutionIn Fig. 6.37, if QT⊥PR,∠TQR=40∘and∠SPR=30∘, find x and y.
View SolutionIn figure, if PQ⊥PS,PQ∣∣SR,∠SQR=280 and ∠QRT=650, then find the values of x and y. Figure
View SolutionIn Figure, if lines PQ and RS intersect at a point T such that ∠PRT=40∘, ∠RPT=95∘and ∠TSQ=75∘, find ∠SQT.
View SolutionIn Figure, side QP and RQ of PQR are produced to point S and T respectively. If ∠SPR=1350and ∠PQT=110∘, find ∠PRQ
View SolutionIf Figure, if QT⊥PR,∠TQR=400 AND ∠SPR=300, find x and y. Figure
View Solution

