In figure, △QRS is an equilateral triangles. Prove that,
(1) arcRS≅arcQS≅arcQR
(2) m(arcQRS)=240∘.
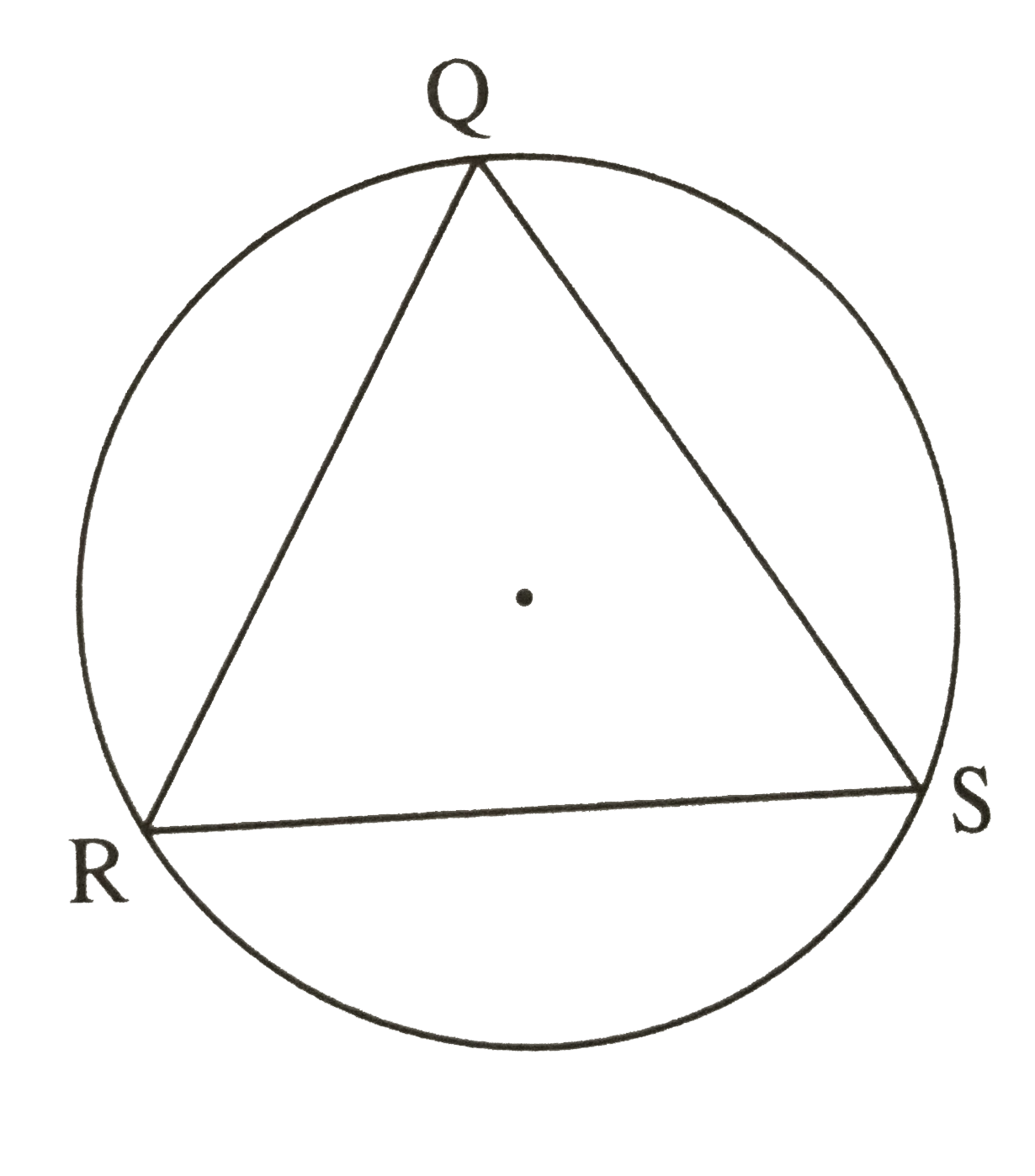
△QRS is an equilateral triangle ……..( Given )
∴SegRS≅segQS≅segQR ……( Sides of an equilateral triangle )
Arcs of the same circle are equal, if the related chords are congruent.
∴arcRS≅arcQS≅arcQR.
Let m(arcRS)=m(arcQS)=m(arcQR)=.......(1)
m(arcRS)+m(arcQS)+m(arcQR)=360∘ .....( Measure of a circle is 360∘)
∴x+x+x=360∘ .....[ From (1) ]
∴3x=360∘
∴x=3603
∴x=120∘
∴m(arcRS)=m(arcQS)=m(arcQR)=120∘
m(arcQRS)=m(arcQR)+m(arcRS) ......( Arc addition postulate )
∴m(arcQRS)=120∘+120∘
∴m(arcQRS)=240∘.
Topper's Solved these Questions
- BOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - CIRCLEEXERCISE - PRACTICE SET 3.415videos
CIRCLE
- BOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - CIRCLEEXERCISE - PRACTICE SET 3.56videos
CIRCLE
- BOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - CIRCLEEXERCISE - PRACTICE SET 3.28videos
CIRCLE
- BOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - CHALLENGING QUESTIONSEXERCISE - SECTION 3 (MODEL QUESTION PAPER FOR PRACTICE ) Solve any one of the following subquestions :1videos
CHALLENGING QUESTIONS
- BOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - COORDINATE GEOMETRY EXERCISE - Assignment 6.513videos
COORDINATE GEOMETRY
Similar Questions
In the figure , ΔQRS is an equilateral triangle.
Prove that
(1) arc RS ≅arcQS≅ arc QR
(2) m (arc QRS ) =240∘.
Proof :
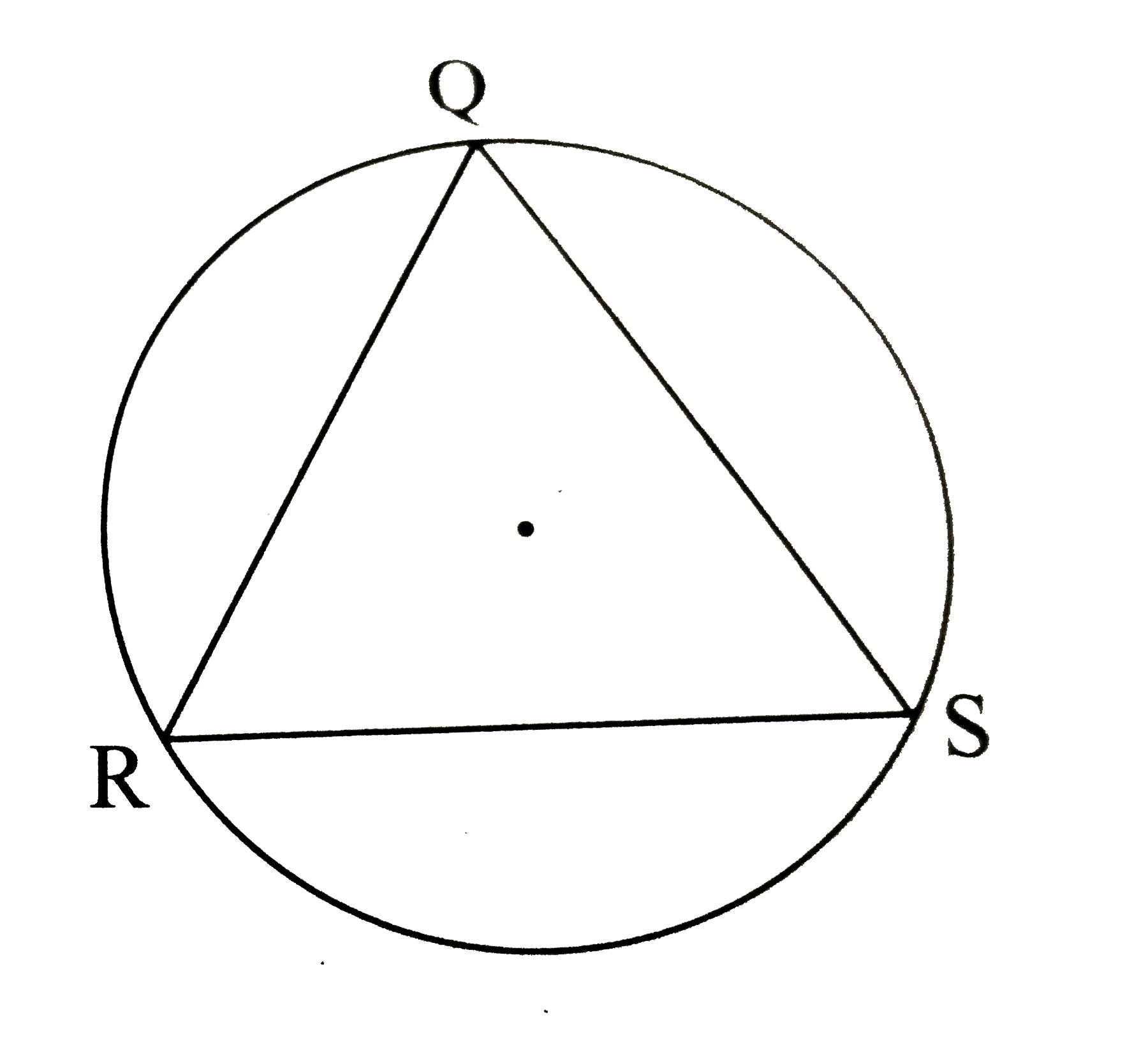 View Solution
View Solution1. In the figure △PQR is an equilateral triangle, the point N is on ray QR such that QR=RN. Prove that PN2=3PR2
View SolutionIn the adjoining figure, ΔPQR is an equilateral triangle. Point S is on seg QR. such that QS=13QR. Prove that : 9PS2=7PQ2.
View SolutionIn the figure ΔPQR is an equilateral triangle. Point S is on seg QR such that
QS=13QR.
Prove that : 9PS2=7PQ2
 View Solution
View SolutionIn the given figure, I Rs = 3√3 cm and RPS is an equilateral triangle, then find the value of QR
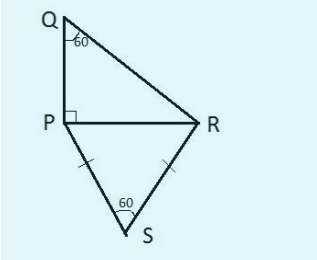 View Solution
View SolutionIn figure, chord AB ≅ chord CD, Prove that, arc AC ≅ arc BD.
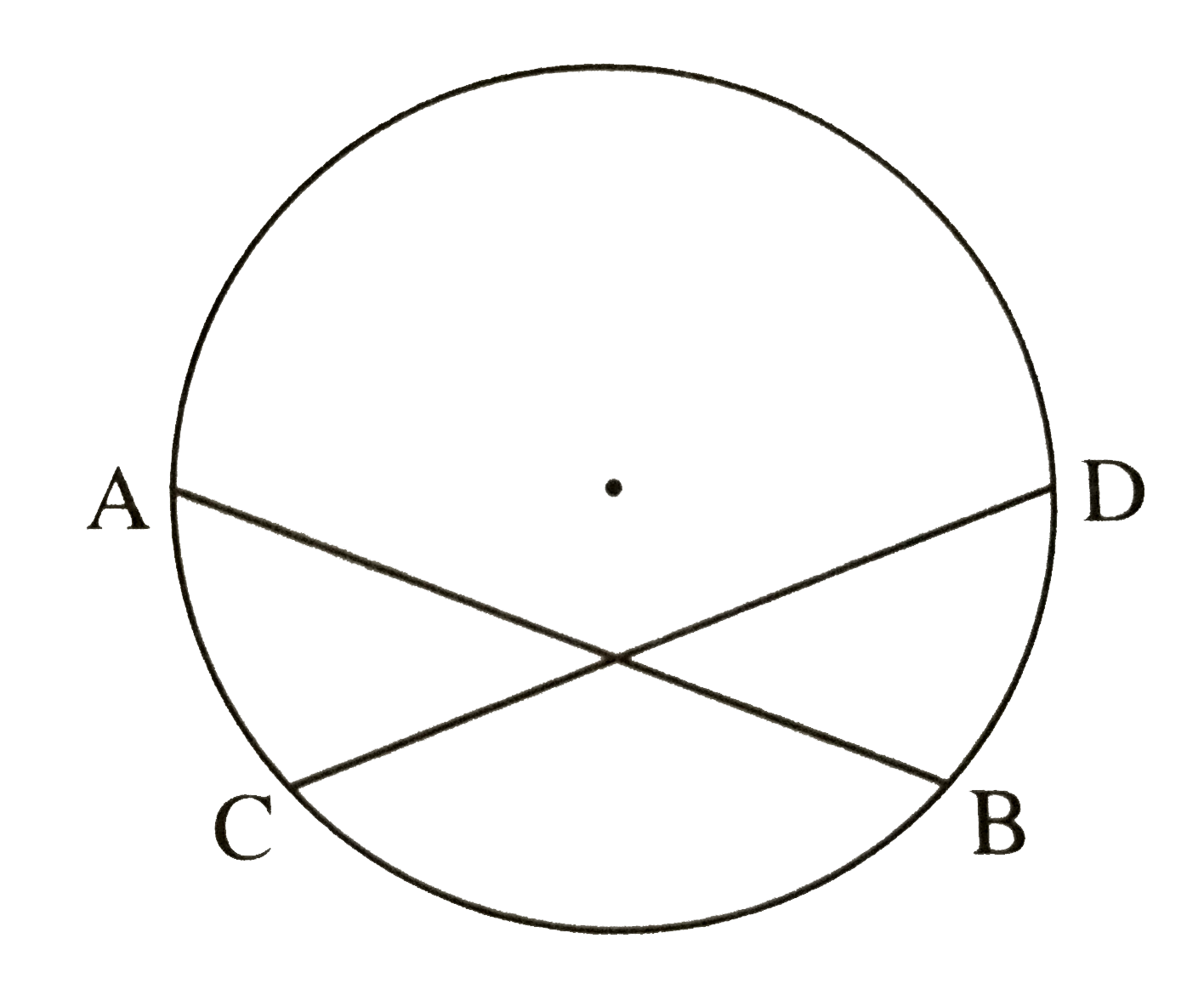 View Solution
View SolutionIn the figure 2.35,ΔPQR is an equilatral triangle. Point S is on seg QR such that QS=13QR. Prove that : 9PS2=7PQ2
View SolutionIn the figure, line RS is tangent at point Q. If ∠PQS=65∘ find m(arc PMQ) and m(arc PNQ ).
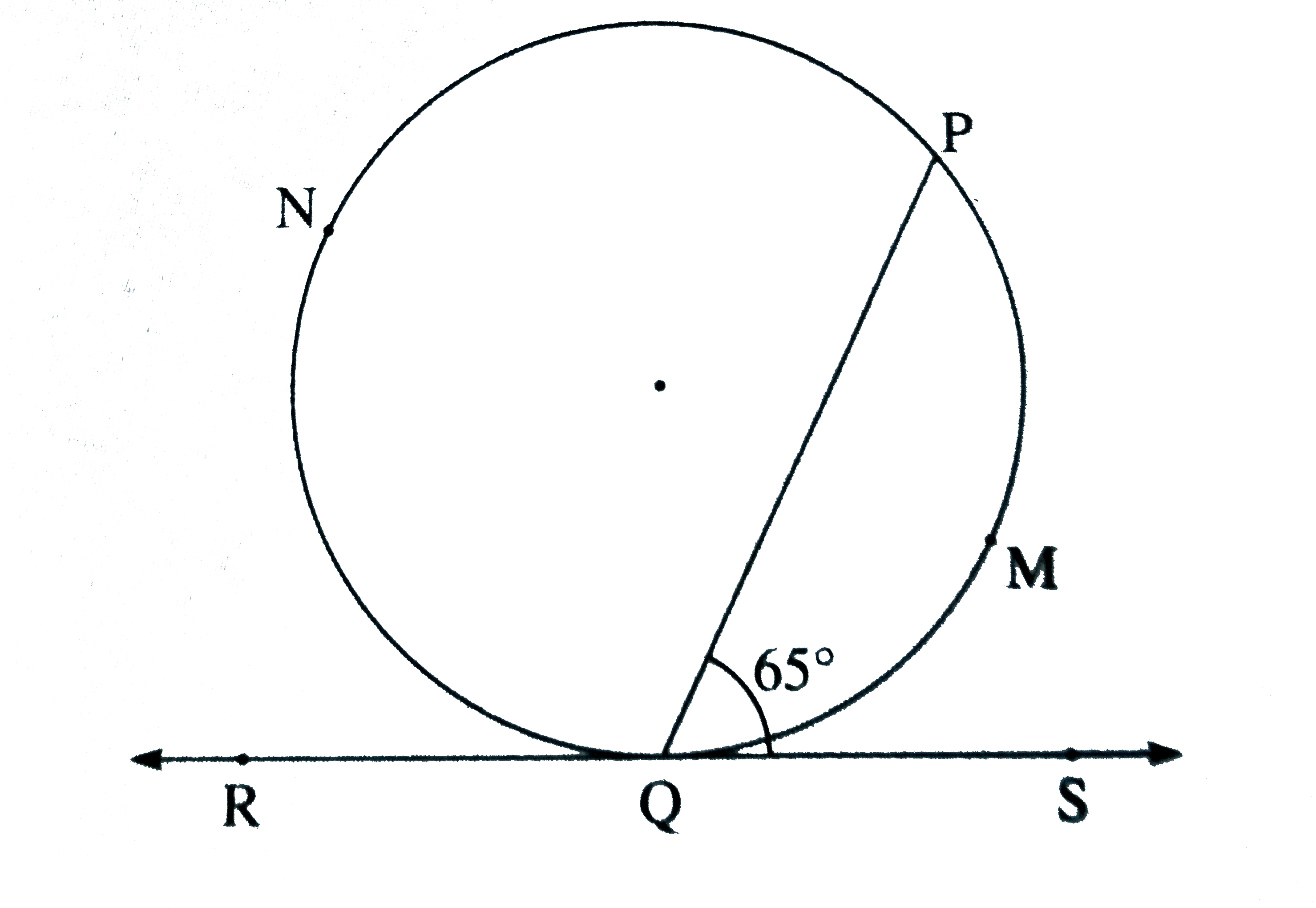 View Solution
View SolutionThe adjoining figure shows a square ABCD and an equilateral triangle DEC. Prove that :
(i) ∠ADE=∠BCE=30∘
(ii) Δ≅ΔBCE
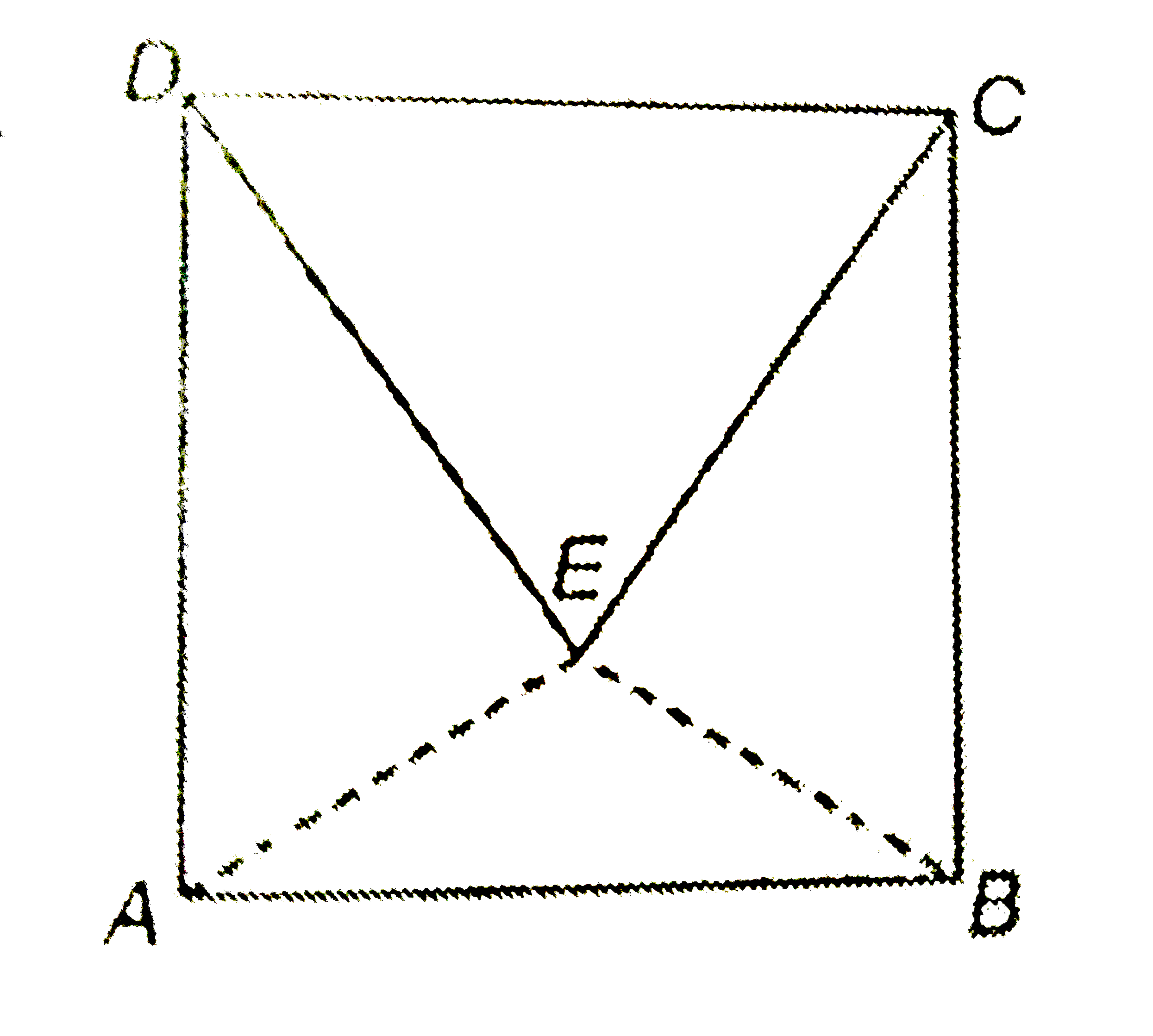 View Solution
View SolutionIn the given figure, ABC is an equilateral triangle; PQ∣∣AC and AC is produced to R such that CR=BP. Prove that QR bisects PC
View Solution

