In the given figure, AB is a diameter of a circle with centre O and CD||BA. If ∠BAC=20∘ , find
(i) ∠BOC (ii) ∠COD
(iii) ∠CAD and (iv)∠ADC
Video Solution
Text Solution
(i) Arc BC subtends ∠BOC at the centre an ∠BAC at a point on the remaining part of the circumstances
∴∠OC=2×∠ABC=2×20∘=40∘
(ii) CD||BA and OC cuts them.
∴∠OCD=∠BOC=40∘ [alt. int. ∠s ] .
∴∠ODC=∠OCD=40∘ [:'∠OC=OD=radius ].
Now, in ΔOCD, we have
∠COD+∠OCD+∠ODC=180∘
⇒∠COD+40∘+40∘=180∘ [∵∠OCD=∠ODC=40∘]
⇒∠COD=180∘−80∘=100∘
(iii) Since the angle at the centre is double the angle at a point on the remaining part of the circumference, we have
∠CAD=12∠COD=(12×100∘)=50∘
(iv) Since BA∣∣CD and AD cuts them, we have
∠ACD=∠CAB=20∘ [alt. int. ∠s].
In ΔACD, we have
∠CAD+∠ACD+∠ADC=180∘
⇒50∘+20∘+∠ADC=180∘
⇒∠ADC=180∘−70∘=110∘
|
Topper's Solved these Questions
- CIRCLES BOOK - RS AGGARWALCHAPTER - CIRCLES EXERCISE - Exercise 12A
22 Videos
- CIRCLES BOOK - RS AGGARWALCHAPTER - CIRCLES EXERCISE - EXERCISE 12B
18 Videos
- BAR GRAPH, HISTOGRAM AND FREQUENCY POLYGONBOOK - RS AGGARWALCHAPTER - BAR GRAPH, HISTOGRAM AND FREQUENCY POLYGONEXERCISE - EXERCISE 17B
16 Videos
- CONGRUENCE OF TRIANGLES AND INEQUALITIES IN A TRIANGLEBOOK - RS AGGARWALCHAPTER - CONGRUENCE OF TRIANGLES AND INEQUALITIES IN A TRIANGLEEXERCISE - Multiple Choice Questions (Mcq)
20 Videos
Similar Questions
Knowledge Check
In the given figure, BOD is the diameter of the circle with centre O. ∠COD=92∘and∠ABD=65∘. Then y equals
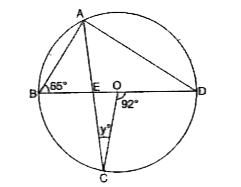 A65∘B46∘C44∘D21∘
A65∘B46∘C44∘D21∘AB is a diameter of the circle with centre O, CD is chord of the circle. If ∠BOC=120∘, then the value of ∠ADC is
A42∘B30∘C60∘D35∘
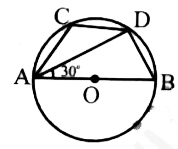
In the given figure, AOB is a diameter of a circle and CD||AB. If ∠BAD=30∘, then ∠CAD = ?
View SolutionIn the figure given below, AD is a diameter of a circle with centre O.If AB ll CD, prove that AB=CD
View Solution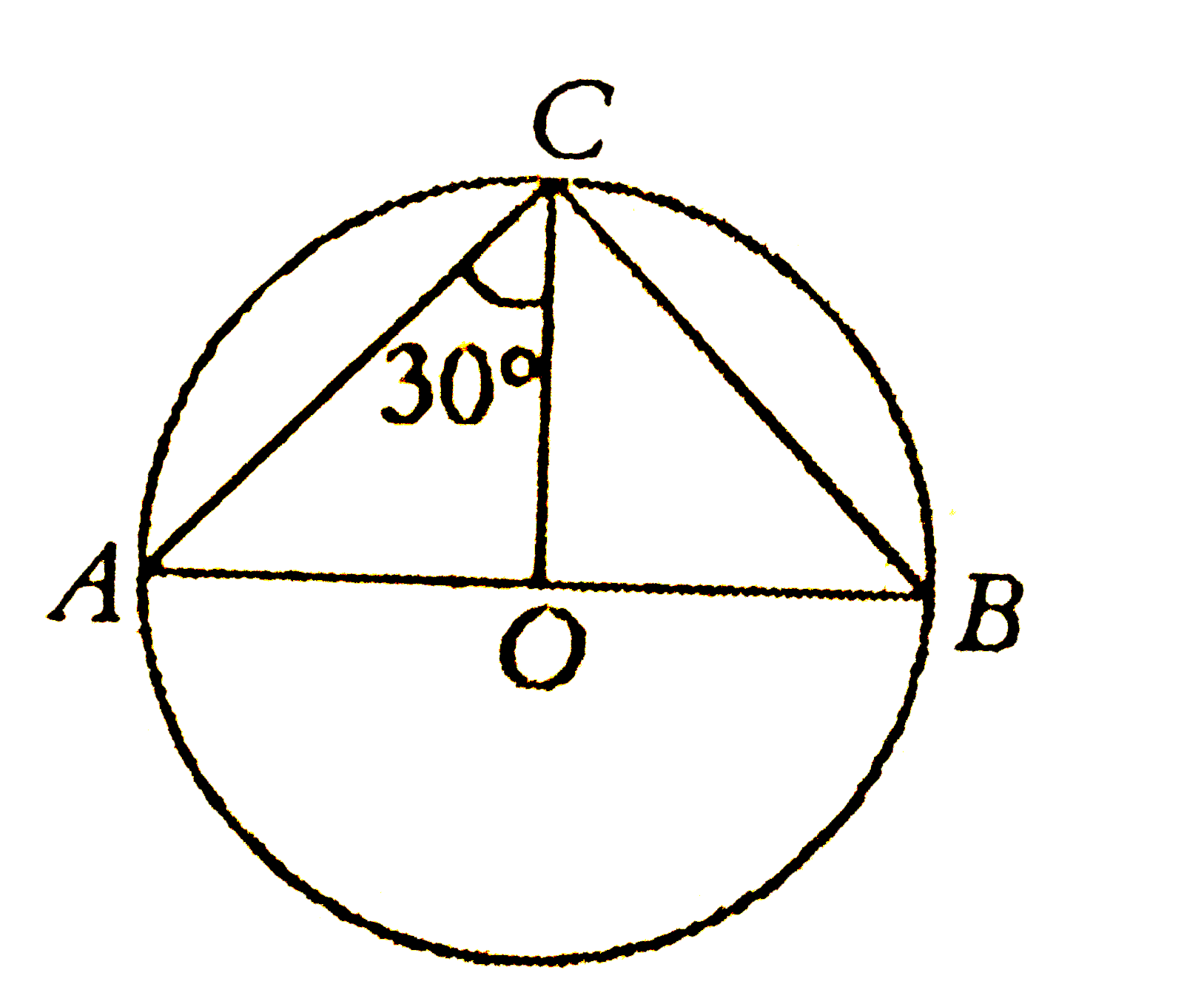
In the figure above (not to scale), AB is the diameter of the circle with centre O. If ∠ACO=30∘, then find ∠BOC.
View SolutionAB and CD are diameters of a circle with centre O. If ∠OBD=50∘, find ∠AOC
View SolutionIn the figure, AB is a diameter of the circle with centre O and OA = 7cm. Find the area of the shaded region
View SolutionAB and CD are diameters of a circle with centre O. If ∠OBD=50∘ then find ∠AOC
View Solution
