Prove that two different circles cannot intersect each other at more than two points.
Video Solution
Text Solution
If possible, let two different circles intersect at three distinct points A,B,C. Then, these points are clearly noncollinear . So, a unique circle can be drawn to pass through these points.This is a contradiction.
|
Topper's Solved these Questions
- CIRCLES BOOK - RS AGGARWALCHAPTER - CIRCLES EXERCISE - EXERCISE 12B
18 Videos
- CIRCLES BOOK - RS AGGARWALCHAPTER - CIRCLES EXERCISE - EXERCISE 12C
26 Videos
- CIRCLES BOOK - RS AGGARWALCHAPTER - CIRCLES EXERCISE - MULTIPLE CHOICE QUESTION(MCQ)
37 Videos
- BAR GRAPH, HISTOGRAM AND FREQUENCY POLYGONBOOK - RS AGGARWALCHAPTER - BAR GRAPH, HISTOGRAM AND FREQUENCY POLYGONEXERCISE - EXERCISE 17B
16 Videos
- CONGRUENCE OF TRIANGLES AND INEQUALITIES IN A TRIANGLEBOOK - RS AGGARWALCHAPTER - CONGRUENCE OF TRIANGLES AND INEQUALITIES IN A TRIANGLEEXERCISE - Multiple Choice Questions (Mcq)
20 Videos
Similar Questions
Knowledge Check
Two chords AB and CD of a circle with centre o intersect each other at the point P. If ∠AOD=20∘ and ∠BOC=30∘, then ∠BPCis equal to:
A50∘B20∘C25∘D30∘
Two indifference curves cannot intersect each other. Explain Why? Use diagram.
View SolutionCan two isothermal curves intersect each other?
View SolutionTwo gases cannot diffuse into each other.
View SolutionIf two lines are not intersect each other then the lines are.........
View SolutionIn figure, two circles intersect each other at points A and E. Their common secant through E intersects the circles at points B and D. The tangents of the circles at points B and D intersect each other at point C. Prove that □ ABCD is cyclic.
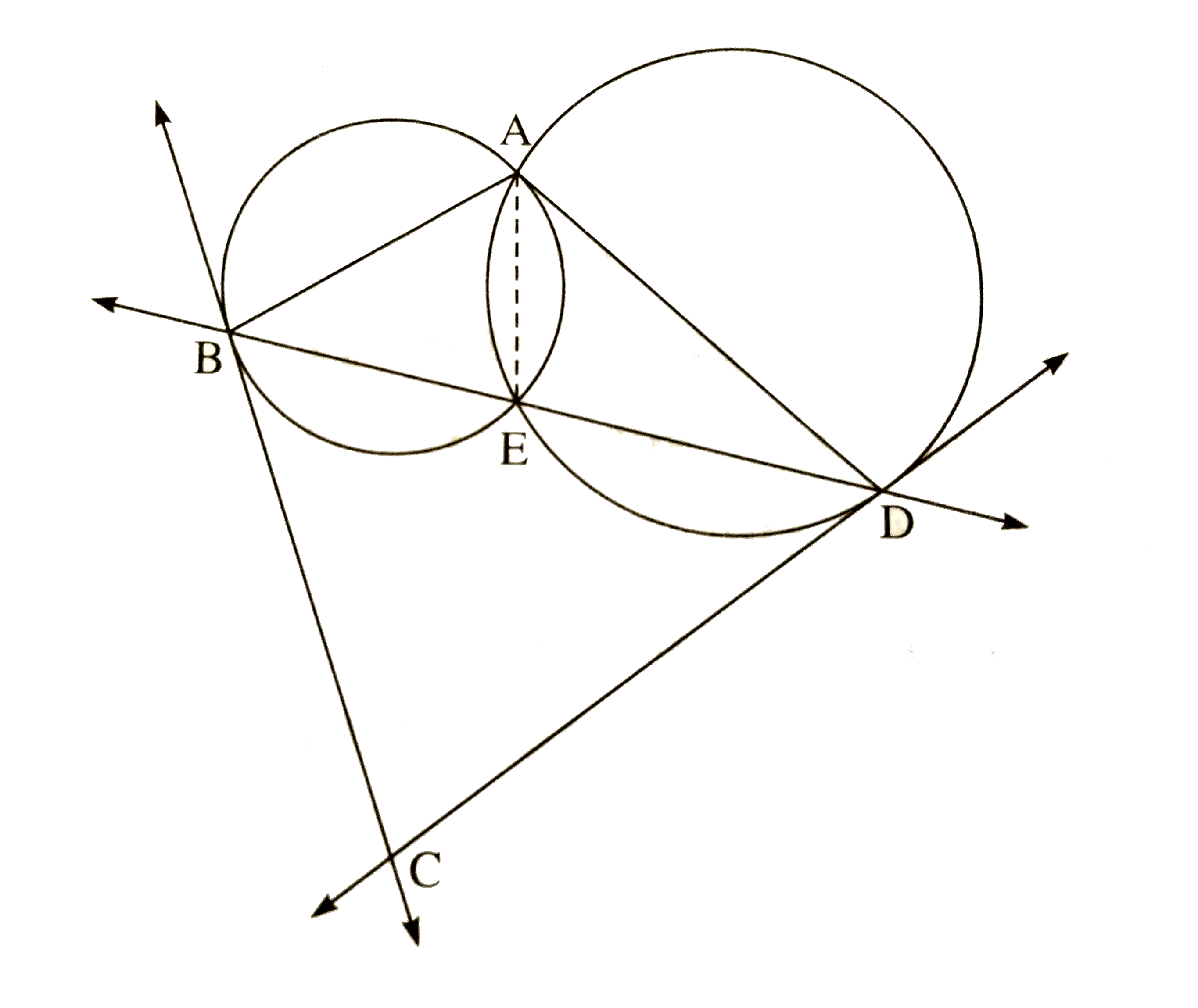
View SolutionProve that two distinct lines cannot have more that one point in common
View SolutionProve that if two lines intersect each other, then the vertically opposite angles are equal.
View Solution
