AB and AC are two chords of a circle of radius r such that AB=2AC. If p and q are the distances of AB and AC from the centre Prove that $4 q^{2} = p^{2} + 3 r^{2}$ .

Video Solution

Text Solution

Verified by Experts

Let $A C = x$ . Then, $A B = 2 x$ .
Let $O L ⊥ A B$ and $O M ⊥ A C$
The, $O L = p, O M = q$ and $O A = r$
Also, $A M = \frac{1}{2} A C = \frac{x}{2}$ and $A L = \frac{1}{2} A B = x$
Clearly, $r^{2} = p^{2} x^{2}$ ...(i) ltbr and $r^{2} = q^{2} + {(\frac{x}{2})}^{2}$ .......(ii)
$∴ q^{2} = r^{2} - \frac{x^{2}}{4} \Rightarrow 4 q^{2} = 4 r^{2} - x^{2}$
$\Rightarrow 4 q^{2} = 4 r^{2} - (r^{2} - p^{2})$ [ using (i) ]
$= 4 q^{2} = 3 r^{2} + p^{2}$
Hence, $4 q^{2} = 3 r^{2} + p^{2}$

|

Updated on:21/07/2023

Class 9 MATHS CIRCLES

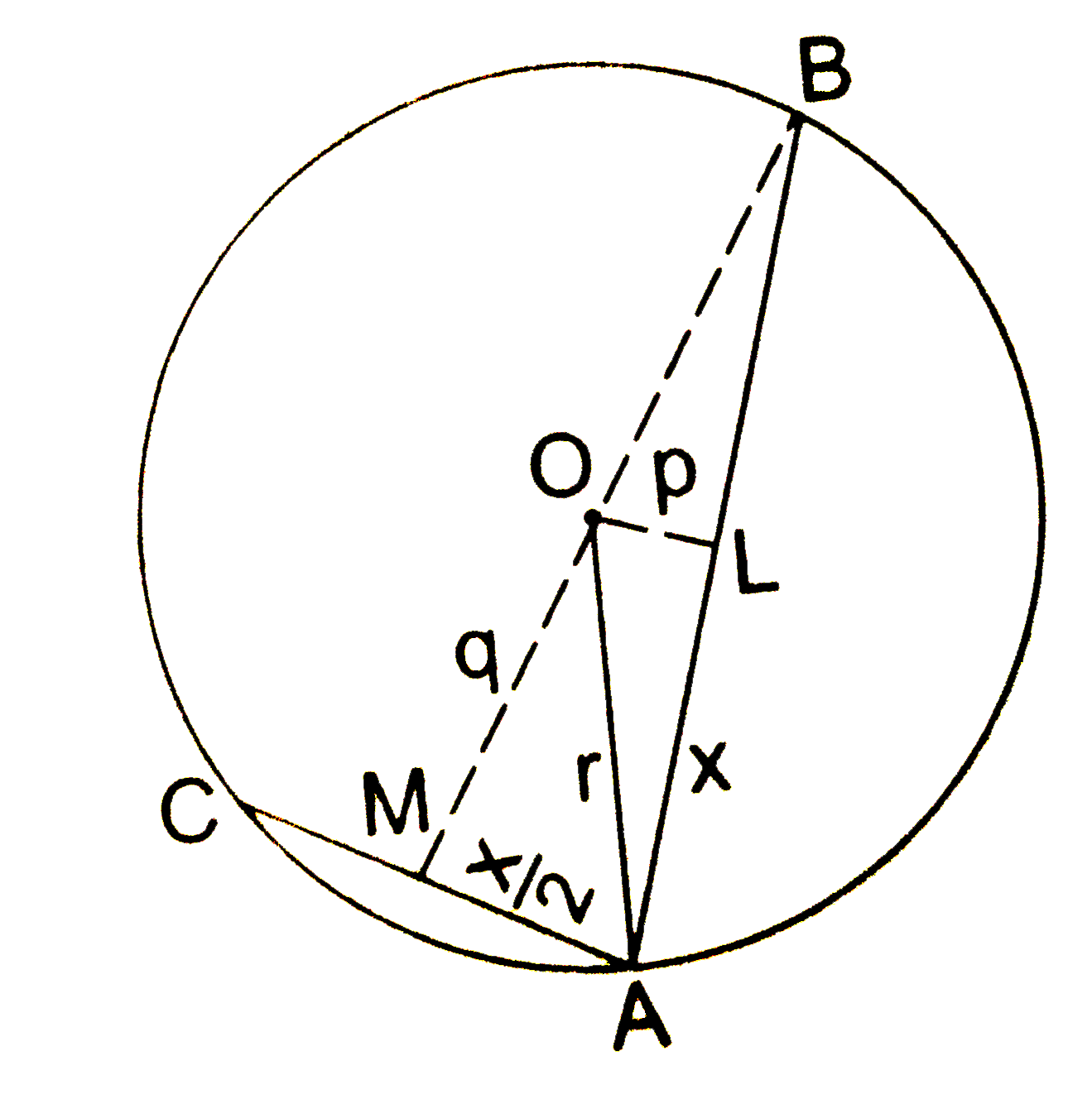
Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
There is a circle of radius 5 cm. There are two chords AB and AC OF equal length = 6 cm . Find the length of chord BC .
A4.8 cm
B10.8cm
C9.6 cm
Dnone of these

RS AGGARWAL-CIRCLES -Exercise 12A

Home
Ask Doubt
Profile