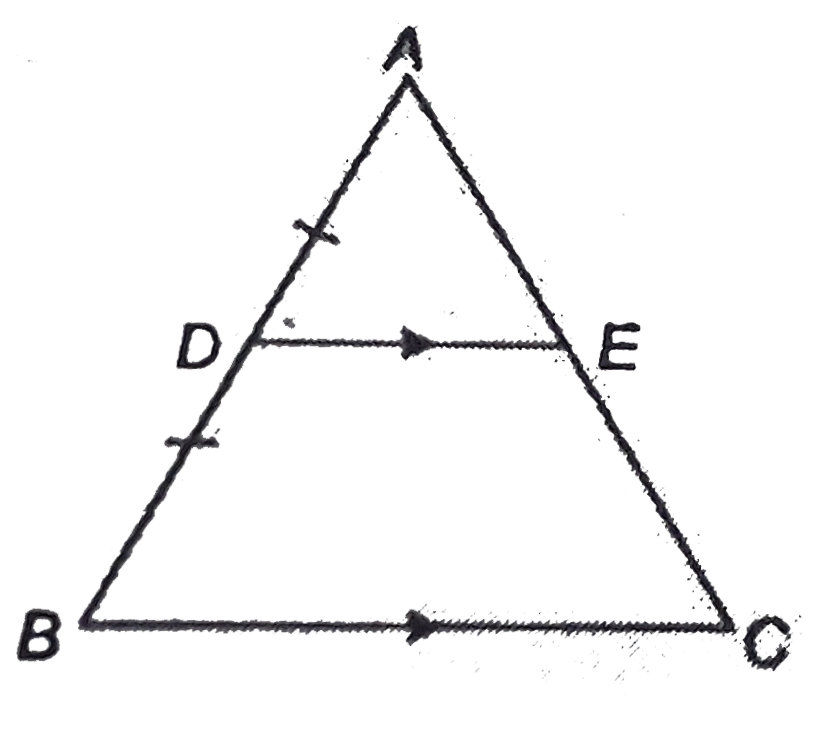
Using Theorem 6.1, prove that a line drawn through the mid-point of one side of a triangle parallel to another side bisects the third side. (Recall that you have proved it in Class IX).
To prove that a line drawn through the midpoint of one side of a triangle and parallel to another side bisects the third side, we will use Theorem 6.1.
1. Consider Triangle ABC: Let triangle ABC be given, where we have points A, B, and C.
2. Identify Midpoint: Let P be the midpoint of side AB. This means that AP = PB.
3. Draw a Parallel Line: Draw a line segment PQ through point P that is parallel to side BC.
4. Apply Theorem 6.1: According to Theorem 6.1, if a line is drawn parallel to one side of a triangle, it divides the other two sides proportionally. Thus, we can write the proportion:
AQQC=APPB
5. Substitute Midpoint Values: Since P is the midpoint of AB, we have:
AP=PB
Therefore, we can substitute this into our proportion:
AQQC=APAP=1
6. Conclude Equal Segments: Since the ratio AQQC=1, it implies that:
AQ=QC
This means that point Q is the midpoint of side AC.
7. Final Statement: Hence, we have proved that the line segment PQ, drawn through the midpoint P of side AB and parallel to side BC, bisects side AC at point Q.
AQQC=APPB
AP=PB
Therefore, we can substitute this into our proportion:
AQQC=APAP=1
AQ=QC
This means that point Q is the midpoint of side AC.
Topper's Solved these Questions
- TRIANGLESBOOK - NCERTCHAPTER - TRIANGLESEXERCISE - EXERCISE 6.6
10 Videos
- TRIANGLESBOOK - NCERTCHAPTER - TRIANGLESEXERCISE - EXERCISE 6.3
16 Videos
- TRIANGLESBOOK - NCERTCHAPTER - TRIANGLESEXERCISE - Exercise 6.1
3 Videos
- SURFACE AREAS AND VOLUMESBOOK - NCERTCHAPTER - SURFACE AREAS AND VOLUMESEXERCISE - EXERCISE 13.2
8 Videos
Similar Questions
The line drawn from the midpoint of one side of a triangle parallel to another side bisects the third side.
View SolutionTheorem 8.10 : The line drawn through the mid-point of one side of a triangle, parallel to another side bisects the third side.
View SolutionProve using similar triangles, that a line drawn through the mid-point of one side of a triangle parallel to another side, bisects the third side.
View SolutionThe line drawn through the mid-point of one side of a triangle, parallel to another side, intersects the third side at its mid-point.
View SolutionThe line drawn through the mid-point of one side of a triangle is parallel to another side and intersects the third side at its mid-point.
View SolutionThe line joining the mid-points of two sides of a triangle is parallel to the third side.
View SolutionUsing Theorem 6.2, prove that the line joining the mid-point of any two sides of a triangle is parallel to the third side. (Recall that you have done it in class IX).
View SolutionProve that the line joining the mid-points of the two sides of a triangle is parallel to the third side.
View Solutionprove that the line segment joining the mid-points of two sides of a triangle is parallel to the third side.
View SolutionProve that the line segment joining the mid points of two sides of a triangle is parallel to its third side.
View Solution
