Complete Solutions to Matching types problems of chapter WORK AND ENERGY of Class 11 book with complete answers and questions
| Class 11 | PHYSICS |
| Chapter | WORK AND ENERGY |
| ANURAG MISHRA | Matching types problems |
| Number Of Questions | 14 |
| Academic Year | 2023-24 |
Match the following :
`{:(,"Column-I",," Column-II"),("(A)","Work done by all the forces",,"(P)Change in potential energy"),("(B)","Work done by conservative forces",,"(Q) Change in kinetic energy"),("(C)","Work done by external forces",,"(R) Change in mechanical energy"),(,,,"(S) None"):}`
View SolutionA particle is suspended from a string of length R. It is given a velocity `u= 3 sqrt(gR)` at the bottom. Match the following:

`{:(,"Column-1",," Column-2"),("(A)","Velocity at B",,"(P) 7 mg"),("(B)","Velocity at C",,(Q)sqrt(5gR) ),("(C)","Tension in string at B",,(R)sqrt(7gR)),("(D)","Tension in string at C",,(S) 5 mg),(,,,"(T)None"):}`
View SolutionA force F=kx (where k is a positive constant) is acting on a particle Work done:
`{:(,"Column-1",," Column-2"),("(A)","in displacing the body from x=2 to x=4",,"(P) Negative"),("(B)","In displacing the body from x=-4 to x=-2",,"(Q) Positive"),("(C)","In displacing the body from x=-2 to x=+2",,"(R) Zero"):}`
View SolutionF-x and corresponding U-x graph are as shown in figure. Three points A,B and C in F-x graphs may be corresponding to P,Q and R in the U-x graph. Match the following

`{:(,"Column-1",," Column-2"),("(A)","A",,"(P) P"),("(B)","B",,"(Q) Q"),("(C)","C",,"(R) R"),(,,,"(S) None"):}`
View SolutionA body is moved along a straight line by a machine delivering a power proportional to time `(P prop t)` Then match the following :
`{:(,"Column-1",," Column-2"),("(A)","Velocity is proportional to",,"(P) t"),("(B)","Displacement proportional to",,(Q) t^(2)),("(C)","Work done proportional to",,(R) t^(3)):}`
View SolutionA pendulum is released from point A as shown in figure. At some instant net force on the bob is making and angle `theta` with the string. Then match the following:

`{:(,"Column-1",," Column-2"),("(A)",For theta=30^(@),,"(P) Particle may be moving between B & A"),("(B)",For theta = 120^(@),,"(Q) Particle may be moving between C & B particle is at A"),("(C)",For theta=90^(@),,"(R) Particle is at A"),("(D)",For theta=0^(@),,"(S) Particle is at B"),(,,,"(T) None"):}`
View SolutionMatch the following :
`{:(,"Column-1",," Column-2"),("(A)","Electrostatic potential energy",,"(P) Positive"),("(B)","Gravitational potential energy",,"(Q) Negative"),("(C)","Elastic potential energy",,"(R) Zero"),("(D)","Magnetic potential energy",,"(S) Not defined"):}`
View SolutionA particle of mass m kg is displaced from one given point to another given point under the action of several conservative and non conservative forces (Neglect relativistic considerations) Now match the following .
`{:(,"Column-1",," Column-2"),("(A)","Displacement of particle",,"(P) Path dependent"),("(B)","Work done conservative force",,"(Q) Path independent"),("(C)","Work done by non-conservative force",,"(R) Frame dependent"),("(D)","Angular displacement",,"(S) Frame independent"),(,,,"(T) Dependent on location of observer in a given frame"):}`
View SolutionIn the figure shown, upper block is given a velocity `6m//s` and very long plank, velocity `3m//s` The following quantities are to be matched when both attain same velocity.

`{:(,"Column-1",," Column-2"),("(A)","Work done by friction on 1 kg block in joule",,"(P) Positive"),("(B)","Work done by friction on 2 kg plank in joule",,"(Q) Negative"),("(C)","Magnitude of change in momentum in N-s of 2 kg plank",,"(R) 3"),("(D)","Change in KE of system consisting of block and plank in joule",,"(S) 7"),(,,,"(T) 2"):}`
View SolutionInitially spring are in natural length An application of external varying force F causes the block to move slowly distance x towards wall on smooth floor:

`{:(,"Column - 1",,"Column - 2",),((A),"Work done by " S_(2) "on block",(P),"zero",),((B),"Work done by "S_(2) and S_(1),(Q),-(1)/(2)((k_(1)k_(2))/(k_(1) + k_(2))) x^(2),),((C),"Work done by F on block",(R),(1)/(2) ((k_(1)k_(2))/(k_(1) + k_(2))) x^(2),),((D),"Work done by "S_(1) "on wall",(S),(1)/(2) (k_(1) k_(2)^(2)x^(2))/((k_(1) + k_(2))),):}`
View SolutionColumn-1 represents potential energy graph for certain system. Column-2 gives statement related to graphs.
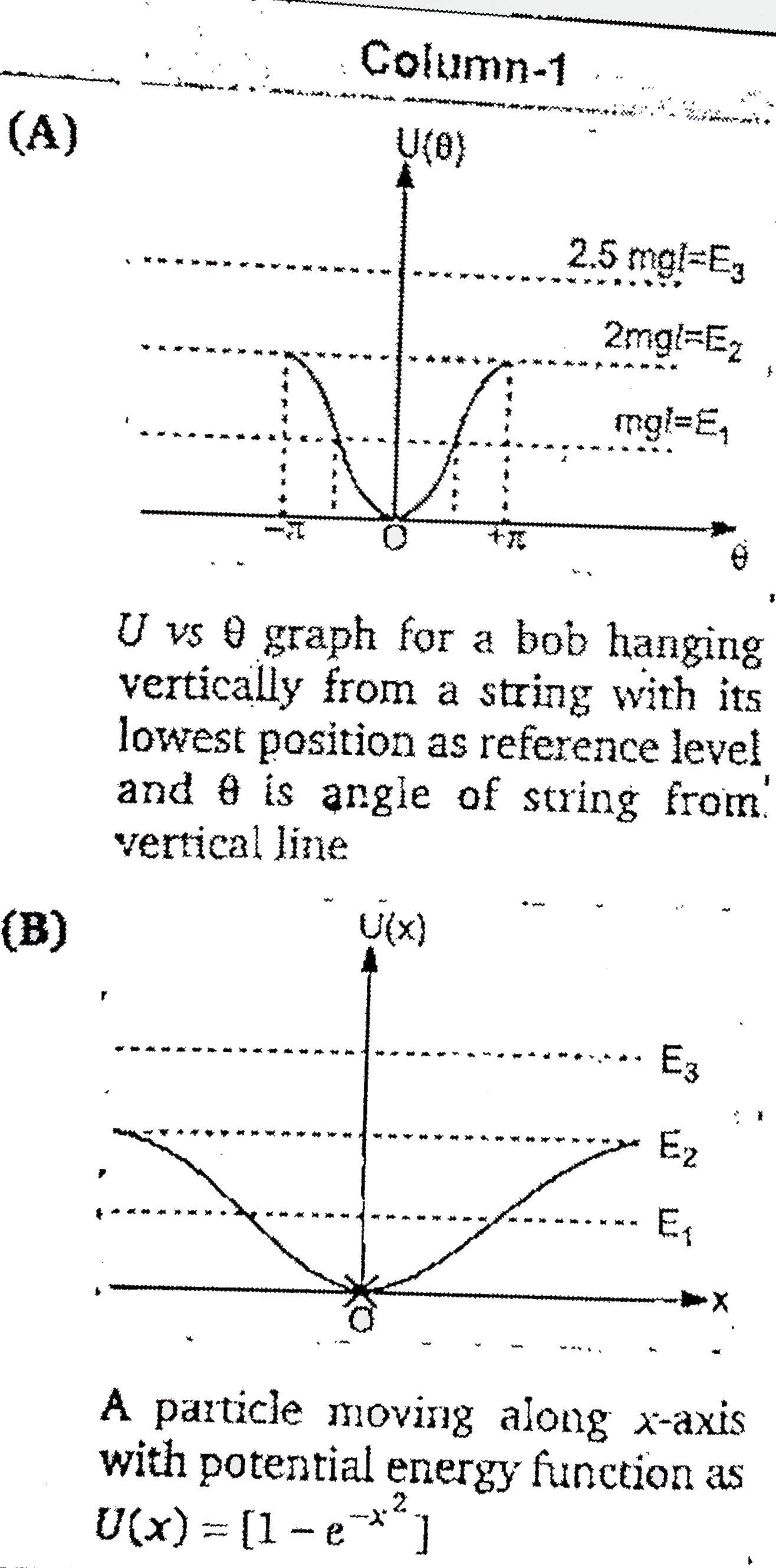
View SolutionA bob tied to an ideal string of length l is released from the horizonatal position shown. A peg P whose height is adjustable, can arrest the free swing of the pendulum, as shown in figure

`{:(,"Column-1",,"Column-2"),("(A)",underset("remaining taut throughout the swing")"For what range of y will the string wind up on the peg",,(P) (2l)/5 lt y lt (2l)/3),("(B)",underset("pendulum become projectile")"For what range of y will the",,(Q)0 lt y lt (2l)/5),("(C)",underset("mechanical energy always remain conserved")"For what value of y will",,(R)(2l)/5 lt y lt l),(,,,(S) l/3 lt y lt (2l)/3):}`
View SolutionIn column-1 a situation is depicted each of which is in vertical plane. The surfaces are frictionless. Match with appropriate entries in column-2.
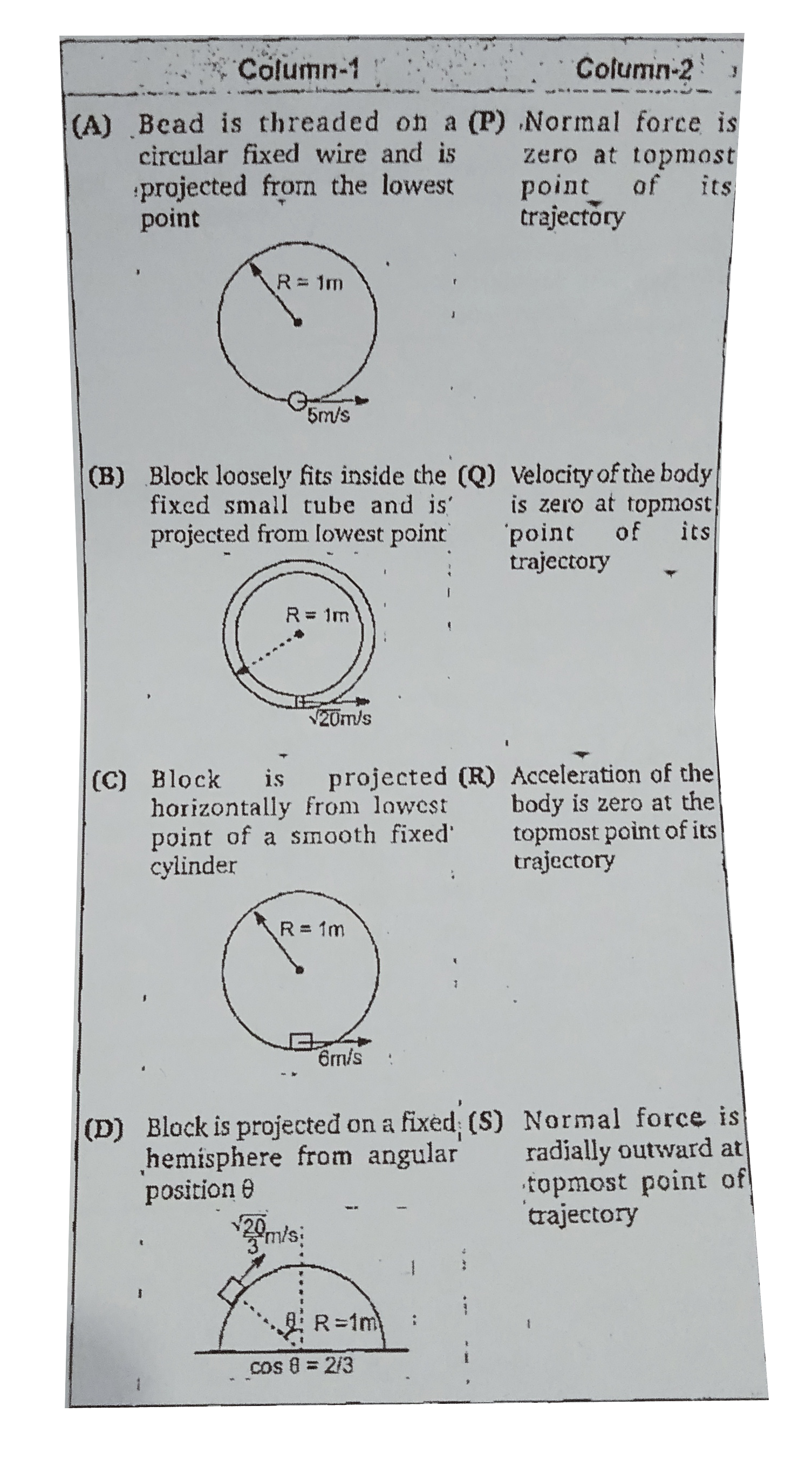
View SolutionA block of mass m is tied with an inextensible light string of length`l`. One end of the string is fixed at point 0. Block is released (from rest) at A. Find acceleration of particle during its motion in vertical plane at positions specified in column-1 and match them with column-2. Given that A and O are at same horizontal level.

`{:(,"Column -1",,"Column -2",),((A),"Highest point",(P),"Acceleration is horizontal",),((B),"At lowest point",(Q),"Acceleration is vertically",),((C),"At " theta = tan^(-1) (sqrt2) "with vertical",(R),"Acceleration is vertically downwards",),(,,(S),"Acceleration has both horizontal and vertical components",):}`
View Solution
Matching types problems questions and complete solutions for chapter WORK AND ENERGY of SHREE BALAJI PHYSICS (HINGLISH) of Class 11