Complete Solutions to LC_TYPE of chapter RIGID BODY DYNAMICS 2 of Class 11 book with complete answers and questions
| Class 11 | PHYSICS |
| Chapter | RIGID BODY DYNAMICS 2 |
| CENGAGE PHYSICS | LC_TYPE |
| Number Of Questions | 8 |
| Academic Year | 2023-24 |
Two discs `A` and `B` are mounted coaxially ona vertical axle. The discs have moments of inertia `l` and `2l` respectively about the common axis. Disc A is imparted an initial angular velocity `2 omega` using the centre potential energy of a spring compressed by a distance `x_(1)`. Disc `B` is imparted angular velocity `omega` by a spring having the same spring constant and compressed by a distance `x_(2)`. Both the disc rotate in the clockwise direction.
The rotation `x_(1)//(x_(2)` is.
View SolutionTwo discs A and B are mounted coaxiallay on a vertical axle. The discs have moments of inertia I and 2 I respectively about the common axis. Disc A is imparted an initial angular velocity `2 omega` using the entire potential energy of a spring compressed by a distance `x_1` Disc B is imparted an angular velocity `omega` by a spring having the same spring constant and compressed by a distance `x_2` Both the discs rotate in the clockwise direction.
When disc B is brought in contact with disc A, they acquire a common angular velocity in time t. The average frictional torque on one disc by the other during this period is
View SolutionTwo discs `A` and `B` are mounted coaxially ona vertical axle. The discs have moments of inertia `l` and `2l` respectively about the common axis. Disc A is imparted an initial angular velocity `2 omega` using the centre potential energy of a spring compressed by a distance `x_(1)`. Disc `B` is imparted angular velocity `omega` by a spring having the same spring constant and compressed by a distance `x_(2)`. Both the disc rotate in the clockwise direction.
The loss of kinetic energy the above process is -
View SolutionA uniform thin cylindrical disk of mass M and radius R is attaached to two identical massless springs of spring constatn k which are fixed to the wall as shown in the figure. The springs are attached to the axle of the disk symmetrically on either side at a distance d from its centre. The axle is massless and both the springs and the axle are in horizontal plane. the unstretched length of each spring is L. The disk is initially at its equilibrium position with its centre of mass (CM) at a distance L from the wall. The disk rolls without slipping with velocity `vecV_0 = vacV_0hati.` The coefficinet of friction is `mu.`
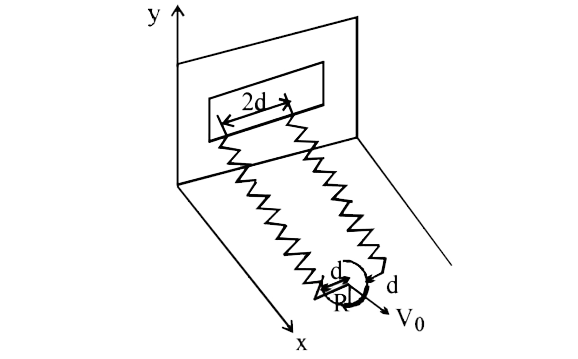
The net external force acting on the disk when its centre of mass is at displacement x with respect to its equilibrium position is.
View SolutionA uniform thin cylindrical disk of mass M and radius R is attaached to two identical massless springs of spring constatn k which are fixed to the wall as shown in the figure. The springs are attached to the axle of the disk symmetrically on either side at a distance d from its centre. The axle is massless and both the springs and the axle are in horizontal plane. the unstretched length of each spring is L. The disk is initially at its equilibrium position with its centre of mass (CM) at a distance L from the wall. The disk rolls without slipping with velocity `vecV_0 = vacV_0hati.` The coefficinet of friction is `mu.`
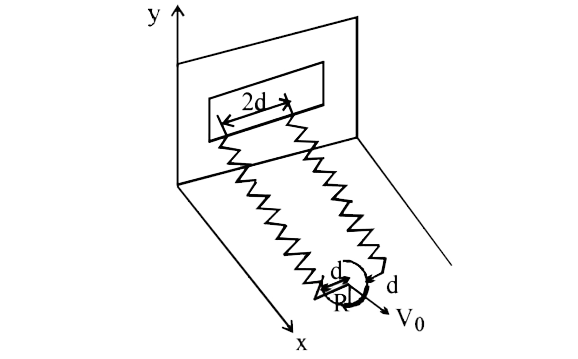
The centre of mass of the disk undergoes simple harmonic motion with angular frequency `omega` equal to -
View SolutionA uniform thin cylindrical disk of mass M and radius R is attaached to two identical massless springs of spring constatn k which are fixed to the wall as shown in the figure. The springs are attached to the axle of the disk symmetrically on either side at a distance d from its centre. The axle is massless and both the springs and the axle are in horizontal plane. the unstretched length of each spring is L. The disk is initially at its equilibrium position with its centre of mass (CM) at a distance L from the wall. The disk rolls without slipping with velocity `vecV_0 = vacV_0hati.` The coefficinet of friction is `mu.`
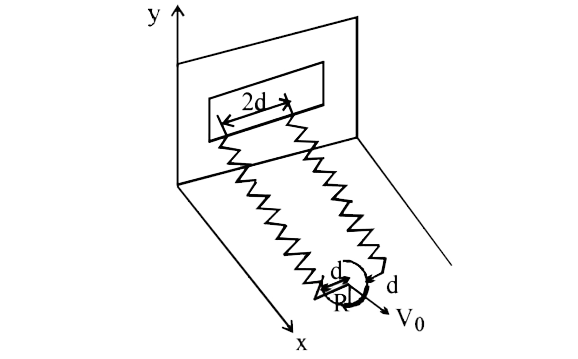
The maximum value of `V_0` for whic the disk will roll without slipping is-
View SolutionThe general motion of a rigid body can be considered to be a combination of (i) a motion of its centre of mass about an axis, and (ii) its motion about an instantaneous exis passing through the centre of mass. These axes need not be stationary. Consider, for example, a thin uniform disc welded (rigidly fixed) horizontally at its rim to a massless, stick as shown in the figure. When the disc-stick system is rotated about the origin on a horizontal frictionless plane with angular speed `omega` the motion at any instant can be taken as a combination of (i) a rotation of the disc through an instantaneous vertical axis passing through its centre of mass (as is seen from the changed orientation of points P and Q). Both these motions have the same angular speed `omega` in this case
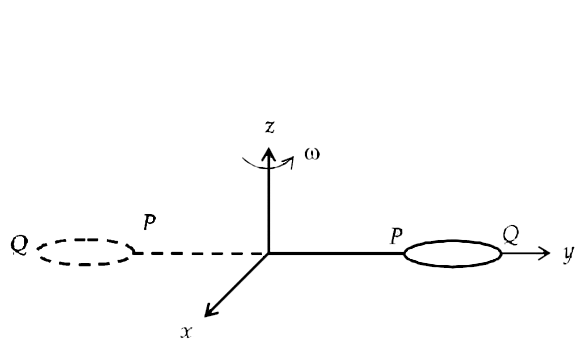
Now consider two similar system as shown in the figure: Case (a) the disc with its face vertical and parallel to x-z plane, Case (b) the disc with its face making an angle of `45^@` with x-y plane and its horizontal diameter parallel to x-axis. In both the cases, the disc is welded at point P, and the systems are rotated with constant angular speed `omega` about the z-axis.
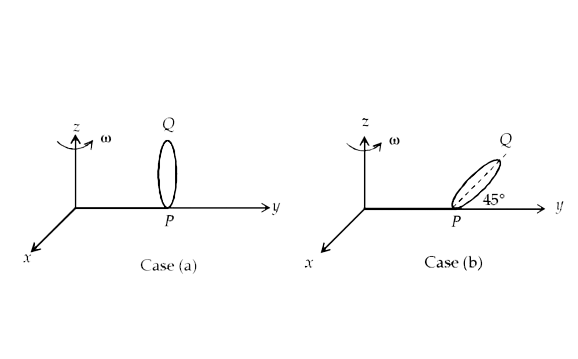
Which of the following statements regarding the angular speed about the instantaneous axis (passing through the centre of mass) is correct?
View SolutionThe general motion of a rigid body can be considered to be a combination of (i) a motion of its centre of mass about an axis, and (ii) its motion about an instantaneous exis passing through the centre of mass. These axes need not be stationary. Consider, for example, a thin uniform disc welded (rigidly fixed) horizontally at its rim to a massless, stick as shown in the figure. When the disc-stick system is rotated about the origin on a horizontal frictionless plane with angular speed `omega` the motion at any instant can be taken as a combination of (i) a rotation of the disc through an instantaneous vertical axis passing through its centre of mass (as is seen from the changed orientation of points P and Q). Both these motions have the same angular speed `omega` in this case

Now consider two similar system as shown in the figure: Case (a) the disc with its face vertical and parallel to x-z plane, Case (b) the disc with its face making an angle of `45^@` with x-y plane and its horizontal diameter parallel to x-axis. In both the cases, the disc is welded at point P, and the systems are rotated with constant angular speed `omega` about the z-axis.
 .`
.`
Which of the following statements about the instantaneous axis (passing through the centre of mass) is correct?
View Solution
LC_TYPE questions and complete solutions for chapter RIGID BODY DYNAMICS 2 of CENGAGE PHYSICS (HINGLISH) of Class 11