Complete Solutions to Illustration of chapter RIGID BODY DYNAMICS 2 of Class 11 book with complete answers and questions
| Class 11 | PHYSICS |
| Chapter | RIGID BODY DYNAMICS 2 |
| CENGAGE PHYSICS | Illustration |
| Number Of Questions | 57 |
| Academic Year | 2023-24 |
A uniform sphere of mass `m` and radius `r` rolls without sliding over a horizontal plane, rotating ahout a horizontal axle `OA`. In the process, the centre of the sphere moves with a veocity `v` along a circle of radius `R`. Find the kinetic energy of the sphere.
View SolutionA uniform rod of mass `m=2 kg` and length `l` is kept on a smooth horizontal plane. If the ends `A` and `B` of the rod move with speeds `v` and `2v` respectively perpendicular to the rod, find the:
a. angular velocity of `CM` of the rod.
b. Kinetic energy of the rod (in Joule).

View SolutionA rod of mass `m` and length `l` is connected with a light rod of length `l`. The composite rod is made to rotate with angular velocity `omega` as shown in the figure. Find the
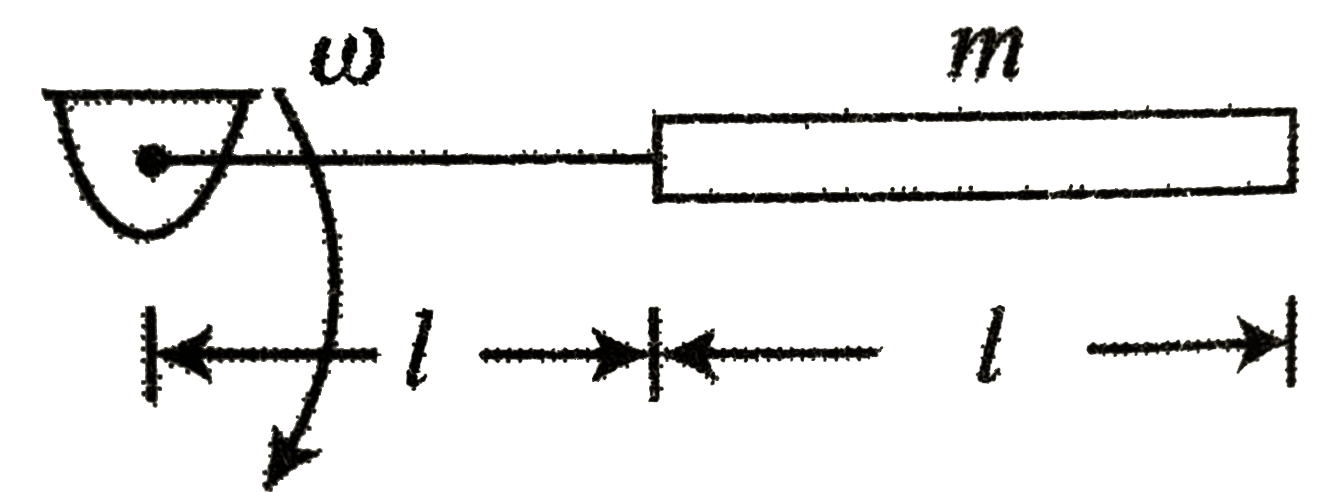
a. translational kinetic energy.
b. rotational kinetic energy.
c. total kinetic energy of rod.
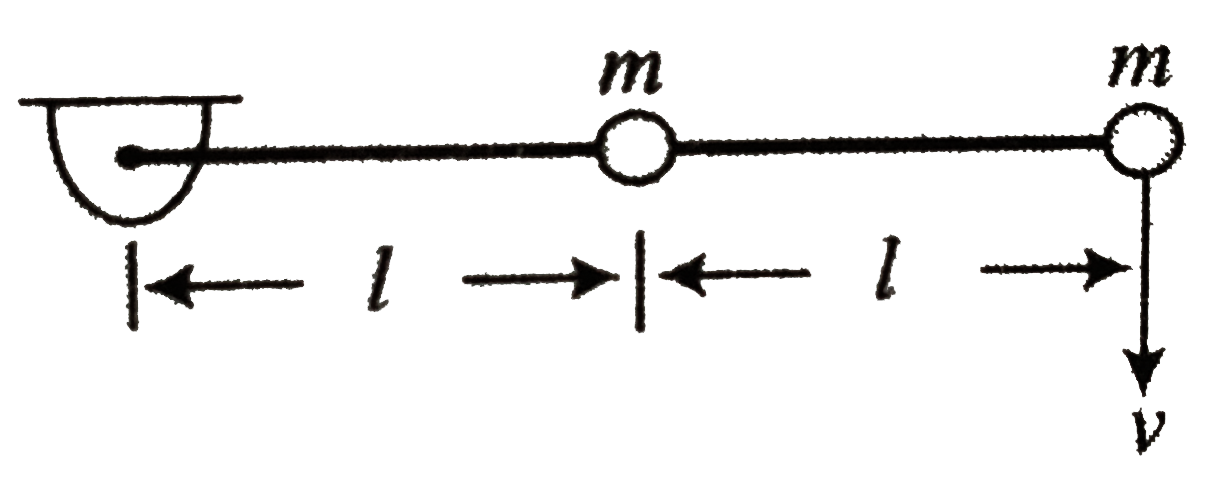
View SolutionTwo beads each of mass `m` are welded at the ends of two light rigid rods each of length `l`. if the pivots are smooth, find the ratio of translational and rotational kinetic energy of system.
View SolutionCalculate the torque developed by ann airplane engine whose output is `2000 HP` at an angular velocity of `2400 rev//min`.
View SolutionA thin horizontal uniform rod `AB` of mass `m` and length `l` can rotate freely about a vertical axis passing through its end `A`. At a certain moment, the end `B` starts experiencing a constant force `F` which is always perpendicular to the original position of the stationary rod and directed in a horizontal plane. The angular velocity of the rod as a function of its rotation angle `theta` measured relative to the initial position should be.
View SolutionA uniform rod of mass `m` and length `l` is pivoted smoothly at `O`. A horizontal force acts at the bottom of the rod.
a. Find the angular velocity of the rod as the function of angle of rotation `theta.`
b.What is the maximum angular displacement of the rod?
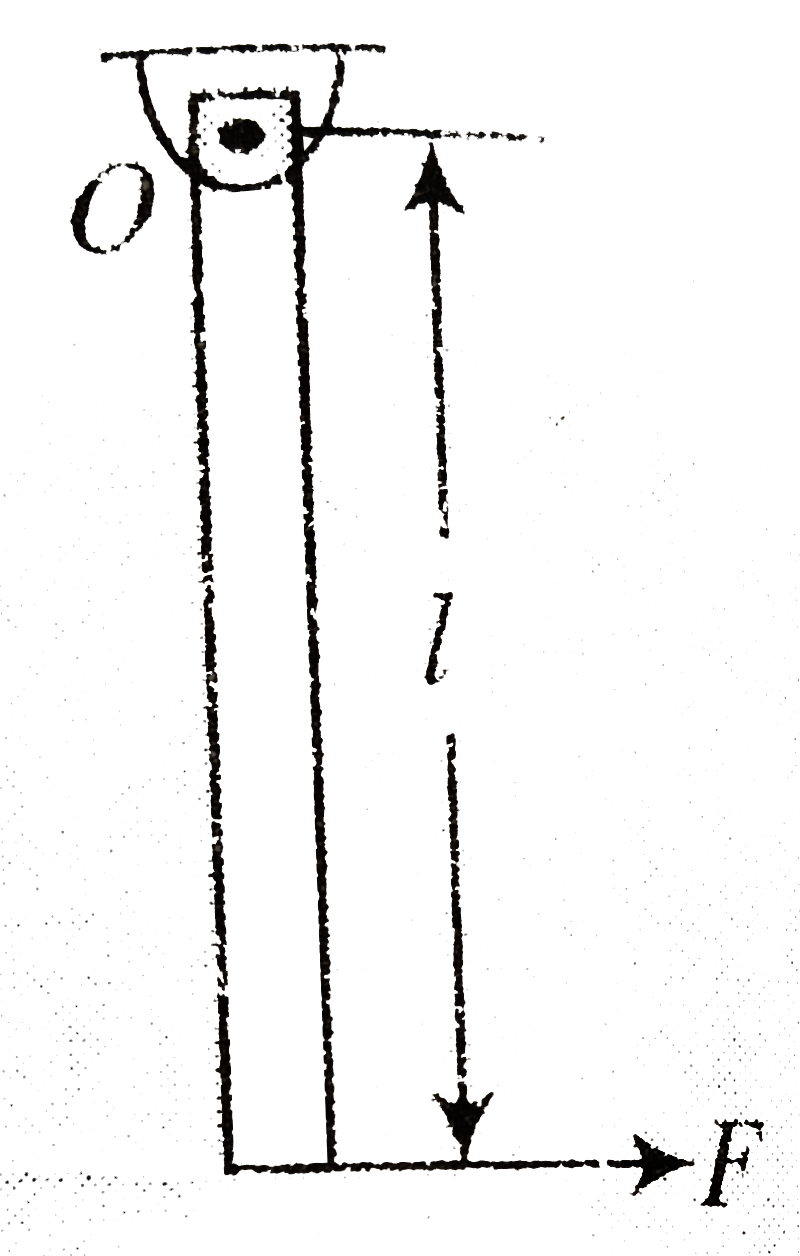
View SolutionThe top is figure has moment of inertia equal to `4.00xx10^(4) kgm^(2)` and is initially at rest. It is free to rotate about the stationary axis `"AA"'`. A string wrapped around a ped alonng the axis of the top is pulled in such a manners as to maintain a constant tension of `5.57N`.If the string does not slip while it is unwound from the peg, what is the angular speed of the top after `80.0 CM` of string has been pulled of the peg?

View SolutionA rod of length `l` is pivoted about a horizontal , frictionless pin through one end. The rod is released from ret in a vertical position. Find the velocity of the `CM` of the rod when the rod is inclined at an angle `theta` from the vertical.

View SolutionA solid cyinder of mass `m=4 kg` and radius `R=10 cm` has two ropes wrapped around it. one near each end. The cylinder is held horizontally by fixing the two free ends of the cords to the hooks on the ceiling such that both the cords are exactly vertical. The cylinder is released to fall under gravity. Find the linear acceleration of the cylinder.
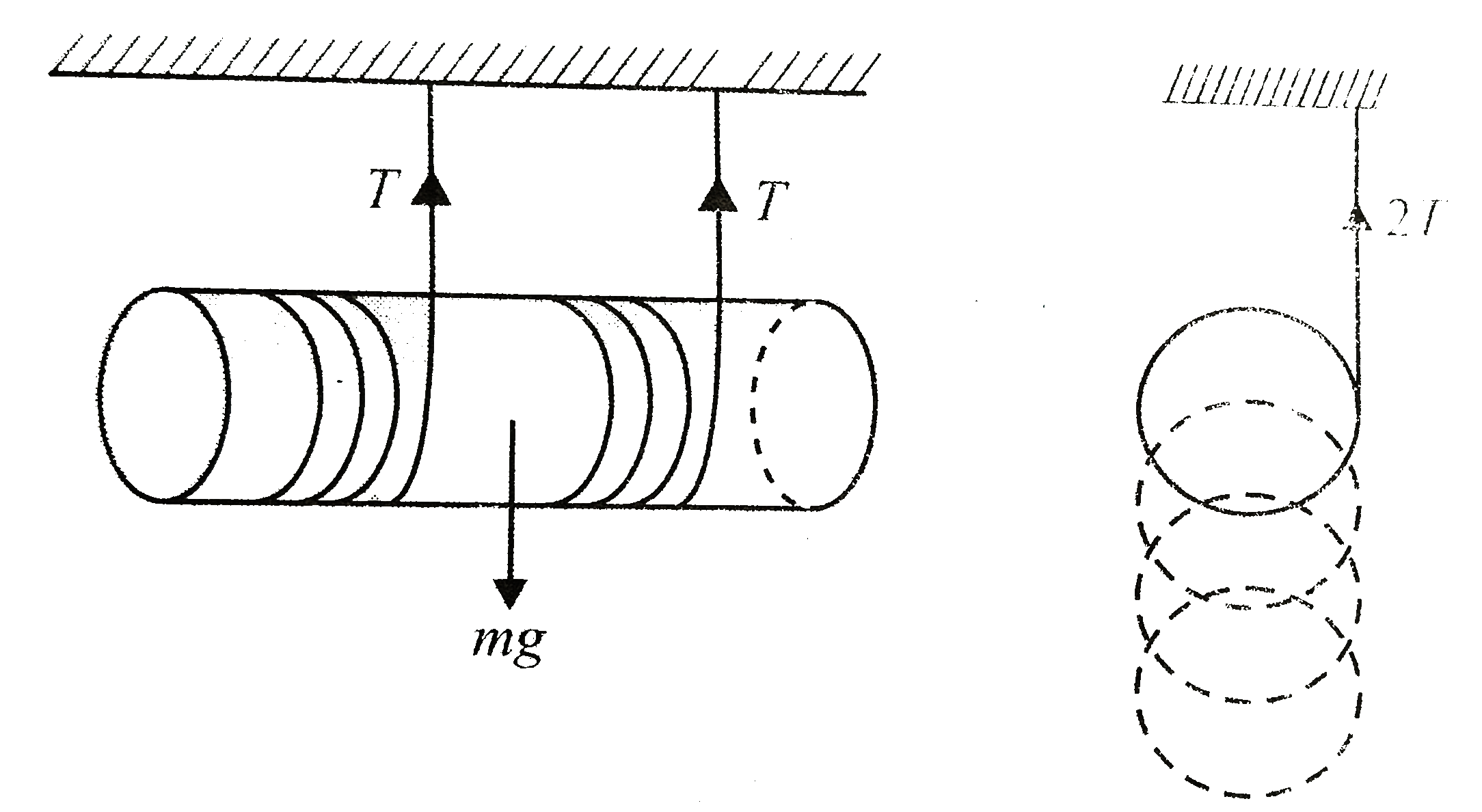
View Solution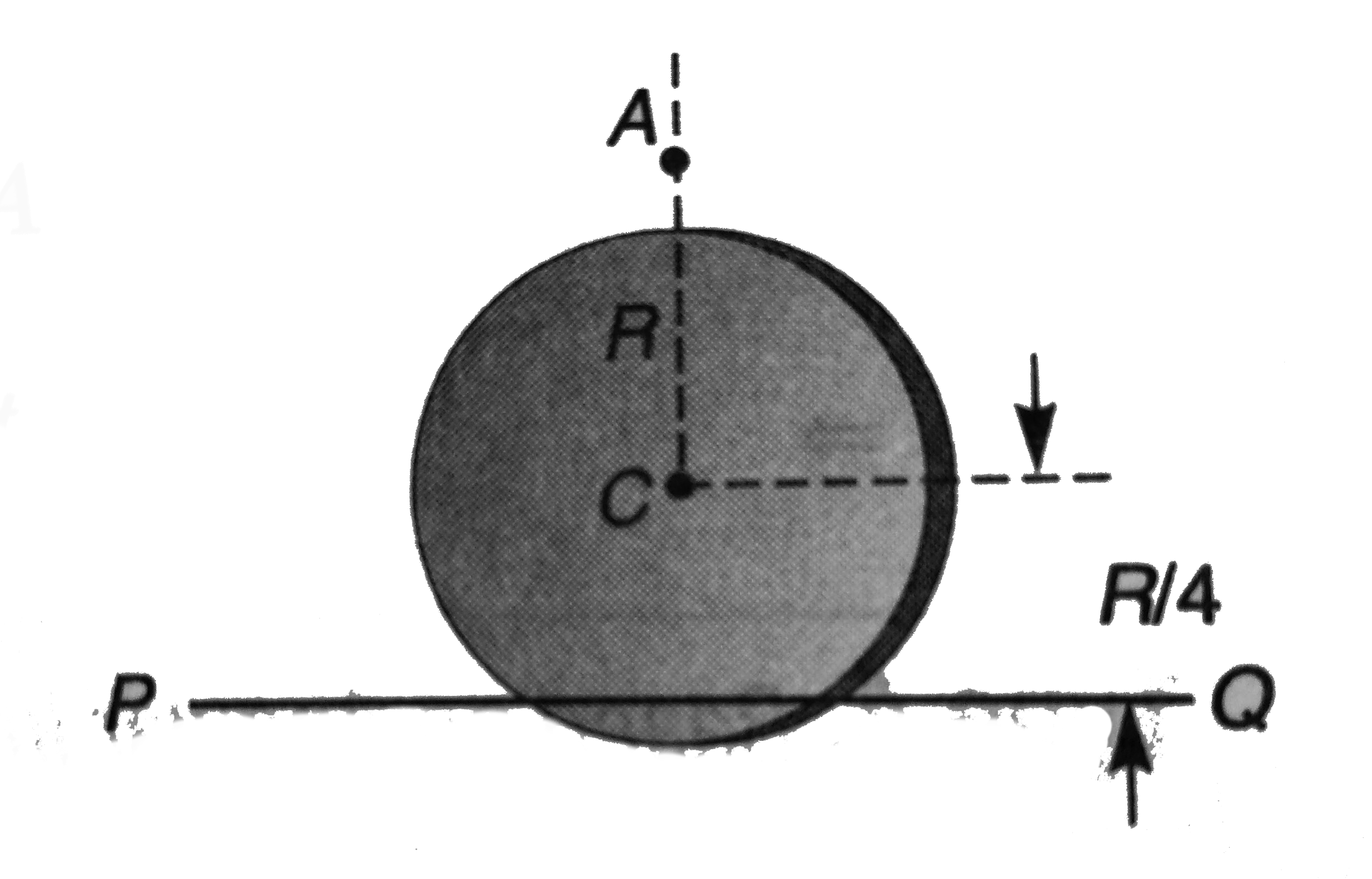
A uniform circular disc has radius `R` and mass `m`. A particle, also of mass `m`, if fixed at a point A on the edge of the disc as shown in the figure. The disc can rotate freely about a horizontal chord PQ that is at a distance `R//4` from the centre `C` of the disc. The line AC is perpendicular to `PQ`. Initially the disc is held vertical with the point A at its highest position. it is then allowed to fall, so that it starts rotation about PQ. Find the linear speed of the particle as it reaches its lowest position.
View SolutionA particle of mass `m` is moving along the line `y=b,z=0` with constnat speed `v`. State whether the angular momentum of particle about origin is increasing. Decreasing or constant.
View SolutionA particle is projected at time t=0 from a point P wilth a speed `v_0` at an angle of `45^@` to the horizontal. Find the magnitude and the direction of the angular momentum of the parallel about the point P at time `t=v_0/g`
View SolutionA uniform disc of mas `M` and radius `R` is smoothly pivoted at `O`. A light inextensible string wrapped over the disc hangs a particle of mass `m`. If the system is released from rest, assuming that the string does not slide on the disc, find the angular speed of the disc as the function of time using impulse momentum equation.
View SolutionFind the angular momentum of a disc about the axis shown in figure in the following situations.
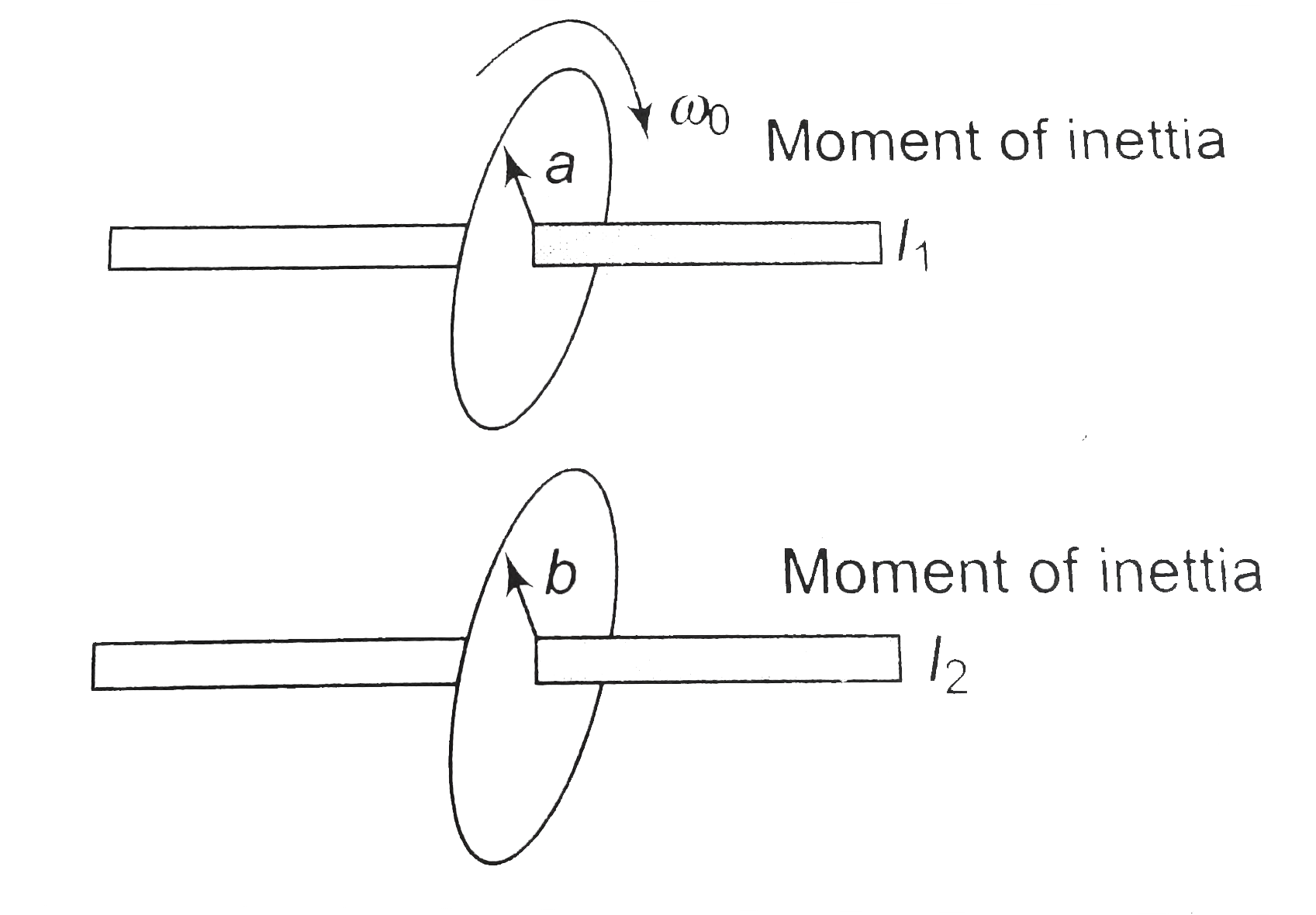
View SolutionThe two uniform discs rotate separately on parallel axles. The upper disc (radius `a` and momentum of inertia `I_1`) is given an angular velocity `omega_0` and the lower disc of (radius `b` and momentum of inertia `I_2`) is at rest. Now the two discs are moved together so that their rims touch. Final angular velocity of the upper disc is.
 .
.
View SolutionA turntable turns about a fixed vertical axis, making one revolution in `10 s`. The moment of inertia of the turntable about the axis is `1200 kgm^(2)`. A man of `80 kg`, initially standing at centre of the turnable, runs out along the radius. What is the angular velocity of the turnable when the man is `2 m` from the centre?
View SolutionA man of mass `m` stands on a horizontal platform in the shape of a disc of mass `m` and radius `R`, pivoted on a vertical axis through its centre about which it can freely rotate. The man starts to move around the centre of the disc in a circle of radius `r` with a velocity `v` relative to the disc. Calculate the angular velocity of the disc.
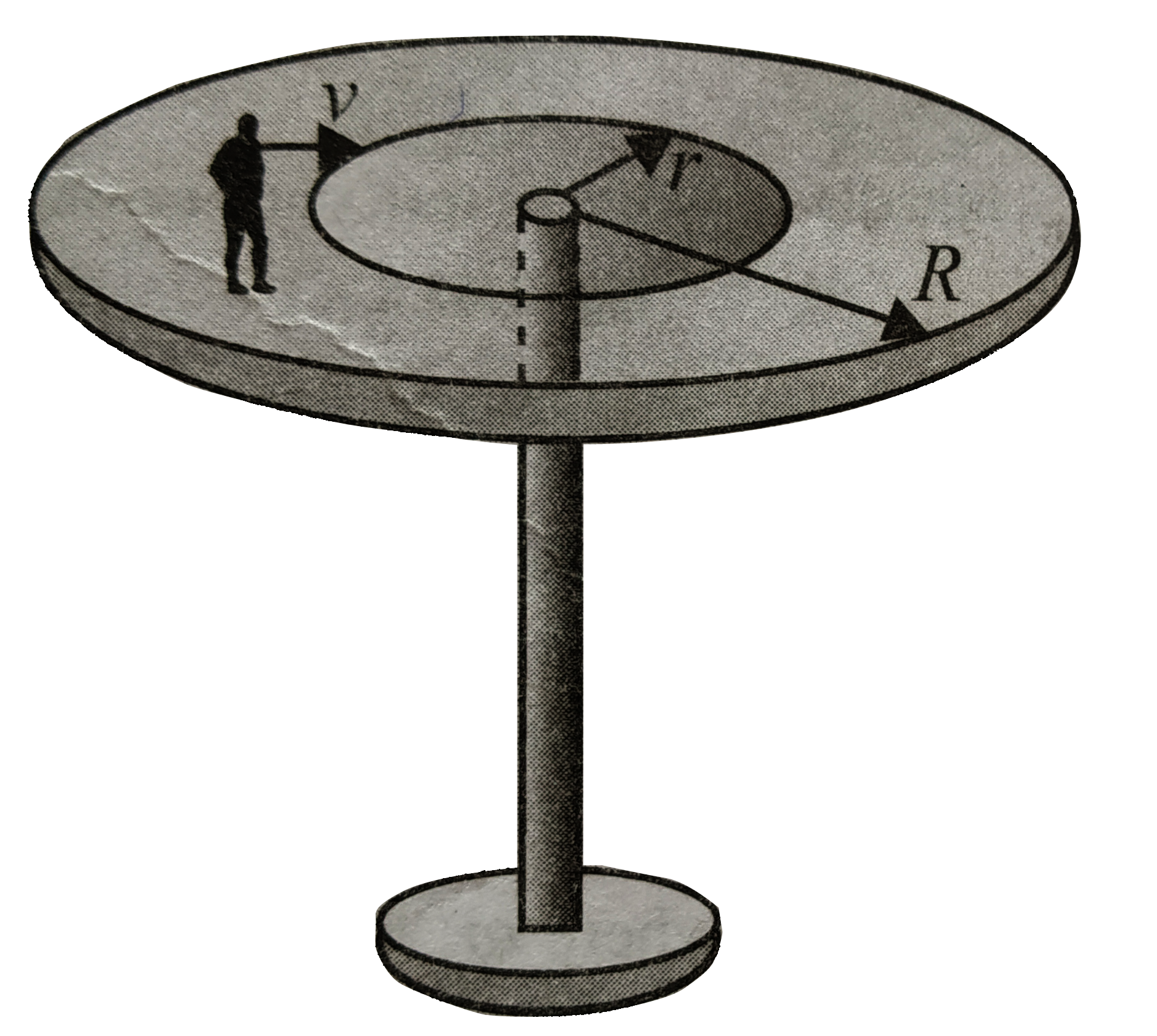
View SolutionA man of mass `100 kg` stands at the rim of a turtable of radius `2 m` and moment of inertia `4000 kgm^(2)` mounted on a vertical frictionless shaft at its centre. The whole system is initially at rest. The man now walks along the outer edge of the turntable with a velocity of `1m//s` relative to the earth
a. With what angular velocity and in what direction does the turntable rotate?
b. Through what angle will it have rotated when the man reaches his initial position on the turntable?
c. Through what angle will it have rotated when the man reaches his initial position relative to the earth?
View SolutionA small block of mass `4kg` is attached to a cord passing through a hole in a horizontal frictionless surface. The block is originally revolving in a circle of radius `0.5 m` about the hole with a tangential velocity of `4m//s`. The cord is then pulled slowly from below, shorting the radius of the circle in which the block revolves. The breaking strength of the cord is `600 N`. What will be radius of the circle when the cord breaks?
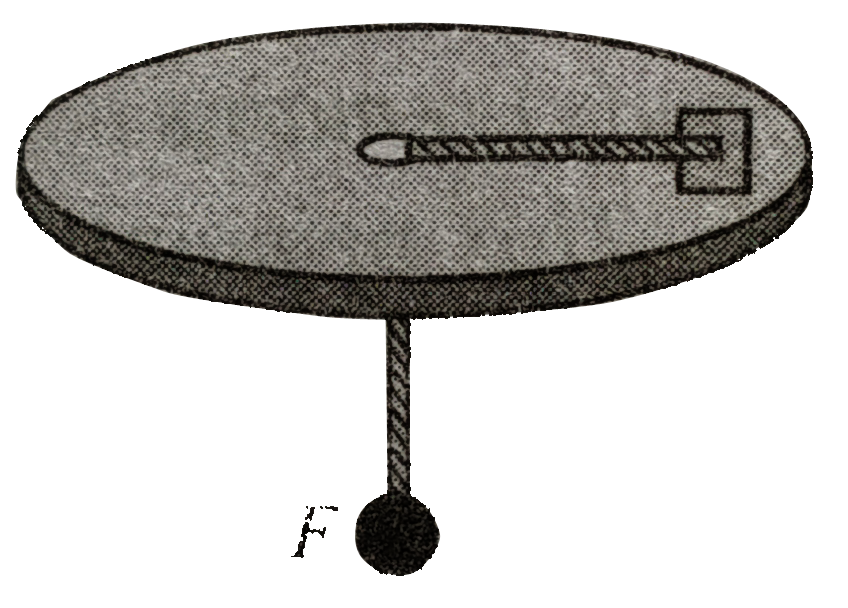
View SolutionTwo skaters each of mass `50 kg`, approach each other along parallel paths separated by `3m`. They have equal ad opposite velocities of `10 m//s`. The first skater carries a long light pole, `3 m` long, and the second skater grabs the end of it as he passes (assume frictionless ice).
a. Described quantitatively the motion of the skaters after they are connected by the pole.
b. By pulling on the skaters reduce their distance to `1m`. What is their motion then?
c. Compare the `KEs` of the system in parts a. and b. where does the change come from?
View SolutionA string is wrapped several times on a cylinder of mass `M` and radius `R`. the cylinder is pivoted about its adxis of block symmetry. A block of mass `m` tied to the string rest on a support positioned so that the string has no slack. The block is carefully lifted vertically a distance `h`, and the support is removed as shown figure.
a. just before the string becomes taut evalute the angular velocity `omega_(0)` of the cylinder ,the speed `v_(0)` of the falling body, `m` and the kinetic energy `K_(0)` of the system.
b. Evaluate the corresponding quanitities `omega_(1), v_(1)` and `K_(1)` for the instant just after the string becomes taut.
c. Why is `K_(1)` less than `K_(0)`? Where does the energy go?
d. If `M=m`, what fraction of the kinetic energy is lost when the string becomes taut?
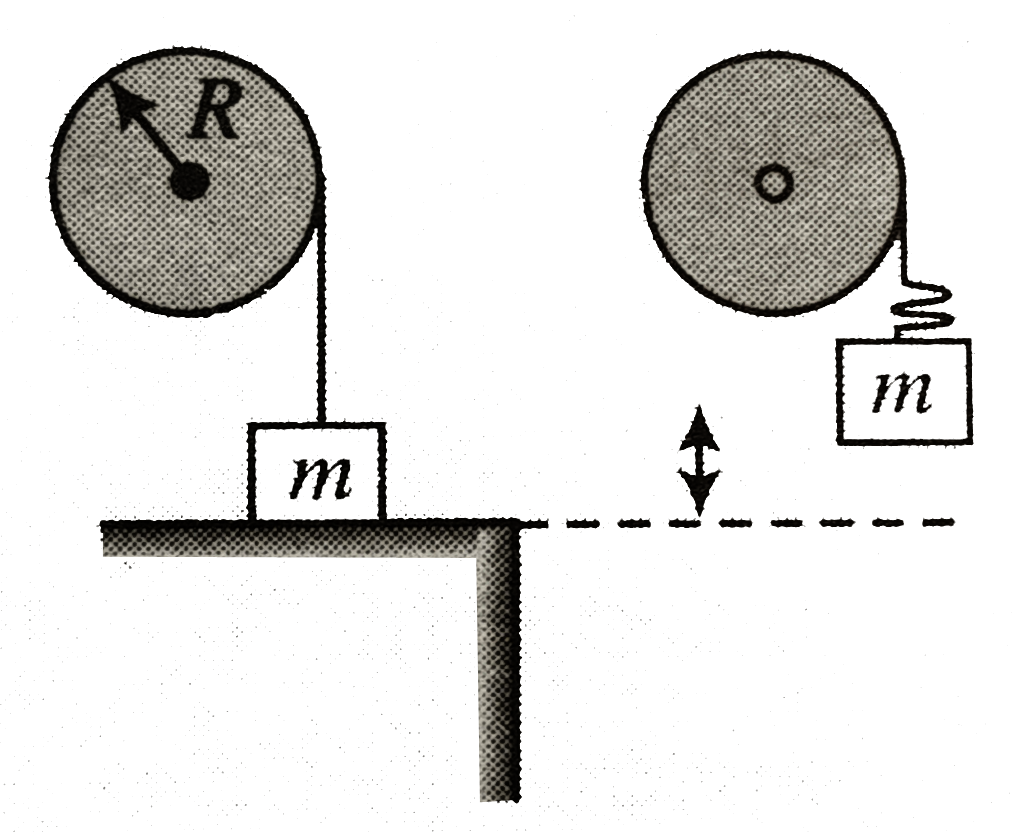
View Solution`AB` is a horizontal diameter of a ball of mass `m=0.4 kg` and radius `R=0.10m`. At time `t=0`, a sharp impulse is applied at `B` at angle of `45^@` with the horizontal as shown in figure so that the ball immediately starts to move with velocity `v_(0)=10ms^(-1)`
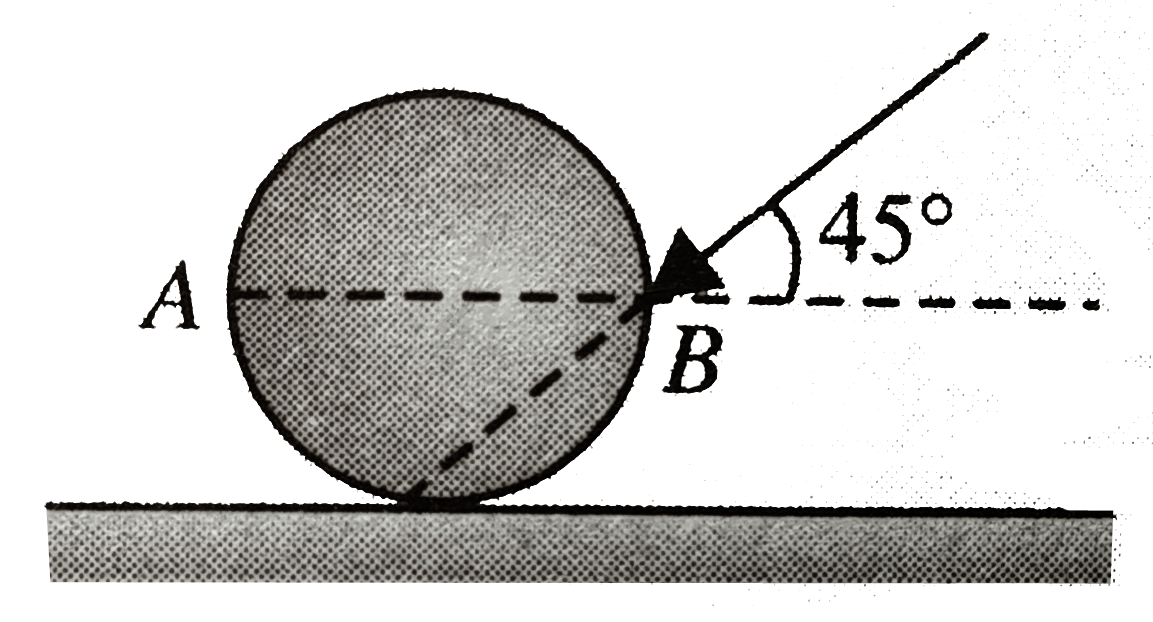
a. Calculate the impulse and angular velcity of ball just after impulse provided.
If coefficient of kinetic friction between the floor and th ball is `mu=0.1`. Calculate. The
b. velocity of ball when it stops sliding,
c. time t at that instant
d. horizontal distance travelled by the ball up to that instant.
e. angular displacement of the ball about horizontal diameter perpendicular to `AB`, up to that instant, and
f. energy lost due to friction (`g=10ms^(-2))`
View SolutionA pulley of radius `b=20 cm` is fixed with a shaft of radus `a=10 cm`. Moment of inertia of shaft puley system is `I=(33-800)kgm^(2)` and the system is free to rotate about axis `O` of the shaft without friction.
A block `B` of mass `m_(2)=8 kg` is resting over ann ideal spring of force costant. `K=2048Nm^(-1)`
Lower end of the spring is fixed to the floor and the spring is vertical. Thread connected betwen shaft and block `B` is just taut.

Another thead is connected between pulley and block `A` of mass `m_(1)=4 kg`. Initially this thread is loose. When block `A` is released first it falls freely through a height `h=405/1024m` then the thread becomes taut and block `B` is jerked into motion.
calculate:
a. Initial compressiion of the spring
b. Velocity of block `B` when it is jerked into motion,
c. Loss of energy during that jerk `(g=10 ms^(-2))`
View SolutionA cylindrical drum, pushed along by a board rolls forward on the ground. There is no slipping at any contact. The distance moved by the man who is pushing the board, when axis of the cylinder covers a distance `L` will be.
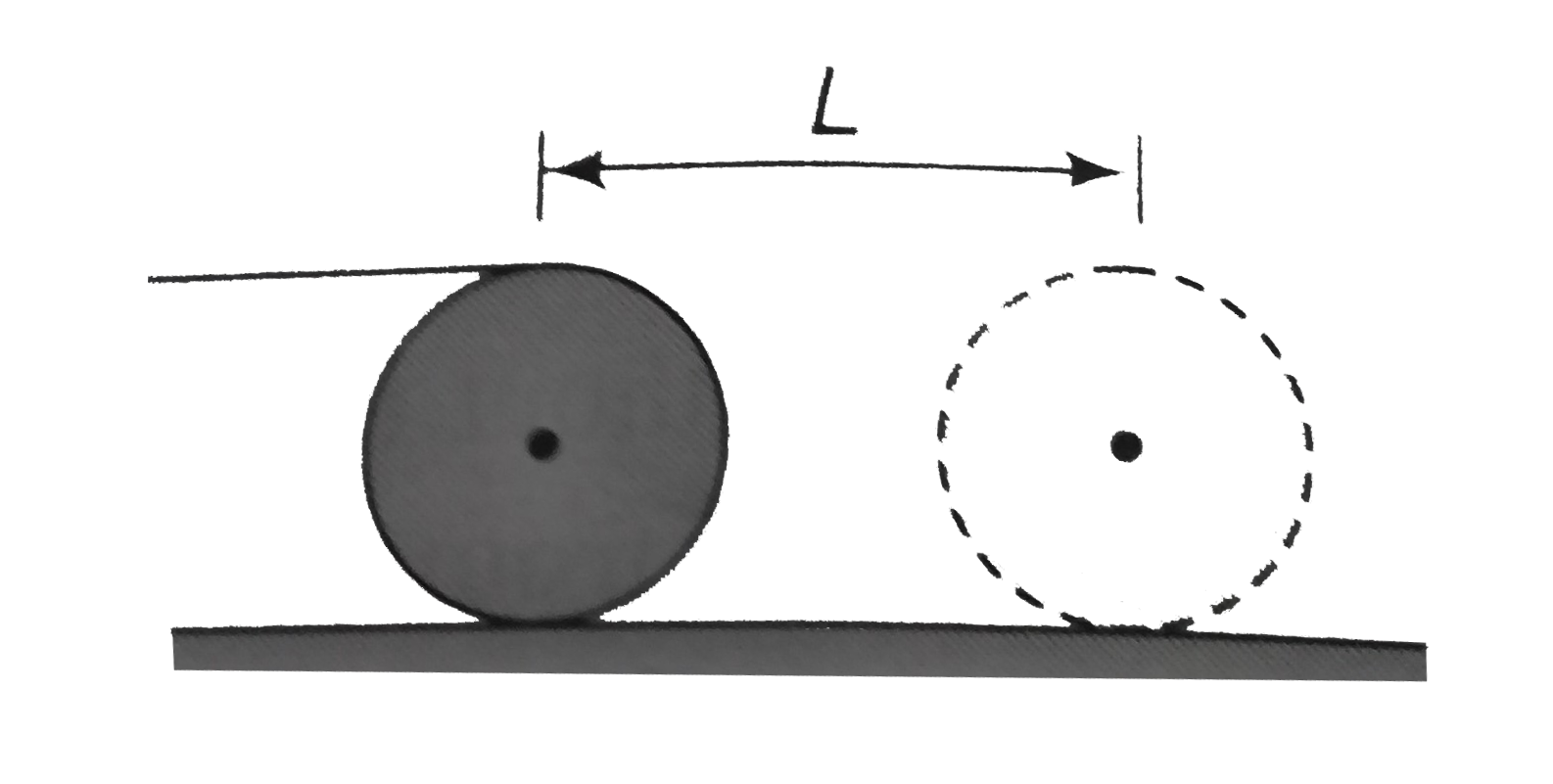
View SolutionA bobbin is pushed along on a rough stationary horizontal surface as shown in the figure. The board is kept horizontal and there is no slipping at any contact points. The distance moved by the board when distance moved by the axis of the bobbin is `l` is
 .
.
View SolutionA uniform disc of radius `R` rolls perfectly over two horizontal plank `A` and `B` moving with velocities `v` and `2v`, respectively. Find the
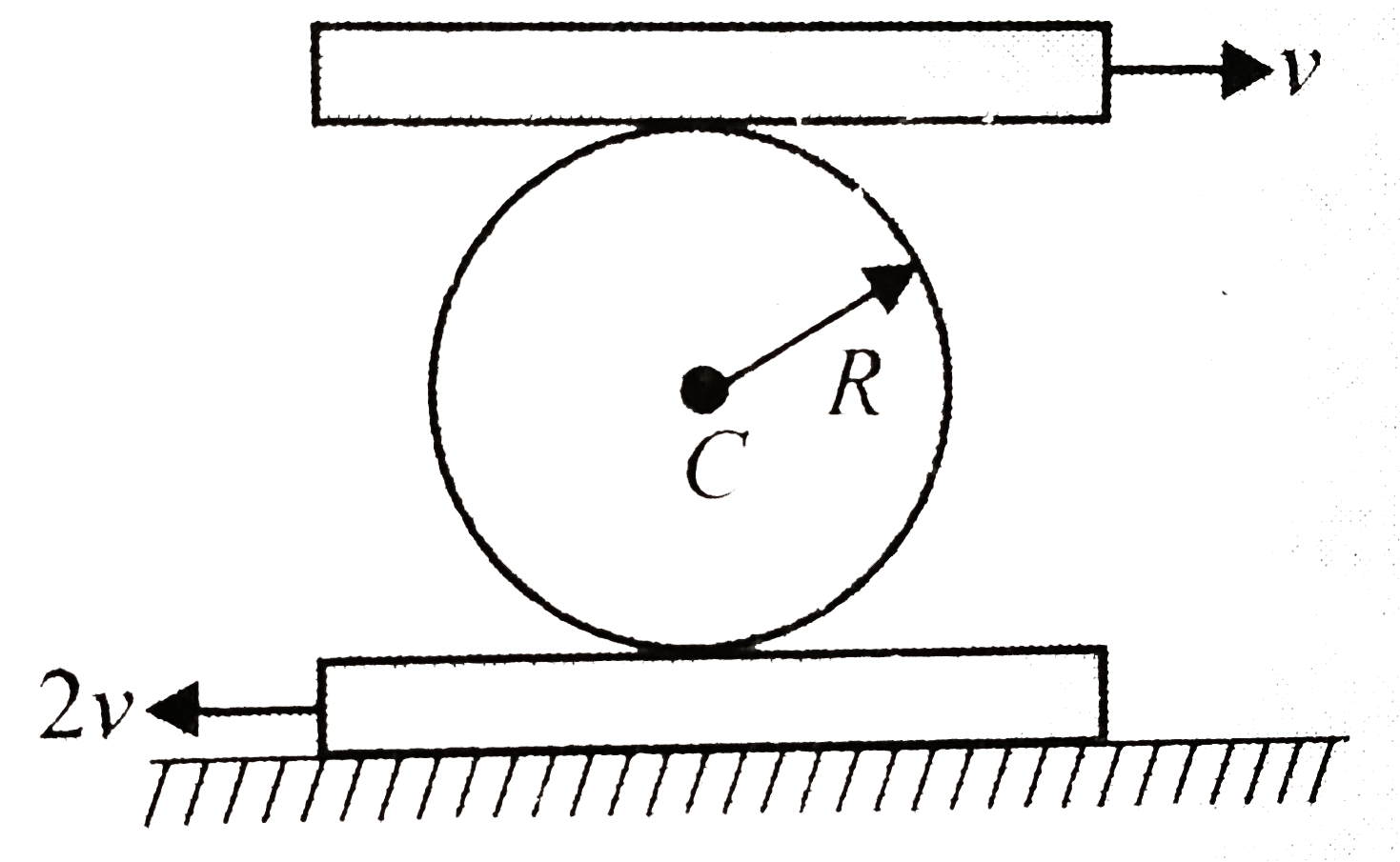
a. velocity of `CM` of the disc.
b. angular velocity of the disc.
View SolutionA wheel rolls purely on ground. Find a point on the periphery of a body which has a velocity equal to the velocity of the centre of mass of the body.
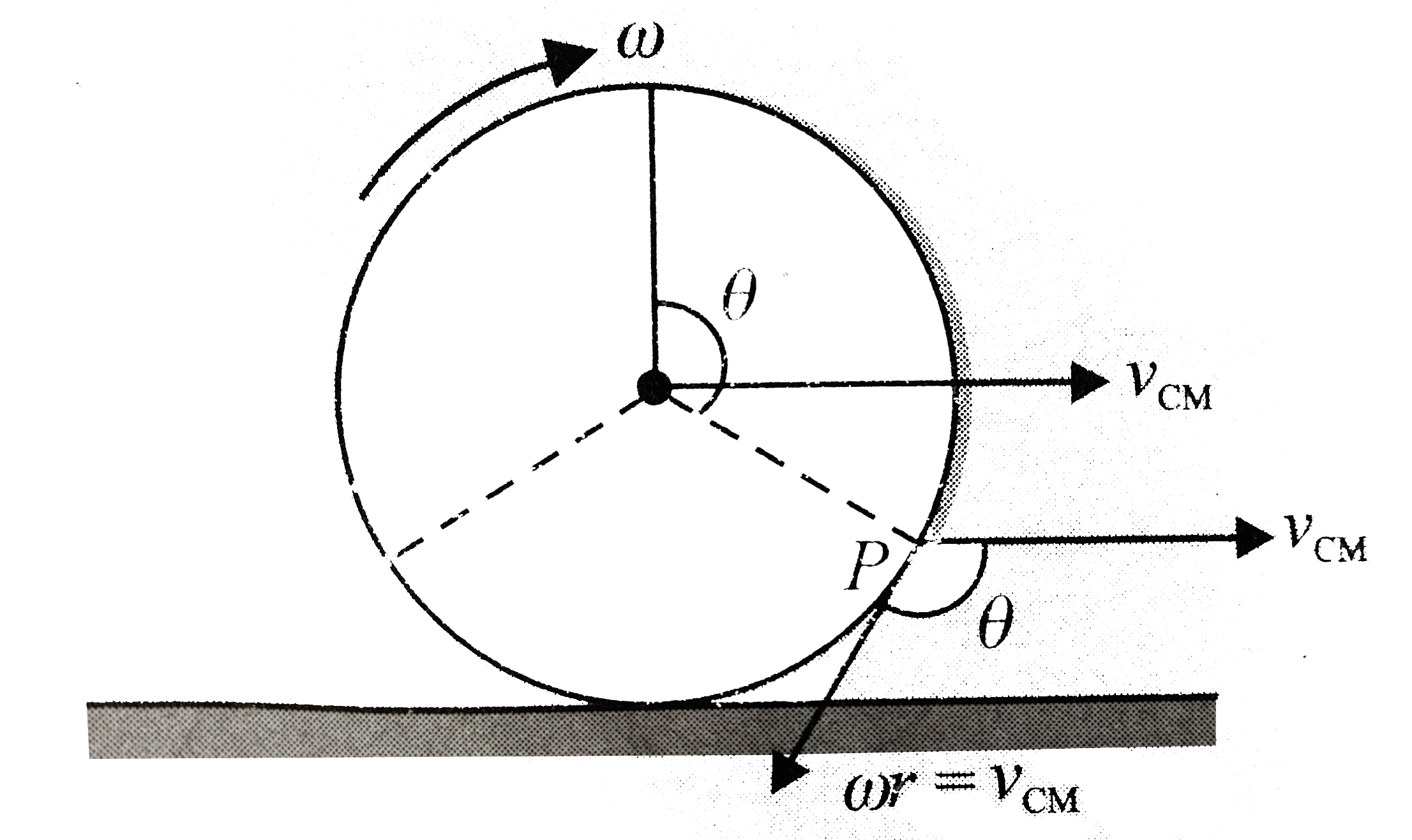
View SolutionA rod of mass `m` is kept on a cylinder and sphere each of radius `R`. The masses of the sphere and cylinder are `m_(1)` and `m_(2)` respectively. If the speed of the rod is `V`, find the `KE` of the system `("rod"+"cylider"+"sphere")`. Assume that the surfaces do not slide relative to each other.

View SolutionA sphere of mass `M` rolls without slipping on rough surface with centre of mass has constant speed `v_0`. If mass of the sphere is `m` and its radius be R`, then the angular momentum of the sphere about the point of contact is.
View SolutionConsider a wheel rolls without slipping and its centre moves with constant acceleration a. Find the acceleration of points `O,P,Q` and `S` when linear velocity of the centre of wheel is `v`.
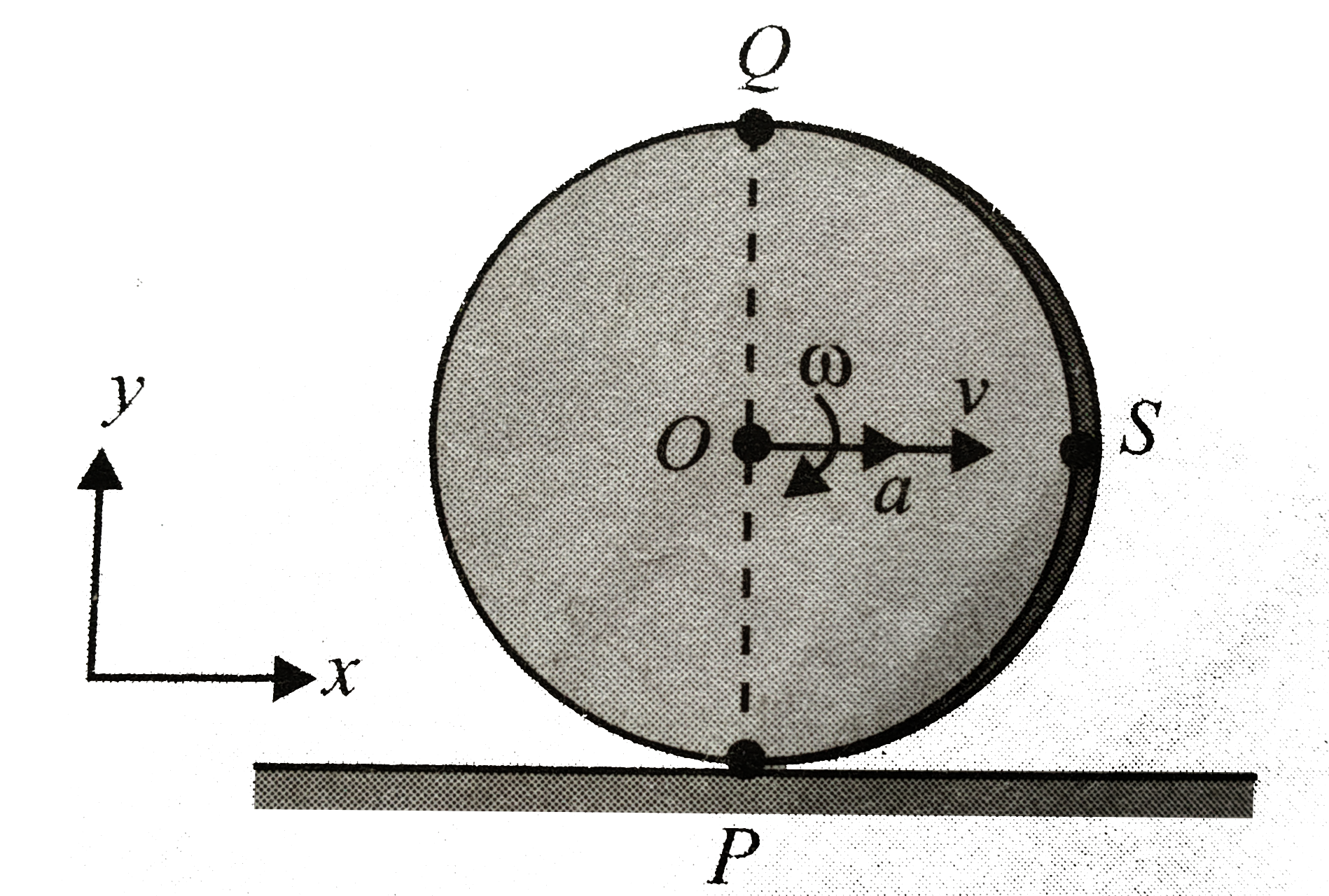
View SolutionA force of magnitude `F` is acting on rolling body of mass `m` and radius `R` as show in figure. What happens when it enters on a smooth surface?

View SolutionA rolling body of mass `m` and radius `R` is rolling on a rough surface without any pulling force as shown in figure. What happens when it enters on a smooth surface?
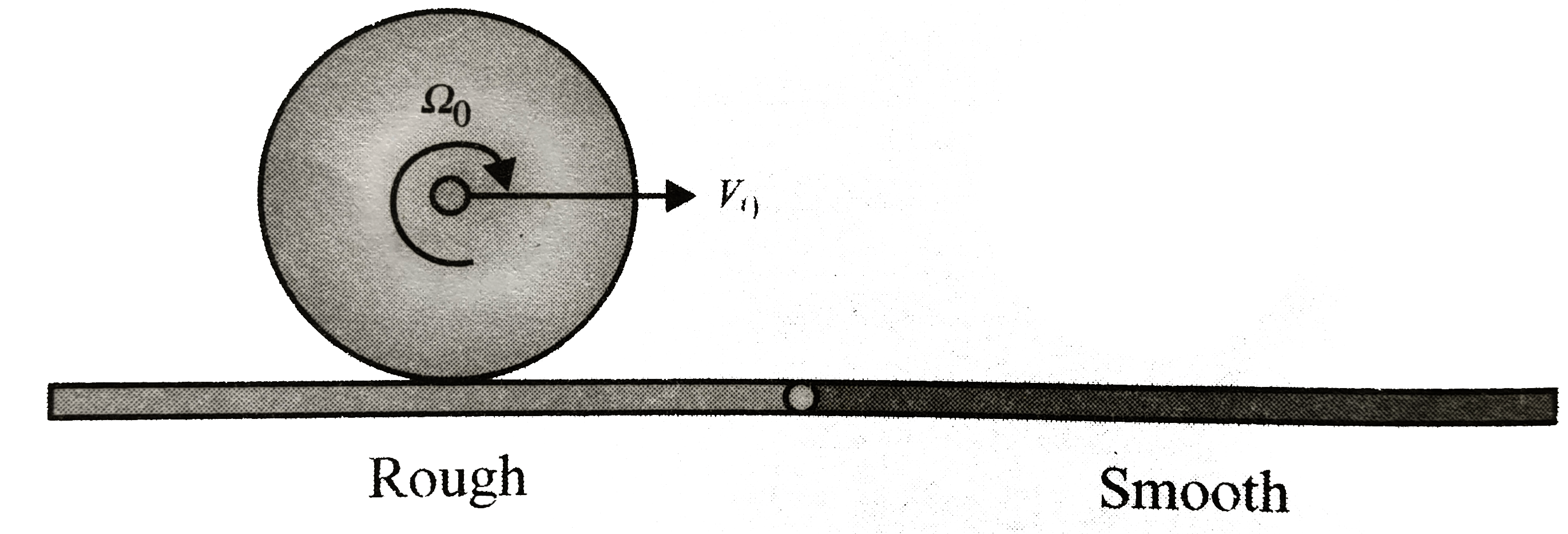
View SolutionA sphere of mass `M` and radius `r` shown in figure slips on a rough horizontal plane. At some instant it has translational velocity `V_(0)` and rotational velocity about the centre `(v_(0))/(2r)`. Find the translational velocity after the sphere starts pure rolling.
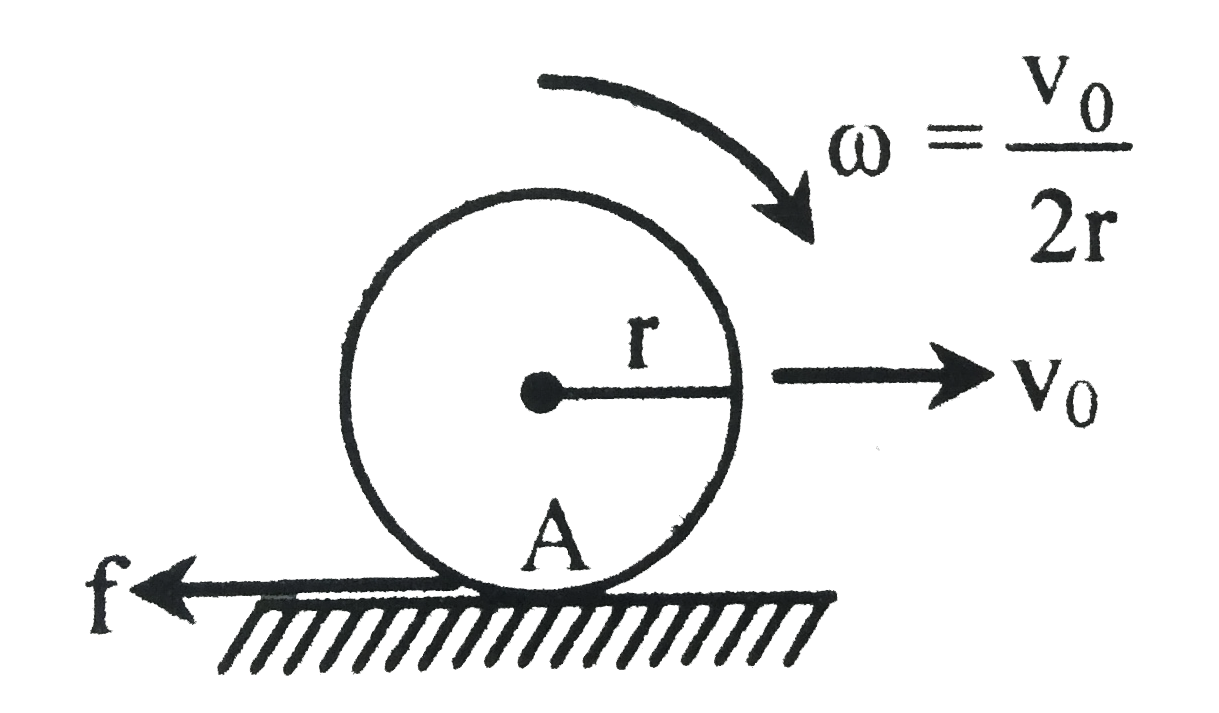 .
.
View SolutionA ball (solid sphere) is thrown down the valley in such a way that it slides with a speed ` v_(0)` initially without rolling. Prove that it will roll without any sliding when its speed falls to `(5/7)v_(0)`. The transition from pure sliding to pure rolling is gradual, so that both sliding and rolling take place during this interval.
View SolutionA uniform disc of mass `m` and radius `R` is projected horizontally with velocity `v_(0)` on a rough horizontal floor so that it starts off with a purely sliding motion at `t=0`. After `t_(0)` seconds, it acquires pure rolling motion as shown in the figure.
(a) Calculate the velocity of the center of mass of the disc at `t_(0)`.
Assuming that the coefficient of friction to be `mu`, calculate `t_(0)`.

View SolutionA plank of mass `M`, whose top surface is rough with coefficient of friction `mu` is placed on a smooth ground. Now a disc of mass `m=M//2` and radius `r` is placed on the plank. The disc is now given a velocity `v_(0)` in the horizontal direction at `t=0`

a. Find the time when the disc starts rolling
b. Find the velocity of the plank and the disc up to that time.
c. Find the distance travelled by the plank up to this instant.
Find the work done by the friction force up to this instant.
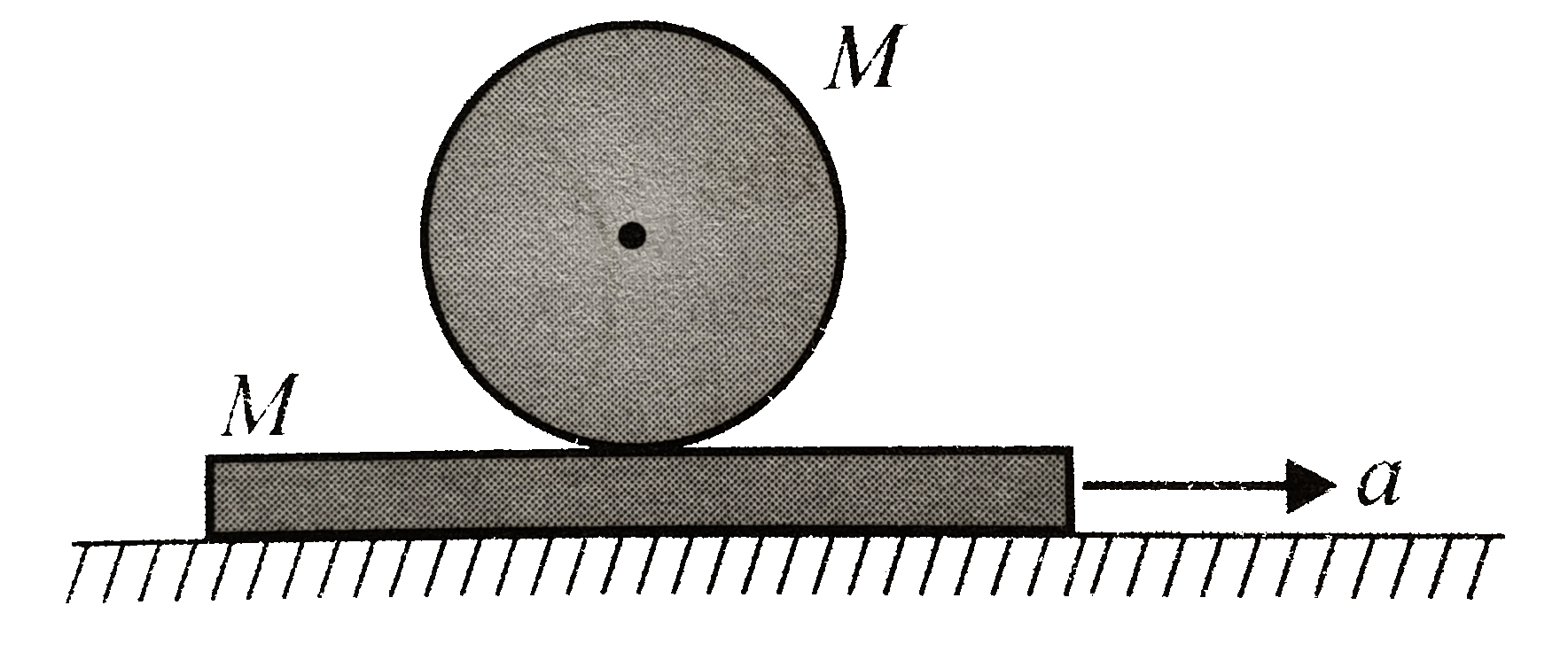
View SolutionA uniform cylinder (mass `M`) of radius `R` is kept on an accelerating platform (mass `M`) as shown in figure. If the cylinder rolls withut slipping on the platform, determine the magnitude of acceleration of the centre of mass of the cylinder. Assuming th coefficient of friction `mu=0.40`, determinete maximum acceleration the platform may have without slip between the clyinder and the platform.
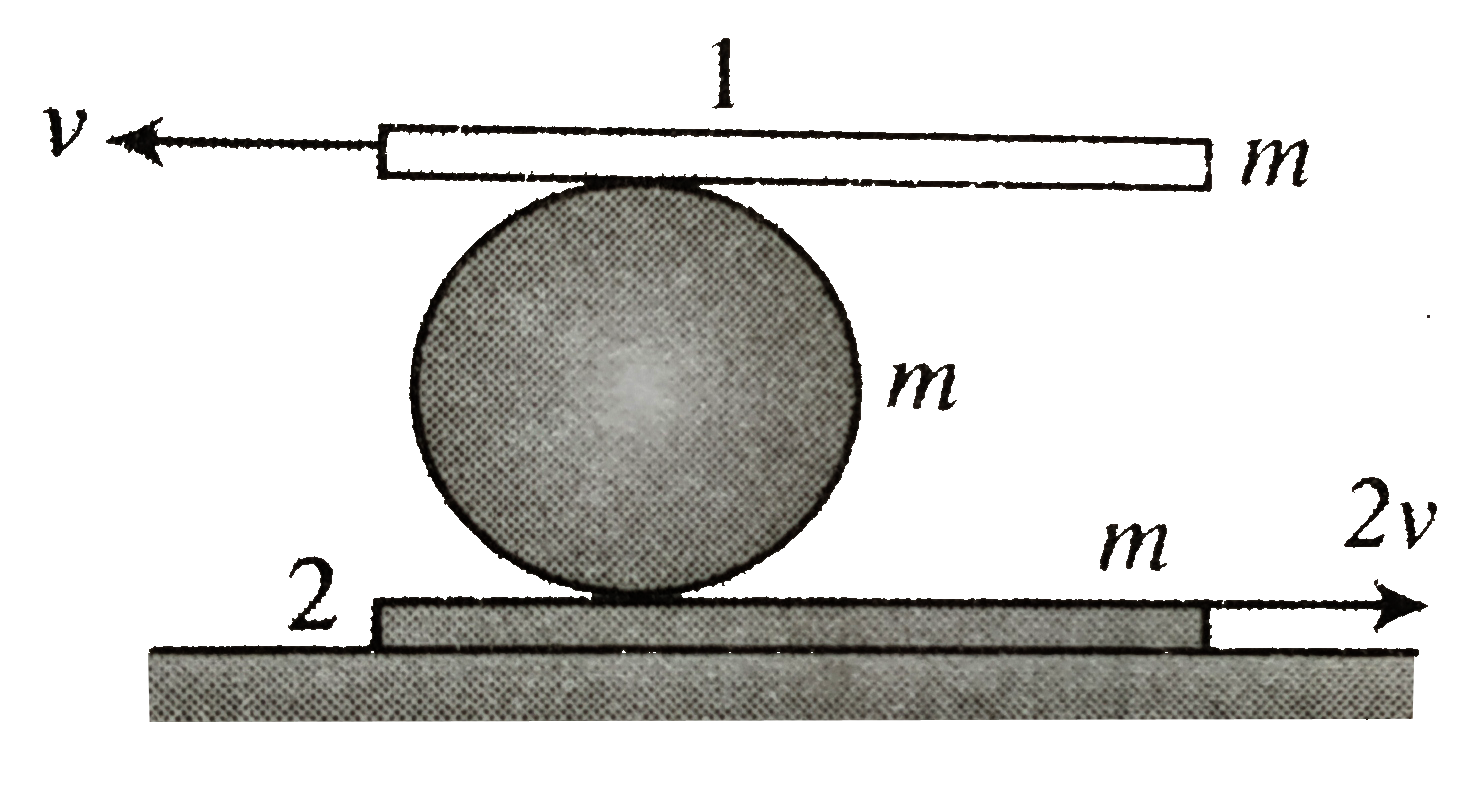
View SolutionTwo plates `1` and `2` move with velocities `-v ` and `2v` respectively. If the sphere does not slide relative to the plates, assuming the masses of each body as `m`, find the kinetic energy of the system (plates+sphere).
View SolutionDraw the direction of frictionn force in the followign cases. If rolling object is

View SolutionA force of magnitude `F` is acting on a rolling body of mass `m` and radius `R` as shown in figure. What happens if the pulling force `F` is removed ? Discuss the different posibilities when the pulling acts on the body.

a. At centre, b. Above centre, c. Below centre
View SolutionA force `F` acts tangentially at the highest point of a sphere `f` mass m kept on a rough horizontal plane. If the sphere rolls without slipping, find the acceleration of the centre of the sphere.

View SolutionA cotton reel has a inner radius `r` and outer radius `R`. Mass of the reel is `M` and moment of inertia about longitudinal rotational axis is `I`. A force `P` is applied at the free end of thread wraped of the reel as shown in fig. If the reel moves without sliding.

a. Determine the frictional force exerted by the table on the reel and the direction in which it acts.
b. In what direction does the reel begin to move?
View SolutionA wheel of radius `R`, mass `m` and moment of inertia `I` is pulled along a horizontal surface by application of force `F` to as rope unwinding from the axel of radius, `r` as shown in figure. Friction is sufficient for pure rolling of the wheel.
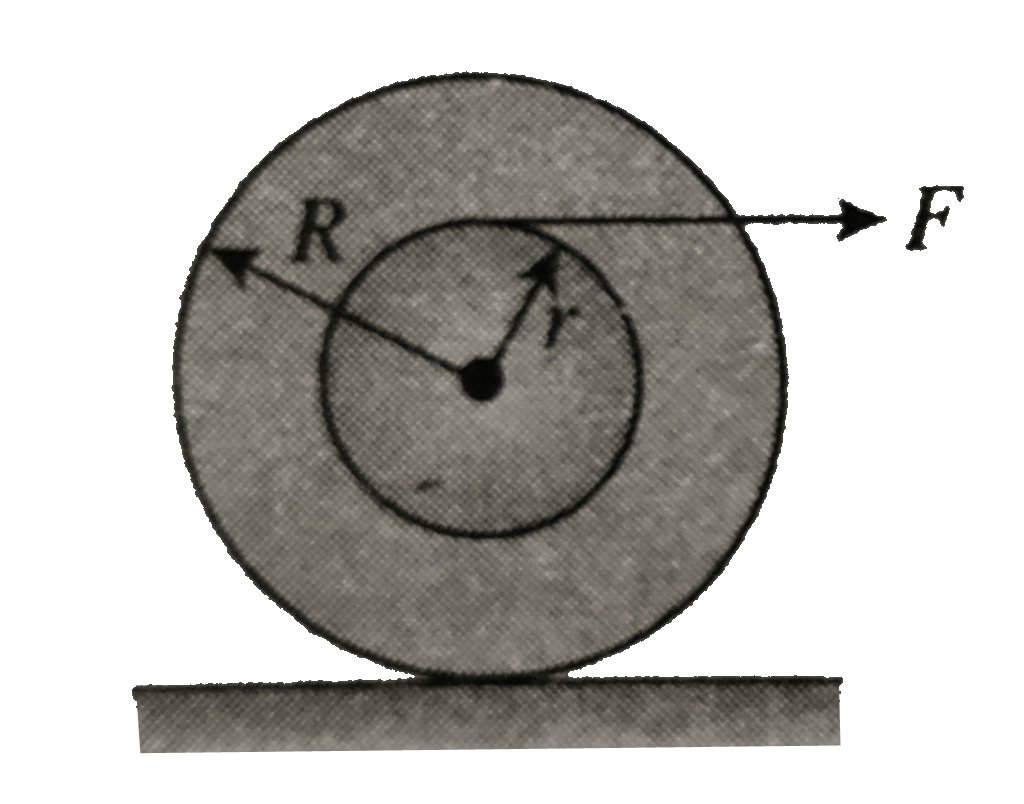
a. What is the linear acceleration of the wheel?
b. Calculate the frictional force that acts on the wheel.
View SolutionA force `F` is applied on a spool (mass `m` and radius `R`) in vertical direction as shown in case I and case II. The radius of gyration `k` of spool is `R//(sqrt(2))`. Assuming pure rolling, find the acceleration of the `CM` of the body, assuming it as a uniform cylinder of mass `m`.

View Solution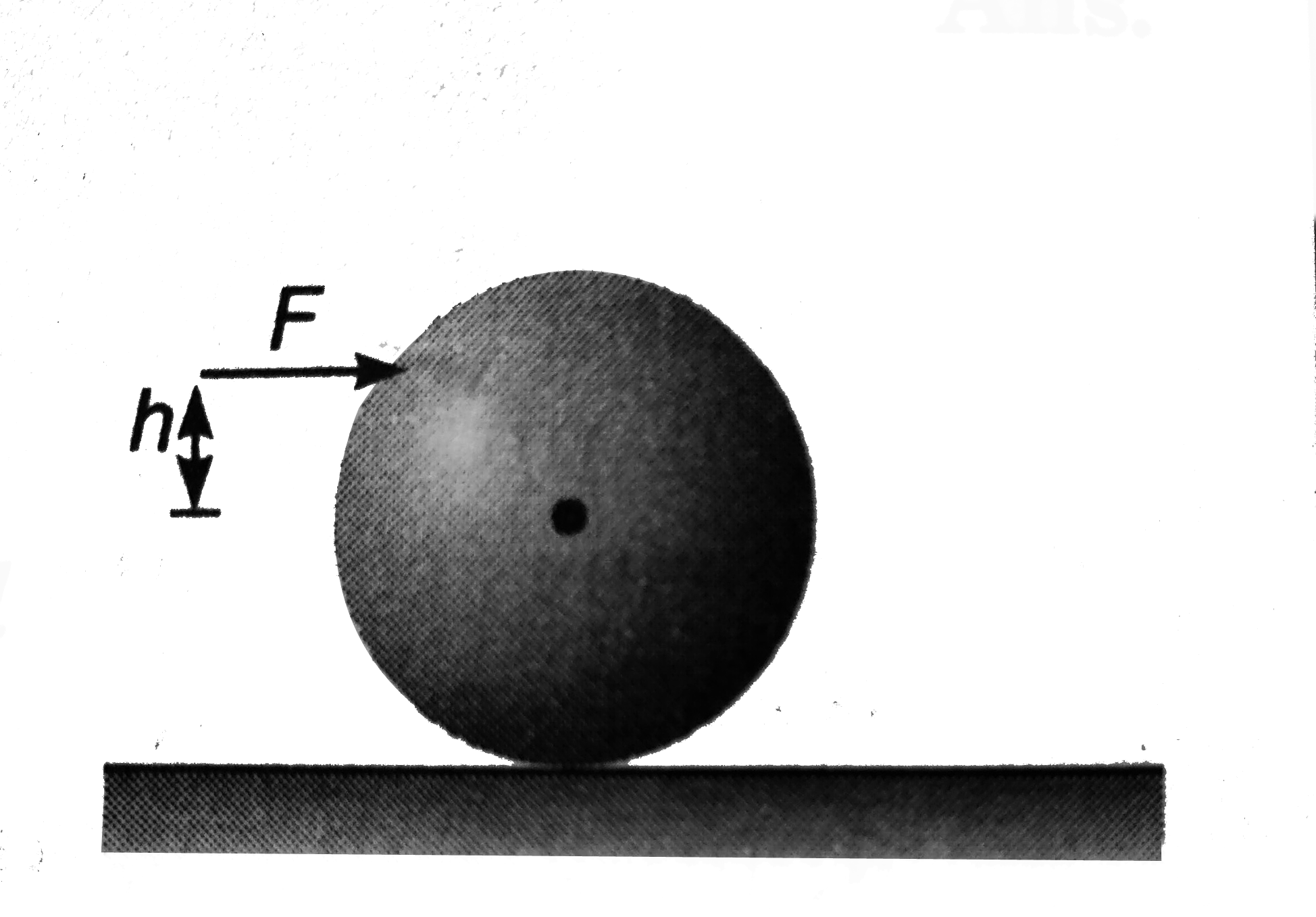
A billiard ball, initially at rest, is given a sharp impulse by a cue. The cue is held horizontally a distance `h` above the centre line as shown in figure. The ball leaves the cue with a speed `v_(0)` and because of its backward slipping eventually acquires a final
speed `(9)/(7)v_(0)` show that `h=(4)/(5)R`
Where `R` is the radius of the ball.
View SolutionA solid cylinder rolls down an inclined plane of height `h` and inclination `theta`. Calculate its speed to the bottom of the plane using acceleration method and energy method. Also calculate the time taken to reach of the bottom.
View SolutionA solid cylinder of mass `M` and radius `R` rolls without slipping down an inclined plane of length `L` and height `h`. What is the speed of its center of mass when the cylinder reaches its bottom
View SolutionA solid sphere is projected up along an inclined plank of inclination `theta=30^@` with a speed `v=2ms^(-1)`. If it rolls without slipping find the maximum distance travelled by it (`g=10 ms^(-2))`
View SolutionA uniform cylinder of radius `r` and radius of gyration is kept on a fixed wedge of inclination `theta`. When we push the wedge with a constant acceleration a, the cylinder rolls up the wedge. Find coefficient of friction between the cylinder and wedge.
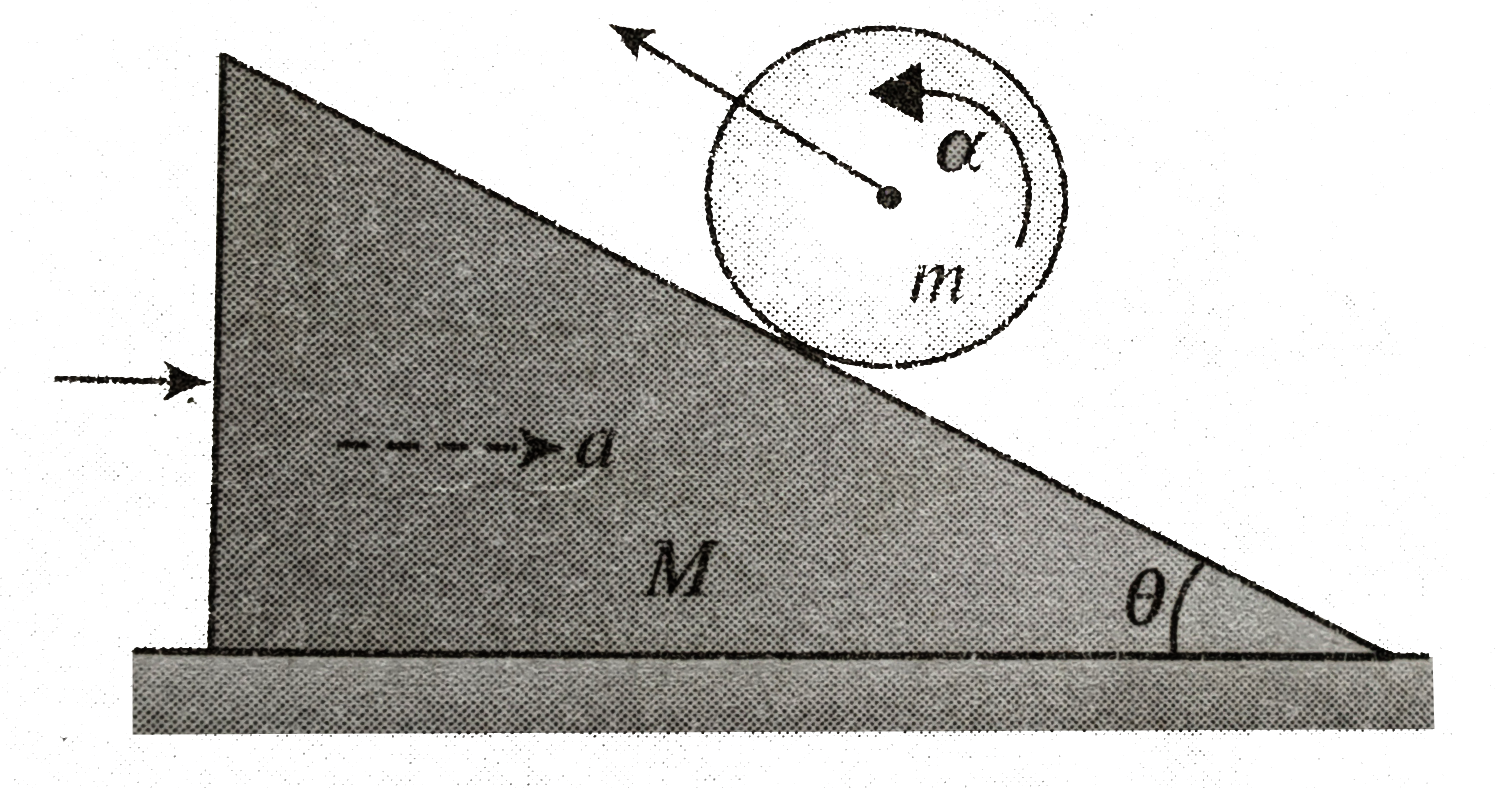
View SolutionA uniform rod of lengh `l` moving with a downward velocity `v_(0)` strikes a peg `P`. If the coefficient of restitutioin of impact is `e`, find the velocity of `CM` of the rod just after impact.

View SolutionA solid cube of wood of side `2a` and mass `M` is resting on a horizontal surface. The cube is constrained to rotate about an axis passing through `D` and perpendicular to face `ABCD`. A bullet of mass `m` and speed `v` is shot at a height of `4a//3` as shown in the figure. The bullet becomes embedded in the cube. Find the minimum value of `v` required to topple the cube. Assume `mgt gtM`.

View SolutionA particle of mass `m=1 kg` collides with the end with velocity `v_(0)=6 ms^(-1)` of a spinning rod of `mass 2m` and length `l=1 m` at the end of he rod. If the coefficient of restitution of collision `e=(2//3), ` find the
a. velocity of the particle
b. angular velocity of the rod just after the impact.
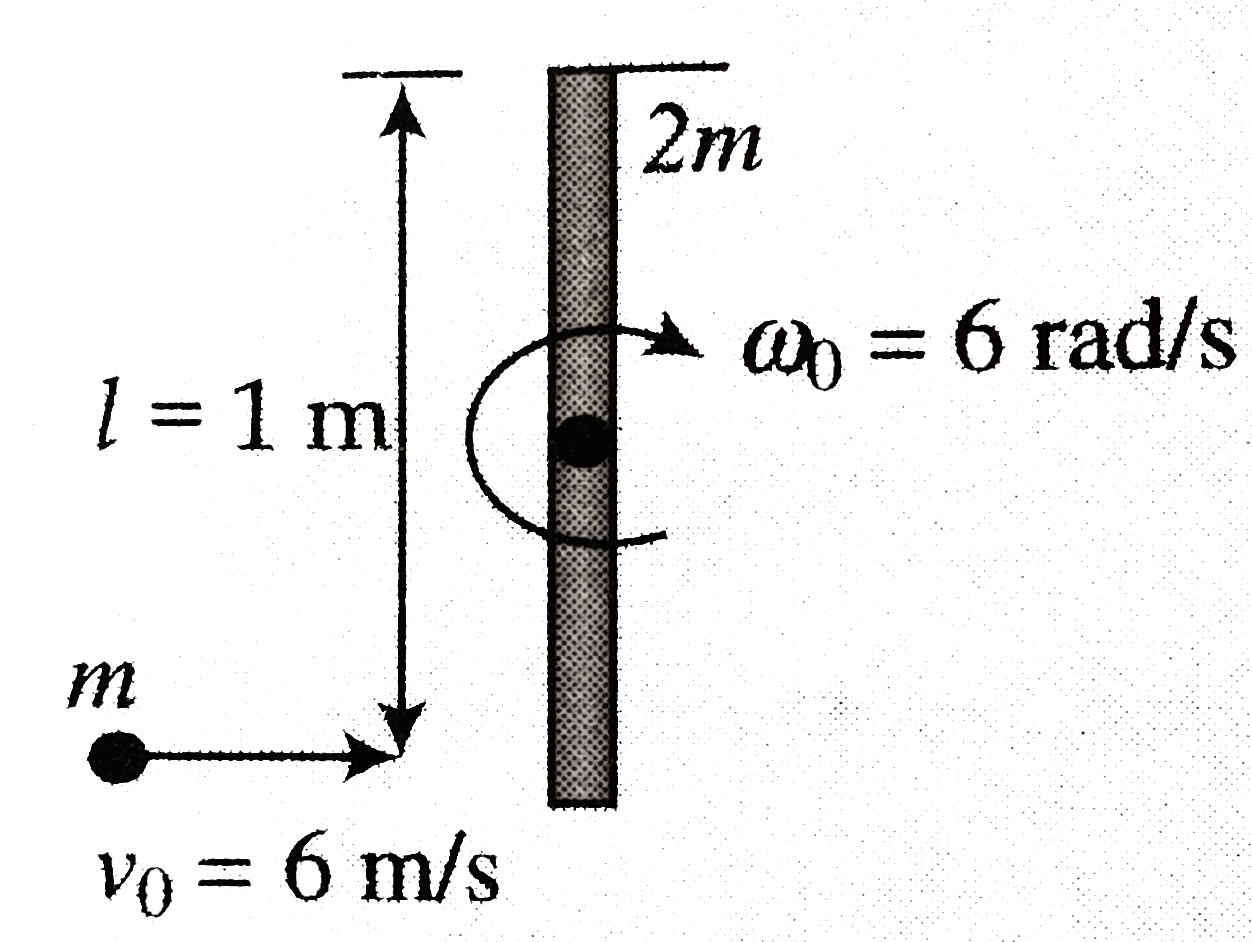
View SolutionA ball of mass `m` collides elastically with a smooth hanging rod of mass `M` and length `l`.
a. If `M=3m` find the value of `b` for which no horizontal reaction occurs at the pivot.
b. For what value of `m//M` the ball falls dead just after the collision assuming `e=(1/2)`?

View SolutionTwo particles `A` and `B` of mass `m` each hit the ends of a rigid bar of mass `M` and length `l` in-elastically from opposite sides the speeds `v` and perpendicular to the rod. The bar is kept on a smooth horizontal plane (as shown). Find the linear and angular speed of the system (bar`+`particle).

View SolutionA vertically oriented uniform rod of mass `M` and length `l` can rotate about its upper end. A horizontally flying bullet of mass `m` strikes the lower end of the rod and gets stuck in it as a result the rod swings through an angle `alpha`. Assuming that `mlt ltM`. find
a. the velocity of the flying bullet:
b. the moment change in the system bullet rod during the impact: what causes that change of momentum.
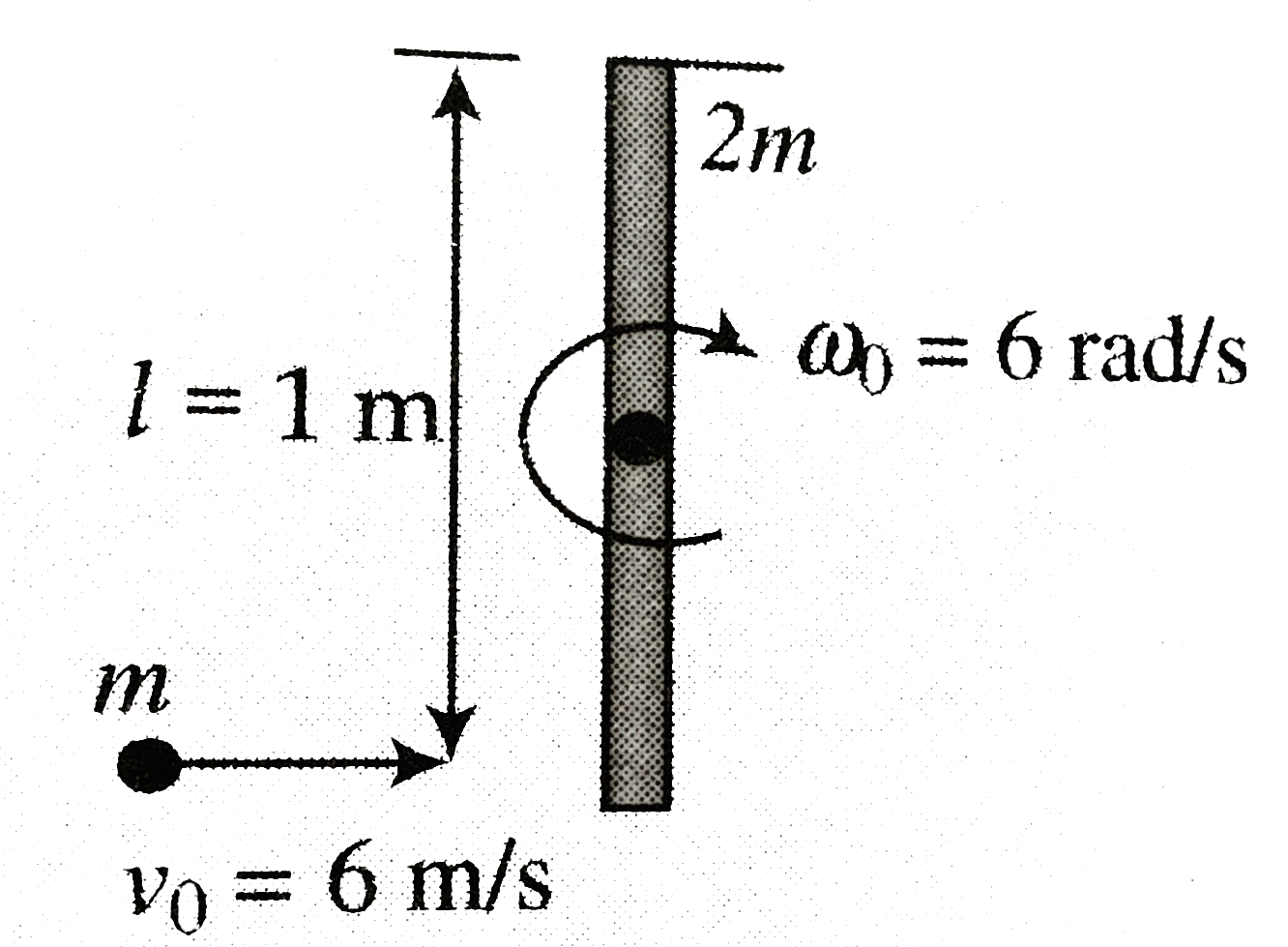
View SolutionA rod AB of mass `M` and length L is lying on a horizontal frictionless surface. A particle of mass `m` travelling along the surface hits the end A of the rod with a velocity `v_(0)` in a direction perpendicular to AB. The collision in elastic. After the collision the particle comes to rest
(a). Find the ratio `m//M`
(b). A point P on the rod is at rest immediately after collision find the distance AP.
(c). Fid the linear speed of the point P a time `piL//3v_(0)` after the collision.
View Solution
Illustration questions and complete solutions for chapter RIGID BODY DYNAMICS 2 of CENGAGE PHYSICS (HINGLISH) of Class 11