Complete Solutions to unsolvedMCQ of chapter WORK, ENERGY AND POWER of Class 11 book with complete answers and questions
| Class 11 | PHYSICS |
| Chapter | WORK, ENERGY AND POWER |
| PHYSICS GALAXY | unsolvedMCQ |
| Number Of Questions | 78 |
| Academic Year | 2023-24 |
An astronaut with space suit has a madd of 110 kg Climbing up a hill 7.3 m high in `7.2` sec required the astronaut to expend a power of 200 W. is the astronaut on the earth ?
View SolutionIn an amusement park passengers riding in a Air racer are revolving around a tall steel tower. At top speed all of its planes fly at `60^(@)` bank, and about 50 m from the tower. In this position the support chains make an angle of `60^(@)` with the vertical Calculate the speed of the planes.
View SolutionA man is drawing water from a well with a bucket which leaks uniformly. The bucket when full weights 20 kg and when it arrives the top only half the water remains. The depth of the water is 20 m What is the work done ?
View SolutionA train of mass 100 metric tons is drawn up an incline of 1 in 49 at the rate of 36 kph by a engine. If the resistance due to friction be 10N per metric ton, calculate the power of the engine if the steam is shut off, how far will the train move before it comes to rest ?
View SolutionAn elevator has mass of 600 kg, not including passengers The elevator is designed to ascend at constant speed a vertical distance of 20 m in 15 sec. It is driven by a motor that can provide up to 30 hp to the elevator, what is the maximum number of passengers that can ride in the elevator ? Assume that an average passenger has a mass of 65 kg
View SolutionConsider a spring that does not obey Hook's law. One end of the spring is held fixed. When it is stretched or compressed by an amount x, the force it exert is `F=ax-bx+cx^(2)` Where `a=50N//m b=150N//m^(2)` and `c=5000N//m^(3)` How much work must be done to stretch this spring by 1m from its unstretched length ?
View SolutionAn object of mass 5 kg falls from rest through a vertical distance of 20 m and reaches a velocity of 10 m/s. How much work is done by the push of the air on the object ? `(g=10m//s^(2)).`
View SolutionA car of mass `M` accelerates starting from rest. Velocity of the car is given by `v=((2Pt)/(M))^((1)/(2))` where `P` is the constant power supplied by the engine. The position of car as a function of time is given as
View SolutionA highway curve with a radius of 750 m is banked properly for a car travelling 120 kph. If a 1590 kg car takes the turn at a speed of 230 kph, how much sideways force must the tires exert against the road if the car does not skid ?
View SolutionA cyclist rides along the circumference of a circular horizontal plane of radius `R`, with the friction coefficient `mu=mu_(0)(1-(r )/(R ))`, where `mu_(0)` is constant and `r` is distance from centre of plane `O`. Find the radius of the circle along which the cyclist can ride with the maximum velocity, what is this valocity?
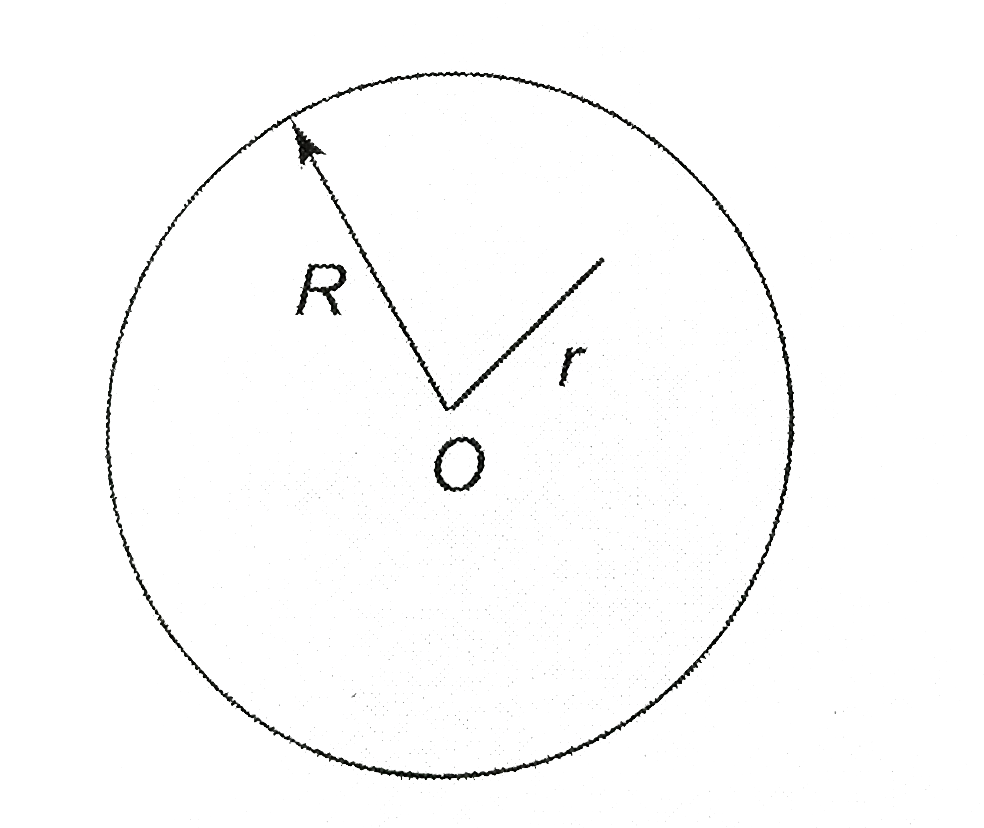
View SolutionAn annular circular brass disk of inner radius `'r'` and outer radius `'R'` is rotating about an axis passing through its centre and perpendicular to its plane with a uniform angular velocity `'omega'` in a uniform magnetic filed of induction `'B'` normal to the plane of the disk. The induced emf between the inner and outer edge of the annular disk is
View SolutionA small ball is suspended from a point O by a light thread of length l. Then the ball is drawn aside so that thread deviates through an angle `theta` from the vertical and set in motion in a horizontal direction at right angles to the vertical plane in which the thread is located. What is the initial velocity that has to be imparted to the ball so that it could deviate through the maximum angle `pi//2` in the process of motion?
View SolutionAn amusement park ride consist of a large verticle cylinder that spins about its axis first fast enough that any person inside is held up againest the wall when the is `mu_(s)` and the radius of the cylider is R

a. Shown that maximum period of revolation neccessary to keep the person from falling is `T = (4 pi^(2) mu_(s) //g)^(1//2)`
b. Obtain a numerical value for taking `R = 4.00m` and `mu_(s) = 0.400` . How many revolations per minute does the cylinder make?
c.If the rate of revolation of the cylinder is make to be some what larger what happens to the magnitude of each one of the forces acting on the person ? What happens to the motion of the person ?
d.If instead the cylinder rate revolation is made to be somewhat smaller what happens to the magnitude happens to the motion of the person ?
View SolutionA designer of race track is appointed on moon for a car race. He has to design a 1.5 km diameter circular race track assuming a speed of 240 `km//hr` for the type of cars used. If everything else is to stay the same in the design, by how many degrees and in which direction with respect to the horizontal designers will have to change the angle at which the track is banked on the moon as compared to the banking angle on earth ? The acceleration of gravity on the moon is `1.62m//s^(2)`
View SolutionThe kinetic energy of a particle moving along a circle of radius `R` depends on the distance covered `s` as `K=lambdas^(2)`, where `lambda` is a constant. Find the force acting on the particle as a function of `s`.
View SolutionTwo bars of masses `m_(1)` and `m_(2)` connected by a non`-`deformed light spring rest on a horizontal plane. The coefficient of friction between the bars and the surface is equal to `mu`. What minimum constant force has to be appled in the horizontal direction to the bar of mass `m_(1)` in order to shift the other bar?
View SolutionShow that the height h to which a man of mass m can jump is given approximately by `h=1/(2g)((4sP)/m)^(2//3)` where P is the maximum power, the man can use and s is the height of centre of mass of man from ground
View SolutionA string of length 1m is fixed at one end and carries a mass of 1 kg at the other end. The string makes `2//pi` revolutions per second around a vertical axis passing through the fixed end. Calculate (i) angle of inclination of the string with the vertical, (ii) the tension in the strong and (iii) the linear velocity of the mass.
View SolutionLight airplanes are design so that their wings can safely provide a lift force of 3.8 times the weight of the airplane. What is the maximum bank angle that a pilot can safely maintain in a constant altitude turn without threatening the safety of the airplane ?
View SolutionA stone with a mass of 0.9 kg is attached to one end of a string 0.8 m long. The string will break if its tension exceeds 500 N. The stone is whirled in a horizontal circle on a frictionless table top. The other end of the string is kept fixed. Find the maximum speed of the stone, it can attain without breaking the string.
View SolutionThe block on the loop shown slides without friction. At what height from A it starts so that it passes against the track at B with a net upward force equal to its own weight? The radius of the loop is R.
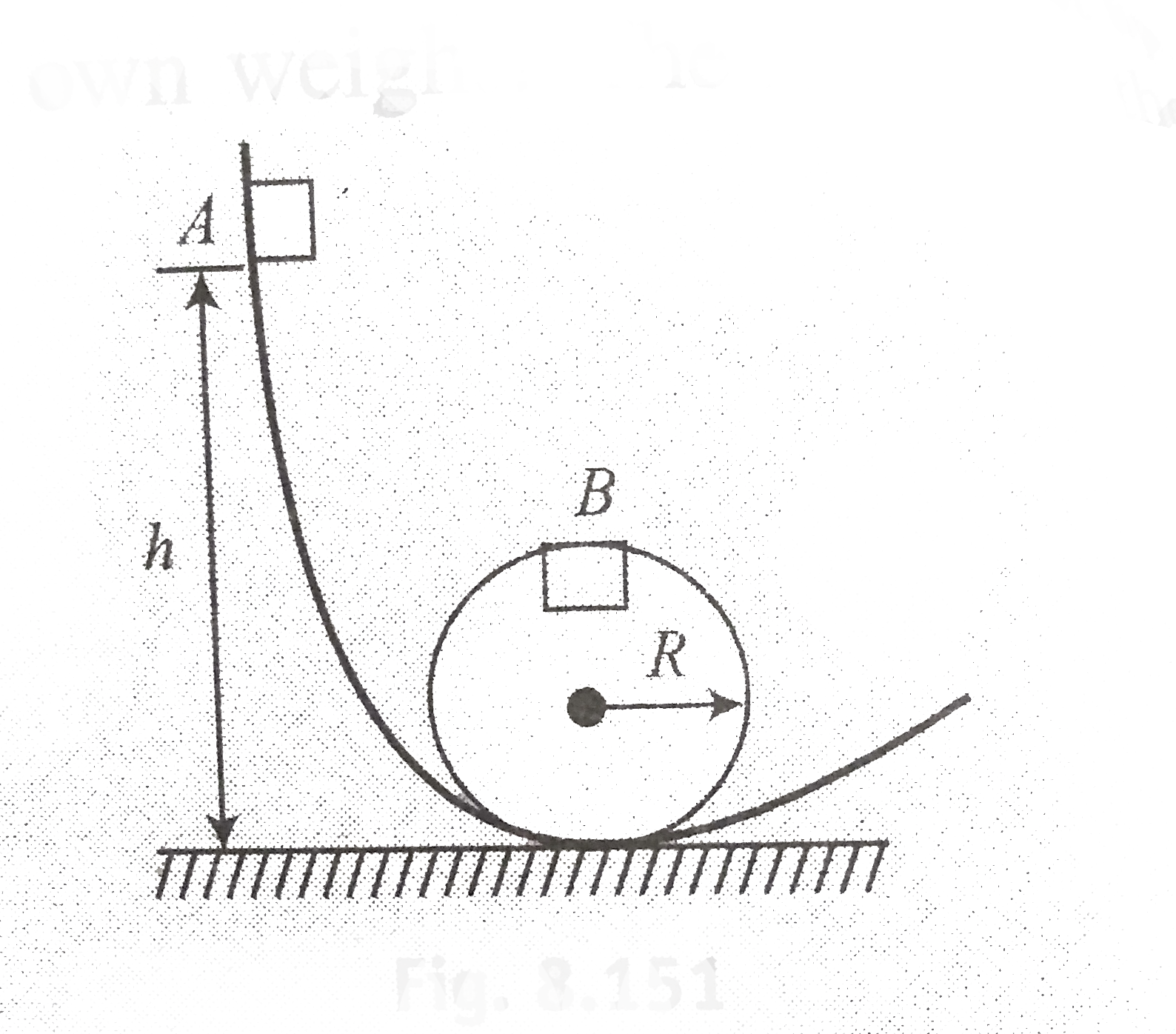
View SolutionA body of mass m was slowly hauled up the hill as shown in the fig. by a force F which at each point was directed along a tangent to the trajectory. Find the work performed by this force, if the height of the hill is h, the length of its base is I and the coefficient ot friction is `mu`.
 .
.
View SolutionAn object is attached toward the origin with a force given by `F=-k//x^(2)` Calculate the work done by the force F when the object moves in the x - direction from `x_(1)` to `x_(2)` if `x_(2) gt x_(1)` is the work done by F positive or negative ? You now exert a force with your hand to move the object slowly from `x_(1)` or `x_(2)`
View SolutionA hemispherical bowl of radius R is rotated about its axis of symmetry which is kept vertical.A small block is kept in the bowl at a position where the radius makes an angle `theta` with the vertical. The block rotates with the bowl without any slipping. The friction coefficient between the block and the bowl surface is `mu`. Find the range of the angular speed for which the block will not slip.
View SolutionA system consists of two springs connected in series and having the stiffness coefficients `k_1` and `k_2`. Find the minimum work to be performed in order to stretch this system by `Deltal`.
View SolutionAircraft experience a lift force (due to air) that is perpendicular to the plane of the wings A small airplane is flying at a constant speed of 280 kph At what angle from the horizontal must the wings of the airplane be tilted for the plane to execute a horizontal turn from east to north with a turning radius of 1200 m ?
View SolutionTwo identical twins, Tui and Kui are playing on a large merry go round in an amusement park. The surface of merry go round is frictionless and is turning at a constant rate of revolution as the twins ride on it. Tui, sitting 2 m from the center of the merry go round, must hold onto one of the metal posts attached to the merry go round with a horizontal force of 90N to keep from sliding off. Kui is sitting at the edge, 4m from the center. With what horizontal force must she hold on to keep form falling off ?
View SolutionA particle of mass 'm' is moving in horizontal circle inside a smooth inverted fixed vertical cone above height 'h' from apex. Angle of cone is `theta`, then
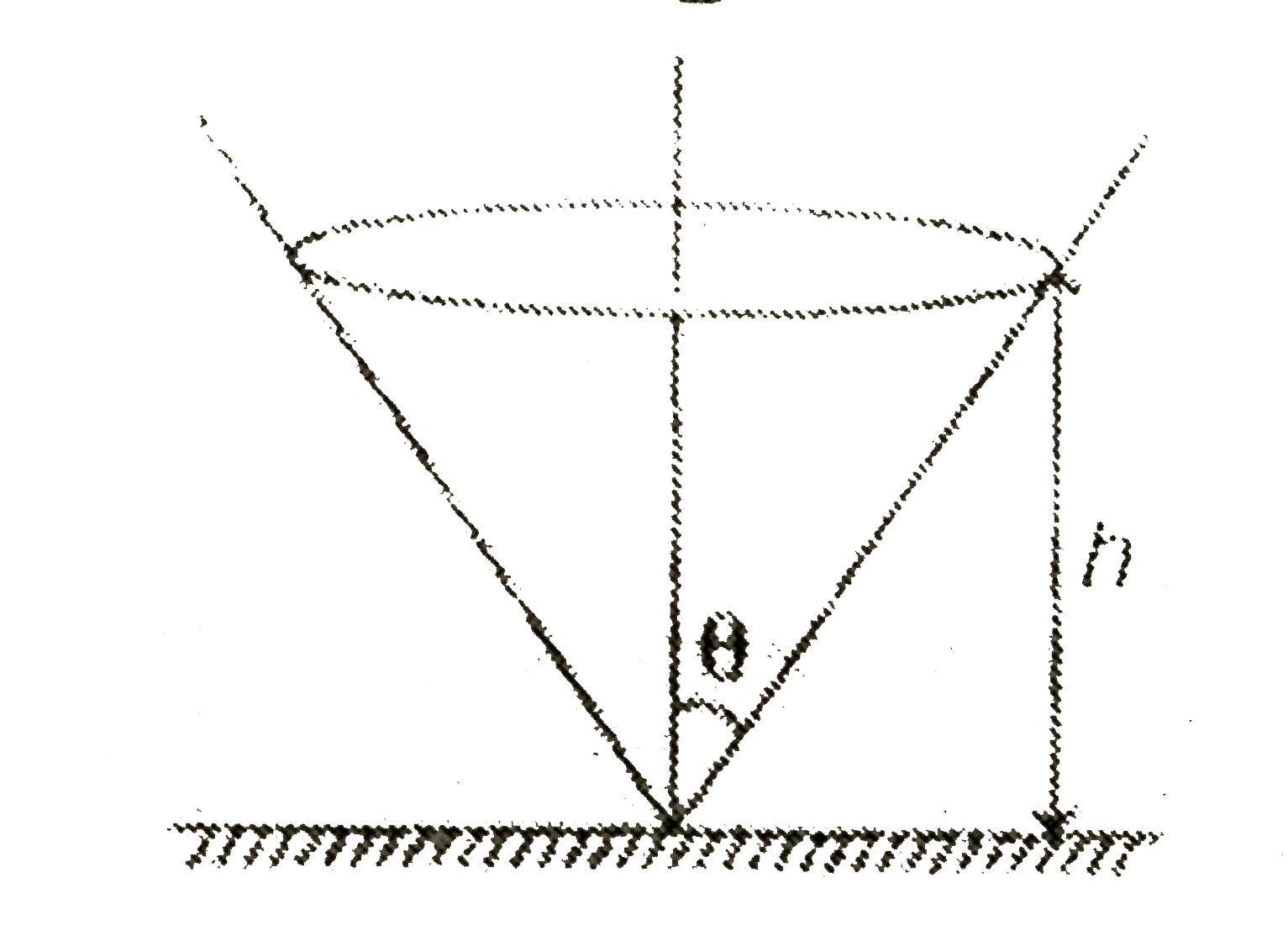
View SolutionAn object is attracted toward the origin with a force of magnitude `F=kx^(3)` where `k=5 N//m^(3)` How much work is done by the force F when the object moves from 1 m to 2 m? is the work positive or negative
View SolutionA designer proposes a design for an automobile crash barrier in which a 1200 kg car moving at 20 `m//s` crashes into a spring of negligible mass that slows the car to a stop. To avoid injuring the passengers, the acceleration of the car as it slow can be no more than 5 g find the required spring constant and find the distance the spring will compress slowing the car to a stop
View SolutionWhat power is developed by a grinding machine whose wheel has a radius of 20 cm and runs at 2.5 revolutions per second when the tool to be sharpened is held against the wheel with a force of 20 kg ? The coefficient of friction between the tool and the wheel is 0.3
[Hint : Power `= Fv = F omega r = mu N omega r` where N is the normal reaction]
View SolutionA particle moving along the x- axis is subjected to a force given by `F=F_(0)(e^(x//a)-1)` where `F_(0)` and are constants Determine an expression for the work done by this force as the particle moves from the origin to the point x=r
View SolutionDuring 0.19 sec, a wheel rotates through an angle of 2.36 rad as a point on the peribhery of the wheel moves with a constant speed of `2.87m//s` What is the radius of the wheel ?
View SolutionOne end of a light string is slipped around a peg fixed in a horizontal table top, while the other end is tied to a 0.5 kg small disc. The disc is given an initial velocity of magnitude `3.4m//s` so that it moves in a horizontal circle of radius 0.75 m. The object comes to rest after 2.5 revolutions (a) For the entire motion, what work is done by the frictional force ? (b) Assume that the magnitude of the frictional force is constant and determine the coefficient of kinetic friction at the interface (c) Determine the tension in the string at the instant that the disc completes the first revolution (d) How much work is done by the tension in the string ?
View SolutionA ball of mass m is attached to a light string of length L and suspended vertically. A constant horizontal force, whose magnitude F equals the weight of the ball, is applied Determine the speed of the ball as it reaches the `90^(@)` level.
View SolutionA smooth table is placed horizontally and a spring of unstretched length `i_(0)` and force constank k has one end fixed to its center. To the other end of the spring is attached a mass m which is making n revolutions per second around the center. Show that the radius r of this unifrom circular motion is `kl_(0)//(k-4 pi^(2)mn^(2))` and the tension T in the spring is `4pi^(2)mkl_(0)n^(2)//(k-4pi^(2)mn^(2))`
View SolutionSuppose that a man (60 kg) is standing in an elevator. The elevator accelerates upward from rest at `1m//s^(2)` for 2 sec then for further 10 sec it moves with the constant velocity and then decelerates at the same rate for 2 sec
(a) For the whole motion, how much work is done by the normal force on the man by the elevator floor?
(b) By man's weight
(c) what average power is delivered by the normal force for the whole motion
(d) What instantaneous power is delivered by the normal force at 7 sec ?
(e) at 13 sec ?
View SolutionIn an industry a 300 kg crate is dropped vertically from a packing mechine onto a conveyor belt moving at `1.2 m//s` The coefficient of kinetic friction between belt and crate is 0.4 After a short time, slipping between the belt and the crate ceases and the crate then moves along with the crate ceases and the crate then moves along with the belt . For the period of time during which the crate is being brought to rest relative to the belt, calculate, for a reference frame (a) the kinetic energy supplied to the crate (b) the magnitude of the kinetic frictional force acting on the crate, the magnitude of energy supplied by the motion (c) Why are the answers to (a) and (c) different
View SolutionA bicyclist of mass 80 kg (including the bicycle) can coast down a `3.4^(@)` hill at a steady speed of 6 kph Pumping hard, the cyclist can descend the hill at a speed of 30 kph Using the same power, at what speed cant he cyslist climb the same hill ? Assume the force of friction is directly proportional to the speed v.
View SolutionAn engineer is designing a spring to be placed at the bottom of an elevator shaft, if the elevator cable should break at a height h above the top of the spring, calculate the required value of the spring constant k, so that passsengers undergo an acceleration of no more than 10 g when brought to rest, Let M be the total mass of the elevator and passengers
View SolutionA man finds that, on level ground, his 800 kg car accelerates from rest to `15m//s` in 10 sec and then coasts to rest from `15m//s` in 500 m Compute the average horsepower delivered by the car.
View SolutionA child's 200gm toy car is driven by an electric motor that has a constant output power. The car climb a `20^(@)` incline at `20cm//s` and can travel on a horizontal table at `40 cm//s` The friction force retarding it is kv, where k is a constant and v is its speed. How steep in incline can it climb with a speed of `30 cm//s` ?
View SolutionA solid body rotates about a stationary axis so that its angular velocity depends on the rotation angle `varphi` as `omega=omega_0-avarphi`, where `omega_0` and a are positive constants. At the moment `t=0` the angle `varphi=0`. Find the time dependence of
(a) the rotation angle,
(b) the angular velocity.
View SolutionThe bob of a simple pendulum of length l is released from a point in the same horizontal line as the point of suspension and at a distance l from it Calculate the tension in the string at the lowest point of its swing
(b) If the string of the pendulum is catched by a nail located verticaly below the point of suspensions and the bob just swings around a complete circle around the nail, find the distance of the nail from the point of suspension
(c) If the string of the pendulum is made of rubber then show that it will be stretched by 3 `mg//k` on reaching the bob at the lowest point. Here k is the force constant of the string
View SolutionThe resistance to motion of an automobile depends on road friction, which is almost independent of speed, and on air drag, which is proportional to speed squared. For a car with a weight of 12000 N, the total resistance force F is given by `F=300+1.8v^(2)` where F is in newtons and v is in meters per second. Calculate the power required to accelerate the car at `0.92 m//s^(2)` when the speed is 80 kph
View SolutionA pendulum bob can swing along a ciircular path on a smooth inclined plane, as shown in Fig. 3E.31, where `m = 1.2 kg, 1 = .075m, theta = 37^(@)`. At the lowest point of the circle the tension in the sting is `T = 110 N`. Determine
(a) the speed of the bob at the lowest point,
(b) the speed of the bob at the highest point on the circle and
(c) the tension in the string at the highest position
(a)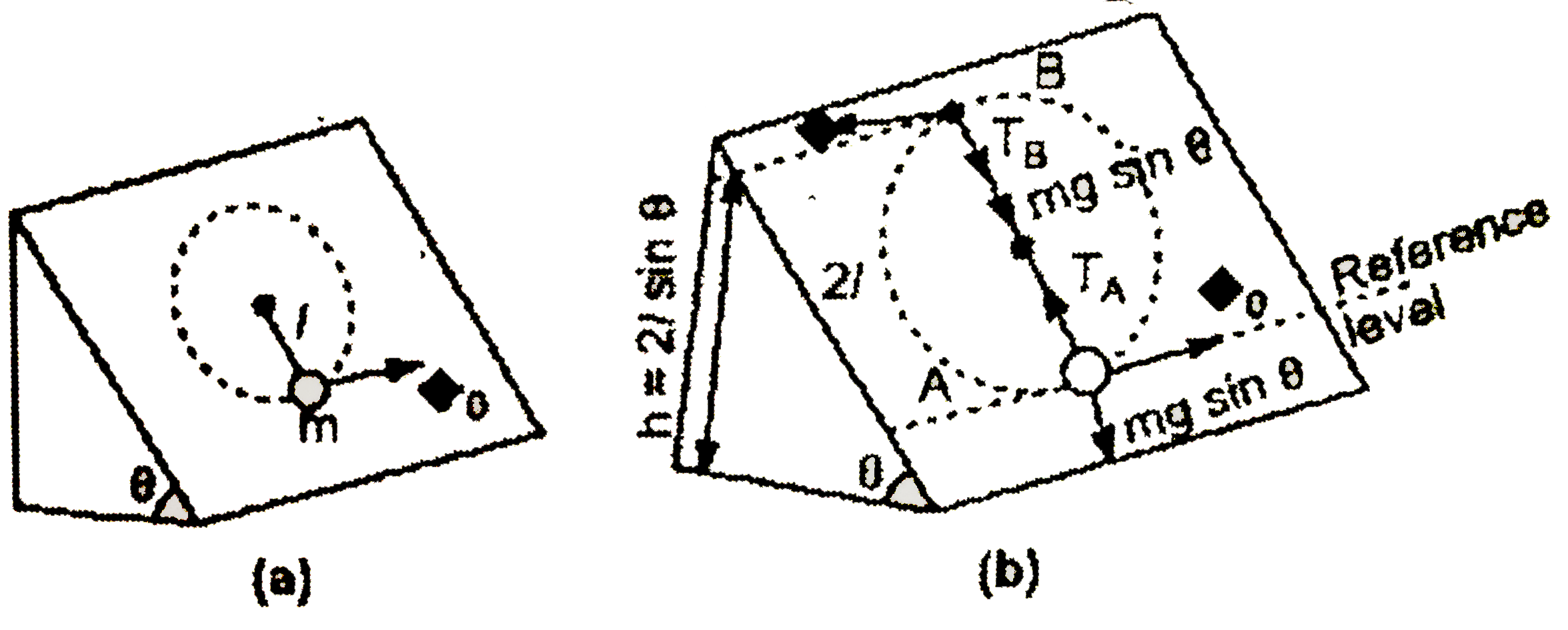
View SolutionA particle of mass 9 kg is moving under the action of a central force whose potential energy is given by `U=10//r` For what energy it will orbit a circle of radius 10 m ? Calculate the time period of this motion
View SolutionA smooth light horizontal rod AB can rotate about a vertical axis passing through its end A. The rod is fitted with a small slieeve of mass m attached to the end A by a weightless spring of length `l_0` and stiffness `x`. What work must be performed to slowly get this system going and reaching the angular velocity `omega`?
View SolutionA particle 'A' moves along a circle of radius R = 50 cm, so that its radius vetor 'r' relative to the point O rotates with the constant angular velocity `omega = 0.4 rad//s`. Then :
View SolutionA particle moves in the plane `xy` with velocity `v=ai+bxj`, where i and j are the unit vectors of the x and y axes, and a and b are constants. At the initial moment of time the particle was located at the point `x=y=0`. Find:
(a) the equation of the particle's trajectory `y(x)`,
(b) the curvature radius of trajectory as a function of x.
View SolutionA shell acquires the initial velocity `v=320m//s`, having made `n=2.0` turns inside the barrel whose length is equal to `l=2.0m`. Assuming that the shell moves inside the barrel with a uniform acceleration, find the angular velocity of its axial rotation at the moment when the shell escapes the barrel.
View SolutionA small object slides without friction from the height `5R//2` and then loops of the verticle loop of radius `R` from which a symmetrical section of angle `2alpha` has been removed. Find angle `alpha` such that after losing contact at `A` and flying through the air, the object will reach point `B`.

View SolutionA point moves along a circle with a speed `v=kt` , where `k=0.5m//s^(2)`
Find the total acceleration of the point the moment when it has covered the `n^(th)` fraction of the circle after the begining of motion, where `n=(1)/(10)` .
View Solution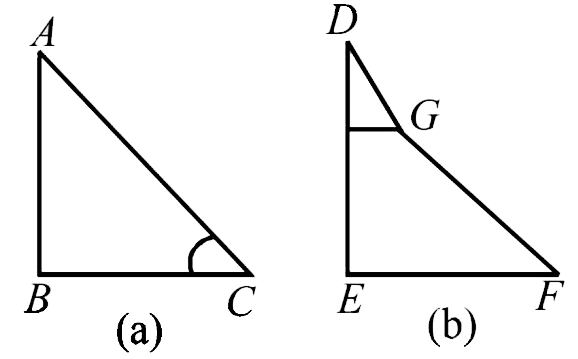
In the figure (a) and (b) `AC` and `GF` are fixed inclined planes `BC = EF = x` and `AB = DE = y` A small block of mass `M` is rdeased from the point `A` it sides down `AC` and maches `C` with a speed `V_(C) ` The same block is relessed from rest from the point `D` it sides down `DGF` and reached the point the `F` with `V_(p) THe coefficients of kiletic friction between the block and the sarface `AC` and `DGF` are `mu` colculate `V_(C) ` and V_`(p)`
View SolutionAB is a quarter of smooth circular track of radius `R=6m`. A particle P of mass `0.5kg` moves along the track from A to B under the action of the following forces.
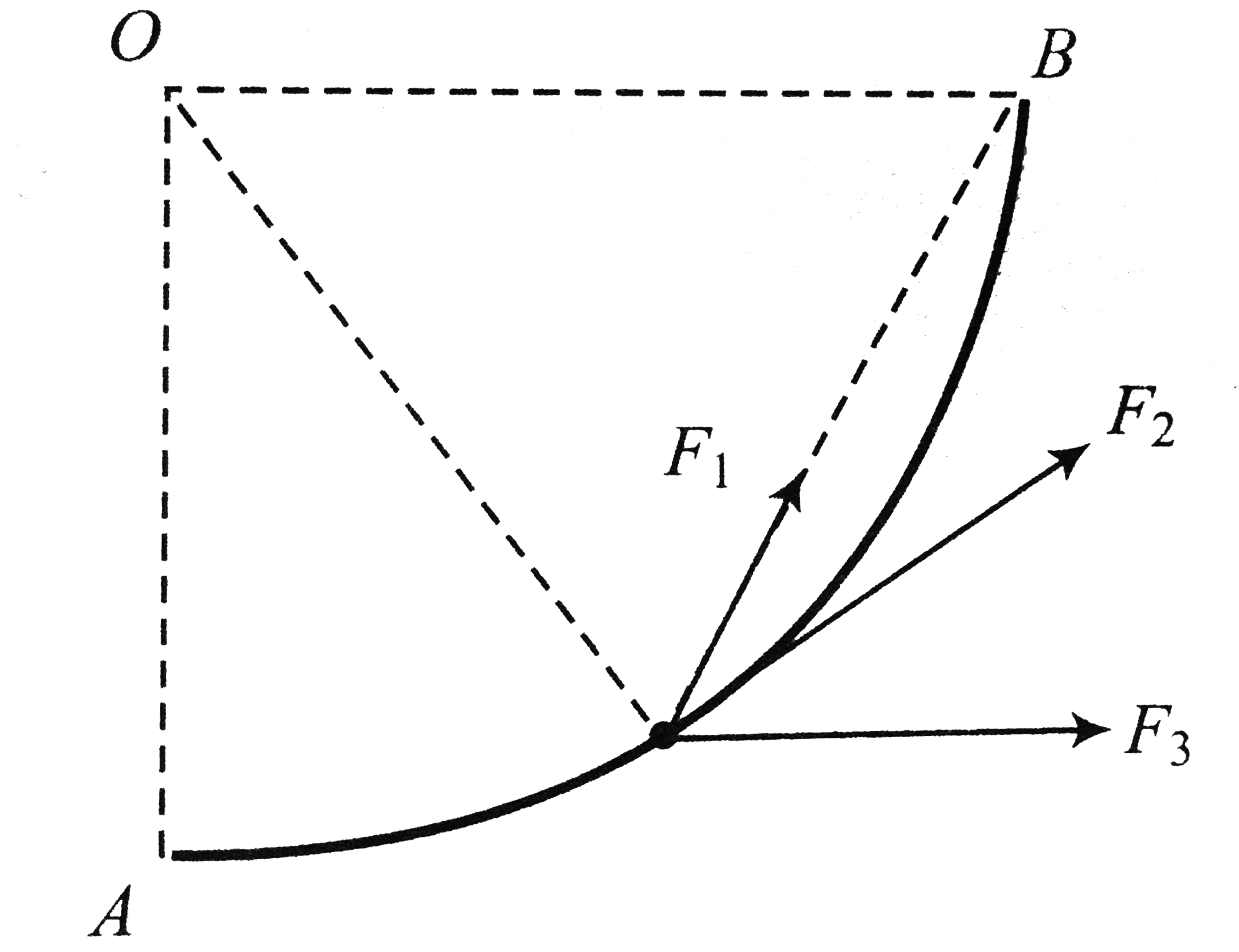
a. A force `F_1` directed always towards the point B, its magnitude is constant and is equal to `20N`.
b. A force `F_2` directed along the instantaneous tangent to the circular track, its magnitude is `(15-10S)N`, where S is the distance travelled in metre.
c. A horizontal force of magnitude `30N`.
Find the work done by forces mentioned in (a), (b) and (c)
View SolutionA `20 g` bullet pierces through a plate of mass `M_(1) = 1 kg` and then comes to rest inside a second plate of mass `M_(2) = 2.98 kg` as shown in Fig. It is found that the two plates, initially at rest, now move with equal velocities. Find the percentage loss in the initial velocity of the bullet when it is between `M_(1)` and `M_(2`. Neglect any loss of material of the plates due to the action of bullet.
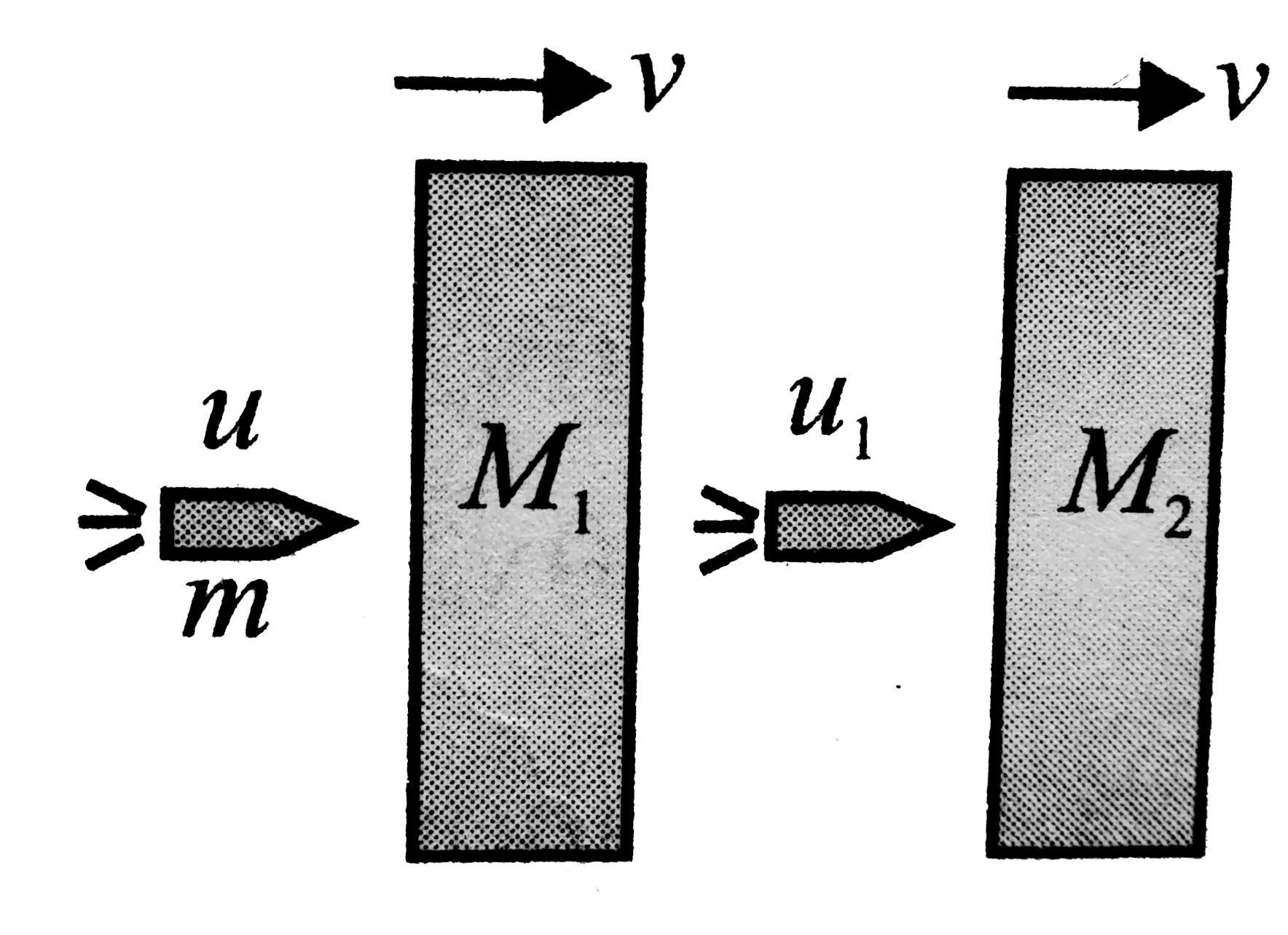
View SolutionA 100 metric ton engine is moving up a slope of gradient `5^(@)` at a speed of 100 metre`//` hour. The coefficient of friction between the engine and the rails is 0.1. If engine has an efficiency of `4%` for converting heat into work, find the amount of coal the engine has to burn in one hour. Burning of 1 kg coal yields 50000J.
View SolutionA particle is projected with a speed u at angle `theta` with the horizontal. Consider a small part of its path near the highest position and take it approximately to be a circular arc. What is the radius of this circle? This radius is called the adius of curvature of the curve at the point.
View SolutionA string with one end fixed on a rigid wall, passing over a fixed frictionless pulley at a distance of `2m` from the wall, has a point mass M of `2kg` attached to it at a distance of `1m` from the wall. A mass `m` of `0.5kg` is attached to the free end. The system is initially held at rest so that the stirng is horizontal between wall and pulley and vertical beyond the pulley as shown in figure.
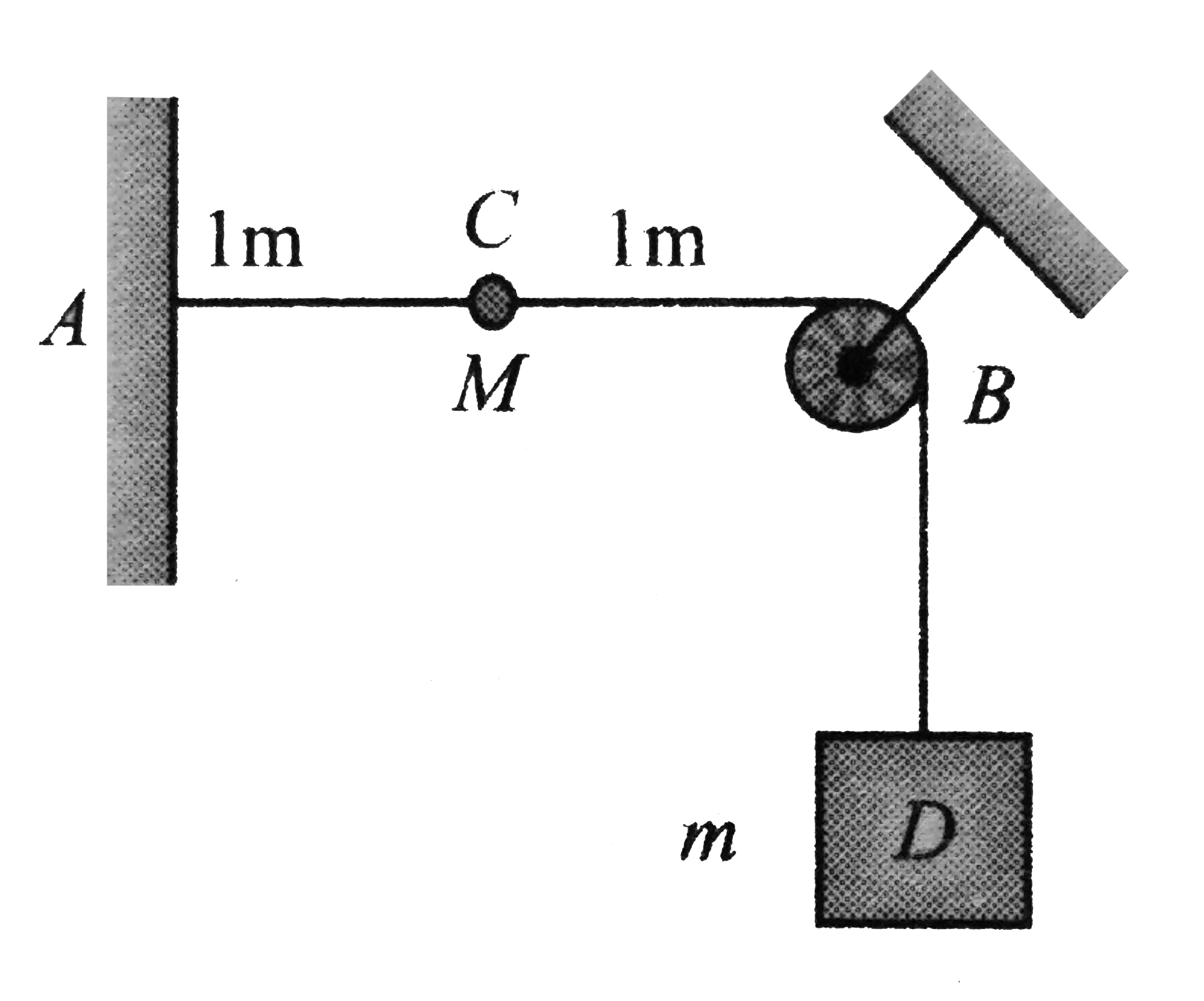
What will be the speed with which point mass M will hit the wall when the system is released? `(g=10ms^-2)`
View SolutionParticle slides down the surface of a smooth fixed sphere of radius r starting from rest at the highest point. Find where it will leave the sphere. Show that it strike the horizontal plane through the lowest point of the sphere at a distance equal to `5[sqrt(5)+4sqrt(2)](r//27)` from the vertical diameter.
View SolutionA heavy particle is suspended by a light thread, the other end of the thread being fixed to a point O. The particle is projected from its lower position in the horizontal direction in the vertical plane through O, with such a velocity that it leaves the circular path after an angular displacement `theta` Show that when the string again becomes taut it makes an angle `3theta-360^(@)` with the downward drawn vertical.
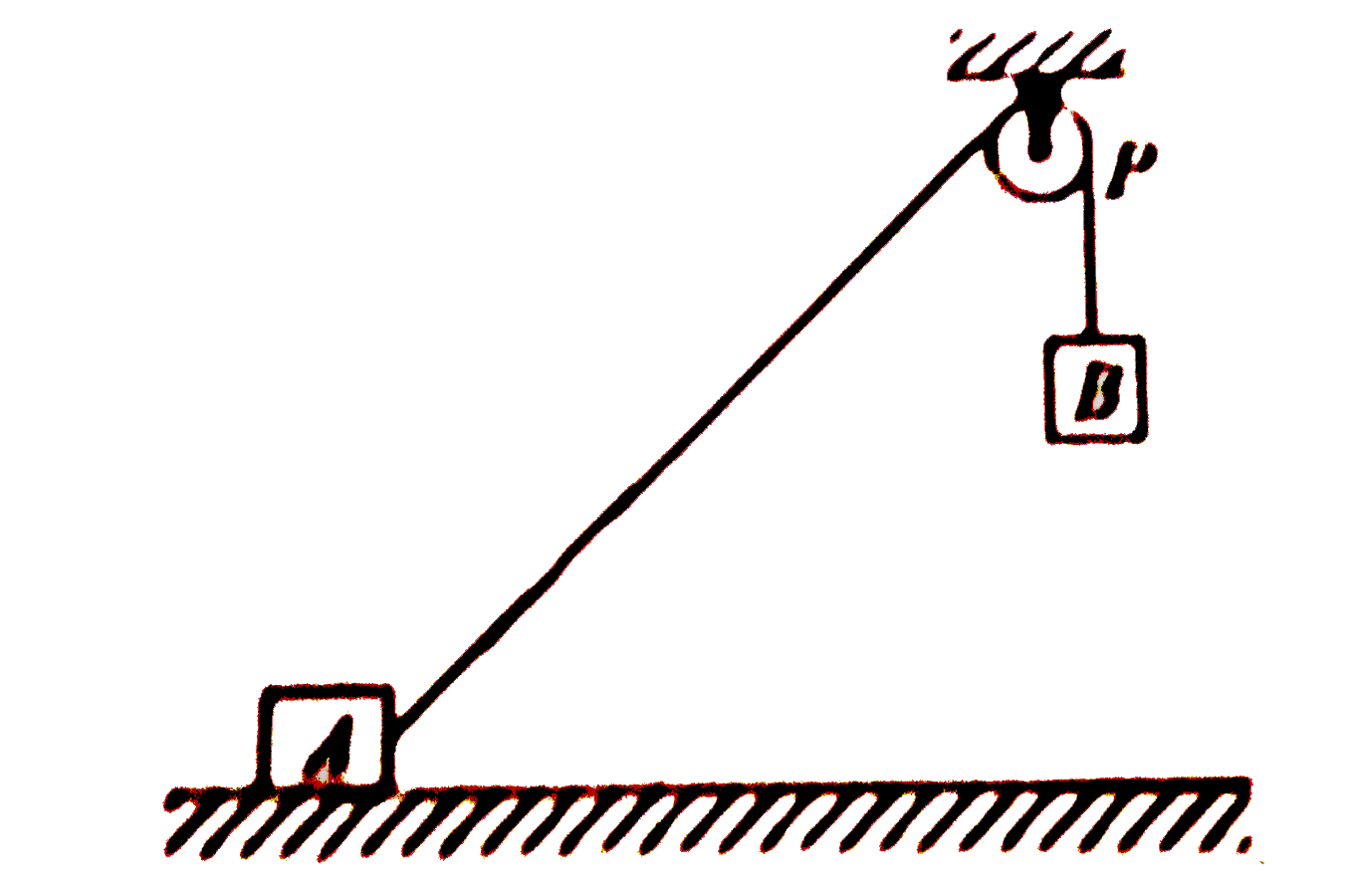
View SolutionA block of mass m is held at rest on a smooth horizontal floor. A light frictionless, small pulley is fixed at a height of 6m from the floor. A light inextensible string of length 16m, connected with A passes over the pulley and another identical block B is hung from the string initial height of B is 5m from the floor as shown in figure -3.130 When the system is released from rest, B starts to move vertically downwards and A slides on the floor towards right
(a) if at an instant string makes an angle `theta` with horizontal, calculate relation between velocity u of A and v of B
(b) Calculate c when B strikes the floor.
View SolutionA smooth rubber cord of length `l` whose coefficient of elasticity is k is suspended by one end from the point O (figure). The other end is fittetd with a catch B. A small sleeve A of mass m starts falling from the point O. Neglecting the masses of the thread and the catch, find the maximum elongation of the cord.
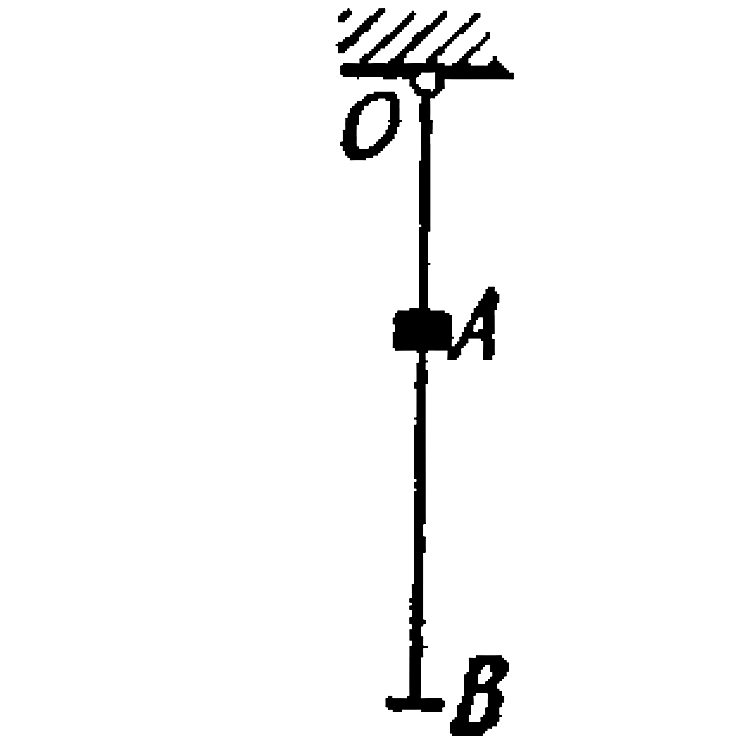
View SolutionThe basket in a front loading automatic clothes dryer rotates about a horizontal axis. The basket rotates so that the force exerted by the basket on clothes located at the basket's edge is zero at the top of the path. If the radius of the basket is 0.65 m, how fast must the basket turn to accomplish this ?
View SolutionA ring of radius R is made from a thin wire of radius r. If `rho` is the density of the material of wire then what will be the gravitational force exerted by the ring on the material particle of mass m placed on the axis of ring of ring at a distance x from its centre. Shown that the force will be maximum when `x=R//sqrt(2)` and the maximum value of force will be given as
`F_(max)=(4pi^(2)G r^(2)rhom)/((3)^(3//2)R^(2))`
View SolutionA space station 960 m in diameter rotates fast enough that the artificial gravity at the outer edge is 1.5 g
(a) What is the frequency of rotation ?
(b) What is its period ?
(c) At what distance from the center will the artificial gravity be 0.75 g ? Take `g=10m//s^(2)`
View SolutionA chain AB of length `l` is located in a smooth horizontal tube so that its fraction of length `h` hangs freely and touches the surface of the table with its end B(figure). At a certain moment the end A of the chain is set free. With what velocity will this end of the chain slip out of the tube?

View SolutionAn electron with mass `9.1xx10^(-31)` kg moves with a speed of `2xx10^(6)m//s` in a circle of 2.85 cm radius under the influence of magnetic field. A protons of mass `1.67xx10^(-27)` kg moving in the same plane with the same speed, experiences the same centripetal force. What is the radius of the proton's orbit?
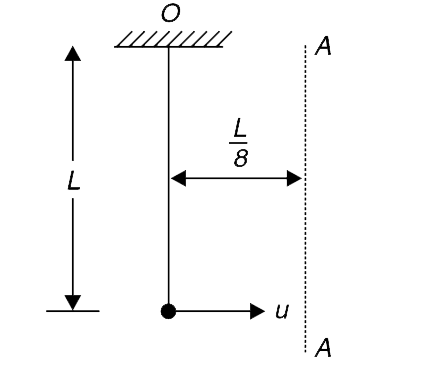
View SolutionA particle is suspended vertically from a point O by an inextensible mass less string of length L. A vertical line AB is at a distance of `L/8` from O as shown in figure. The particle is given a horizontal velocity u. At some point, its motion ceases to be circular and eventually the object passes through the line AB. At the instant of crossing AB, its velocity is horizontal. Find u.
View SolutionA cyclist intends to cycle up a `12^(@)` hill 100 m high Assuming the mass of bicycle plus person is 78.0 kg
(a) Calculate how much work must be done against gravity
(b) If each complete revolution of the pedals moves the bike 5.10 m along its path, calculate the average force that must be exerted on the pedals tangent to their circular path. Neglect friction and other losses. THe pedals turn in a circle of diameter 36 cm.
View SolutionA rod of length 1m and mass `0.5 kg` is fixed at one end is initially hanging vertical. The other end is now raised until it makes an angle `60^(@)` with the vertical. How much work is required ?
View SolutionIn a vertical circle, AB is the horizontal diameter Let AD and AE are two cords of the circle which subtend the angel `theta` and `2theta` at the centre of the circle respectively. If a particle slides along the two cords from A to D and A to E and the ratio of the time durations it take to travel the distances AD and AE is `1:n` then prove that :
`(n^(2)-1) costheta =1`
View SolutionA penny with a mass m sits on a horizontal turntable at a distance r from the axis of rotation. The turn table accelerates at a rate of `alpha rad//s^(2)` from t=0 The penny starts slipping at `t=t_(1)` The friction coefficient on the surface of turn table and the penny is `mu` find :
(i) The direction in which it starts sliding
(ii) The magnitude of frictional force at time `t=t_(2)(t_(2) gt t_(1))`
View SolutionA block of mass 5.0 kg is suspended from the end of a vertical spring which is stretched by 10 cm under the load of the block. The block is given a sharp impulse from below so that it acquires an upward speed of 2.0 m/s. How high will it rise? Take `g=10 m/s^2`
View SolutionA `20 kg` block is originally at rest on a horizontal surface for which the coefficient of friction is `0.6`. A horizontal force `F` is applied such that it varies with time as shown in the figure (a) & (b). If the speed of the block after `10 s` is `8v` then find `v`. ( Take `g=10m//s^(2)`)
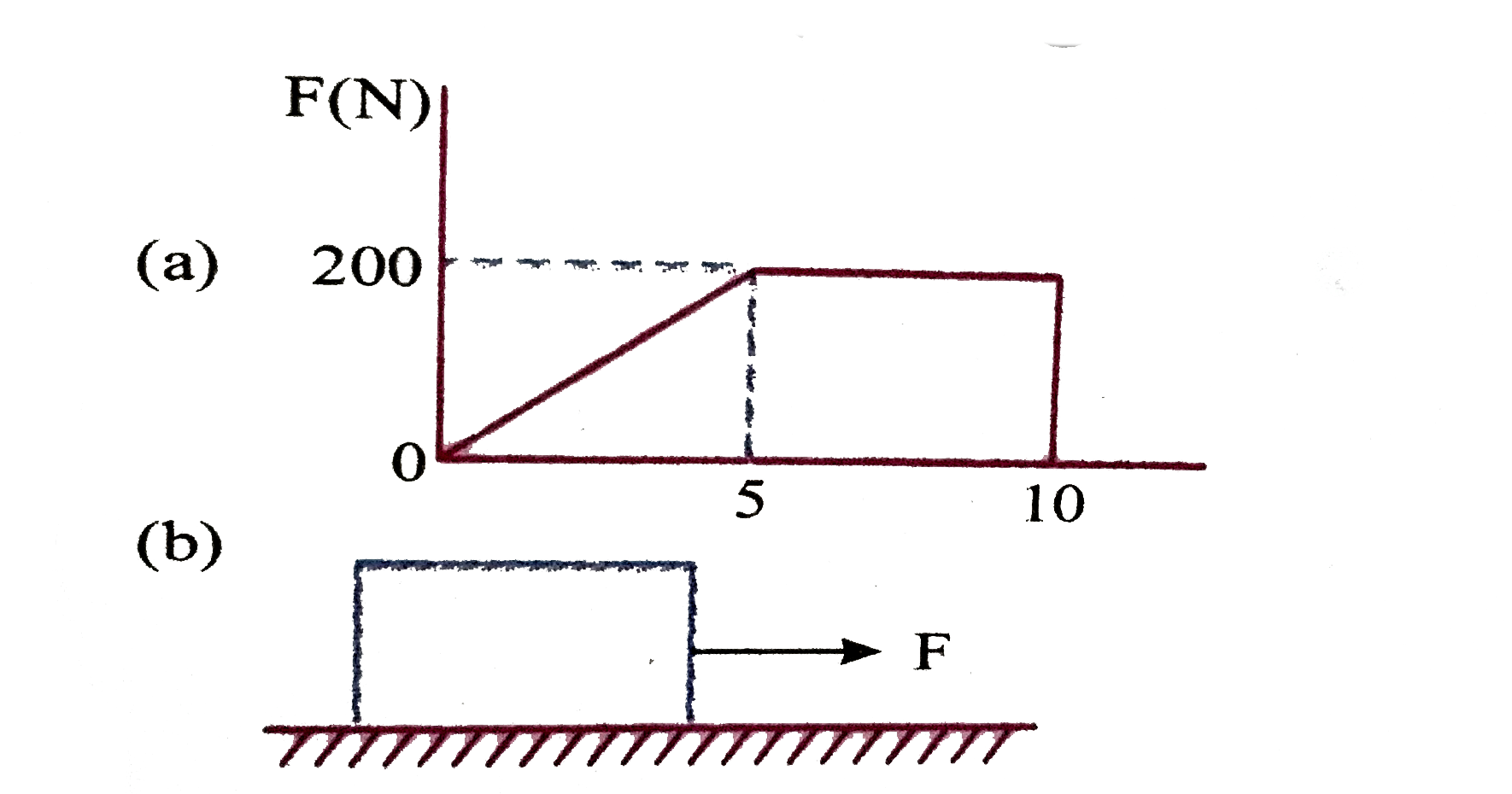
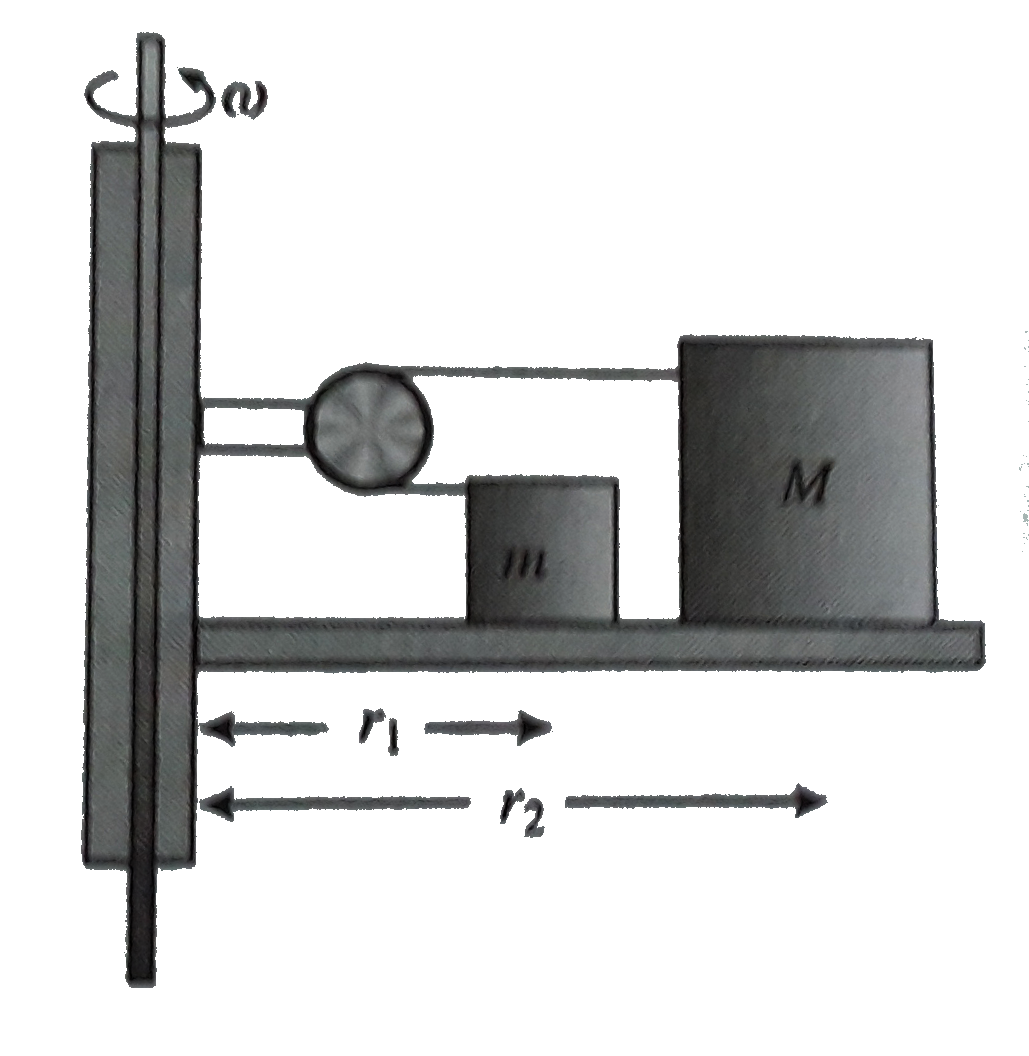
View SolutionTwo block of masses `m` and `M` are connected by a chord passing around a frictionless pulley which is attached to a rotating frame , which rotates about a vertical axis with an angular velocity `omega` If the coefficient of friction between the two masses and the surface are `mu_(1)` and `mu_(2)` respectively determine the value of `omega` at which the block starts sliding radially `(M gt m)`
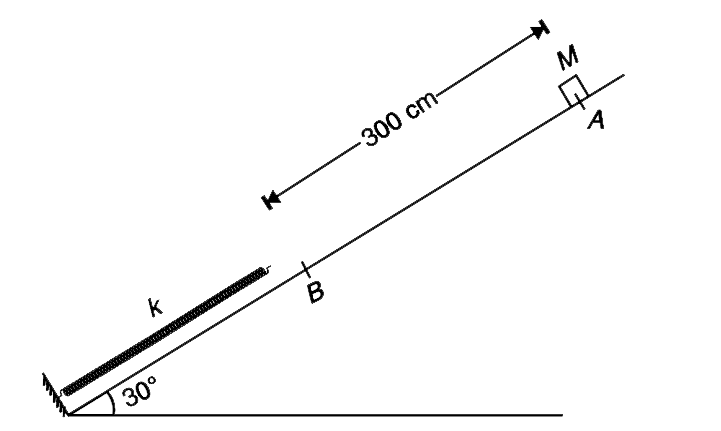
View SolutionA block of mass M = 40 kg is released on a smooth incline from point A. After travelling through a length of 30 cm it strikes an ideal spring of force constant `K = 1000 N//m` . It compresses the spring and then gets pushed back. How much time after its release, the block will be back to point A?
View SolutionA heavy particle of mass m is in motion on a smooth surface of hemisphere of radius R and there is no friction. At the initial instant the particle is at the topmost point A and has an initial velocity `v_(0)` At what point will the particle leave the surface of the hemisphere ? Also determine the value of `v_(0)` for which the particle will leave the sphere at the initial instant.
View Solution
unsolvedMCQ questions and complete solutions for chapter WORK, ENERGY AND POWER of GK PUBLICATIONS PHYSICS (HINGLISH) of Class 11