Complete Solutions to Magnetic Field Due To Current of chapter SOURCE AND EFFECT OF MAGNETIC FIELD of Class 12 book with complete answers and questions
| Class 12 | PHYSICS |
| Chapter | SOURCE AND EFFECT OF MAGNETIC FIELD |
| A2Z | Magnetic Field Due To Current |
| Number Of Questions | 44 |
| Academic Year | 2023-24 |
The magnetic field `dvecB` due to a small current element `dvecl` at a distance `vecr` and element carrying current `i` is,
View SolutionFor the magnetic field to be maximum due to a small element of current carrying conductor at a point, the angle between the element and the line joining the element to the given point must be
View SolutionA particle carrying a charge equal to `100` times the charge on an electron is rotating per second in a circular path of radius `0.8 metre`. The value of the magnetic field produced at the centre will be (`mu_(0)=` permeability for vacuum)
View SolutionAn electron is revolving round a proton, producing a magnetic field of `16 weber//m^(2)` in a circular orbit of radius `1 Å. Its angular velocity will be
View SolutionIn hydrogen atom, an electron is revolving in the orbit of radius `0.53 Å` with `6.6xx10^(15) rotations//second`. Magnetic field produced at the centre of the orbit is
View SolutionA neutral point is obtained at the centre of a vertical circular coil carrying current. The angle between the plane of the coil and the magnetic meridian is
View SolutionA vertical wire kept in `Z-X` plane carries a current from `Q` to `P` (see figure). The magnetic field due to current will have the direction at the origin `O` along
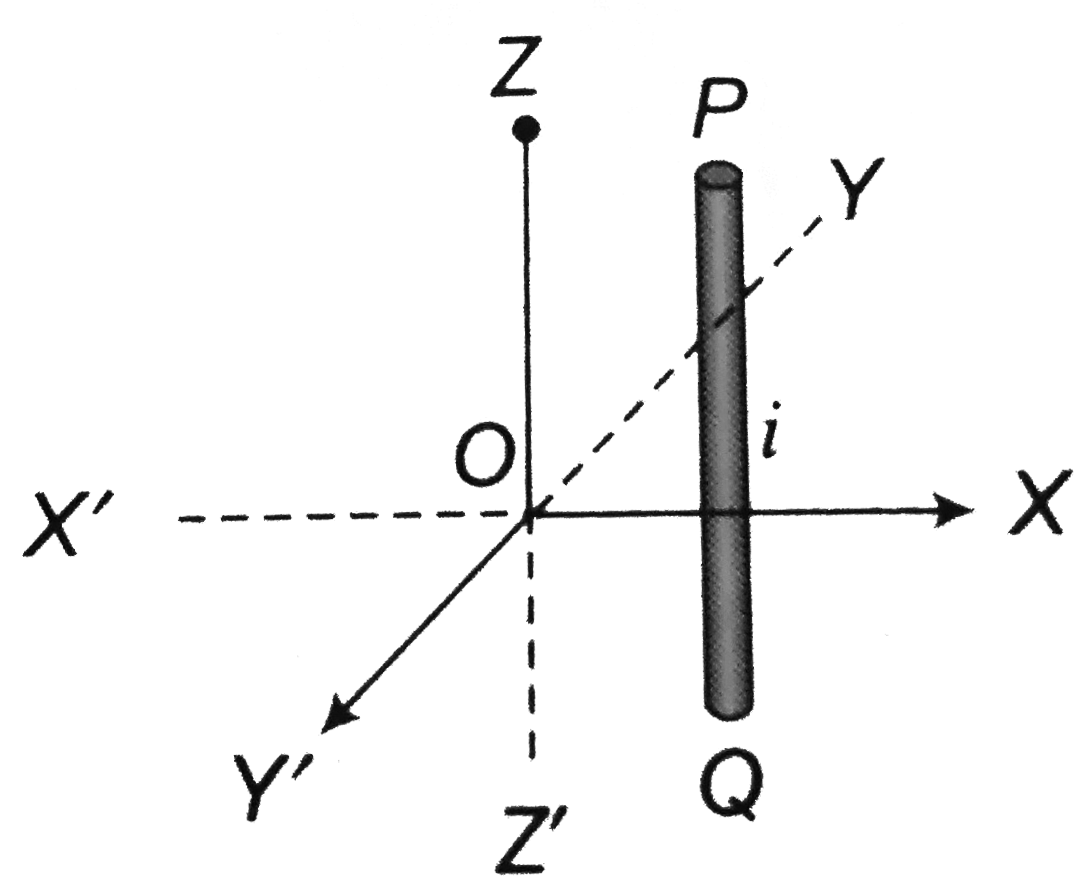
View SolutionA current flows in a conductor from east to west. The direction of the magnetic field at a points above the conductor is
View SolutionThe current is flowing in south direction along a power line. The direction of magnetic field above the power line (neglecting earth's field) is
View SolutionIn a hydrogen atom, an electron moves in a circular orbit og radius `5.2xx10^(-11)m` and produces a magnetic induction of `12.56 T` at it necleus. The current produced by the motion of the electron will be (Given `mu_(0)=4pixx10^(-7) W//bA-m`)
View SolutionA charge `+2q` moves vertically upwards with speed `v`, a second charge `-q` moves horizontally to the right with the same speed `v`, and a third charge `+q` moves horizonatlly to the right with the same speed `v`. The point `P` is located a perpendicular distance a away from each charge as shown in the figure. The magnetic field at point `P` is
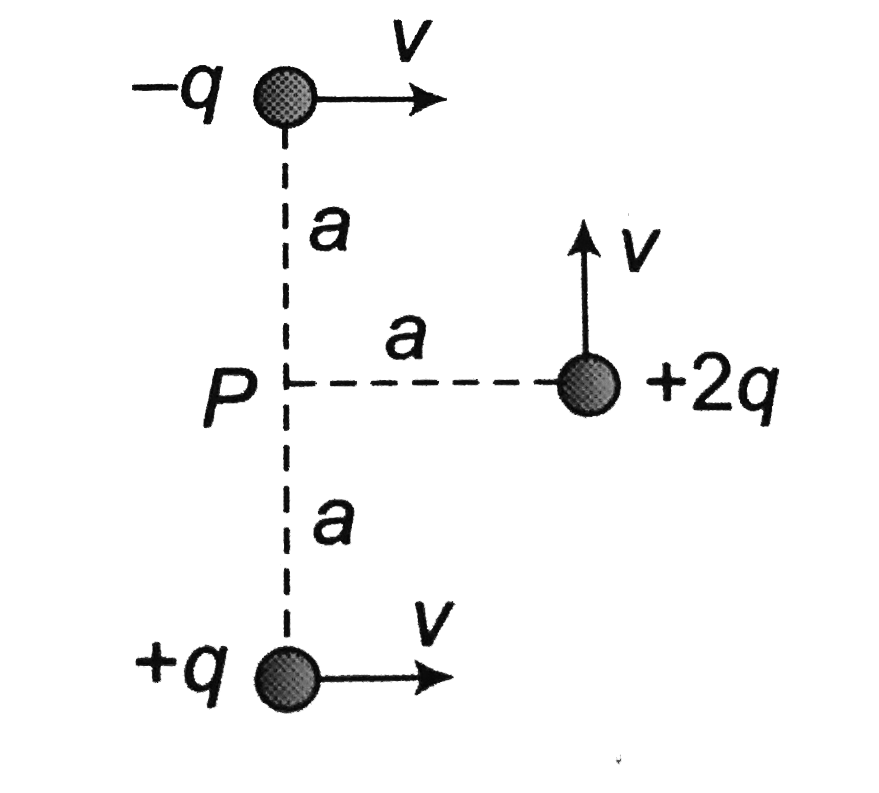
View SolutionA straight section `PQ` of a circuit lies along the `X`-axis from `x=-a/2` to `x=a/2` and carriers a steady current `i`. The magnetic field due to the section `PQ` at a point `X=+a` will be
View SolutionA long, straight wire carrying a current of 30 A is placed in an external, uniform magnetic field of `4.0 xx 10^-4` T exists from south to north.Find at a point 2.0 away from the wire.
View SolutionA long, straight wire carrying a current of 30 A is placed in an external, uniform magnetic field of `4.0 xx 10^-4` T exists from south to north.Find at a point 2.0 away from the wire.
View SolutionA long vertical wire carriers a current of `10` amperes flowing upwards through it at place where the horizontal component of the earth's magnetic induction is `0.3` gauss. Then the total magnetic induction at a point `5 cm` from the wire due magnetic north of the wire is
View SolutionA long vertical wire in which a current is flowing produces a neutral point with the earth's magnetic field at a distance of `5 cm` from the wire. If the horizontal component of the earth's magnetic induction is `0.18` gauss, then of the wire is
View SolutionA current of `5` flowing is passed through a straight wire of length `6 cm`, then the magnetic induction at a point `5 cm` from the either end of the wire is :
View SolutionA particle carrying a charge equal to `100` times the charge on an electron is rotating per second in a circular path of radius `0.8 metre`. The value of the magnetic field produced at the centre will be (`mu_(0)=` permeability for vacuum)
View SolutionTwo straight long conductors `AOB` and `COD` are perpendicular to each other and carry currents `I_(1)` and `I_(2)`. The magnitude of the magnetic induction at a point `P` at a distance `d` from the point `o` in a direction perpendicular to the plane `ABCD` is :
View SolutionTwo very thin metallic wires placed along X- and Y-axes carry equal currents as shown in Fig. AB and CD are lines at `45^@` with the axes. The magnetic field will be zero on the line
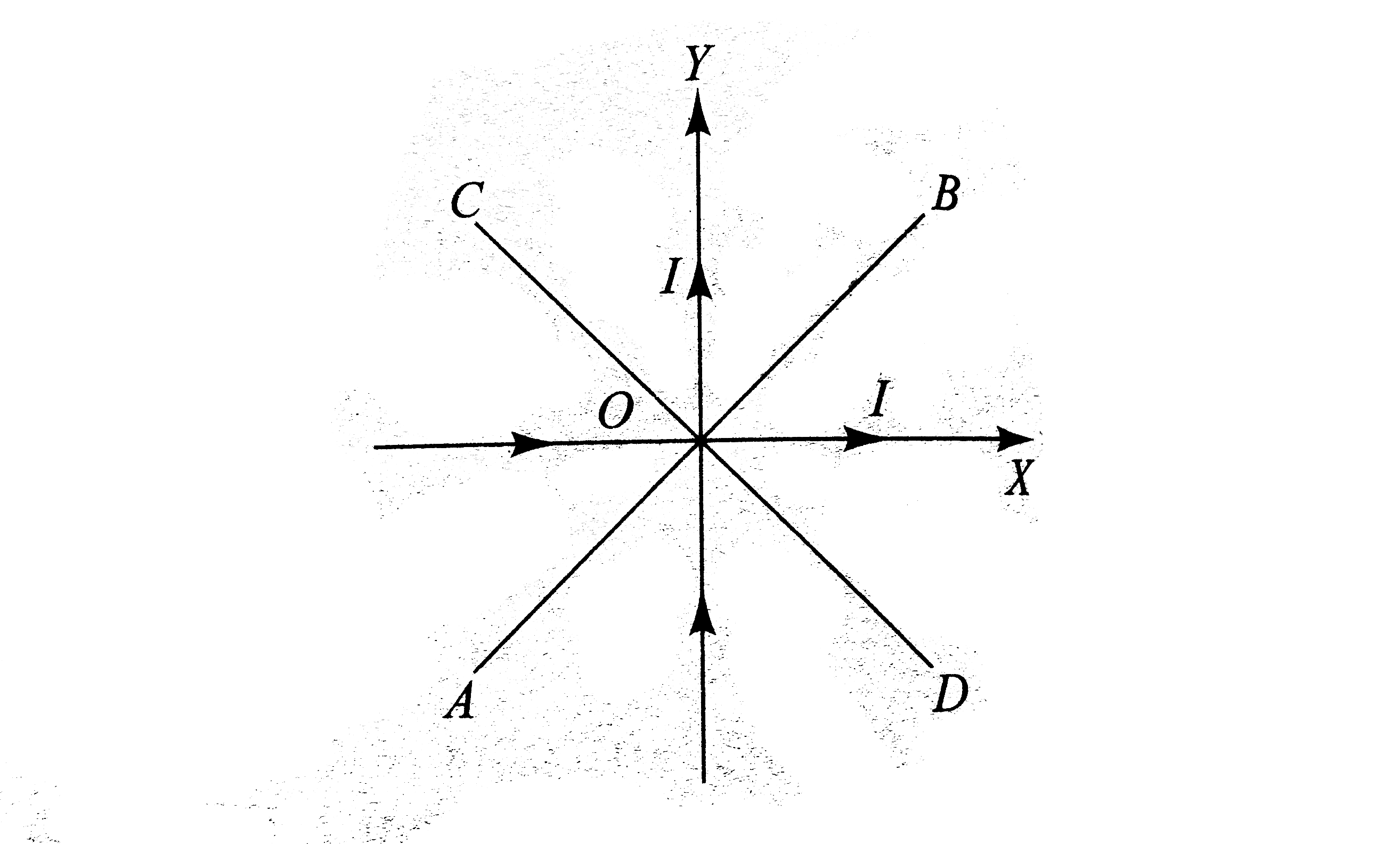
View SolutionIn fig. two long parallel wires carry equal currents in opposite directions. Point `O` is situated midway between the wires and `X-Y` plane contains the two wires and the position `Z`-axis comes normally out of the plane of paper. The magnetic field `B` at `O` is non-zero along
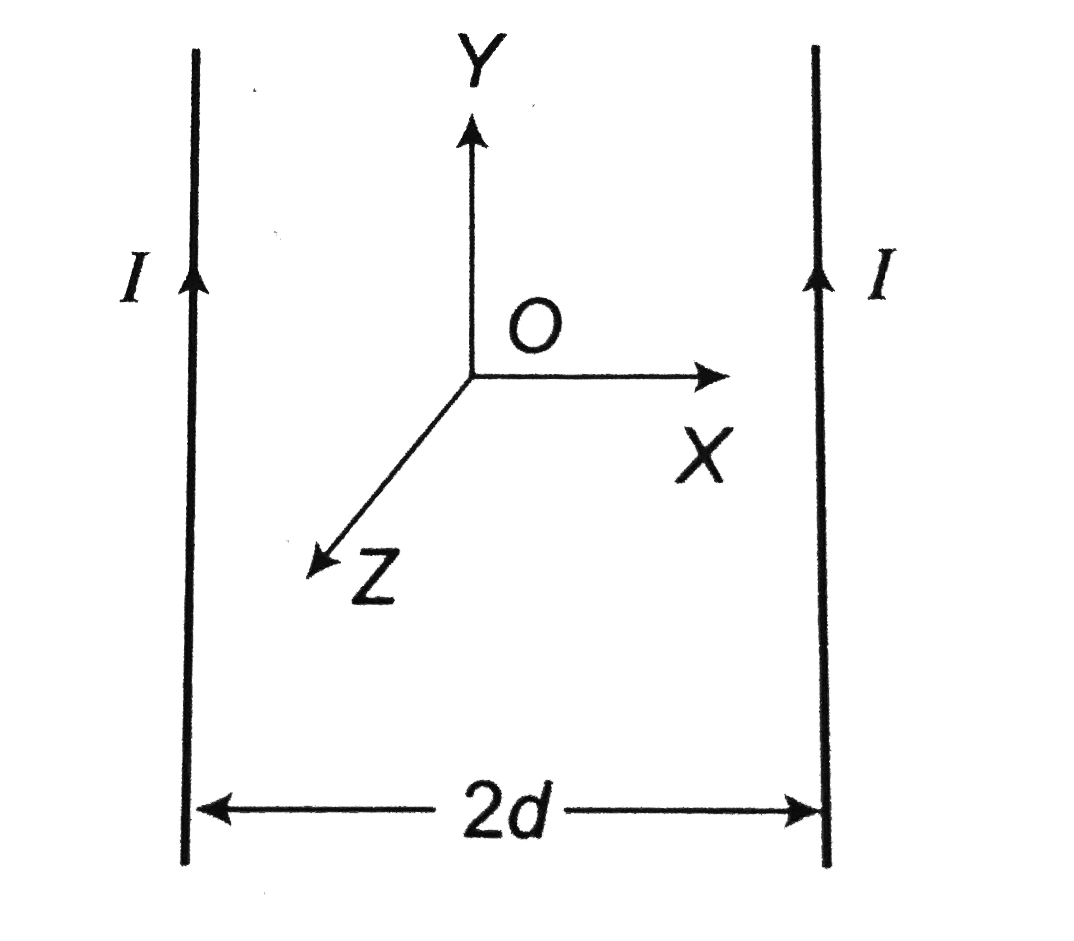
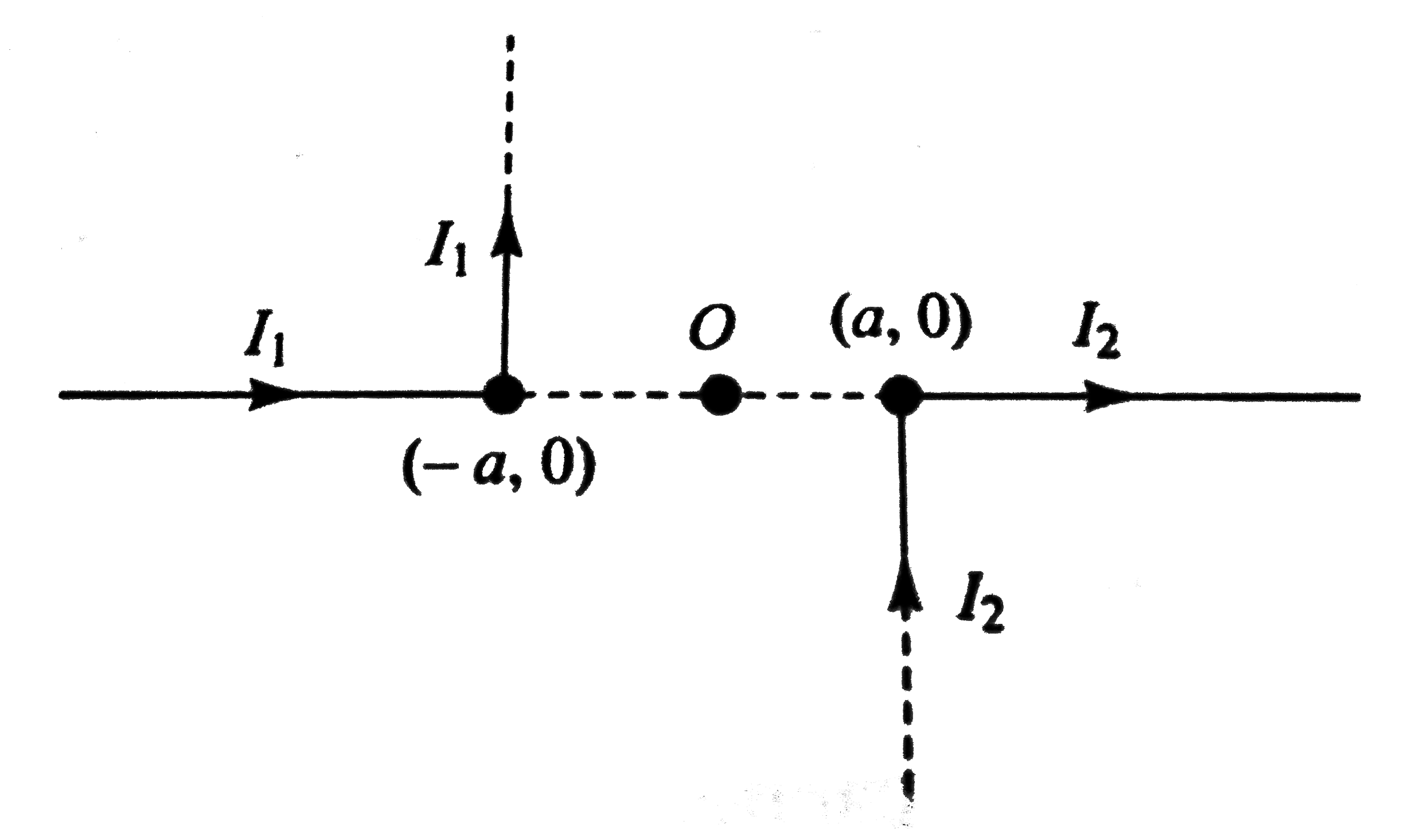
View SolutionCurrent `I_1 and I_2` flow in the wires shown in Fig. The field is zero at distance x to the right of O. Then
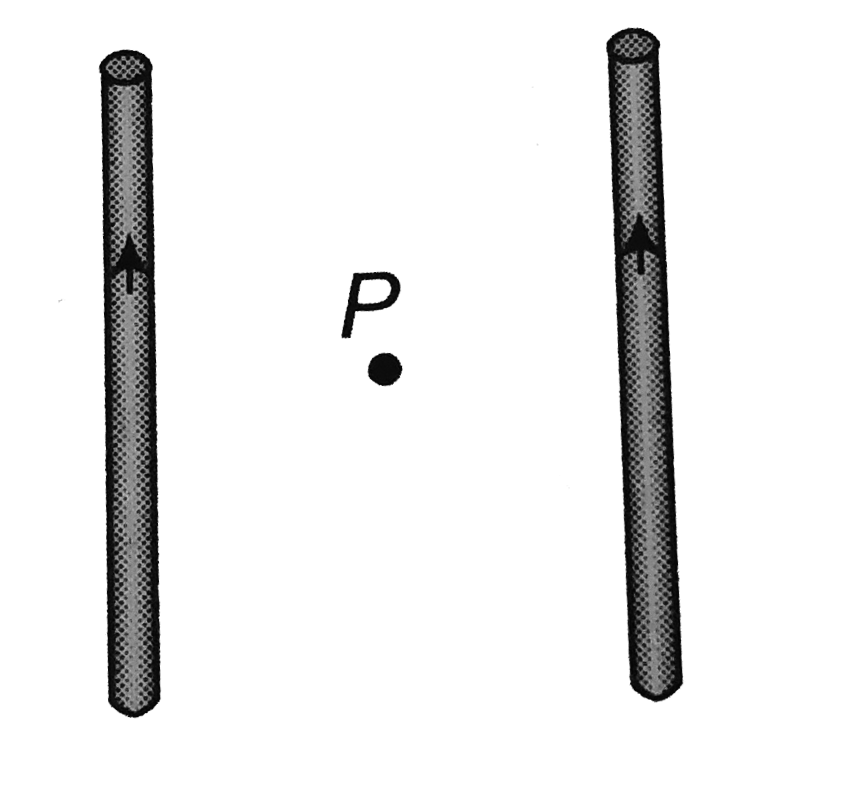
View SolutionTwo long straight wires are set parallel to each other. Each carriers a current `i` in the same direction and the separation between them is `2r`. The intensity of the magnetic field midway between them is
View SolutionTwo infinitely long, thin, insulated, straight wires lie in the x-y plane along the x- and y- axis respectively. Each wire carries a current I, respectively in the positive x-direction and positive y-direction. The magnetic field will be zero at all points on the straight line:
View SolutionA wire carrying current `I` and other carrying `2I` in the sam direction produce a magnetic field `B` at the midpoint. What will be the field when `2I` wire is swiched off?
View SolutionTwo long parallel wires `P` and `Q` are both perpendicular to the plane of the paper with distance `5m` between them. If `P` and `Q` carry current of `2.5` amp and `5` amp respectively in the same direction, then the magnetic field at a point half way between the wires is
View SolutionTwo parallel wire carrying equal currents in opposite directions are placed at `x=+-a` parralel to y-axis with `z=0`. Magnetic field at origin O is `B_1` and at `P(2a,0,0) is B_2`. Then, the ratio `B_1//B_2` is
View SolutionTwo parallel wires carrying equal currents `i_(1)` and `i_(2)` with `i_(1)gti_(2)`. When the current are in the same direction, the `10 mT`. If the direction of `i_(2)` is reversed, the field becomes `30 mT`. The ratio `i_(1)//i_(2)` is
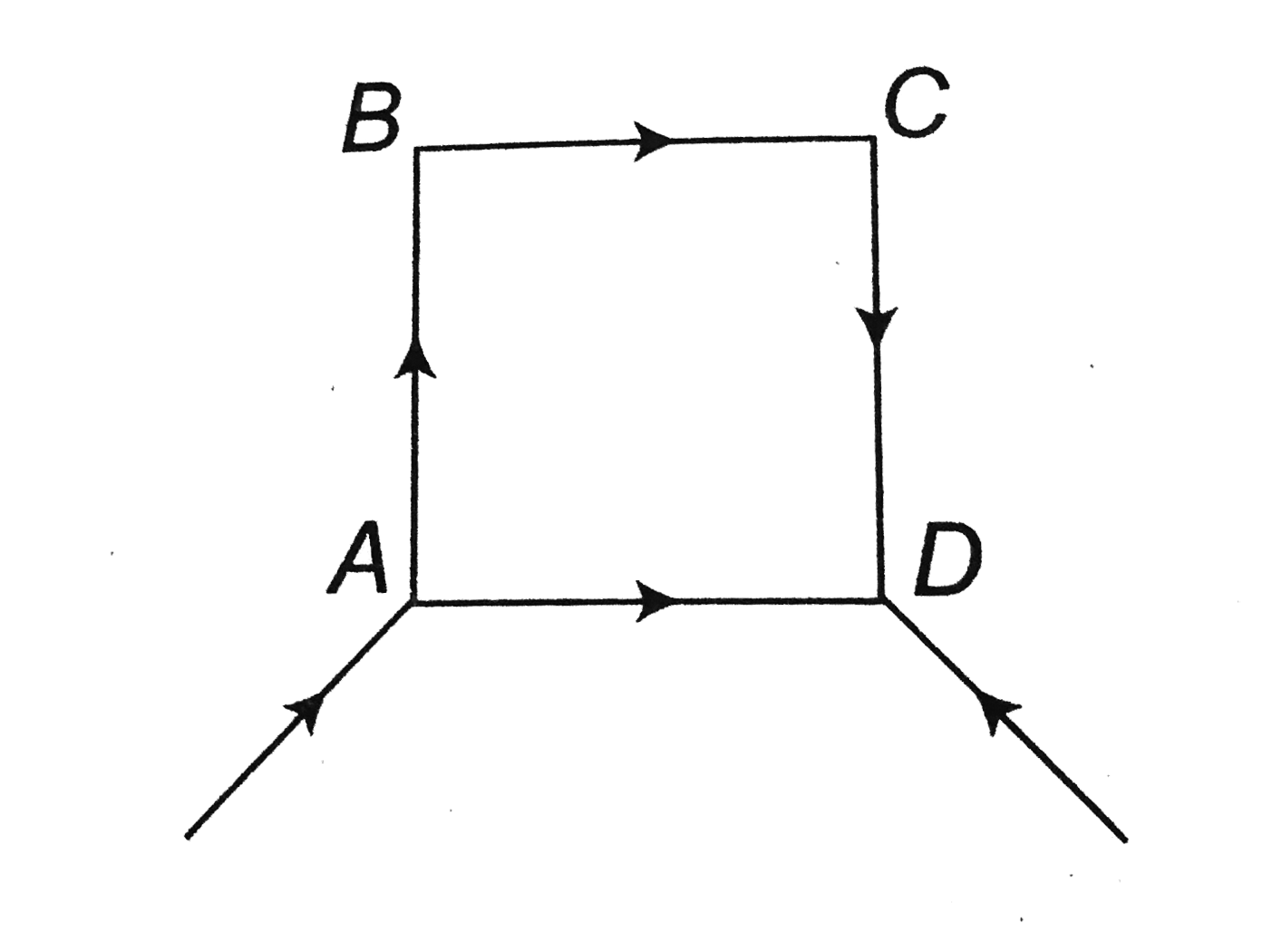
View Solution`ABCD` is a square loop made of a uniform conductivity wire. The current enters the loop at `A` and leaves at `D`. The magnetic field is
View SolutionA wire in the from of a square of side `'a'` carries a current `i`. Then the magnetic induction at the centre of the square wire is (Magnetic permeability of free space=`mu_(0)`)
View SolutionTwo long parallel wires are at a distance ` 2d` apart. They carry steady equal currents flowing out of the plane of the paper , as shown. The variation of the magnetic field `B` along the line `XX` is given by
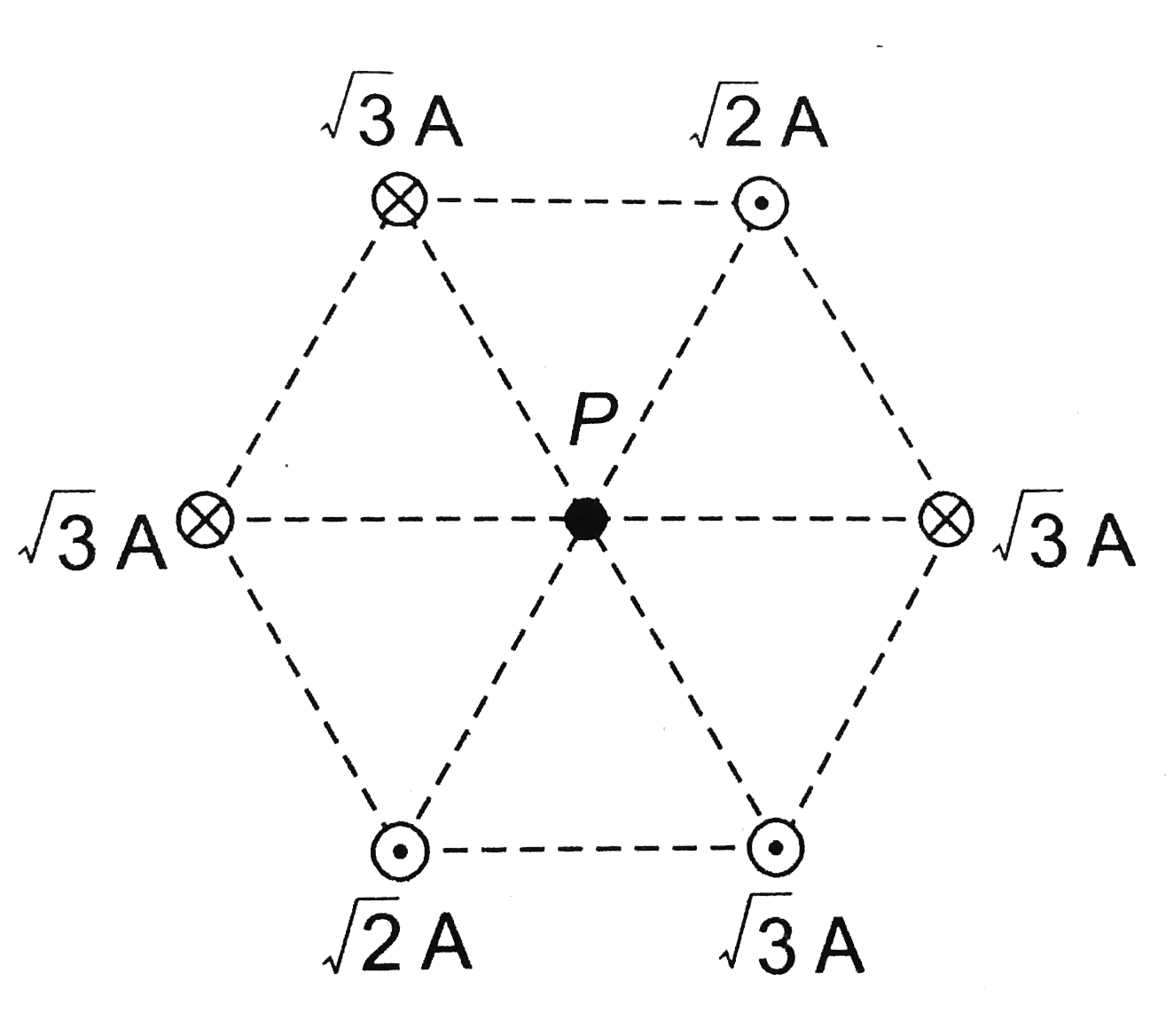
View SolutionConsider a set of six infinite long staright parallel wires arranged perpendicular to the plane of parper in a hexagon as shown. The length of the each side of the hexagon is `3 cm`. What is the magnitude and direction of the magnetic field at point `P`?
View SolutionA straight wire current element is carrying current `100A`, as shown in figure. The magnitude of magnetic field at point `P` which is at perpendicular distance `(sqrt(3)-1)m` from the current element if end `A` and end `B` of the element subtend angle `30^(@)` and `60^(@)` at point `P`, as shown, is:
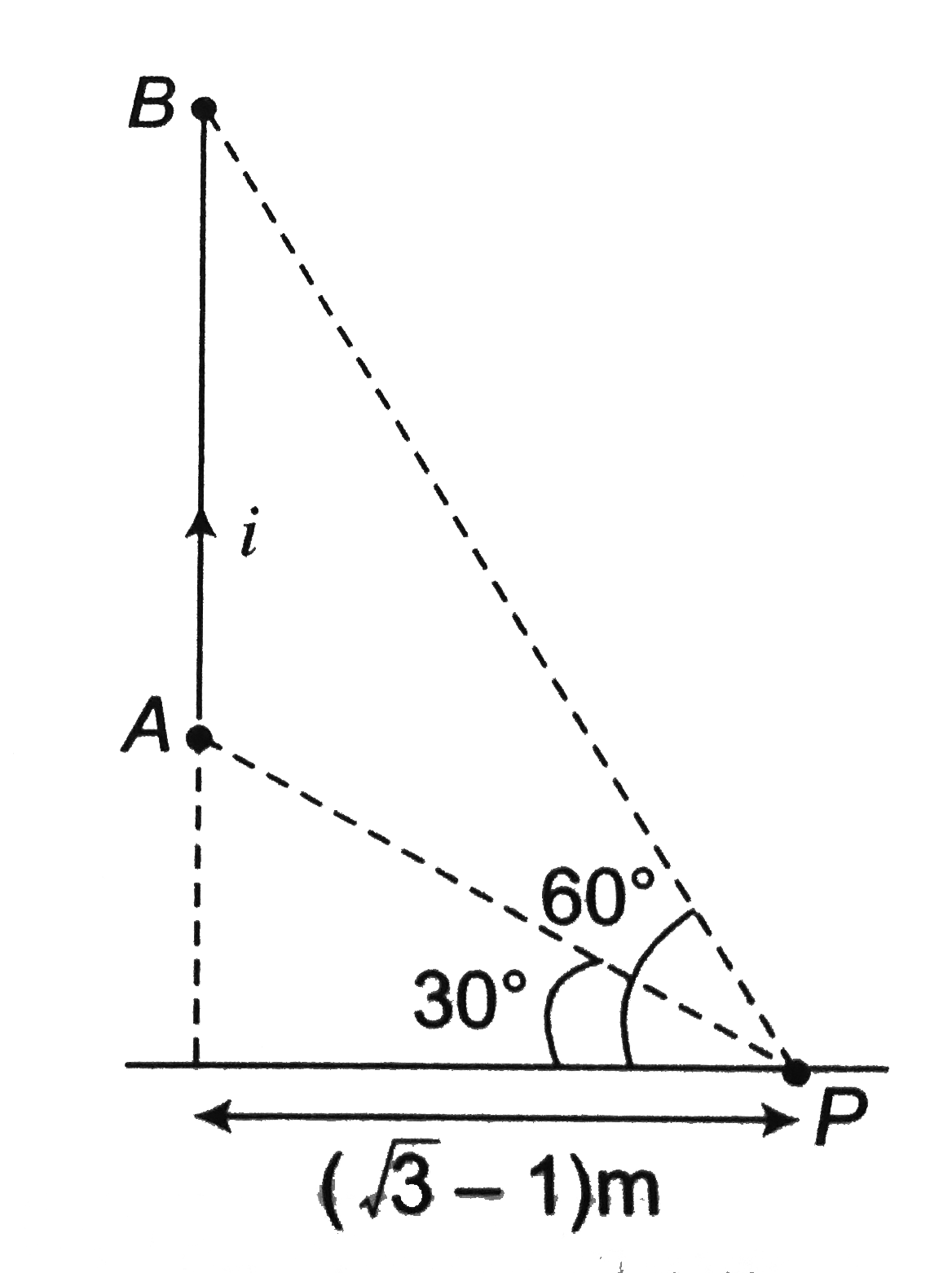
View SolutionThe magnetic field at the centre of an equilateral triangular loop of side 2L and carrying a current i is
View SolutionTwo wires AO and OC carry equal currents i as shown in Fig. One end of both the wires extends to infinity. Angle AOC is `alpha`. The magnitude of magnetic field is point P on the bisector of these two wires at a distance r from point O is

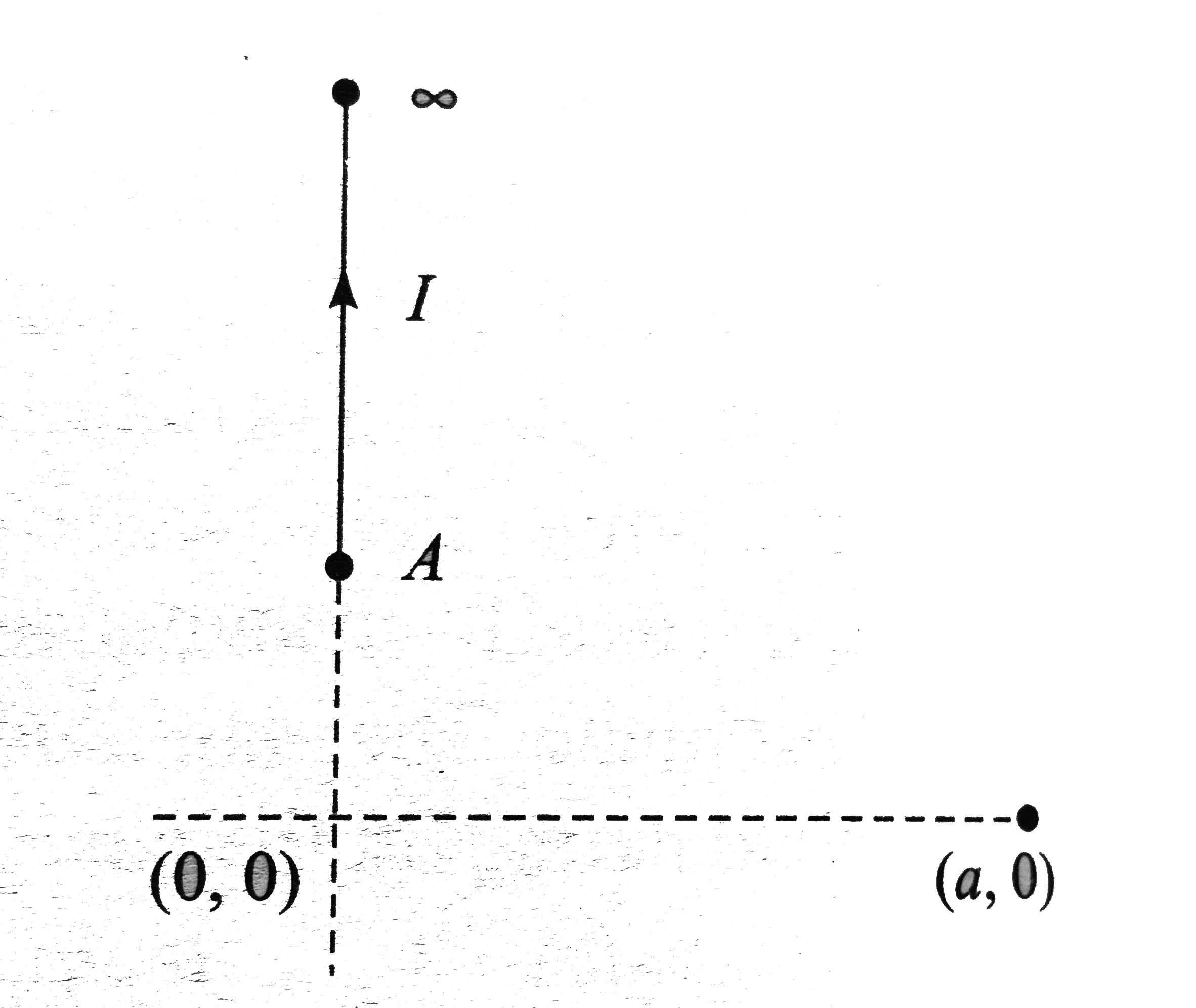
View SolutionAn infinitely long wire carrying current I is along Y-axis such that its one end is at point A(0,b) while the wire extends up to `+oo`. The magnitude of magnetic field strength at point (a,0) is
View SolutionThe magnetic field at O due to current in the infinite wire forming a loop as a shown in Fig.
View SolutionA current I flows through a thin wire shaped as regular polygon of n sides which can be inscribed in a circle of radius R. The magnetic field induction at the center of polygon due to one side of the polygon is
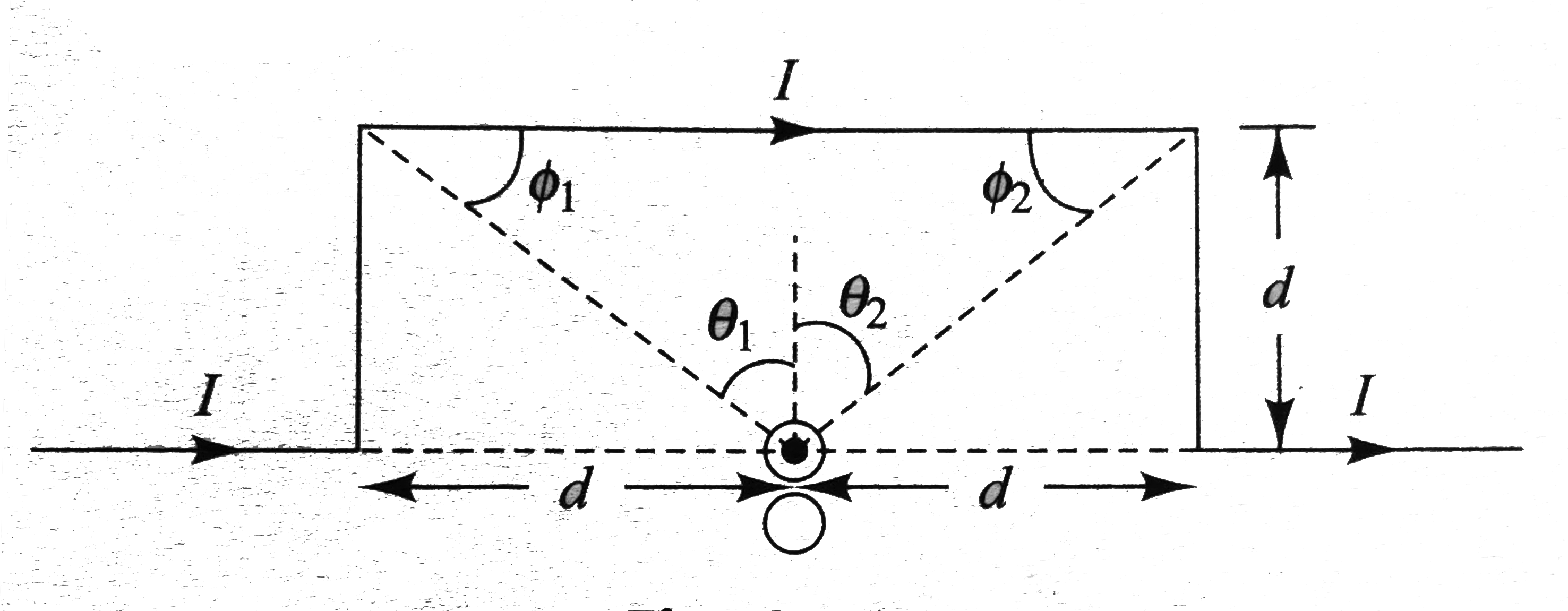
View SolutionTwo long wires `PQR` and `MNP` carry equal current `I`as shown such that `QR` and `NP` are parallel. Find the magnetic field at origin `O`.

View SolutionA circular current carrying coil has a radius R. The distance from the centre of the coil on the axis where the magnetic induction will be `(1//8)^(th)` of its value at the centre of the coil is,
View SolutionThe field normal to the plane of a wire of `n` turns and radis `r` which carriers `i` is measured on the axis of the coil at a small distance `h` from the centre of the coil. This is smaller than the field at the centre by the fraction.
View SolutionTwo straight long conductors `AOB` and `COD` are perpendicular to each other and carry currents `I_(1)` and `I_(2)`. The magnitude of the magnetic induction at a point `P` at a distance `d` from the point `o` in a direction perpendicular to the plane `ABCD` is :
View SolutionAn infinitely long conductor ` PQR` is bent to form a light angle as shown in Figure . A current `I` flows through `PQR` . The magnetic field due to this current at the point `M` is `H_(1)`. Now , another infinitely long straight conductor `QS` is connected at `Q` so that current is `(I)/(2)` in `QR` as well as in `QS` , the current in `PQ` remaining unchanged . The magnetic field at `M` is now `H_(2)`. The ratio `(H_(1))/(H_(2)` is given by
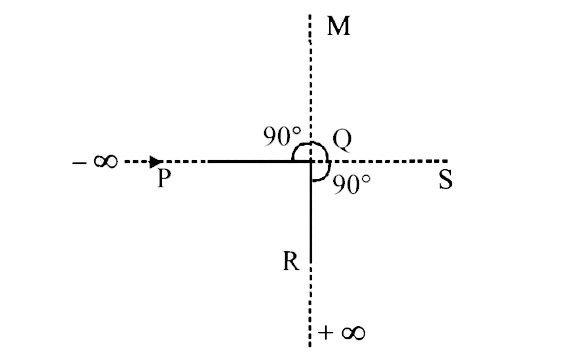
View SolutionA long straight wire along the `z`- axis carries a current ` I` in the negative ` z- direction` . The magnetic vector field ` vec(B)` at a point having coordinates (x,y) in the ` Z = 0` plane is
View Solution
Magnetic Field Due To Current questions and complete solutions for chapter SOURCE AND EFFECT OF MAGNETIC FIELD of A2Z PHYSICS (HINGLISH) of Class 12
All Exercises from A2Z Class 12 PHYSICS SOURCE AND EFFECT OF MAGNETIC FIELD
- AIIMS Questions
- AIPMTNEET Questions
- Assertion- Reason
- Force On A Current Carrying Conductor In Magnetic Field
- Magnetic Dipole
- Magnetic Field Due To Circular Arc
- Magnetic Field Due To Current
- Motion Of Charged Particle In Magnetic Field
- Problems Based On Mixed Concepts
- Section B - Assertion Reasoning
- Section D - Chapter End Test