Complete Solutions to Single Correct Answer Type of chapter PARABOLA of Class 12 book with complete answers and questions
| Class 12 | MATHS |
| Chapter | PARABOLA |
| CENGAGE NEW DPP | Single Correct Answer Type |
| Number Of Questions | 46 |
| Academic Year | 2023-24 |
The equation `x^(2) - 2xy +y^(2) +3x +2 = 0` represents
02:36View SolutionThe length of the latus rectum of `3x^(2) -4y +6x - 3 = 0` is
02:17View SolutionIn the adjacent figure a parabola is drawn to pass through the vertices B,C and D of the square ABCD. If `A(2,1),C(2,3)`, then focus of this parabola is
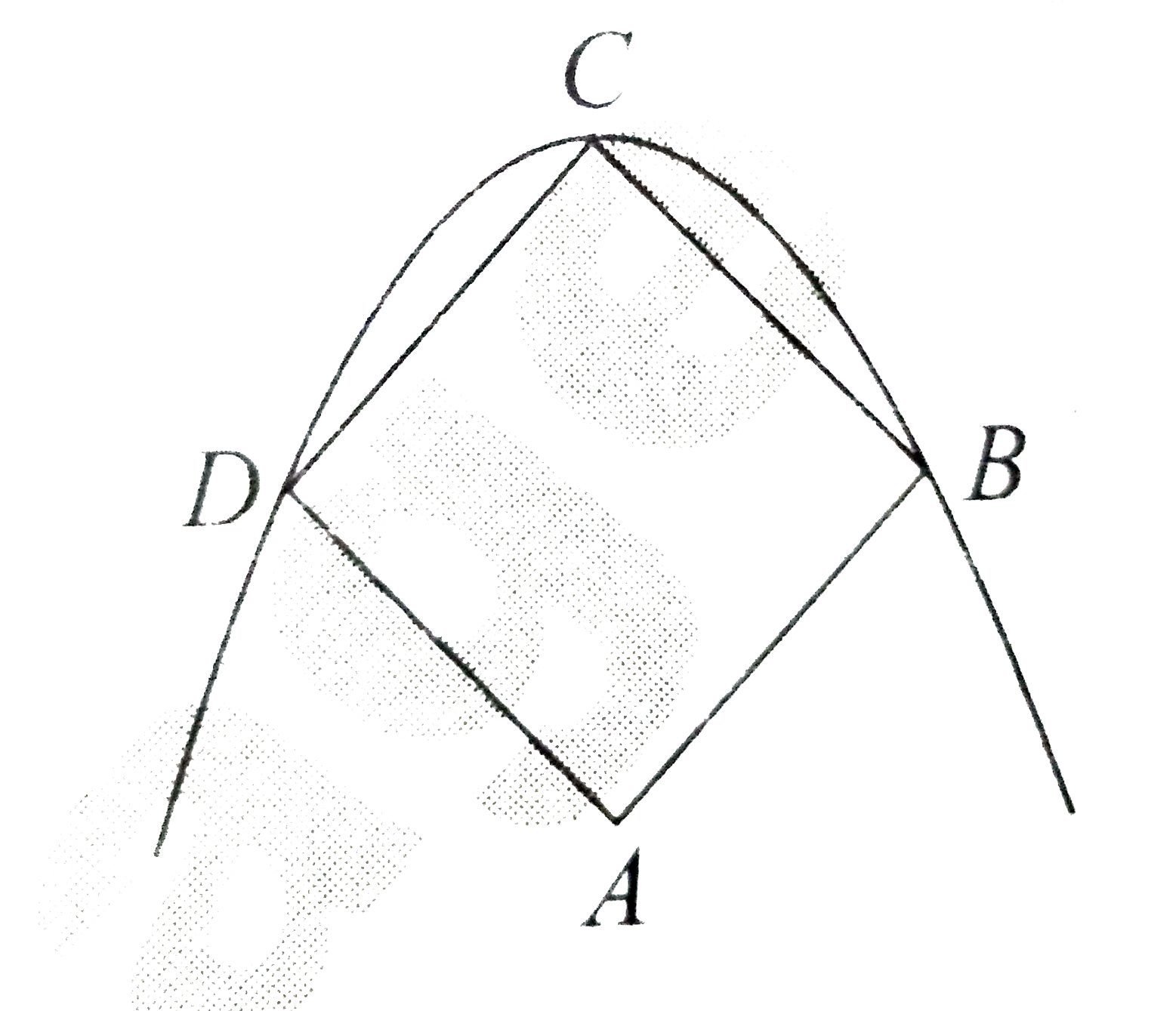 03:16View Solution
03:16View SolutionLength of the latus rectum of the parabola `sqrt(x) +sqrt(y) = sqrt(a)` is
03:25View SolutionConsider the parabola `x^(2) +4y = 0`. Let `P(a,b)` be any fixed point inside the parabola and let S be the focus of the parabola. Then the minimum value at `SQ +PQ` as point Q moves on the parabola is
View SolutionIf the points (2,3) and (3,2) on a parabola are equidistant from the focus, then the slope of its tangent at vertex is
01:14View SolutionLet `A(x_(1),y_(1))` and `B(x_(2),y_(2))` be two points on the parabola `y^(2) = 4ax`. If the circle with chord AB as a dimater touches the parabola, then `|y_(1)-y_(2)|` is equal to
View Solution`y = sqrt(3)x +lambda` is drawn through focus S of the parabola `y^(2)= 8x +16`. If two intersection points of the given line and the parabola are A and B such that perpendicular bisector of AB intersects the x-axis at P then length of PS is
03:27View SolutionIf the point `(2a,a)` lies inside the parabola `x^(2) -2x - 4y +3 = 0`, then a lies in the interval
03:35View SolutionIf AFB is a focal chord of the parabola `y^(2) = 4ax` such that `AF = 4` and `FB = 5` then the latus-rectum of the parabola is equal to
02:15View SolutionLength of the focal chord of the parabola `(y +3)^(2) = -8(x-1)` which lies at a distance 2 units from the vertex of the parabola is
01:40View SolutionLet `A (0,2),B` and C be points on parabola `y^(2)+x +4` such that `/_CBA (pi)/(2)`. Then the range of ordinate of C is
03:19View Solution`lx +my = 1` is the equation of the chord PQ of `y^(2) = 4x` whose focus is S. If PS and QS meet the parabola again at R and T respectively, then slope of RT is
View SolutionA line from `(-1,0)` intersects the parabola `x^(2)= 4y` at A and B. Then the locus of centroid of `DeltaOAB` is (where O is origin)
04:30View SolutionAll the three vertices of an equilateral triangle lie on the parabola `y = x^(2)`, and one of its sides has a slope of 2. Then the sum of the x-coordinates of the three vertices is
View SolutionFind the equations of the chords of the parabola `y^2= 4ax` which pass through the point (- 6a, 0) and which subtends an angle of `45^0` at the vertex.
View SolutionTwo equal circles of largest radii have following property:
(i) They intersect each other orthogonally,
(ii) They touch both the curves `4(y+2) = x^(2)` and `4(2-y) =x^(2)` in the region `x in [-2 sqrt(2),2sqrt(2)]`. Then radius of this circle isView SolutionA and B are two points on the parabola `y^(2) = 4ax` with vertex O. if OA is perpendicular to OB and they have lengths `r_(1)` and `r_(2)` respectively, then the valye of `(r_(1)^(4//3)r_(2)^(4//3))/(r_(1)^(2//3)+r_(2)^(2//3))` is
View SolutionA line `ax +by +c = 0` through the point `A(-2,0)` intersects the curve `y^(2)=4a` in P and Q such that `(1)/(AP) +(1)/(AQ) =(1)/(4)` (P,Q are in 1st quadrant). The value of `sqrt(a^(2)+b^(2)+c^(2))` is
05:44View SolutionSuppose a parabola `y = x^(2) - ax-1` intersects the coordinate axes at three points A,B and C, respectively. The circumcircle of `DeltaABC` intersects the y-axis again at the point `D(0,t)`. Then the value of t is
04:53View SolutionThe line `x - b +lambda y = 0` cuts the parabola `y^(2) = 4ax (a gt 0)` at `P(t_(1))` and `Q(t_(2))`. If `b in [2a, 4a]` then range of `t_(1)t_(2)` where `lambda in R` is
04:05View SolutionIf the parabola `y=(a-b)x^2+(b-c)x+(c-a)` touches x- axis then the line `ax+by+c=0` passes through a fixed point
04:11View SolutionA normal to parabola, whose inclination is `30^(@)`, cuts it again at an angle of
03:48View SolutionIf `(-2,5)` and (3,7) are the points of intersection of the tangent and normal at a point on a parabola with the axis of the parabola, then the focal distance of that point is
View SolutionThe angle of intersection between the curves `x^(2) = 4(y +1)` and `x^(2) =-4 (y+1)` is
02:40View SolutionThe parabolas `y^2=4ac` and `x^2=4by` intersect orthogonally at point `P(x_1,y_1)` where `x_1,y_1 != 0` provided (A) `b=a^2` (B) `b=a^3` (C) `b^3=a^2` (D) none of these
05:47View SolutionSum of slopes of common tangent to `y = (x^(2))/(4) - 3x +10` and `y = 2 - (x^(2))/(4)` is
04:52View SolutionThe slope of normal to be parabola `y = (x^(2))/(4) -2` drawn through the point `(10,-1)` is
View SolutionThe tangent and normal at the point `P(4,4)` to the parabola, `y^(2) = 4x` intersect the x-axis at the points Q and R, respectively. Then the circumcentre of the `DeltaPQR` is
View SolutionThe point on the parabola `y^(2) = 8x` at which the normal is inclined at `60^(@)` to the x-axis has the co-ordinates as
03:38View Solutionabout to only mathematics
01:01View SolutionFrom an external point P, a pair of tangents is drawn to the parabola `y^(2)=4x`. If `theta_(1)andtheta_(2)` are the inclinations of these tangent with the x-axis such that `theta_(1)+theta_(2)=pi//4`, then find the locus of P.
06:11View SolutionA variable parabola `y^(2) = 4ax, a` (where `a ne -(1)/(4))` being the parameter, meets the curve `y^(2) +x - 2 = 0` at two points. The locus of the point of intersecion of tangents at these points is
06:44View SolutionIf X is the foot of the directrix on the a parabola. PP' is a double ordinate of the curve and PX meets the curve again in Q. Then prove that P' Q passes through the focus of the parabola.
15:05View SolutionLet PQ be the latus rectum of the parabola `y^2 = 4x` with vetex A. Minimum length of the projection of PQ on a tangent drawn in portion of Parabola PAQ is
View SolutionThrough the vertex O of the parabola `y^(2) = 4ax`, a perpendicular is drawn to any tangent meeting it at P and the parabola at Q. Then OP, 2a and OQ are in
08:04View SolutionTangents PQ and PR are drawn to the parabola `y^(2) = 20(x+5)` and `y^(2) = 60 (x+15)`, respectively such that `/_RPQ = (pi)/(2)`. Then the locus of point P is
07:35View SolutionThe locus of centroid of triangle formed by a tangent to the parabola `y^(2) = 36x` with coordinate axes is
View Solution`PC` is the normal at P to the parabola `y^2=4ax, C` being on the axis. `CP` is produced outwards to disothat `PQ =CP` ; show that the locus of Q is a parabola.
View SolutionIf three parabols touch all the lines `x = 0, y = 0` and `x +y =2`, then maximum area of the triangle formed by joining their foci is
View SolutionIf `2x +3y = alpha, x -y = beta` and `kx +15y = r` are 3 concurrent normal of parabola `y^(2) = lambda x` then value of k is
03:32View SolutionLet `(2,3)` be the focus of a parabola and `x + y = 0` and `x-y= 0` be its two tangents. Then equation of its directrix will be
02:34View SolutionIn the following figure, `AS = 4` and `SP = 9`. The value of SZ is
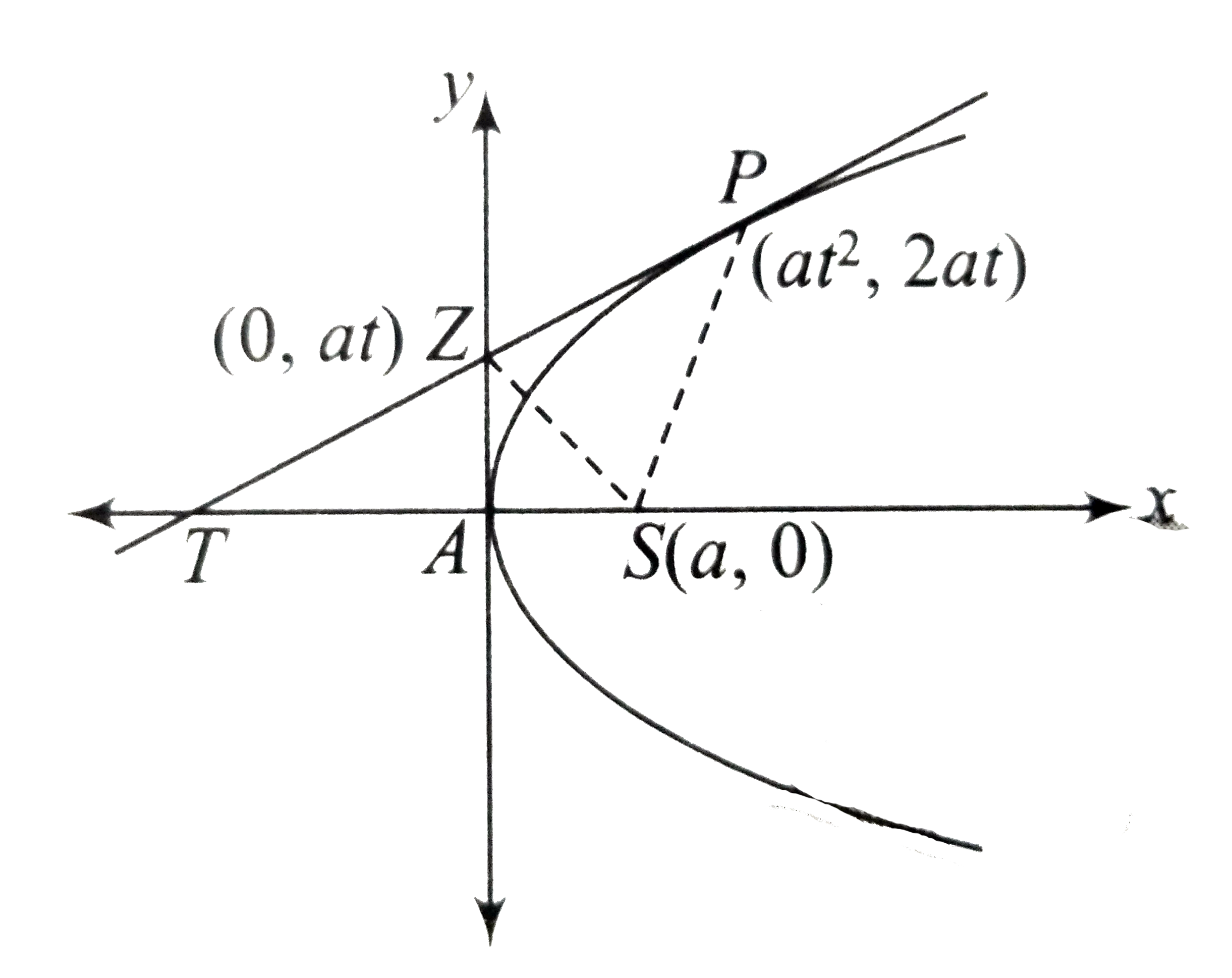 06:26View Solution
06:26View Solution`TP ` and `TQ ` are any two tangents to a parabola and the tangent at a third point `R` cuts them in `P'` and `Q'`. Prove that `(TP')/(TP)+(TQ')/(TQ)=1`
09:06View SolutionThe distance of two points P and Q on the parabola `y^(2) = 4ax` from the focus S are 3 and 12 respectively. The distance of the point of intersection of the tangents at P and Q from the focus S is
04:43View SolutionA parabola having directrix `x +y +2 =0` touches a line `2x +y -5 = 0` at (2,1). Then the semi-latus rectum of the parabola, is
08:08View Solution
Single Correct Answer Type questions and complete solutions for chapter PARABOLA of CENGAGE of Class 12
All Exercises from CENGAGE NEW DPP Class 12 MATHS PARABOLA
- Comprehension Type
- Examples
- Exercise (Comprehension)
- Exercise (Matrix)
- Exercise (Multiple)
- Exercise (Numerical)
- Exercise (Single)
- Exercise 5.1
- Exercise 5.2
- Exercise 5.3
- Exercise 5.4
- Exercise 5.5
- Exercise 5.6
- Exercise 5.7
- JEE Advenced Single Answer Type
- JEE Main Previous Year
- Matching Column Type
- Multiple Correct Answers Type
- Question Bank
- Single Correct Answer Type
